Abstract
Strontium-90 (90Sr) is the major long-lived radionuclide derived from the Chernobyl accident, and is still being detected in the heavily contaminated catchments of the Chernobyl Exclusion Zone. This study examines the long-term decrease in the dissolved-phase 90Sr concentration and the concentration–discharge (90Sr-Q) relationship in stream water since the accident. We show that the slow decline in 90Sr follows a double-exponential function, and that there is a clear relationship between 90Sr and Q. This study is the first to reveal that the log(90Sr)-log(Q) slope has been gradually decreasing since the accident. This trend persists after decay correction. Thus, it is not caused by the physical decay of 90Sr and environmental diffusion, but implies that the concentration formation processes in stream water have been changing over a long period. We propose a hydrochemical model to explain the time-dependency of the 90Sr-Q relationship. This paper presents a mathematical implementation of the new concept and describes the model assumptions. Our model accurately represents both the long-term 90Sr trend in stream water and the time-dependency of the 90Sr-Q relationship. Although this paper considers a small catchment in Chernobyl, the conceptual model is shown to be applicable to other accidental releases of radionuclides.
Similar content being viewed by others
Introduction
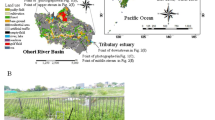
It has been 34 years since the Chernobyl nuclear power plant (CNPP) accident in Ukraine (Fig. 1), when large amounts of radionuclides were released into the environment. At present, high levels of dissolved-phase strontium-90 (90Sr) are still being detected in the small catchment streams inside the Chernobyl Exclusion Zone (CEZ) (Fig. 2b). The main long-term source of exchangeable and available 90Sr in the CEZ soils is micron-sized “fuel particles” (FP) that are gradually dissolving. These are derived from the accidental release from CNPP Unit 4 on 26 April, 1986, and were formed by the mechanical destruction of nuclear fuel. The dissolution rate of FP in CEZ soils varies from several percent to several tens of percent of the activity inventory per year, depending on the degree of oxidation of the particle UO2 matrix and the ambient geochemical conditions (e.g. soil solution pH and oxygen availability)1,2,3,4,5,6. The 90Sr from small streams in the CEZ is discharged into the main Pripyat river, which is a critical component of the Dnieper river–reservoir system, one of the largest surface water systems in Europe7. Through this river system, 90Sr is also transported to the Kiev metropolitan area in Ukraine. Therefore, predicting and evaluating the radionuclide flux within the water system, and using models to evaluate the potential radiological consequences to the downstream population, remain important tasks.
The annual-scale dissolved 90Sr concentration in the CEZ rivers is known to exhibit a rapid decrease in recently deposited activity followed by slower transfers during subsequent years, and this can be adequately expressed by an exponential function8,9,10. At the same time, the 90Sr concentration varies widely over the course of a year. For instance, the 90Sr concentration increases with increasing water levels in the Pripyat river during the snowmelt season11,12. The short-term temporal variations in 90Sr concentration in the CEZ rivers have been expressed using two- and three-dimensional numerical simulations13,14,15. The differential equations governing the water and sediment transport, and the calculation of radionuclide concentrations using the distribution coefficient (Kd) between sediment and water, have been used in short-term simulations.
The relationship between dissolved ions and/or other substances and the discharge rate (C-Q relationship) is a general phenomenon that has been widely observed for soluble ions and dissolved organic carbon in various river water systems worldwide16,17,18,19,20,21,22,23. The tight coupling between 90Sr and the stream discharge rate (Q) in CEZ rivers can be explained by the empirical and theoretical background and parametric modelling developed in previous hydrochemical studies24,25,26. Based on these studies, the variations in stream chemistry with respect to discharge can be explained by the variations in water and solute fluxes with respect to depth for a representative soil profile19,22. Here, the source of 90Sr observed in the CEZ rivers is the oxidized/non-oxidized FP released by the accident2,3,4,5. The 90Sr dissolved from the FP is thought to migrate into the soil before being transported by the water flux, finally reaching the river water. The difference between 90Sr in Chernobyl and the dissolved ions observed in other catchments is that the soil solute concentration profile (in this case, vertical 90Sr concentration profile in soil) changes over time. Thus, the 90Sr-Q relationship is assumed to change with time. Therefore, the goal of this study is to examine the long-term 90Sr concentration formation process by focusing on the 90Sr-Q relationship and to develop a conceptual model and mathematical implementation that explain the observed data in the field. The analysis was performed using long-term field measurement data collected biweekly from the Sakhan catchment in the CEZ (Fig. 1). The results of this study will not only inform better conceptual models for accident-derived radionuclide concentrations in rivers, but also provide new concepts regarding the dissolved ion concentration formation process in rivers.
Theory
Based on the clear relationship between 90Sr and Q from Chernobyl24,25,26, we assumed that the 90Sr-Q relationship in Chernobyl rivers could be described by the parametric C-Q model16,17,18,19,20,21,22,23. As the C-Q relationship, which is primarily determined by the solutes produced from mineral weathering/leaching, is governed by geochemical processes and climate, it is assumed to be in a steady state. It is also assumed that the radionuclide concentrations in the local streams are affected by weathering/leaching processes1,2,3,4,5 in the environment. For instance, the radioactive half-life of 90Sr is 28.79 years, which is too fast to assume a steady state in the C-Q relationship. Thus, the time series of observed dissolved 90Sr concentrations should be corrected by the long-term diminishing trend. The long-term diminishing trend of 90Sr concentrations (Cref: Bq/m3) in the stream is expressed as follows:
where t (days) is the number of days after the Chernobyl accident (26/4/1986), λ (1/yr) is the physical decay rate (= 0.024) of 90Sr, and α, β (Bq/m3), k1, and k2 (1/yr) are empirically determined (radionuclide-specific) constants. An exponential model from previous studies8,9,10 was used to describe the decrease in 90Sr concentrations over decadal time-scales. The constants α, β, k1, and k2 were set so as to minimize the root mean square error (RMSE) between the annual averaged values and the predicted values using the ‘optim’ package in the R software27. The annual averaged values were used to avoid excessive fluctuations from non-averaged data in the model validation stage.
Igarashi model
This is a new conceptual model that derives a C-Q relationship by developing the idea of a vertical distribution of the lateral flow of water across the vertical profile of soil water chemistry near the river19,22. The key assumption in our model is that the distribution of the lateral flow of soil22 and the vertical profile of radionuclides in the soil provides a stream discharge and determines the radionuclide concentrations in a stream. The radionuclide concentration profile in the soil is then represented by the following modified equation:
where c(z, t) is the concentration as a function of depth z and time t since fallout on the surface (26/4/1986). Cref is the long-term diminishing trend of 90Sr concentration, and d is a model coefficient. The z-axis has negative values below the ground surface, and intersects the ground surface at z0. Thus, the 90Sr concentration in the stream is defined by an integral expression, which can be solved analytically to give:
This type of power-law function also represents an empirical method of describing the log(C)-log(Q) relationship. It has been clarified mathematically that the relationship between 90Sr and Q in a stream obeys a log-log relationship. In this study, the model parameters were calibrated against observed 90Sr concentration data. The objective function implemented in the Markov chain Monte Carlo modelling is the standard log-likelihood function. Details can be found in the Supplementary Information.
Results
Long-term decline and seasonal fluctuation of 90Sr concentration in CEZ streams
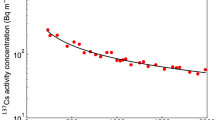
The long-term trends of 90Sr and Q are shown in Fig. 2(a,b). Unfortunately, data for Q and the 90Sr concentration were not available for the four years following the accident. Thus, we focused on the long-term time series in 90Sr and Q. Firstly, we found that the long-term data indicate large seasonal variations in Q (Fig. 2a). High values of Q were observed from March–May each year, which is defined as the snowmelt season, with the highest Q recorded on April 13, 2013 (Q = 4.2 mm/day). After the snowmelt season, Q began to decrease and stayed relatively low during the summer. The lowest Q was recorded between August 4 and December 5, 2015 (Q = 0.004 mm/day). 90Sr concentrations exhibit a long-term decline following a double-exponential function. We applied the exponential function to the 90Sr concentration data measured from 1990–2016 (Fig. 2b). The fitted parameters of the exponential model are α = 9357 (Bq/m3), β = 2500 (Bq/m3), k1 = 0.254 (1/yr), and k2 = 0.002 (1/yr). A large seasonality was also observed in the long-term decrease in 90Sr concentrations. High 90Sr values were observed during the snowmelt season, and 90Sr decreased during the summer. Here, the mean observed 90Sr concentrations in stream water decreased from ~3340 (Bq/m3) in the period 1990–1995 to ~1450 (Bq/m3) from 2012–2016. Using the observed 90Sr concentrations and Q, the wash-off of 90Sr through the stream discharge (from 1993–2016) was estimated to be ~5.0 (kBq/m2). This is only 1% of the initial 90Sr inventory across the whole watershed.
Response of 90Sr concentration to stream discharge rate
The relationship between 90Sr concentration and Q is shown in Fig. 3. A power-law function (y = px q) was fitted for each period (Fig. 3), and the parameter p was found to decrease according to the physical decay of 90Sr and diffusion in the environment; the power-law exponent q (slope of log(90Sr)-log(Q)) also decreased over time (Fig. 3, a1–a3). This study is the first to show the time-dependency of the 90Sr-Q relationship from long-term field observations in the Sakhan catchment. When the 90Sr concentration was corrected according to its long-term attenuation (90Sr/Cref), the constant parameter p was found to range from 1.41–1.73, but the power-law exponent q continued to decrease even after the attenuation correction (Fig. 3, b1–b3). This result means that the time-dependency of the 90Sr-Q relationship is not caused by the long-term attenuation of the 90Sr concentration, but by the changing concentration formation processes over a long period of time.
Response of dissolved-phase 90Sr concentrations (A) and 90Sr/Cref (B) to discharges rate. (1), (2), and (3) for each panel indicate the period used for aggregation, 1991–2000, 2001–2010, and 2011–2016, respectively. Grey dots indicate the observed values. Black dots and bars represent averages and quantiles for observed 90Sr, divided into 10 classes by the x-axis. Dashed lines express the best-fitted power-law regression lines for each period.
Discussion
Long-term decline of 90Sr concentration
The contamination of stream water by 90Sr continues to pose problems in the CEZ. Long-term monitoring and simple modelling are needed to ensure the safety of water usage in downstream areas and to further understand the dissolved 90Sr dynamics. For this study, we obtained data from continuous observations of dissolved-phase 90Sr concentrations and stream water discharges from 1990–2016 in the Sakhan catchment, located northwest of CNPP. We then applied a double-exponential model to the long-term 90Sr data. As shown in Fig. 2b, we were able to model the long-term decline in the 90Sr concentration. The best-fit parameter values for k1 and k2 were found to be 0.27 (1/yr) and 0.03 (1/yr), respectively. Note that our 90Sr data start from 1990, five years after the accident. In general, exponential functions for the decay of radionuclide concentrations in rivers are thought to consist of a fast “flush” of activity as a result of rapid wash-off processes, a slow decline as a result of soil fixation and redistribution processes, and a very-long-term “equilibrium” situation8,9. According to Sasina et al.10, the fast flush phase of 90Sr concentrations originating from the Chernobyl accident in Belarusian rivers lasted for about 0.04 years after the accident (there is a distance of about 90 km from CNPP to the nearest point). Thus, the fast flush of activity as a result of rapid wash-off processes would already have finished by the earliest monitoring year of 1990, and so the values obtained for k1 and k2 represent the slow decline as a result of soil fixation and redistribution processes and the very-long-term equilibrium situation. For 90Sr deposited in rivers by global fallout (e.g. from atmospheric nuclear weapons testing), the slow decay after the initial sharp drop in concentration is represented by a parameter value of 0.09 (1/yr)9. The long-term attenuation component calculated from a study of 90Sr in five Belarusian rivers was also estimated to be 0.09 (1/yr)10. From our results, the long-term reduction in 90Sr concentrations in the Sakhan catchment (k1 = 0.27 (1/yr)) is three times higher than the rates reported for other rivers only 90 km away. Other rivers in Belarus are also located in the Belarusian Chernobyl area, and it is unlikely that k has been affected by climatological differences. This finding may be attributed to the fact that the main source of 90Sr in the Sakhan catchment soils is non-oxidized FP that is dissolving relatively slowly;2,3,4,5 this forms the “Western trace” of Chernobyl contamination across the Sakhan watershed. The dissolution rate of 90Sr from non-oxidized FP is 7–10 times slower than from oxidized FP, and model estimates2 show that, in 2016, the main portion of radionuclides (up to 47% at pH = 6.0) was still within the particle matrix in the Western trace, whereas the basic portion of FP had dissolved by this time at other points. Therefore, the slow dissolution of FP (which has already disappeared from other basins) incorporating 90Sr in the Sakhan catchment could lead to the higher k value compared with the other regions mentioned above. Overall, k is an integral parameter that can be influenced by both hydrological run-off processes and geochemical factors (controlling the leaching of 90Sr from FP and redistribution in soils) in the catchment. Further studies are needed to clarify this issue.
90Sr-Q relationship and its time-dependency
An accurate description of the response of the 90Sr concentration to Q is another important mission in this study. The variation of 90Sr corresponded to increases in Q during the snowmelt season and subsequent decreases in summer (Fig. 2b). The recurring annual pattern in Q, which rises in the spring and falls in the summer, is clearly influenced by snow melting in spring11,25,26 and the increase in evapotranspiration in summer28. As for the 90Sr-Q relationship, studies have shown that 90Sr increases when the flow rate of the main stream of the Pripyat River increases during the snowmelt period14,15, and 90Sr concentration have also been found to increase with spring floods in small catchments under the influence of discharge levels25,26. Interestingly, the normalized surface wash-off coefficient for dissolved-phase 90Sr (Nl(90Sr)) during summer rainfall events is almost twice that of the snowmelt season1. This is because 90Sr exchange between wash-off liquid and topsoil cannot occur when the topsoil layer is frozen29. However, as reported in previous studies14,25,26, high 90Sr concentrations appear in high-Q periods (i.e. snowmelt season, heavy rainfall). Here, the discharge process could be the key to understanding the formation of solutes in the river. As shown by Freed et al.25,26, there is a clear correspondence between the stream flow and the groundwater level, and in the Chernobyl area, which is characterized by small differences in elevation within the basin, small increases in the groundwater level would cause large increases in the area of saturated soils. A relatively large contribution from the near-surface layer could then recharge the discharge level, leading to high dissolved-phase 90Sr concentrations in the snowmelt season. In addition, the effect of Nl(90Sr) on the 90Sr concentration in the river could be limited, because this region is too flat for the wash-off liquid to reach the river30. Frequent sampling could reveal a link between heavy rainfall events and 90Sr wash-off during the summer, therefore, requires further study.
Another key finding of this study besides the clear 90Sr-Q relationship is the time-dependency of the 90Sr-Q relationship from long-term field observations (Fig. 3a). It is also worth noting that the time-dependency of the 90Sr-Q relationship is preserved after the attenuation correction of 90Sr (Fig. 3b). Clearly, the log(90Sr/Cref)-log(Q) slope during the first 10-year period (1991–2000) is greater than that in the last six years (2011–2016). If the 90Sr concentration in the stream is determined by the interaction between water and solutes in the soil, the time-dependency of the 90Sr-Q relationship is presumably the result of a temporal change in the solute concentration in the soil19,22. This is because the water flow pathways in the soil do not change over timescales of a few decades. The solute concentration in the soil, which is the source of 90Sr in the stream water, is thought to have changed over time just after the accident. In fact, the vertical profile of the 90Sr concentration in the soil changes with time. This is the result of leaching from the initially deposited FP and subsequent downward movement in the soil1,31. Various previous studies have discussed the C-Q relationship. In particular, previous attempts to introduce parametric models and explain the C-Q relationship conceptually could help us to understand the time-dependency of the 90Sr-Q relationship.
New parametric model of 90Sr concentration
A previous hydrochemical study19 showed that the stream water chemistry can be accurately explained by a conceptual model that builds on the interaction of the vertical profiles of lateral water fluxes and soil solution chemistry. The mathematical implementation of this conceptual model provides an analytical solution, and a physical explanation as a power function, which is empirically used to describe the relationship between concentration and discharge22. Here, we propose a new conceptual hydrochemical model to explain the time-dependency of the 90Sr-Q relationship. The results of our model (Eq. (4)) are compared against measurements for 1990–2016 in Fig. 4a. Our model shows that the long-term diminishing trend of 90Sr is caused by the physical decay of 90Sr and diffusion in the environment, as well as the variation of 90Sr concentration corresponding to changes in Q. Table 1 reports the best fitting parameter with the range of credible values and the agreement between the modelled and observed 90Sr concentrations. Regardless of some uncertainty introduced by measurement errors, the model is sufficiently reliable to link 90Sr and Q for the purposes of this work (R2 = 0.66). Figure 4c shows the response of 90Sr to Q for three periods (C1: 1991–2000, C2: 2001–2010, C3: 2011–2016), and the red dashed lines show the model result for the middle of each period. Our model also adequately reproduces the time-dependency of the 90Sr-Q relationship for each of these periods (Fig. 4c). The key assumption in our model is that the distribution of the lateral flow of soil and the vertical profile of radionuclides in the soil provides a stream discharge and the radionuclide concentration in a stream. This assumption is supported by the following physical processes observed in the CEZ. During high-flow events (i.e. snowmelt, heavy rainfall), 90Sr is directly leached from the contaminated near-surface soil and/or top layer of floodplain soils in the CEZ14. The resulting high groundwater table produces lateral flow and increases the chances that more water will pass through the highly contaminated surface soil layer. This highly contaminated soil water enters streams and rivers, leading to an increase in both flow rate and 90Sr concentration in watercourses. Studies conducted in the Borschi watershed of the CEZ25,26 indicate that near-channel wetland areas can act as a source of 90Sr to surface water. In this study, we assumed that the 90Sr concentration profile in the soil is represented by an exponential function with a time component. Actually, the vertical profile of the 90Sr concentration in soil changes with time according to vertical migration1,31 and transport by the water flow25,26. It has been reported that 90Sr had accumulated in deeper areas (more than 10 cm deep) and was depleted nearer the surface (~5 cm depth) of wetland sediments near the stream some 14 years after the accident26. However, we also need to focus on the source of 90Sr in the catchment. As shown by Ivanov et al.32, the peak 90Sr concentration was on the soil surface in the Sakhan catchment, even 21 years after the accident. This is because the source of 90Sr in Sakhan is non-oxidized FP with a slow dissolution rate2,3,4,5. Thus, these previous studies support the use of Eq. (3) to represent the temporal changes in the vertical profile of the 90Sr concentration in soil. In addition, our model structure, in which the concentration converges to zero at low flow rates (Q → 0), is justified by the actual hydrological processes. This is because the source of the river water is assumed to be deep groundwater when Q is asymptotically close to zero, and the 90Sr concentration, which originates from FP, is assumed to be low, so the 90Sr concentration eluted from the groundwater can be assumed to be close to zero.
Time series of observed (black) and modelled values (red) of dissolved-phase 90Sr concentrations. Orange dots indicate the posterior predictive credible interval (A). The relationship between observed and modelled values of dissolved-phase 90Sr concentrations. The response of dissolved phase 90Sr concentrations to discharges rate for each period are also shown in (C1-3). Red dashed lines in (C) indicate the model result for the middle of each period.
This study is the first to show the long-term diminishing trend of the 90Sr concentration through the physical decay of 90Sr and diffusion in the environment, as well as variations in the 90Sr concentration corresponding to changes in the discharge rate. The mathematical implementation based on assumptions from previous studies enables us to describe the time-dependency of the 90Sr-Q relationship. If the solute concentration gradient in the soil involves hydrochemical processes in the river, our model can be applied to other radionuclides besides 90Sr. Finally, this study is the first to examine the relationship between dissolved-phase radionuclides and stream discharge from a hydrochemical perspective, and highlights the applicability of this scheme to other radionuclides in rivers.
Methods
Experiments were conducted at the Sakhan river in the CEZ (51.41°N, 30.00°E). This river lies within one of the sub-catchments of the Pripyat River, and has a total surface area of 186.9 km2 (Fig. 1). Annual rainfall in the CEZ is 500–600 mm/year. Monthly minimum and maximum temperatures are −6 °C and 19 °C, respectively. The catchment is largely covered by grassy meadow and wetlands. The forest area is occupied by coniferous and mixed forests33. The geological strata are composed of sandy quaternary deposits33. The topography is relatively flat. Local research institutes have been measuring the radionuclide concentrations in the local environment, including in soil and river water collected from various sites in the CEZ, over a 30-year period since soon after the CNPP accident. The 90Sr concentration and water discharge have been measured at the Sakhan river bridge monitoring station, located 7 km northwest of the nuclear power plant. The initial average 90Sr concentration in the soil was estimated to be 470.7 kBq/m2 (in 1986)5. The main long-term source of exchangeable and available 90Sr in the Sakhan catchment is non-oxidized FP2,3,4,5. The measurement data used in this study were obtained from two research institutes, the Ukrainian Hydrometeorological Institute (data period: 1990–1998) and the State Agency of Ukraine on Exclusion Zone Management and Chernobyl Ecocentre (data period: 1999–2016). Sampling was carried out in intervals of several weeks to several months in the initial phase. Continuous biweekly-interval sampling commenced in March, 1992. The 90Sr measurement error, available only for the period 1999–2016, was 10.9 ± 2.01% (mean ± S.D.). Water discharge data were obtained by integrating the cross-section observations of flow velocity and water depth from a concrete revetment under a bridge. To verify our estimates, flow rates were also measured directly using an electromagnetic current meter from March–May 2018, and the differences in the results were found to be insignificant.
References
Konoplev, A. V., Bulgakov, A. A., Popov, V. E. & Bobovnikova, T. I. Behavior of long-lived Chernobyl radionuclides in a soil-water system. Analyst 117, 1041–1047 (1992).
Kashparov, V. A. et al. Kinetics of fuel particle weathering and 90Sr mobility in the Chernobyl 30-km exclusion zone. Health Phys. 76, 251–259 (1999).
Kashparov, V. A. et al. Dissolution kinetics of particles of irradiated Chernobyl nuclear fuel: influence of pH and oxidation state on the release of radionuclides in the contaminated soil of Chernobyl. J. Nucl. Mater. 279, 225–233 (2000).
Kashparov, V. A. et al. Kinetics of dissolution of Chernobyl fuel particles in soil in natural conditions. J. Environ. Radioact. 72, 335–353 (2004).
Kashparov, V. A. et al. Spatial datasets of radionuclide contamination in the Ukrainian Chernobyl Exclusion Zone. Earth Syst. Sci. Data 10, 339–353 (2018).
Beresford, N. A. et al. Thirty years after the Chernobyl accident: What lessons have we learnt? J. Environ. Radioact. 157, 77–89 (2016).
IAEA: International Atomic Energy Agency, [Radioactivity in the Dnieper River Basin] Radiological Conditions in the Dnieper River Basin, Assessment by an international expert team and recommendations for an action plan, Radiological Assessment Reports Series. [15–20] (IAEA, Vienna, 2006).
Smith, J. T. et al. Global Analysis of the Riverine Transport of 90Sr and 137Cs. Environ. Sci. Technol. 38, 850–857 (2004).
Smith, J. T. et al. The “AQUASCOPE” simplified model for predicting 89,90Sr, 131I, and 134,137Cs in surface waters after a large-scale radioactive fallout. Health Phys. 89, 628–644 (2005).
Sasina, N. V. et al. “Blind” testing of models for predicting the 90Sr activity concentration in river systems using post-Chernobyl monitoring data. J. Environ. Radioact. 92, 63–71 (2007).
Voitsekhovitch, O. et al. Hydrological Processes and their influence on radionuclide behaviour and transport by surface water pathways as applied to water protection after Chernobyl accident. UNESCO Chernobyl Program Proceedings of an international Workshop, Paris. France P. 88 (1993).
Vakulovsky, S. M. et al. Cesium-137 and strontium-90 contamination of water bodies in the areas affected by releases from the Chernobyl nuclear power plant accident: an overview. J. Environ. Radioact. 23, 103–122 (1994).
Zheleznyak, M. J. et al. Mathematical modeling of radionuclide dispersion in the Pripyat-Dnieper aquatic system after the Chernobyl accident. Sci. Total Environ. 112, 89–114 (1992).
Zheleznyak, M. J. The mathematical modelling of radionuclide transport by surface water flow from the vicinity of the Chernobyl Nuclear Power Plant. Cond. Matter Phys. 12, 37–49 (1997).
Zheleznyak, M. J. et al. Simulation of countermeasures to diminish radionuclide fluxes from the Chernobyl zone via aquatic pathways. Radiat. Prot. Dosim. 73, 181–186 (1997).
Johnson, N. M. et al. A Working Model for the Variation in Stream Water Chemistry at the Hubbard Brook Experimental Forest, New Hampshire. Water Resour. Res. 5, 1353–1363 (1969).
Langbein, W. B., Dawdy, D. R. Occurrence of dissolved solids in surface waters. U.S. Geol. Survey Prof. Paper 501-D, USGS, Washington P. 116–117 (1964).
Bishop, K. H., Grip, H. & O’Neill, A. The origins of acid runoff in a hillslope during storm events. J. Hydrol. 116, 35–61 (1990).
Bishop, K. H. et al. Resolving the Double Paradox of rapidly mobilized old water highly variable responses in runoff chemistry. Hydrol. Process. 18, 185–189 (2004).
Godsey, S. E., Kirchner, J. W. & Clow, D. W. Concentration-discharge relationships reflect chemostatic characteristics of US catchments. Hydrol. Process. 23, 1844–1864 (2009).
Öquist, M. G. et al. Dissolved Inorganic Carbon Export Across the Soil / Stream Interface and Its Fate in a Boreal Headwater Stream. Environ. Sci. Technol. 43, 7364–7369 (2009).
Seibert, J. et al. Linking soil- and stream-water chemistry based on a Riparian Flow-Concentration Integration Model. Hydrol. Earth Syst. Sci. 13, 2287–2297 (2009).
Oda, T. et al. Effect of bedrock flow on catchment rainfall-runoff characteristics and the water balance in forested catchments in Tanzawa Mountains, Japan. Hydrol. Process. 27, 3864–3872 (2013).
Matsunaga, T. et al. Characteristics of Chernobyl-derived radionuclides in particulate form in surface waters in the exclusion zone around the Chernobyl Nuclear Power Plant. J. Contam. Hydrol. 35, 101–113 (1998).
Freed, R., Smith, L. & Bugai, D. Seasonal Changes of the 90 Sr Flux in the Borschi Stream, Chernobyl. Environ. Sci. and Pollut. Res. 1, 48–56 (2003).
Freed, R., Smith, L. & Bugai, D. The effective source area of 90Sr for a stream near Chernobyl, Ukraine. J. of Contamin. Hydrol. 71, 1–26 (2004).
R Development Core Team R: A language and environment for statistical computing. R Foundation for Statistical Computing, Vienna, Austria. ISBN 3-900051-07-0, URL, http://www.R-project.org (2008).
Didovets, I. et al. Assessment of Climate Change Impacts on Water Resources in Three Representative Ukrainian Catchments Using Eco-Hydrological Modelling. Water 9, https://doi.org/10.3390/w9030204 (2017).
Konoplev, A.V, Mobility and Bioavailability of Radiocesium and Radiostrontium of Chernobyl Origin (In Russian). DS Degree Thesis. RIARAE. (1998).
Igarashi, Y. et al. Impact of Wildfire on 137Cs and 90Sr Wash-off in Heavily Contaminated Forests in the Chernobyl Exclusion Zone. Environ. Pollut. 259, https://doi.org/10.1016/j.envpol.2019.113764 (2020).
Bulgakov, A. A. et al. Mechanisms of the Vertical Migration of Long-Lived Radionuclides in Soils within 30 Kilometers of the Chernobyl Nuclear Power Station. Sov. Soil Sci. 23, 46–51 (1991).
Ivanov, Y. A. et al. Long-term dynamics of radionuclide vertical migration in soils of the Chernobyl nuclear power plant exclusion zone. Printed at, http://sti.srs.gov/fulltext/SRNL-STI-2009-00770.pdf (2009)
Matoshko, A. et al. Sedimentological Study of the Chernobyl NPP Site to Schematise Radionuclide Migration Conditions. Environ. Geol. 46, 820–830 (2004). å
Acknowledgements
This work was supported by the Science and Technology Research Partnership for Sustainable Development JST-JICA, Japan (SATREPS project; PI. Kenji Nanba; JPMJSA1603) and the Institute of Geological Sciences Grant No.III-11-14 (from the Ukrainian National Academy of Sciences). We thank the staff of ECOCENTRE and UHMI in Ukraine for their assistance in conducting the fieldwork. We dedicate this study to the memory of our colleague and friend, Mr. Olexander Kadygrib, who contributed to this work from his great experience of Chernobyl. We thank Stuart Jenkinson, PhD, from Edanz Group (www.edanzediting.com/ac) for editing a draft of this manuscript.
Author information
Authors and Affiliations
Contributions
Y.I.: conception and design of the work; analysis of data. Y.O.: conception and design of the work; interpretation of data. J.S., S.O., S.K., V.D. and G.L.: provision of river data (core data of this work). H.L.: analysis of GIS and contamination map, D.B., A.K., M.Z., Y.W. and K.N.: interpretation of data. All authors reviewed the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Igarashi, Y., Onda, Y., Smith, J. et al. Simulating dissolved 90Sr concentrations within a small catchment in the Chernobyl Exclusion Zone using a parametric hydrochemical model. Sci Rep 10, 9818 (2020). https://doi.org/10.1038/s41598-020-66623-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-020-66623-4
This article is cited by
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.