Abstract
Advancement of optoelectronic and high-power devices is tied to the development of wide band gap materials with excellent transport properties. However, bipolar doping (n-type and p-type doping) and realizing high carrier density while maintaining good mobility have been big challenges in wide band gap materials. Here P-type and n-type conductivity was introduced in β-Ga2O3, an ultra-wide band gap oxide, by controlling hydrogen incorporation in the lattice without further doping. Hydrogen induced a 9-order of magnitude increase of n-type conductivity with donor ionization energy of 20 meV and resistivity of 10−4 Ω.cm. The conductivity was switched to p-type with acceptor ionization energy of 42 meV by altering hydrogen incorporation in the lattice. Density functional theory calculations were used to examine hydrogen location in the Ga2O3 lattice and identified a new donor type as the source of this remarkable n-type conductivity. Positron annihilation spectroscopy measurements confirm this finding and the interpretation of the experimental results. This work illustrates a new approach that allows a tunable and reversible way of modifying the conductivity of semiconductors and it is expected to have profound implications on semiconductor field. At the same time, it demonstrates for the first time p-type and remarkable n-type conductivity in Ga2O3 which should usher in the development of Ga2O3 devices and advance optoelectronics and high-power devices.
Similar content being viewed by others
Introduction
A wide band gap energy has become a key parameter for the future development of high-power transistors and optoelectronic devices1 and wide band gap oxides, such as ZnO, have been shown to exhibit excellent characteristics2. However, their deployment in many applications has been hindered due to the lack of conductivity control or the difficulty of realizing high carrier density with good mobility. Bipolar doping (realizing both n-type and p-type) is one of the big challenges in wide band gap materials but it is crucial for most devices2,3. Further, substitutional doping of elements, the common method to provide charge carriers, often causes disorder, suppressing carrier mobility and there is always a trade-off between increasing the maximum attainable carrier density and maintaining good mobility in oxides. In this work, we report how to induce p-type and n-type conductivity in an ultra-wide band gap oxide (Ga2O3) through controlling hydrogen (H) incorporation in the lattice without further substitutional doping and demonstrate a sheet carrier density of 1016 cm−2 with electron mobility 100 cm2 V−1 S−1 at room temperature leading to 10−4 Ω.cm resistivity. Such high electron density and good mobility is remarkable for oxide semiconductors. We identify a new donor concept behind this remarkable conductivity. While recent works reported room temperature electron mobility of 153 cm2/(Vs)4, 130 cm2/(Vs)5, and 176 cm2/(Vs)6 for unintentionally doped β-Ga2O3 grown by different techniques, the carrier concentrations were significantly low (<1017 cm−3) in these reports. The same trend is true for Si and Ge doped Ga2O37 where the carrier concentrations are often low for 100 cm2/(Vs) electron mobility. For p-type conductivity we report a hole density of 1015 cm−2, but with very low hole mobility less than 1 cm2 V−1 S−1, which is expected from the flat valence band of Ga2O3.
The study was carried out on β-Ga2O3, as it is emerging as a promising material for high power devices due to its large band gap (~4.5–5 eV) and high breakdown field of 8 MV/cm. It is receiving significant attention in the scientific community as a potential candidate for a wide range of applications8,9,10,11,12,13. β-Ga2O3 is the most stable polymorph of the Ga2O3 phases, with a monoclinic crystal structure of space group C2/m11. It behaves as an insulator in its defect free crystalline form. As of today, only one type of conductivity (n-type) has been achieved by doping β-Ga2O3 with Sn, Ge or Si during growth14,15,16,17. With respect to p-type conductivity, there has not been any significant success. Only deep acceptors (with activation energy >1 eV) for undoped and doped samples have been reported where acceptor ionization was critically low at room temperature18,19.
Hydrogen is known to have a strong influence on the electrical conductivity of semiconductors20. In β-Ga2O3, monoatomic H has a low formation energy and can occupy both interstitial and substitutional sites to act as a shallow donor21. The complex crystal structure of β-Ga2O3 allows for the formation of many configurations where interstitial hydrogen (\({{\rm{H}}}_{i}^{+}\)) forms a bond with oxygen, creating electronic states which are close in energy. According to J. Varley et.al.13, Hi acts as a shallow donor, although not stable and substitutional hydrogen, HO, has low formation energy only under oxygen poor condition. Despite these theoretical predictions on the possibility of n-type conductivity due to H-incorporation in various locations, there has not been any report on significant experimental success. In this work, we generate H-donors and H-acceptors in Ga2O3 by controlling H incorporation on cation vacancy sites, not as Hi or HO. A cation vacancy is an electrical compensating acceptor in semiconductors including β-Ga2O322. Although cation vacancies have high formation energy in some oxide semiconductors (e.g. SnO2, In2O3), previous first principle calculations showed that their formation energy is significantly lower in β-Ga2O3 and hence a high probability of H-decorated VGa formation can be achieved after incorporating H into the crystal22,23.
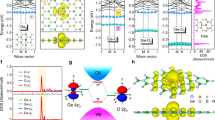
It is necessary to understand the interaction of H2 with the surface of metal-oxide semiconductors to gain insight on the process of H-incorporation into the crystal. H-incorporation into the crystals at high temperature occurs in two steps. At first, H2 dissociates and becomes attached to the surface, then diffuses into the bulk crystal. Depending on the nature of the materials, H2 can follow either homolytic or heterolytic dissociation pathways. In case of homolytic cleavage, H2 molecule dissociates to form two H-atoms that become attached to the oxygen on the crystal surface. On the other hand, H2 dissociates to form a proton and a hydride during heterolytic cleavage where the proton and hydride become attached to the oxygen and metal atoms respectively. The redox capacity of metals determines the type of dissociation that is most likely to occur. Density functional theory (DFT) predicts that H2 tends to dissociate heterolytically on nonreducible oxide (e.g MgO, γ-Al2O3) surfaces while following a homolytic pathway on reducible oxide (e.g. CeO2) surfaces24. β-Ga2O3 was found to be nonreducible via DFT25. Therefore, it is most likely that H2 follows heterolytic dissociation as shown in Fig. 1a. The adsorbed proton and hydride diffuse into the bulk crystal at high temperatures. The proton is attracted toward the negatively charged VGa while the hydride is attracted toward the positively charged or neutral VO, as shown in Fig. 1b.
Schematic diagrams showing hydrogen incorporation in β-Ga2O3 (a) hydrogen molecules coming in contact with the surface at elevated temperature and dissociating heterolytically. The electron cloud of H2 is attracted toward gallium while the proton is attracted toward oxygen. (b) The proton and hydride ion are attached to oxygen and gallium atoms, respectively, on the crystal surface and diffuse through the bulk crystal at high temperatures. The proton is attracted toward the negatively charged gallium vacancy. (c) Ga vacancy decorated with two hydrogen as predicted from DFT calculations providing stable acceptor state (d) Ga vacancy decorated with four hydrogen as predicted from DFT calculations providing stable donor state.
Results and Discussions
Electrical parameters of the samples measured by Hall measurement system are shown in Table 1. The details of the measurements are given in the method section. Table 1 shows that the as-grown samples were highly resistive, but after H2 diffusion they showed an increase in carrier density and p-type conductivity. H2 diffusion at 700 °C for 1 hr led to unstable conductivity that decays with time (Table 1a). However, H2 diffusion at 950 °C for 2 hrs led to a greater increase in carrier density and stable p-type conductivity over time (Table 1b). Sheet carrier density of 1015 cm−2 was achieved but hole mobility lower than 1cm2/VS was measured which is expected due to the flat band nature of valence band made of predominately O 2p states. Other procedures were carried out to incorporate H2 into different sites in the undoped β-Ga2O3. One sample was annealed in O2 flow and another was annealed with Ga in a closed ampoule at 950 °C for 2 hrs. This process should fill up the respective (anion or cation) vacancies. After that, hydrogen was diffused into the crystals at 580 torr in a closed ampoule at 950 °C for 2 hrs. O2 –annealing followed by H2 diffusion led to high n-type conductivity (stable over time) and remarkable sheet carrier density of about 1016 cm−2 with electron mobility 100 cm2/Vs (Table 1c). The thickness of the conductive layer where H diffuses in is 500 nm as revealed from depth resolved positron measurements in Fig. 2a. This indicates a remarkable conductivity of 10−4 Ω.cm. The sample exhibits 9-orders of magnitude increase in conductivity and 10-orders of magnitude increase in carrier density. In contrast, annealing in Ga followed by H2 diffusion did not lead to a significant increase in conductivity (Table 1c). Both sole H-diffusion and H-diffusion after O2-anneal treatments were carried out on other as-grown undoped Ga2O3 samples and led to the same results. Samples preserved their p-type or n-type conductivity with no decay or negligible decay after months. The hole sheet number was measured after several months and found to be very 9.4 × 1014 cm−2 (initial sheet hole number was 1.2 × 1015 cm−2); after that the carrier concentration seems to be very stable with time with no further decay. The electron sheet number is stable at 1016 cm−2. As the samples have been processed at very high temperatures, we expect that further processing at high temperature would not be a problem. It is also expected that the samples should sustain high voltage owing to the wide band gap of Ga2O3. However we have not built a device to test their stability under high voltage, future studies for further device development are necessary and would be highly valuable.
(a) Defect parameters S and W measured by Doppler Broadening of Positron Annihilation Spectroscopy (DBPAS) as a function of penetration depth, S and W are defined as the fraction of positrons annihilating with valence and core electrons respectively. The lower x-axis represents the positron energies and the upper x-axis represents the penetration depth. The graph shows that H2 diffuses about 500 nm in the crystal. Positron Annihilation Lifetime Spectroscopy (PALS) data of (b) as-grown and (c) annealed in H2 (950 °C for 2 hours) (d) annealed in O2 followed by H2 (950 °C for 2 hours) samples. EP = Positron implantation energy, Zmean = positron implantation depth, τ = positron lifetime, I = intensity of lifetime component, the graphs (b, c, and d) show the two positron lifetime components and their intensities in each sample. τ1 is related to the positrons annihilate in the bulk and τ2 is related to the positrons annihilate in the defect sites. τ2 has significantly decreased after H2 diffusion (graph c) because of the change of the charge state of the defect center (VGA-2H)−1. After O2 + H2 annealing, the contribution of τ2 is almost zero because the (VGA-4H)+1 center has positive charge state and does not trap positrons.
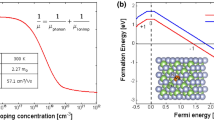
Figure 3 shows the temperature dependence of sheet resistance and sheet number of the p-type and n-type Ga2O3 samples, signifying the ionization of carrier regions followed by extrinsic semiconductor behavior at higher temperatures. Intrinsic semiconductor behavior cannot occur at room temperature as band to band transitions are not possible in Ga2O3 at this temperature because of the ultra-wide band gap. It is noteworthy to mention that the freeze out regions for the two samples are consistent with the donor/acceptor ionization energy calculated by thermally stimulated luminescence technique presented in later section. It is useful to compare these current measurements by the recent work by Ekaterina et. al.18 who performed temperature dependent Hall-effect measurements for deep acceptors in unintentionally doped β-Ga2O3 with ionization energy more than 1 eV, their measurements showed that the activation region is in the range of 300–650 K consistent with deep acceptors. Figure 3 here shows that the activation occurs for donor and acceptor at significantly lower temperatures, which confirms the shallow nature of the induced donor and acceptor states. Figure 3d shows the electron mobility behavior with temperature.
Temperature dependent transport properties of the n-type and p-type H2 treated Ga2O3 samples. (a) sheet resistance, (b) sheet number, (c) sheet number logarithm plotted as a function of 1000/T. (d) The dependence of n-type mobility on temperature. The mobility was found to be 100 cm2/VS at room temperature; it was normalized to the highest value at low temperature because of the noise in the cryostat system.
The realization of p-type and n-type conductivity after H2 diffusion can be explained as follows. Since the n-type conductivity of the samples was realized after filling up oxygen vacancies and since we know that H-interstitials which act as shallow donors are not stable in Ga2O313, we attribute the origin of n- and p-type conductivity to hydrogen decorated gallium vacancies, VGa-H. A Ga-vacancy acts as a deep acceptor with −3 charge state (VGa)3−. During the diffusion of hydrogen into the crystal, the surface adsorbed proton (Fig. 1a,b) becomes attracted toward the (VGa)3− where it stabilizes the negative charge and, therefore, lowers the acceptor state. This results in H-decorated Ga-vacancy (VGa-2H)1− (as represented in Fig. 1c) and p-type conductivity. At lower temperatures (e.g. 700 °C), protons are less likely to diffuse deep inside the bulk crystal. This results in a decrease in conductivity over time due to the reverse diffusion at room temperature. However, the high p-type conductivity persists over time and becomes stable for the sample exposed to H2 at higher temperature and for a longer period of time due to the diffusion of H+ deeper into the crystal.
The sample that is exposed to the H2 after filling up VO (after annealing in O2) showed high n-type conductivity. In this case, more H are diffused into the VGa due to the absence/reduction of VO leading to the formation of (VGa-4H)1+ as represented in Fig. 1d, which acts as a donor. That is, the absence of VO in this case means that the only available traps for H are VGa, which thus become filled to a greater extent. The contribution of n-type conductivity from Hi or Ho is not prominent as filling up VGa followed by H-diffusion shows a negligible increase in carrier concentration. Moreover, it confirms that the H-decorated VGa are primarily responsible for the induced n-conductivity in the samples. It should be also noted that oxygen vacancies are confirmed by now to be deep donors in Ga2O3 and not source of conductivity13.
Density functional theory was used to examine H-incorporation into a Ga-vacancy. The details of the calculation are given in the method section. The results are presented in Table 2. The binding energy of one H+ ion to the Ga-vacancy is -4.4 eV. The DFT calculations reveal that, as N (the number of H ions) increases, at least up to N = 4, the reaction remains exothermic, though the strength of the binding, per H atom, decreases. The energy gained by adding the 4th H+ ion is only −0.8 eV, much less than the −4.4 eV gained by adding the 1st H+ ion. If the trend persisted, this suggests that no more than 4 H+ ions can be favorably accommodated into VGa. Thus, these calculations indicate that a single VGa can accommodate up to 4 H+ ions, changing the net charge of the complex from 3- (when N = 0) to 1+ (when N = 4), and confirm that (VGa-4H)1+ (Fig. 1d) is more favorable than Hi+. These calculations verified our interpretation of the electrical transport measurements that (VGa-4H)1+ is the dominant donor in the treated highly conductive n-type sample. This cation vacancy filled with the relevant numbers of H+ represents a new type of donor that does not create disorder in the lattice suppressing electron mobility as in the case of standard dopants on substitutional or interstitial sites.
Low temperature thermally stimulated luminescence spectroscopy was performed on the samples to reveal the shallow donor/acceptor levels. At low temperatures, shallow donor/acceptor level can be provided with carriers by optical injection that get trapped at respective levels. These carriers can be released by thermal excitation that reveals valuable information about the shallow donor/acceptor levels. The details of the experiments can be found in the method section and in the text and Supplementary Figure 1 in the Supplementary Information file. Figure 4a displays the TSL emission for as-grown, p-type and n-type H2 treated Ga2O3. The as-grown sample shows no peak corresponding to shallow levels. Each of the other two samples shows a peak at low temperature indicating the formation of shallow level. The peak formed at 107 K in the p-type H2-anneal sample (red curve in Fig. 4a) is associated with the formation of shallow acceptors with ionization energy of 42 meV, calculated using the simplified model of TL developed by Randal and Williams26,27,28. The ionization energy of the donor, emerging after O2-annealing followed by H2-diffusion (green curve in Fig. 4a), was also calculated by the initial rise method from the peak at 111 K and found to be 20 meV. Figure 4b,c shows the corresponding flat band diagram and corresponding donor and acceptor state. The details of the calculation of donor/acceptor ionization energy is provided in the text and Supplementary Figure 2 in the Supplementary Information.
Thermally stimulated luminescence emission (a) of the samples annealed at 950 °C for two hours in different environments and the as-grown sample. Data points for annealed samples were normalized from 0 to 1. Data points for as-grown sample were normalized from 0 to 0.5 to minimize noise (no glow peak). Peaks were fitted with a Gaussian function. The two peaks appeared at low temperature after H2 diffusion, and after O- anneal followed by H-diffusion are associated with the induced shallow acceptor and shallow donor in the samples respectively and they were used for calculating the ionization energies. The flat band diagrams showing donor and acceptor states of the samples after direct hydrogen diffusion (b) and hydrogen diffusion after filling up oxygen vacancies (c).
To further understand the effect of H-incorporation and confirm our interpretation of the origin of conductivity, we carried out Positron Annihilation Spectroscopy (PAS) measurements. The details of the experiments and data analysis are given in the method section. Positron Annihilation Spectroscopy is a powerful technique to investigate cation vacancy type defects29. It has also been established as an effective tool to probe the incorporation of hydrogen in cation vacancies as partial or complete passivation of vacancies by hydrogen strongly impact positron trapping30,31,32. We have performed Positron Annihilation Lifetime Spectroscopy (PALS) and Doppler Broadening of Positron Annihilation Spectroscopy (DBPAS), two varieties of Positron Annihilation Spectroscopy that gives valuable information about the electronic environments in cation vacancy sites. Figure 2a presents the defect parameters S and W (defined in Fig. 2) as a function of depth for the two treated samples. The large values of S at the very beginning of the two curves are common in all DBPAS measurements, indicating the formation of positronium at the surface. The graph shows a large difference between the two samples only in the first 500 nm (where H diffuses in) with lower S values and higher W values for the sample annealed in O2 followed by H2, which exhibits high n-type conductivity. The decrease in S-parameter is an indication for the suppression of positron trapping at cation or neutral vacancies. Thus, these measurements confirm the decrease of negatively charged and neutral vacancies in the O2-annelead followed by H2-diffusion sample. This must be due to filling of Ga-vacancies with more than three H-ions leading to a positive charge state and the formation of a shallow donor as indicated by the immense increase in n-type conductivity. This (H-VGa)1+ complex has a positive charge state and cannot trap positrons, leading to the substantial decrease in S-parameter. On the other hand, sole H2-diffusion leads to partial filling of VGa with hydrogen maintaining a negative charge state and leading to shallow acceptors, which imparts p-type conductivity. This (H-VGa)1− complex is still an active positron trap which leads to a higher S-value.
Depth resolved measurements of PALS revealed two major positron lifetime components for each sample (example of PALS spectra measured for the p-type and n-type samples is given in Supplementary Figure 3 in the Supplementary Information file). Figure 2b–d show the lifetime components and their intensity as a function of depth for the as-grown sample, and the H2 diffused, and O2-annealed followed by H2-diffused samples. A distinctive difference can be seen in the intensity and magnitude of the positron lifetime components among the three samples. The large second lifetime component τ2 indicates the presence of VGa-related defects with negative charge states. For as-grown Ga2O3, τ2 is about 470 ps with about 25 to 30% intensity across the sample depth (Fig. 2b). After H2-anneal, τ2 was reduced to ~320 ps indicating partial filling of VGa related defects with hydrogen while its intensity was reduced to about 13% (Fig. 2c) due to the decrease of positron trapping at these vacancies as result of less negativity. After annealing in O2 followed by H2-diffusion, almost all positrons annihilate with lifetimes close to the bulk lifetime (Fig. 2d)33. The intensity of τ2 was reduced to about less than 1% indicating almost complete absence of positron trapping at defects providing strong evidence for filling up VGa related defects with H2 transforming them into donors with a positive charge state, which cannot trap positrons. Thus, DBPAS and PALS measurements explicitly confirm our interpretation for the origin of n-type and p-type conductivity.
Precise doping and carrier control is important to realize β-Ga2O3 based bipolar devices34,35. In this work, we found that the best way to control the number of H in VGa is to remove VO and keep H2 pressure the same. By doing this, we can increase the number of H incorporating in the VGa. Here, the number of H resides in the VGa is governed by the thermodynamic stability of the complex and the availability of hydrogen atoms. However, the number of H incorporated in the VGa can also be controlled by adjusting H2 pressure. A detail study of the effect of hydrogen pressure on the type and concentration of carriers would be of great interest to further develop different processes for H incorporation.
Conclusions
In summary, by controlling H-incorporation in the lattice, we have demonstrated the development of stable p-type and n-type Ga2O3, which is expected to significantly advance optoelectronics and high-power devices. In the meantime, we illustrated a potential simple method for tuning and switching the conductivity of semiconductors between p-type and n-type with the realization of remarkable high carrier density and good mobility in wide band gap oxides, which is a significant challenge by common substitutional doping methods. A concept for new donor type as cation vacancy filled with the relevant numbers of H+ was introduced and found to be behind the remarkable n-type conductivity. This new donor type does not create disorder in the lattice, which often suppresses carrier mobility in the case of standard doping.
Methods
Hydrogen incorporation process
High quality β-Ga2O3 samples grown by Edge- defined Film-fed Growth (EFG) method were obtained from Tamura Inc., Japan. A number of undoped highly resistive samples (5 mm × 5 mm × 0.5 mm) were selected and placed in a quartz ampoule with one open end that was connected to a vacuum pump to pump the air out and evacuate the ampule. After that, the tube was filled with H2 gas at 580 torr pressure. After filling the tube with hydrogen, the open end was properly sealed. The ampoule was placed in an oven where temperature can be precisely controlled. The temperature was increased in two steps up to the desired value and H2 was allowed to diffuse into the crystal for 1 or 2 hours. A few other samples of same dimensions were first annealed in oxygen flow at 950 °C and then hydrogen following the same procedure, while others were annealed first with gallium, then hydrogen following the same procedure.
Hall-effect measurements
Van der Pauw Hall-effect measurements were performed to determine the electrical transport properties of the samples. The measurements were carried out from 30 K to room temperature (298 K) and at constant magnetic field of 9300 G. Four indium contacts were made in a square arrangement on the surface of each sample and carefully adjusted to keep the contacts as small as possible. Current-voltage linearity was checked every time to make sure that the contacts were good and resistivity does not vary more than 10% between different contact points. Temperature dependent measurements of the carrier concentration were carried out from 30 K or below to room temperature using a closed cycle cryostat.
Computational analysis
Density functional theory, as implemented in the Vienna ab-initio Simulation Package (VASP)36,37, was used to examine H-incorporation into a Ga-vacancy. These calculations were performed on a 1 × 4 × 2 supercell of β-Ga2O3, containing a total of 160 atoms in the defect-free structure. A Г-centered 2 × 2 × 2 Monkhorst-Pack k-point mesh38 was used to sample the Brillouin zone. The energy cutoff for the planewaves was 400 eV. Pseudopotentials based on the projector augmented wave method39 and the Perdew, Burke, and Ernzerhof (PBE)40 generalized gradient approximation (GGA) exchange-correlation functional (which should be good enough for our purpose here) were used. Calculations were continued until the maximum component of the force on any atom was less than 0.02 eV/angstrom, with one exception (the charged Ga-vacancy), where such a tight convergence was not possible. In this case, the maximum force was 0.024 eV/angstrom. Both monopole corrections (using a calculated dielectric constant of 4.16, which is a bit higher but similar to previously reported values)41 and an alignment correction were applied to the energies. Instead of averaging the potential to perform the alignment correction, we simply shifted the density of states such that the deepest state in the material aligned across different structures, which has been shown to give similar corrections42. In any case, the magnitude of this correction was no greater than 0.1 eV.
A VGa was created by removing a tetrahedrally-coordinated Ga ion from the cell, as this vacancy structure has been identified as being more favorable43. A net charge of −3 was imposed on the structure. H+ ions with a charge of +1 were inserted into the resulting vacancy structure (leaving the total number of electrons in the system constant but reducing the net charge of the cell). The resulting binding energy for each configuration was computed via the following relationship:
where \(E({[{V}_{{\rm{Ga}}}{\rm{NH}}]}^{(-3+N)})\) is the energy of the system with the Ga vacancy filled with N H+ ions, \(E({\rm{Bulk}}\,{{\rm{Ga}}}_{2}{O}_{3})\) is the energy of defect-free β-Ga2O3, \(E({{V}_{{\rm{Ga}}}}^{3-})\) is the energy of the isolated Ga vacancy in a 3- charge state, and \(E({H}^{+})\) is the energy of an isolated 1+ H interstitial. With this definition, a negative energy indicates an exothermic or favorable reaction. We did not perform a systematic search for the lowest energy H interstitial position but performed multiple minimizations where the H was randomly displaced to find a reasonable structure. The structure found here, in which the H+ ion is bonded to one of the three-fold coordinated oxygen ions, is similar to that described by Varley et al.13.
Thermal stimulated luminescence spectroscopy (TSL)
Thermal stimulated luminescence (TSL) spectroscopy26,27,28,44,45,46 was performed on the samples to calculate the donor and acceptor ionization energies45. The measurements were performed using an in-house built spectrometer26,47, from −190 °C to 25 °C. The samples were first placed in a dark compartment and irradiated with UV light at −190 °C for 30 min. After irradiation, the temperature of the samples was set to increase at constant rate (60 °C/min) and the emission spectra were recorded from 200 to 800 nm at every 5 seconds. The glow curves which represent the emission intensity as a function of temperature were constructed from the integration of emission over wavelengths at each temperature.
Positron annihilation spectroscopy
We carried out positron annihilation spectroscopy (PAS), which is a well-established technique to detect and characterize cation vacancies in semiconductors and oxides48,49. Both Doppler Broadening of Positron Annihilation Spectroscopy (DBPAS) and Positron Annihilation Lifetime Spectroscopy (PALS) were employed. DBPAS measurements were carried out using a mono-energetic variable energy positron beam at Helmholtz-Zentrum Dresden-Rossendorf (HZDR) facility in Dresden, Germany50. Positrons are emitted from an intense 22Na source and a tungsten moderator and accelerated to discrete energy values Ep in the range of Ep = 0.05–35 keV. Such positron implantation energy, Ep allows penetrating up to about 1.8 µm in Ga2O3. Doppler broadened spectra representing positron annihilation distribution for each Ep were acquired using a single high-purity germanium detector with energy resolution of 1.09 ± 0.01 keV at 511 keV and the S and W parameters (defined in the caption of Fig. 2) were calculated from the peak. PALS has been established as the most effective method to probe cation vacancy related defects, distinguishing between their types and providing information about their concentrations49. PALS was performed at the Mono-energetic Positron Spectroscopy (MePS) pulsed beam, which is the end station of the radiation source ELBE (Electron Linac for beams with high Brilliance and low Emittance) at HZDR facility in Dresden Germany50. The lifetime spectrum was measured at each positron energy Ep up to 16 keV with a time resolution of 205 ps. All lifetime spectra contained at least 5 × 106 counts and were analyzed as a sum of time-dependent exponential decays, N(t) = Σi Ii/τi·exp(−t/τi) convoluted with the Gaussian’s functions describing the spectrometer timing resolution, using the PALSfit fitting software51. Depth-resolved measurements of PALS revealed two major positron lifetime components for each sample.
References
Higashiwaki, M., Sasaki, K., Kuramata, A., Masui, T. & Yamakoshi, S. Gallium oxide (Ga2O3) metal-semiconductor field-effect transistors on single-crystal β-Ga2O3 (010) substrates. Appl. Phys. Lett. 100, 013504 (2012).
Selim, F., Weber, M., Solodovnikov, D. & Lynn, K. Nature of native defects in ZnO. Phys. Rev. Lett. 99, 085502 (2007).
Ogo, Y. et al. p-channel thin-film transistor using p-type oxide semiconductor, SnO. Appl. Phys. Lett. 93, 032113 (2008).
Oishi, T., Koga, Y., Harada, K. & Kasu, M. High-mobility β-Ga2O3(201) single crystals grown by edge-defined film-fed growth method and their Schottky barrier diodes with Ni contact. Appl. Phys. Express 8, 031101 (2015).
Irmscher, K., Galazka, Z., Pietsch, M., Uecker, R. & Fornari, R. Electrical properties of β-Ga2O3 single crystals grown by the Czochralski method. J. Appl. Phys. 110, 063720 (2011).
Zhang, Y. et al. MOCVD grown epitaxial β-Ga2O3 thin film with an electron mobility of 176 cm2/V s at room temperature. APL. Mater. 7, 022506 (2018).
Neal, A. T. et al. Donors and deep acceptors in β-Ga2O3. Appl. Phys. Lett. 113, 062101 (2018).
Mastro, M. A. et al. Perspective-Opportunities and Future Directions for Ga2O3. ECS J. Solid. State Sci. Technol. 6, 356 (2017).
Higashiwaki, M. & Jessen, G. H. The dawn of gallium oxide microelectronics. Appl. Phys. Lett. 112, 060401 (2018).
Pearton, S. J. et al. Perspective: Ga2O3 for ultra-high power rectifiers and MOSFETS. Appl. Phys. Rev. 5, 011301 (2018).
Geller, S. Crystal Structure of β-Ga2O3. J. Chem. Phys. 33, 676 (1960).
Hajnal, Z. et al. Role of oxygen vacancy defect states in the n-type conduction of β-Ga2O3. J. Appl. Phys. 86, 3792 (1999).
Varley, J., Weber, J., Janotti, A. & Van de Walle, C. Oxygen vacancies and donor impurities in β-Ga2O3. Appl. Phys. Lett. 97, 142106 (2010).
Ueda, N., Hosono, H., Waseda, R. & Kawazoe, H. Synthesis and control of conductivity of ultraviolet transmitting β-Ga2O3 single crystals. Appl. Phys. Lett. 70, 3561 (1997).
Villora, E. G., Shimamura, K., Yoshikawa, Y., Ujiie, T. & Aoki, K. Electrical conductivity and carrier concentration control in β-Ga2O3 by Si doping. Appl. Phys. Lett. 92, 202120 (2008).
Sasaki, K., Higashiwaki, M., Kuramata, A., Masui, T. & Yamakoshi, S. Si-ion implantation doping in β-Ga2O3 and Its application to fabrication of low-resistance ohmic contacts. Appl. Phys. Express 6, 086502 (2013).
Feng, Z., A U Bhuiyan, A. F. M., Karim, M. R. & Zhao, H. MOCVD homoepitaxy of Si-doped (010) β-Ga2O3 thin films with superior transport properties. Appl. Phys. Lett. 114, 250601 (2019).
Chikoidze, E. et al. P-type β-gallium oxide: A new perspective for power and optoelectronic devices. Mater. Today Phys. 3, 118–126 (2017).
Qian, Y. P. et al. Mg-doped p-type β-Ga2O3 thin film for solar-blind ultraviolet photodetector. Mater. Lett. 209, 558–561 (2017).
McCluskey, M., Tarun, M. & Teklemichael, S. Hydrogen in oxide semiconductors. J. Mat. Res. 27, 17 (2012).
Janotti, A. & Van de Walle, C. G. Hydrogen multicentre bonds. Nat. Mater. 6, 44 (2007).
Korhonen, E. et al. Electrical compensation by Ga vacancies in Ga2O3 thin films. Appl. Phys. Lett. 106, 242103 (2015).
Weiser, P., Stavola, M., Fowler, W., Qin, Y. & Pearton, S. Structure and vibrational properties of the dominant O-H center in β-Ga2O3. Appl. Phys. Lett. 112, 232104 (2018).
Garcia-Melchor, M. & Lopez, N. Homolytic Products from Heterolytic Paths in H2 Dissociation on Metal Oxides: The Example of CeO2. J. Phys. Chem. C. 118, 20 (2014).
Helali, Z., Jedidi, A., Syzgantseva, O. A., Calatayud, M. & Minot, C. Scaling reducibility of metal oxides. Theoret. Chem. Acc.: Theory, Computation, Modeling, Springer Verl. 136(9), 100 (2017).
Mackay, D. T., Varney, C. R., Buscher, J. & Selim, F. A. Study of exciton dynamics in garnets by low temperature thermo-luminescence. J. Appl. Phys. 112, 023522 (2012).
Islam, M. M., Rana, D., Hernandez, A., Haseman, M. & Selim, F. A. Study of trap levels in β-Ga2O3 by thermoluminescence spectroscopy. J. Appl. Phys. 125, 055701 (2019).
Mahesh, K., Weng, P. & Furetta, C. Thermoluminescence in solids and its applications. Nuclear Technology, Kent, England, (1989).
Weber, M. H., Selim, F. A., Solodovnikov, D. & Lynn, K. G. Defect engineering of ZnO. Appl. Surf. Sci. 255, 68 (2008).
Selim, F. A. et al. Positron lifetime measurements of hydrogen passivation of cation vacancies in yttrium aluminum oxide garnets. Phys. Rev. B 88, 174102 (2013).
Huang, L. J., Lau, W. M., Simpson, P. J. & Schultz, P. J. Depth profiling of hydrogen passivation of boron in Si(100). Phys. Rev. B 46, 4086–4091 (1992).
Winarski, D. J. et al. Induced conductivity in sol-gel ZnO films by passivation or elimination of Zn vacancies. AIP Adv. 6, 095004 (2016).
Ting, W. Y., Kitai, A. H. & Mascher, P. Crystallization phenomena in β-Ga2O3 investigated by positron annihilation spectroscopy and X-ray diffraction analysis. Mater. Sci. Eng., B 91, 541 (2002).
Zhang, F. et al. Toward controlling the carrier density of Si doped Ga2O3 films by pulsed laser deposition. Appl. Phys. Lett. 109, 102105 (2016).
Sasaki, K., Higashiwaki, M., Kuramata, A., Masui, T. & Yamakoshi, S. Si-Ion Implantation Doping in β-Ga2O3 and Its Application to Fabrication of Low-Resistance Ohmic Contacts. Appl. Phys. Express 6, 086502 (2013).
Kresse, G. & Furthmuller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169 (1996).
Kresse, G. & Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 59, 1758 (1999).
Monkhorst, H. J. & Pack, J. D. Special points for Brillouin-zone integrations. Phys. Rev. B 13, 5188 (1976).
Blöchl, P. E. Projector augmented-wave method. Phys. Rev. B 50, 17953 (1994).
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 77, 3865 (1996).
Sturm, C., Furthmüller, J., Bechstedt, F., Schmidt-Grund, R. & Grundmann, M. Dielectric tensor of monoclinic Ga2O3 single crystals in the spectral range 0.5–8.5 eV. Apl. Mat. 3, 106106 (2015).
Yadav, S. K., Uberuaga, B. P., Nikl, M., Jiang, C. & Stanek, C. R. Band-Gap and Band-Edge Engineering of Multicomponent Garnet Scintillators from First Principles. Phys. Rev. Appl. 4, 054012 (2015).
Varley, J. B., Peelaers, H. & Janotti, A. and Van de Walle, C.G. Hydrogenated cation vacancies in semiconducting oxides. J. Phys.: Condens. Matter 23, 334212 (2011).
Varney, C. R., Mackay, D. T., Pratt, A., Reda, S. M. & Selim, F. A. Energy levels of exciton traps in yttrium aluminum garnet single crystals. J. Appl. Phys. 111, 063505 (2012).
Ji, J., Boatner, L. A. & Selim, F. A. Donor characterization in ZnO by thermally stimulated luminescence. Appl. Phys. Lett. 105, 041102 (2014).
Reda, S. M., Varney, C. R. & Selim, F. A. Radio-luminescence and absence of trapping defects in Nd-doped YAG single crystals. Results Phys. 2, 123 (2012).
Selim, F. A., Khamehchi, A., Winarski, D. & Agarwal, S. Synthesis and characterization of Ce:YAG nano-phosphors and ceramics. Optical Mater. Express 6, 3704 (2016).
Krause-Rehberg, R. & Leipner, H. S. Positron Annihilation in Semiconductors. Springer-Verlag (1999).
Selim, F. A. et al. Generation and characterization of point defects in SrTiO3 and Y3Al5O12. Results Phys. 5, 28 (2015).
Wagner, A., Butterling, M., Liedke, M. O., Potzger, K. & Krause-Rehberg, R. AIP Confer. Pro. 1970, 040003 (2018).
Olsen, J. V., Kirkegaard, P., Pedersen, N. J. & Eldrup, M. PALSfit: A new program for the evaluation of positron lifetime spectra. Phys. Status Solidi C. 4, 4004 (2007).
Acknowledgements
BPU acknowledges helpful discussions with David Andersson and Ghanshyam Pilania. PAS experiments were carried out at ELBE at the Helmholtz-Zentrum Dresden-Rossendorf e.V., a member of the Helmohltz Association. We would like to thank the facility staff for assistance. This work was supported as part of FUTURE (Fundamental Understanding of Transport Under Reactor Extremes), an Energy Frontier Research Center funded by the U.S. Department of Energy, Office of Science, Basic Energy Sciences. Los Alamos National Laboratory is operated by Triad National Security, LLC, for the National Nuclear Security Administration of U.S. Department of Energy (Contract No. 89233218CNA000001).
Author information
Authors and Affiliations
Contributions
F.A. Selim, B.P. Uberuaga and M.M. Islam wrote the manuscript. F.A. Selim designed the experiments and interpreted the results. M. M. Islam carried out most of the experiments except PAS, analyzed the data, prepared the graphs and calculated the ionization energies. B.P. Uberuaga performed the computational analysis. M.O. Liedke, M. Butterling, and A. Wagner performed PAS experiments. D. Winarski contributed in preparing and measuring samples. Y. Wang and P. Hosemann contributed in the analysis discussion and revised the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Islam, M.M., Liedke, M.O., Winarski, D. et al. Chemical manipulation of hydrogen induced high p-type and n-type conductivity in Ga2O3. Sci Rep 10, 6134 (2020). https://doi.org/10.1038/s41598-020-62948-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-020-62948-2
This article is cited by
-
Ir impurities in \(\alpha\)- and \(\beta\)-\(\text {Ga}_{2}\text {O}_{3}\) and their detrimental effect on p-type conductivity
Scientific Reports (2023)
-
The effects of hydrogenation on the properties of heavy ion irradiated β-Ga2O3
Journal of Materials Science: Materials in Electronics (2023)
-
Unintentional doping effect in Si-doped MOCVD β-Ga2O3 films: Shallow donor states
Science China Materials (2023)
-
Positron lifetime spectroscopy applied to pure Tellurium
Indian Journal of Physics (2022)
-
Localized UV emitters on the surface of β-Ga2O3
Scientific Reports (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.