Abstract
Annual rings record the intensity of cosmic rays (CRs) that had entered into the Earth’s atmosphere. Several rapid 14C increases in the past, such as the 775 CE and 994CE 14C spikes, have been reported to originate from extreme solar proton events (SPEs). Another rapid 14C increase, also known as the ca. 660 BCE event in German oak tree rings as well as increases of 10Be and 36Cl in ice cores, was presumed similar to the 775 CE event; however, as the 14C increase of approximately 10‰ in 660 BCE had taken a rather longer rise time of 3–4 years as compared to that of the 775 CE event, the occurrence could not be simply associated to an extreme SPE. In this study, to elucidate the rapid increase in 14C concentrations in tree rings around 660 BCE, we have precisely measured the 14C concentrations of earlywoods and latewoods inside the annual rings of Japanese cedar for the period 669–633 BCE. Based on the feature of 14C production rate calculated from the fine measured profile of the 14C concentrations, we found that the 14C rapid increase occurred within 665–663.5 BCE, and that duration of 14C production describing the event is distributed from one month to 41 months. The possibility of occurrence of consecutive SPEs over up to three years is offered.
Similar content being viewed by others
Introduction
Since the phenomenon of rapid increase in CR intensity in 775 CE was solved1,2,3, 14C analysis in annual rings has played a major role in searching for another rapid 14C increase event4,5. While such events have been suggested to originate from an extreme SPE6,7,8,9,10, the other type with a rather long period of 14C increase has recently been reported in German oak tree rings11. It is the 14C increase in ca. 660 BCE whose 14C increment is comparable to that of the 775 CE event. Its rise time of 3 to 4 years is, however, longer than that of approximately 1 year in the 775 CE event.
There are several possible CR sources capable of such extraordinary 14C increment, i.e. galactic cosmic rays (GCRs) modulated by a variation in the interplanetary magnetic fields due to solar activities, gamma-rays from supernova (SN) and gamma-ray burst (GRB), and an extreme SPE. Recently, O’Hare et al. showed sharp increases of 10Be and 36Cl concentrations with high-resolution in Greenland ice cores around 2610BP (~660 BC)12. We name the phenomenon the “~660 BCE event” including the 14C increase at ca. 660 BCE.
O’Hare et al. mentioned that the solar modulation of GCRs was not suitable to explain the enhancement of 10Be, 36Cl, and 14C concentrations even by decreased solar activities with showing the significant large excesses against the typical amplitude of an 11-year solar cycle12. The increases of 14C coincide with those of the ice core-based radionuclides, exceeding the variations of 11-year cycle in the range of 4‰13. Also, both SN and GRB were inappropriate for the ~660 BCE event, as the large amount of 10Be were evidently detected in the ice cores. It is expected that undetectable amount of 10Be atoms are produced by the gamma-ray origin12,14. According to energy spectrum estimation based on the measurements of 10Be and 36Cl in ice core, the origin of the ~660 BCE event was suggested to be an extreme SPE with very hard energy spectrum which is an order of magnitude larger than the largest SPE during the instrumental era12.
O’Hare et al., also, mentioned that the 2.3-yr long peak of the 10Be in the ~660 BCE event is likely to have been caused by a rapid production rate increase and a residence time in the atmosphere due to the subsequent transport from the stratosphere to the deposition12. However, it is still unclear whether the events, in particular on the longer rise time of 14C, are triggered by a single short pulse of a huge SPE or by a consecutive occurrence of considerably large SPEs, while Güttler et al. suggest a short duration less than 1 year for the 775 CE event using a carbon cycle box model7. Precise 14C profiles of the ~660 BCE event will provide us a clue to figure out the origin of 14C increase with a rather long rise time. Hence, we carried out accurate 14C measurements with higher time resolution using Japanese tree rings. We present the precise 14C time profile, and its production profile calculated quantitatively using a carbon cycle box model.
Results
We investigated 14C concentrations for the earlywoods and latewoods for the period 669–633 BCE in Cryptomeria japonica dug out from Choukai volcano in northern Japan (Fig. S1), known locally as “Choukai–Jindai Cedar” (see methods for the dating of the sample and Fig. S2 for 14C profile). Figure 1 shows the 14C concentrations using the notation of Δ14C by Stuiver and Polach15 for 669–656 BCE. The Δ14C profile shows a rapid increase of 9.8 ± 2.2‰ from the latewood in 665 BCE (plotted in 664.3 BCE in the figure) to the latewood in 664 BCE, going up to a whole of 16.3 ± 2.1‰ by a gradual increase until the latewood in 662 BCE, after which the increment gradually decreased. On the other hand, Δ14C in every earlywood of 663–661 BCE were comparable to those in every latewood of the preceding year. This feature is considered to reflect a seasonal variation of 14C concentration in the atmosphere, i.e. 14C concentrations during the period when latewoods ring formed (August–September) are higher than those of earlywoods (April–July), as stratosphere–troposphere exchange occurs strongly during spring/summer of the Northern Hemisphere16,17.
Measured 14C concentrations (Δ14C) in earlywoods (open circles) and latewoods (solid circles) of Choukai–Jindai cedar annual rings in 669–656 BCE. Since earlywoods and latewoods generally form in spring-to-summer and in summer-to-autumn, respectively, the time resolution of the obtained data set is not strictly half a year. Every earlywoods and latewoods are plotted at 1st June and 1st September, respectively.
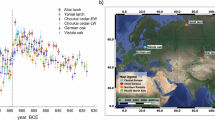
Figure 2 shows a comparison of the annual Δ14C data sets for 670–646 BCE between the Choukai–Jindai cedar and the German oak rings previously published11. In the comparison, our data were averaged to an annual resolution. Increments from the minimum to the peak of both tree series were mutually comparable. In 669–651 BCE, the average Δ14C values of the German oak was (8.9 ± 0.4)‰, which is (1.9 ± 0.5)‰ higher than those of the Choukai–Jindai cedar; the disparity is considered as a kind of regional offset relating to the location of Japanese archipelago on the fringe of the pacific ocean18. However, the peak position of Δ14C from the German oak seemed to lie one year behind that of the Choukai–Jindai cedar. Although we could not compare the two series directly as the annual data was not a simple average of earlywoods and latewoods, an age mistake of each dendro-model, or a difference of the physiology between oaks and cedars ring growth (see Methods), was taken as a possible reason.
Comparison of annual Δ14C profiles between the Choukai–Jindai cedar (blue solid circles) and the German oak (black solid triangles) (Park et al.11) in 670–646 BCE. Δ14C values of the Choukai–Jindai cedar show averages of earlywoods and latewoods. For series comparison of the two series, the vertical axis of the Choukai series is shifted −2‰ from that of the German oak series. The increments from the minimum to the peak in the Choukai–Jindai cedar and German oak series are (14.3 ± 1.5)‰ over 4 years and (13.3 ± 2.1)‰ over 6 years, respectively.
The Δ14C variation appears to be a profile due to a distinct 14C input with a duration of longer than a year receiving the global carbon cycle. Employing the 11-box model by Güttler et al.7, but with several conditions, i.e. stratosphere-troposphere exchange times of 1.5-year and 2.0-year16 and 14C production share rates between the stratosphere and troposphere (strat:trop = 70%:30%, 80%:20%, and 90%:10%), we examined Δ14C response to a square pulsed 14C input (single-pulsed event). Three free parameters of pulse duration, pulse height, and, pulse start date for single-pulsed events were estimated by fitting calculation to the Δ14C data using the box model. Details of the box model and the fitting calculation are described in the methods section. The fitting results are shown by contour maps of reduced χν2 values in the Supplementary Information (Fig. S3). Figure 3 shows the contour map containing the smallest reduced χν2 value among all the six conditions, i.e., the exchange times of 1.5 years and the share rate (70%:30%) in the stratosphere-troposphere. In this condition, durations from one month to 41 months (3.4 years) cannot be rejected at 95% confidence level, according to the pulse start dates from 665 BCE to 663.5 BCE. The total input 14C productions were in a range of (1.3 – 1.5) × 108 [atoms/cm2] based on the 95% confidence level. Even with the other conditions, also, the durations were up to 30–39 months in same manner. For any conditions, moreover, the total input 14C productions were almost same on the basis of 95% confidence level (Table S1). In the case of the 775 CE event, the best-fitted duration of a square-pulsed 14C input is less than a year1,7 and longer durations than 1.5 years can be rejected with 95% confidence level7; therefore, characteristics of input durations of 14C between the 775 CE event and the ~660 BCE event are different, i.e. the ~660 BCE event allows a long-term 14C input.
Contour map of reduced χν2 values as a function of pulse duration and pulse start date for the pulse height including the best-fitted single-pulsed event. The conditions of fitting calculation in the 11-box model are 1.5 years, and 70%: 30% for the exchange time and share rate of input 14C production between the stratosphere and troposphere, respectively. Outside of the red region is rejected with 95% confidence level.
Discussions
A clear distinction between the ~660 BCE and 775 CE events is the rise times of 14C concentrations, which are 3 years and 1–2 year, respectively. It is very important to figure out whether the large 14C production is triggered by a single SPE or by multiple SPEs, not only in space–earth environment science but also in solar physics. However, there has been presented no evidence of multiple SPEs for the known rapid 14C increase events like the 775 CE event, because multi-annual 14C inputs (>1.5 years) associated with the event have been clearly rejected7. On the contrary, the ~660 BCE event allows duration up to ~41 months (a few years), and a step like increment profile of 14C concentrations is appeared in Fig. 4, which might imply a multiple SPEs case.
(Upper panel) Best-fitted profile by the 11-box model calculation on the Δ14C data set for single-pulsed (black line) and double-pulsed (red line) events. (Lower panel) 14C production rates injected in the atmosphere and achieving the best-fit profile of the Δ14C in the upper panel (black line: single-pulsed event; red line: double-pulsed event).
A sequence of SPEs originating from the similar activity burst is a scenario of the multiple SPEs case. The example is the ones during July-November 1989, October-November 2003, or January 2005, etc.19. Also, a long-separated consecutive-event with an interval up to a few years is another possible scenario of the multiple SPEs case. Hence, we tested a double square-pulsed 14C input with an interval (double-pulsed event). Figure 4 shows the best-fitted profile on the Δ14C data set and the input 14C production rates of double-pulsed event (the fitting details are in Fig. S4 and Methods). The best fitted parameters are the pulse start date of 663.7 BCE, the interval of 14 months, and the total 14C production of 1.4 × 108 [atoms/cm2], under the conditions of the exchange times of 1.5years and the share rate of 70% and 30% in the stratosphere-troposphere. For the single pulsed-event, we, also, plotted the best-fitted profile and the input 14C production rates in Fig. 4 compared with the double-pulsed event. The best fitted parameters are the pulse start date of 664.9 BCE, the pulse duration of 24 months, and the total 14C production of 1.4 × 108 [atoms/cm2], under the conditions of the exchange times of 1.5years and the share rate of 70% and 30% in the stratosphere-troposphere. Thus, the total 14C productions are almost same in both the pulsed-events and the start dates of double-pulsed events are confined in the range of the single-pulsed events (Tables S1 and S2), indicating a possibility of multiple SPEs.
The net production of 14C was (1.3 – 1.5) × 108 [atoms/cm2] for the single-pulsed event with the same fitting condition to the 775 CE event by Güttler et al.7, i.e. 2.0-year exchange time and 70%:30% share rate. The estimated production of the ~660 BCE event was 32%~40% smaller than that of the 775 CE event, e.g. 2.2 × 108 [atoms/cm2]7, but ~15% larger than that of the 994 CE event, e.g. (1.22 ± 0.37) × 108 [atoms/cm2]8. Suppose the ~660 BCE event was similar to the 775 CE and 994 CE events, then the event scale should be between the two latter events. O’Hare et al., also, showed the event scales of the ~660 BCE event by fluences based on 36Cl/10Be ratios, e.g. F30MeV fluence of the ~660 BCE event is 26 ± 27% smaller than that of the 775 CE event12, which is consistent with our result of the 14C production ratio, taking account of the error. To compare the event scale, we calculated 14C production rates for the contemporary SPEs observed in 1956 and 197220,21 using Phits simulator22 and calculation method by Kovaltsov et al.23. (see Methods). The calculation presents that the net 14C production of the ~660 BCE event is equivalent to roughly 50 times larger than that of SPE1956 which is the strongest of the known hard spectrum events in the cotemporary observed SPEs. Consequently, the single-pulse scenario (as proposed in O’Hare et al.12) is consistent with the new 14C data, while a longer production is also possible.
In conclusion, we reveal that it is possible that the ~660 BCE event has rather a long-time 14C injection up to 3.4 years in the atmosphere by the finely measured 14C data set. The 14C measurements of earlywoods and latewoods provide us a constraint on the occurrence time of the SPEs that brought the ~660 BCE event. Our research demonstrates that 14C analysis using a finely cut tree ring is advantageous in the field of remarkable cosmic event studies. The estimated scale of the ~660 BCE SPE is much larger than historically recorded SPEs. Our finding indicates that such extreme events might have occurred consecutively within a few years.
Methods
Dating of choukai–jindai cedar
Calendar-dating of the outer tree ring in contact with the bark was independently estimated as 477.5 ± 12.5 BCE and 460 ± 8 BCE by a wiggle matching from two kinds of 14C measurements using liquid scintillation counter and accelerator mass spectrometer (AMS), respectively, indicating the consistent age24,25. On the other hand, dendrochronology indicated that a remarkable sector collapse of Choukai volcano occurred at 466 BCE by an analysis of woods dug out of a vicinity of the volcano, using the dendrochronology calibration chart in Japan by Mitsutani26. Moreover, debris avalanche deposits related to volcanic eruptions were shown in a volcanology study of Choukai volcano27,28. From these, it was inferred that the wood sample was buried by the debris avalanche deposits in 466 BCE in agreement the date of wiggle matching.
Pretreatments and measurements
Each earlywood and latewood of annual tree rings were separately taken out of a block of boiled wood using tweezers. As the tree-ring was rather thick with typically 3–5 mm width, separating earlywoods and latewoods was not so difficult. Alpha (α)-cellulose was extracted for each separated sample, as it is the most reliable chemical component corresponding to the concentration of 14C taken in tree ring cell walls during growth. The α-cellulose yield from the wood sample was approximately 15% by weight. Graphite samples were produced by burning 3 mg of the cellulose. The weight ratios of the graphite to 1 mg of iron powder were kept between 0.6 and 1.2. Measurements of 14C in the graphite samples were carried out using a 500 KV tandem accelerator at the Yamagata University (YU–AMS)29. The 14C concentrations for the graphite samples were obtained using the measured values of the 14C/12C ratio for samples and 4990 C oxalic acid, which is a standard sample. The measured ratio for a blank sample using IAEA-C1 was typically 1.0 × 10−15. Duplicate to sextuple measurements in most of earlywoods and latewoods in 669–633 BCE were performed with the accuracy of approximately 2.3‰ in every single measurement. Reproducibility of the multiplicate measurements was checked by Chi-squared test. Only two out the 70 items in the data set included one rejected 14C value at 95% confidence level. Hence, we expressed the measured data set by the weighted averages and the errors in the weighted averages for multiple measurements.
Difference in oak and cedar physiologies
Oak is a ring-porous angiosperm whose leaves sprout from May and fall down in late October. A row of vessels with a large diameter in oak trees is positioned in an initial portion of earlywoods and its lignification is through within approximately four weeks before leaf-out in the year30,31. With this, the photosynthates forming its xylem should be stored for production in the parenchyma cells at the previous year, meaning, the 14C concentrations in alpha cellulose constructing the xylem are taken up from the atmosphere of the preceding year. On the contrary, cedar is a type of conifers, which are more primitive plants transporting water through tracheids than angiosperms. In cedars, earlywood is produced in spring and early summer while latewood is formed in late summer30. At approximately 350 km south along the Japan sea side from Choukai volcano, the 14C contents of a cedar tree ring for 10 years from 1989 were comparable in each year to the observed atmospheric 14C concentrations in the area during mid-June and early-September, indicating that the cedar tree ring reflects the 14C concentration in the atmosphere of the year it grows32.
Calculation of 14C Production rate
Figure S5 depicts an exponential function of the Δ14C profile fitted to the 14C data set of earlywoods and latewoods using least squares method. The decay constant was 13.0 ± 1.4 years, which is comparable to the value 14.2 years from a contemporary Spanish pine tree ring due to atomic detonations in the 1960s33. It evidently indicates a process of carbon cycle in which a pulsed 14C is injected into the atmosphere, and then the 14C are dissolved in the surface ocean. We simulated the Δ14C profile for pulsed 14C productions using the 11-box model7. The calculation procedures were almost similar to that of Güttler et al.7, except that we used 14C half-life of 5730 years. We used fluxes between boxes in Fig. 3 of Güttler et al.7, and initial state of 14C inventories in Table 1 of Güttler et al.7. In the simulation, we examined the pulsed 14C productions for the two kinds of exchange times of 1.5 years and 2.0 years16 between the stratosphere and troposphere, taking account of the three cases of the 14C productions shared into two boxes of the stratosphere and troposphere, i.e. stratosphere:troposphere = 70%:30%, 80%:20%, and 90%:10%.
The fitting calculation was conducted for 30 Δ14C data of earlywoods and latewoods between 669 BCE and 655 BCE. We assumed the growth periods of earlywoods and latewoods as April–July and August–September, respectively; therefore, the measured Δ14C values of earlywoods and latewoods were compared through averaging the simulated values of the two periods. The validity between the measured and simulated data sets was evaluated using reduced χν2 values (χ2/ν, ν: degree of freedom). The injected pulse shape was simplified to a square pulse of 14C production in the atmosphere. Two types of pulsed inputs were examined, which indicate a single pulsed event and a double pulsed event with an interval. Three fitting parameters coming down to the dof = 27 are employed in the single pulse input, i.e. pulse height, pulse width, and pulse start time, which express total 14C production, duration, and beginning time for a single-pulsed event, respectively. In the double pulsed input, four fitting parameters coming down to the dof = 26 are employed, i.e. pulse heights of the first and second pulses, time interval between the first and second pulses, and the first pulse start time. For the double pulsed event, we simply fixed to one month the pulse widths of the first and second pulses. The pulse width, the beginning time of the event, and the time interval were shown with a month resolution. The fitting results are shown in Figs. S3 and S4. Table S1 shows the fitting results for each condition.
14C Production rate by SPEs
We calculated an altitude distribution of 14C production up to 40 km in the atmosphere with the proton energy range of 20 MeV to 5 GeV using Phits simulator22. The resulting 14C yield function was very consistent with the previous works23,34. From the approximately 60 GLE events since 1956, two GLE events of SPE1956 and SPE1972 were chosen as typical SPEs representing hard and soft energy spectra, respectively20,21. The net 14C productions by SPE56 and SPE 72 are 2.8 × 106 [atoms/cm2] and 6.2 × 105 [atoms/cm2], respectively, calculated at every latitude taking account of a geomagnetic cut off rigidity35 from their fluence spectra and the altitude production yields. The production rate of SPE56 was consistent with the value 2.90 × 106 [atoms/cm2] by Kovaltsov et al.23. Based on our calculation, the ~660 BCE event is 52–53 times larger than SPE56, and 230–240 times larger than SPE72 if the energy spectrum of the ~660 BCE event is comparable to the SPEs.
Data set
The data set that supports the findings of this study is shown in Table S3.
References
Miyake, F., Nagaya, K., Masuda, K. & Nakamura, T. A signature of cosmic-ray increase in AD 774-775 from tree rings in Japan. Nature 486, 240–242 (2012).
Usoskin, I. G. et al. The AD775 cosmic event revisited: the Sun is to blame. Astron. Astrophys. 552, L3, https://doi.org/10.1051/0004-6361/201321080 (2013).
Jull, A. J. T. et al. Excursions in the 14C record at A.D. 774–775 in tree rings from Russia and America. Geophys. Res. Lett., 41, https://doi.org/10.1002/2014GL059874 (2014).
Miyake, F., Masuda, K. & Nakamura, T. Another rapid event in the carbon-14 record of tree rings. Nat. Commun. 4, 1748, https://doi.org/10.1038/ncomms2873 (2013).
Fogtmann-Schulz, A. et al. Cosmic ray event in 994 C.E. recorded in radiocarbon from Danish oak, Geophys. Res. Lett., 44, https://doi.org/10.1002/2017GL074208 (2017).
Miyake, F. et al. Cosmic ray event of A.D. 774–775 shown in quasi-annual 10Be data from the Antarctic Dome Fuji ice core. Geophys. Res. Lett. 42, 84–89, https://doi.org/10.1002/2014GL062218 (2015).
Güttler, D. et al. Rapid increase in cosmogenic 14C in AD 775 measured in New Zealand kauri trees indicates short-lived increase in 14C production spanning both hemispheres. Earth and Planetary Science Letters 411, 290–297, https://doi.org/10.1016/j.epsl.2014.11.048 (2015).
Mekhaldi, F. et al. Multiradionuclide evidence for the solar origin of the cosmic-ray events of AD 774/5 and 993/4. Nat. Commun. 6, 8611, https://doi.org/10.1038/ncomms9611 (2015).
Büntgen, U. et al. Tree rings reveal globally coherent signature of cosmogenic radiocarbon events in 774 and 993 CE. Nat. Commun. 9, 3605, https://doi.org/10.1038/s41467-018-06036-0 (2018).
Uusitalo, J. et al. Solar superstorm of AD 774 recorded subannually by Arctic tree rings. Nat. Commun. 9, 3495, https://doi.org/10.1038/s41467-018-05883-1 (2018).
Park, J., Southon, J., Fahrni, S., Creasman, P. P. & Mewaldt, R. Relationship between solar activity and Δ14C peaks in AD 775, AD 994, and 660 BC. Radiocarbon 59(4), 1147–1156 (2017).
O’Hare, P. et al. Multiradionuclide evidence for an extreme solar proton event around 2,610 B.P. (∼660 BC). PNAS 116(13), 5961–5966, https://doi.org/10.1073/pnas.1815725116 (2019).
Stuiver, M. & Braziunas, T. F. Sun, ocean, climate and atmospheric 14CO2: An evaluation of causal and spectral relationships. Holocene 3, 289–305, https://doi.org/10.1177/095968369300300401 (1993).
Pavlov, A. K. et al. AD 775 pulse of cosmogenic radionuclides production as imprint of a Galactic gamma-ray burst. MNRAS 435, 2878–2884, https://doi.org/10.1093/mnras/stt1468 (2013).
Stuiver, M. & Polach, H. A. Discussion: reporting of 14C data. Radiocarbon 19, 355–363 (1977).
Nydal, R. Further Investigation on the Transfer of Radiocarbon in Nature. J. Geophys. Res. 73(12), 3617–3635 (1968).
Yasuike, K., Yamada, Y. & Komura, K. Long-term variation of 14C concentration in atmospheric CO2 in Japan from 1991 to 2000, J. Radioanal. Nucl. Chem. 275, 313–323 (2008).
Suzuki, K. et al. Precise comparison of 14C ages from Choukai Jindai cedar with IntCal04 raw data. Radiocarbon 52(4), 1599–1609, https://doi.org/10.1017/S0033822200056332 (2010).
GLE database: https://gle.oulu.fi.
Webber, W. R., Higbie, P. R. & McCracken, K. G. Production of the cosmogenic isotopes 3H, 7Be, 10Be, and 36Cl in the Earth’s atmosphere by solar and galactic cosmic rays. J. Geophys. Res. 112, A10106, https://doi.org/10.1029/2007JA012499 (2007).
Tylka, A. & Dietrich, W. A new and comprehensive analysis of proton spectra in ground-level enhanced (GLE) solar particle events, 31th International Cosmic Ray Conference, Universal Academy Press, Lodź Poland (2009).
Sato, T., Yasuda, H., Nitta, K., Endo, A. & Sihver, L. Development of parma: Phits based analytical radiation model in the atmosphere. Radiat. Res. 170, 244–259 (2008).
Kovaltsov, G. A., Mishev, A. & Usoskin, I. G. A new model of cosmogenic production of radiocarbon 14C in the atmosphere. Earth and Planetary Science Letters 337–338, 114–120 (2012).
Sakurai, H. et al. 14C dating of ~2500-yr -old Choukai Jindai cedar tree rings from Japan using highly accurate LSC measurement. Radiocarbon 48(3), 401–408 (2006).
Takahashi, Y. et al. Comparison of 14C ages between LSC and AMS measurements of Choukai jindai cedar tree rings at 2600 cal BP. Radiocarbon 52, 895–900, https://doi.org/10.1017/S0033822200045987 (2010).
Mitsutani, T. Dendrochronology and cultural assets. Art of Japan 421:92–3 (2001) In Japanese.
Ohba, T. et al. Eruptive History at Chokai Volcanoduring the last 4000 years: Implication from Ash Layers Preserved in Peat Soil., Kazan., 57, 65–76 In Japanese with English abstract. (2012).
Inokuchi, T. Gigantic Landslides and Debris Avalanches on Volcanoes in Japan –Case Studies on Bandai, Chokai and Iwate Volcanoes–. Report of the National Research Center for Disaster Prevention. 41, 163–275 In Japanese with English abstract (1988).
Tokanai, F. et al. Present status of YU-AMS system. Radiocarbon 55(2), 251–259, https://doi.org/10.1017/S0033822200057350 (2013).
Speer, J. H. Fundamentals of Tree-Ring Research, The University of Arizona Press, (2010).
Takahashi, S. Shinrin Gijyutsu, 887, 28–31 In Japanese (2016).
Yamada, Y., Yasuike, K. & Komura, K. Relationship between Carbon-14 Concentrations in Tree-ring Cellulose and Atmospheric CO2. Journal of Nuclear and Radiochemical Sciences 9(2), 41–44 (2008).
Rakowski, A. Z. et al. Radiocarbon concentration in modern tree rings from Valladolid, Spain, Nuclear Instruments and Methods in Physics. Research B 268, 1110–1112, https://doi.org/10.1016/j.nimb.2009.10.111 (2010).
Poluianov, S. V., Kovaltsov, G. A., Mishev, A. L. & Usoskin, I. G. Production of cosmogenic isotopes 7Be, 10Be, 14C, 22Na, and 36Cl in the atmosphere: Altitudinal profiles of yield functions. J. Geophys. Res. Atmos. 121, 8125–8136, https://doi.org/10.1002/2016JD025034 (2016).
Lifton, N., Smart, D. F. & Shea, M. A. Scaling time-integrated in situ cosmogenic nuclide production rates using a continuous geomagnetic model. Earth and Planetary Science Letters 268, 190–201 (2008).
Acknowledgements
We are deeply indebted to Dr. Kayo Suzuki and Dr. Yui Takahashi for basic preparations of the Choukai-Jindai cedar sample. We appreciate a reviewer for very important suggestion about data analytical methods. This work was supported by JSPS KAKENHI grant number JP10640245 and JP16H06005.
Author information
Authors and Affiliations
Contributions
H.S. designed the study. H.S., F.T., and To.M. conducted the 14C measurements. H.S., F.T., H.M., and F.M. performed the data analyses. H.S. and F.M. modelled the 14C production rates with a carbon cycle calculation with the comments by K.H. and K.M. M.S., Ta.M., and M.O. commented of the tree-ring samples. H.S. and F.M. mainly performed the writing the manuscript based on the joint discussions by all authors.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Sakurai, H., Tokanai, F., Miyake, F. et al. Prolonged production of 14C during the ~660 BCE solar proton event from Japanese tree rings. Sci Rep 10, 660 (2020). https://doi.org/10.1038/s41598-019-57273-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-019-57273-2
This article is cited by
-
The timing of the ca-660 BCE Miyake solar-proton event constrained to between 664 and 663 BCE
Communications Earth & Environment (2024)
-
Absolute dating of the European Neolithic using the 5259 BC rapid 14C excursion
Nature Communications (2024)
-
Global ozone loss following extreme solar proton storms based on the July 2012 coronal mass ejection
Scientific Reports (2023)
-
Extreme Solar Events: Setting up a Paradigm
Space Science Reviews (2023)
-
Cosmogenic radionuclides reveal an extreme solar particle storm near a solar minimum 9125 years BP
Nature Communications (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.