Introduction

The rate at which deeply-sourced magma enters a magmatic system (magma supply rate) and the rate at which it comes out of it (magma output rate) are two essential parameters for estimating the endogenous growth of a volcano and for predicting its behaviour in the future1. These two parameters determine whether a magma chamber is in non-equilibrium steady state (i.e the magma input equal to the output), or whether it is subject to accumulation (pressurization) or emptying (depressurization) phenomena, that necessarily have a strong control on the type and timescale of surface activity2.

The magma supply rate (Qin) is classically estimated from gas flux data (mainly sulphur dioxide), by using the so-called “petrologic method”3,4. This method is based on a simple mass balance, from which the amount of gas emitted into the atmosphere, is proportional to the amount of magma that is supplied above the exsolution level of the volatile phase3,4. Hence, by measuring the SO2 outgassed at the vent (ϕSO2, in tonnes day−1) and by assessing the volatile loss (i.e. ΔXS) suffered by the ascending magma (typically calculated as the initial volatile content in melt inclusions, minus the residual content in the groundmass), we can calculate the magma supply rate (Qin in m3 s−1) as:

$${Q}_{in}=\frac{{\varnothing }_{so2}}{{\rho }_{m}\cdot 2{\rm{\Delta }}{X}_{S}\cdot 86.4}$$
(1)

where ρm is the dense rock equivalent (DRE) magma density (kg m−3) and 86.4 is the unit’s conversion factor (from tonnes day−1 to kg s−1).

The magma output rate (Qout) is instead calculated from repeated measurements of volumes erupted over discrete time intervals, or through a space-based thermal approach5. The latter, also called “thermal proxy”, derives from an energy balance of lava flows, whereby there exists a proportionality between the area, the surface temperature, and the discharge rate that feeds lava flows6,7,8. Hence, the DRE magma output rate (Qout), can be calculated from the satellite-derived Volcanic Radiative Power (VRP) as8:

$${Q}_{out}=\frac{{\rho }_{lava}}{{\rho }_{m}}\cdot \frac{VRP}{crad}$$
(2)

where ρlava (kg m−3) is the bulk densities of the lava flow and crad (J m−3) is an empirical best-fit parameter that relates the lava discharge rate to the thermal radiation for any given rheological case8.

To these two rates of magma transport, a third parameter of vital importance needs to be considered to fully constrain the magma budget of a volcano. This is represented by the rate of magma accumulation, or withdrawal (dQm = Qin − Qout), that determines if a volcano is inflating or deflating9,10. However, because magma is compressible, only a fraction of the net magma volume entering or leaving the chamber (ΔVm = Vin − Vout) will be accommodated by the expansion/contraction of the chamber itself (ΔVc), so that10,11:

$${\rm{\Delta }}{V}_{m}=(1+\frac{{\beta }_{m}}{{\beta }_{c}})\cdot {\rm{\Delta }}{V}_{c}$$
(3)

where βm and βc are the compressibilities of magma and chamber, respectively. In turn, subsurface volume changes (ΔVc) are generally constrained from the surface displacements observed by geodetic techniques, such as GPS and InSAR12, although source geometry and material properties can also play a fundamental role in modulating the ground deformation11,13.

It is therefore clear that there must be a direct correlation between the rates of degassing, eruption and deformation, through which the magma budget is satisfied (eq. 3).

At closed-vent volcanoes (i.e. volcanoes with sealed conduits that may exhibit edifice-wide long-term ground uplift due to reservoir pressurization14), the classic model of the “volcano deformation cycle”, is generally operative2. According to this model, during inter-eruptive periods, the magma output rate (Qout) is equal to zero, so that all the magma supply (Qin) gradually inflates a chamber beneath the volcanic edifice, causing uplift. Once the eruption starts (i.e. once a critical pressure is reached at which point the chamber ruptures), Qout becomes much higher than Qin, so the volcano deflates, because of withdrawal from the magma chamber9,10. In some cases, this long term inflation/deflation cycle has shown predictable behaviours, allowing successful forecast of eruptions15,16,17.

On the other hand, it is now well established that open-vent volcanoes (i.e. volcanoes characterized by persistent degassing and continual emission of magmatic-related products directly to the atmosphere18), emit an excess of gas to that contained in the erupted magma19,20. This unbalance, commonly known as “excess degassing”19,20, has been ascribed to phenomena of endogenous or cryptic growth, in which some unerupted magma is continuously intruded in the volcano edifice, or ascends, degasses and then sinks back down the conduit to mix with a deep reservoir1,4,21,22,23,24,25. In most open-vent volcanoes this large unbalance does not translate into significant long-term surface deformation, but only into short-term, localized deformation related to shallow conduit processes14. For example, short-term deformation cycles have been observed in association with Strombolian or Vulcanian eruptions26,27 as well as with variations in the lava lake level28. Also, is not uncommon to observe deflation phenomena during major eruption phases, that temporary drain the magma chambers9,29 or the upper portion of the plumbing system30,31,32. According to some authors the lack of long-term inflation at these open-vent volcanoes cannot be explained by magma reservoirs being too deep to create detectable uplift, but rather by the possibility of magma to rise toward the surface without pressurizing the magma reservoirs14.

Therefore, is still unclear how the large amount of unerupted magma ΔVm, characterizing open-vent volcanoes is related to the subsurface volume changes ΔVc inferred from ground deformation, although the two volumes are clearly linked (eq. 3).

Mt. Etna has been object of several works focused on the balance between supplied and erupted magma, all showing that only a small fraction of magma entering the degassing zone (3–4 km b.s.l.), is actually extruded23,29,33. Most studies have examined this open-vent behaviour over periods of decades, suggesting an overall endogenous growth by intrusion and the creation of a cryptic plutonic complex within Etna’s sedimentary basement23,29,33,34. Some detailed magma budget was also presented for three individual eruptions of Mt. Etna35, suggesting that over timescale of weeks, the balance between input and output rates is variable and much more complex than previously thought. However, this study did not consider the magma budget during the periods between eruptions, thus lacking one half of the whole history. First clues on Etna’s magma budget during a sequence of short-lived paroxysms36 suggests that the majority of the magma erupted during these events was likely stored within the conduit, having gone through extensive degassing for days to weeks prior to the paroxysms. Notably, all these works23,29,33,34,35,36 focused on the magma budget of Mt. Etna over different timescale (from decades to days), but none looked at the relationships with the volcano deformation over the same timescales.

On the other hand, ground and satellite measurements of deformation suggest that Mt. Etna is affected by an overall long-term inflation trend, periodically interrupted by more or less important deflation periods29,37,38,39,40, a pattern somehow similar to the classic closed-vent volcano deformation cycle. One work first correlated this deformation pattern with the long-term bulk magma budget characterizing the 1993–2005 period29, but it does not have the sufficient temporal detail to explore the validity of the above model in the mid-, or short-term.

In this work we used satellite thermal data, derived from Moderate Resolution Imaging Spectroradiometer – MODIS (Fig. 1a) and SO2 flux data, derived from Ozone Monitoring Instrument – OMI (Fig. 1b), to constrain the magma supply and output rates (methods) characterizing the activity of Mt. Etna between 2004 and 2010 (Fig. 1c). These two independent datasets provide for the first time a clear, quantitative view of the magma accumulation and withdrawal processes affecting the volcano plumbing system at different timescales. These results are finally compared with the deformation data measured by the CGPS network during the same period38,39 and highlight the great potential of using combined satellite data to monitor quantitatively the endogenous growth of open-vent volcanoes.

Figure 1
figure 1

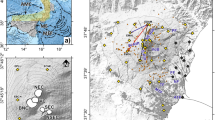
(a) Simplified map of Etna summit craters and main lava flows emplaced between 2004 and 2010. (shaded relief map derived from Tinitaly Digital Elevation Model70; http://tinitaly.pi.ingv.it/). (b) Example of OMI image acquired over Mt. Etna on 17 November 2006 showing an SO2-rich plume (colorscale refers to Dobson Units; DU) drifting hundreds of kilometres toward north-east. Based on eq. 1 the sulphur flux rate measured at that time is consistent with a magma supply rate Qin, equal to 1.6 ± 0.5 m3 s−1. (c) Coeval MODIS-MIROVA image showing the thermal anomaly produced by the lava flow descending on the Valle del Bove. According to eq. 2, the thermal flux recorded on 17 November 2006 was produced by a magma output rate, Qout, equal to 6.5 ± 1.9 m3 s−1. The evident unbalance between Qin and Qout suggests the empting of a shallow magma chamber consistent with a stage of deflation of the volcano edifice, as measured by GPS network (cfr. Fig. 4). (OMSO2 Level 2G data courtesy of Goddard Earth Sciences Data and Information Services Center - GES DISC; https://disc.gsfc.nasa.gov/). (MODIS Level 1b radiance data courtesy of LANCE-MODIS system https://lance-modis.eosdis.nasa.gov/; Base Map data courtesy of Google).

Full size image

Activity of Mt. Etna between 2004 and 2010

After ∼20 months of quiescence, and without precursory seismicity or ground deformation, a new eruption started on 7 September 2004, through a complex set of fractures opened in the summit area of Mt. Etna39. The eruption produced a voluminous lava flow (40–64 × 106 m3) within the Valle del Bove (Fig. 1c) and maintained low rate, steady conditions up to the end, occurring on 8th March 200541,42. Geophysical, geochemical and petrologic data suggest that the magma feeding the 2004–2005 activity was likely to have been stored in the shallow plumbing system during the 2000 and 2001 activity, where it experienced volatile loss and extensive crystallization41,43,44.

Few months after the 2004 eruption, between November 2005 and January 2006, the amplitude of volcanic tremor increased abruptly, although neither effusive nor paroxysmal activity was observed at the summit vents45. This event was associated with mild inflation of the summit of the volcano and with increased SO2 flux, allowing some authors to interpret it as a failed eruption, resulting from recharging of the volcanic feeder at depth (>3 km b.s.l.)45. Following this intrusive event, minor explosive activity occurred in the summit area (Bocca Nuova – BN- and Voragine –VOR - craters; Fig. 1c) during 2006, whereas the Northeast Crater (NEC; Fig. 1c) was the site of deep-seated explosions, which increased in early July 2006. The heightening of NEC activity was the prelude to the 2006 eruption that started on 14 July and that was characterised by two main phases of activity46. The first phase (14–24 July) lasted 10 days during which Strombolian and effusive activity (emitting 3–6 × 106 m3 of lavas) took place along a short fissure on the lower ESE flank of the SEC cone47. The second phase lasted from 31 August until 14 December and consisted of 20 eruptive episodes at or near the summit of the SEC cone producing ash plumes. From 12 October onward, an almost persistent effusive activity took place from a number of vents opened on the south of SEC46. (Fig. 1c). As a whole the 2006 eruptive activity produced two main lava fields emplaced on the south-west and on the south-east flank of the volcano, with a total lava volume of 20–37 × 106 m3 42,46.

After the end of the 2006 eruption, a recharge phase was indicated by continuous inflation showed by GPS measurements38,39. The recharge phase was accompanied by seven short-lived lava-fountaining episodes that erupted a total of ∼12 × 106 m3 of lava, and marked the shifting of activity from the SEC to the newly formed new SE crater (NSEC)48,49,50. The last powerful episode occurred on 10 May 2008, only 3 days before the onset of the 2008 eruption51. Unlike the previous two eruptions, the initial phase of the May 2008 eruption was preceded and accompanied by strong seismic release and by marked deformation of the volcano flanks52.

Moreover, in this case, the eruptive fissure propagated inside the Valle del Bove, producing the long-lived 2008–2009 eruption that lasted 419 days (the longest eruption since 1991–1993) and that emitted 68–74 × 106 m3 of lava42,53 (Fig. 1c). After the end of this eruption Mt. Etna was characterized only by continuous degassing and by sporadic minor explosive phenomena for 17 months49. Overall, the 2004–2010 activity of Mt. Etna was composed of 3 major eruptions, 1 intrusive event and 7 episodes of lava fountaining, that altogether erupted 151–173 × 106 m3 of lava42.

Results

The time series of Volcanic Radiative Power (VRP) recorded between 2004 and 2010 (methods) outlines a continuous thermal emissions (∼1.65 thermal detections per day) with VRP ranging from less than 1 MW to ∼8.5 GW (Fig. 2a). The 3 major eruptions (2004–2005, 2006, and 2008–2009; grey fields in Fig. 2a) as well as the 7 paroxysmal episodes occurred on 2007 and 2008 (green dashed lines in Fig. 2a) are clearly visible and they separate from the low and continuous thermal emission that characterise the entire period investigated. The statistical analysis of the VRP (log-transformed), indicates a bimodal distribution, with two distinct regimes within the entire population of data (nobs = 3320; Fig. 2c). The low thermal regime, characterized by VRP < 40 MW ( < 7.6 log-transformed), was typical of inter-eruptive periods, when the thermal source was likely produced by weak strombolian activity, hot degassing cracks within the summit craters, as well as by the cooling of previously emplaced lava flows (during the post-eruptive phases; Fig. 2a). Conversely, the high thermal regime (VRP > 40 MW) corresponds to periods of major effusive activity, which also includes the 7 lava-fountaining episodes occurred in 2007 and 2008 (Fig. 2a). It is thus clear that a change from low to high thermal regimes (peaking at 4 MW and 252 MW, respectively) corresponds to a transition from open-vent to effusive activity, in manner similar to that already observed at Stromboli volcano54.

Figure 2
figure 2

Time series of (a) Volcanic Radiative Power - VRP and (b) sulphur dioxide flux - ϕSO2 retrieved over Mt. Etna between September 2004 and February 2010 (from MODIS and OMI data, respectively; see methodology). Grey fields outline the occurrence of the three major eruptions accompanied by lava effusion. Yellow field outlines the failed eruption in winter 2005–200645. Green bars outline the seven fountaining episodes (paroxysms) occurred between 2007 and 200848. (c) Frequency of distribution for VRP datasets (log-transformed data). Note the bimodal distribution of log VRP marking the transition from the low to the high thermal regime for VRP = 40 MW (balck dashed line in (a)). (d) Frequency of distribution for ϕSO2 dataset (log-transformed data) showing unimodal pattern and arithmetic mean at log SO2 = 3.5 (ϕSO2 = 3259 tonnes day−1).

Full size image

The SO2 flux data (Fig. 2b) also indicate a continuous degassing between 2004 and 2010 (∼0.37 SO2 detections per day), but with an unimodal distribution of the data (nobs = 753; Fig. 2d). The average ϕSO2 obtained from the OMI data (Methods) is equal to 3259 tonnes day−1, in excellent agreement with the flux measured by ground measurements during 2005–200855 (see methods). It is worth nothing that during the inter-eruptive phases, the average flux was 3023 tonnes day−1, while during the 3 effusive phases (grey fields in Fig. 2) the average ϕSO2 raised to 4301 tonnes day−1. This suggests that the effusive eruptions of Mt. Etna were characterized by a bulk increase of the degassing rate of only ∼42% with respect to the persistent, long term emission.

Notably, the failed eruption that occurred during the winter of 2005–200645 (yellow field in Fig. 2) was accompanied by a monthly-long pulse of ϕSO2, while there were no anomalous thermal emissions recorded in the VRP time series (the cooling trend remained essentially unperturbed; Fig. 2a). In contrast, all the lava-fountain episodes are evident in the VRP time series (Fig. 2a), but not particularly in the ϕSO2 time series (with exception of those occurred on 29 March 2007 and 10 May 2008).

This may be due to the impulsive nature of the phenomenon (i.e. duration less than 1 day, on average) which coupled with the low sampling rate of OMI (1 image per day) makes challenging the detection of all short-lived SO2 emissions associated to these episodes36.

In general, it can be observed that both thermal and gas emissions have been continuous during 2004–2010 (Fig. 2a,b), remarking the persistent open-vent behaviour of Mt. Etna. Nevertheless, the thermal flux shows wide and evident increases during major eruptions (almost two orders of magnitude), leading to a bimodal distribution of VRP (Fig. 2c). On the contrary, ϕSO2 shows an unimodal distribution (Fig. 2d) and a more homogeneous trend, with smoothed, monthly-long oscillations accompanying the major eruptions and the intrusive episode (Fig. 2b).

Discussion

The magma supply rate and the magma output rate have been estimated using eqs 1 and 2, whose detailed parametrization is described in the method section. However, some aspects must be clarified regarding the interpretation of these parameters and their volcanological significance at open-vent volcanoes.

The magma supply rate calculated by eq. 1 (Qin) is in fact a “magma degassing rate”, since it provides an estimate of the quantity of magma that rises above the exsolution level of SO2 and degasses1,19,23. Therefore, it should be interpreted in a broad sense, as the rate of magma entering the shallow portion of the magma system where a convective degassing cell is operative (about 3–4 km b.s.l. for Etna4,23,33).

On the contrary, the magma output rate (Qout) calculated through eq. 2 provides an estimate of the lava discharge rate during the effusive phases8. However, during phases of open-vent activity, the magma output rate assumes an ambiguous meaning, and should be considered an “apparent output rate”, since there is no net extrusion of lava. In this case, the application of eq. 2 can still be used to infer the rate at which the magma reaches the surficial levels in the conduit (i.e., few tens/hundreds meters at most), where it radiates and cools before being cycled back8,36,56. In this scenario, the threshold separating the low thermal regime (open-vent activity) from the high radiating regime (effusive activity) indicates that an ascending volumetric flux of 0.16 ± 0.05 m3 s−1 represents a physical barrier for the convective magma transport within the conduit(s) of Mt. Etna. Above this threshold the magma can no longer be recycled in the conduit and must be partially or completely extruded.

Accordingly, the two magma fluxes (Qin and Qout) sample the rate of magma transport at different depths56, being the SO2‐derived flux relative to the magma circulation above the exsolution level of SO2, and the IR‐derived flux relative to very shallow (i.e. during open-vent) or surficial (during effusive activity) levels.

The time series of Qin and Qout, as well as the respective cumulative volumes (Vin and Vout) estimated for the 2004–2010 period, are shown in Fig. 3. The direct comparison of these rates clearly shows the overall unbalance due to the excessive degassing of Mt. Etna, with supply rate generally exceeding the output rate. This unbalance is particularly evident during inter-eruptive periods, while it is cancelled, or sometimes reversed, during the major eruptive phases (Fig. 3a).

Figure 3
figure 3

(a) Time series of magma input (Qin) and output (Qout) rates, derived from SO2 and thermal data (eqs 1 and 2; see methods for details). (b) Cumulative volumes of magma supplied (Vin) and erupted (Vout) throughout the 2004–2010 period. Uncertainties of ±30% in fluxes/volumes values (see methods) is shown by the respective errorbars. Coloured fields and bars as in Fig. 2.

Full size image

In general, our volume calculations (Fig. 3b) indicate that between October 2004 and February 2010, 257.0 ± 77.1 × 106 m3 of magma entered the system with an average Qin of 1.65 ± 0.49 m3 s−1 (52.1 ± 5.6 × 106 m3 y−1), while 164.0 ± 49.2 × 106 m3 came out of the system with an average Qout of 0.92 ± 0.28 m3 s−1 (29.1 ± 8.73 × 106 m3 y−1). This unbalance results in a net magma accumulation rate equal to 22.9 ± 13.7 × 106 m3 y−1 (Fig. 4a), a value similar to that measured during 1975–1995 period (∼25.1 × 106 m3 y−1)23, but lower than the accumulation rate characterizing the 1993–2005 period (∼64.1 × 106 m3 y−1)33.

Figure 4
figure 4

(a) Time series of cumulative ΔVm, retrieved from magma budget calculations (ΔVm = Vin − Vout); (b) time series of the length variations between the EMAL and EMGL CGPS stations (inset), modified from Bruno et al.38. Note the good correlation between the overall trends of the two datasets, as well as the excellent correspondence between the main phases of magma accumulation/withdrawal (a), leading to coeval and coherent inflation/deflation cycles (b). Coloured fields and bars as in Fig. 2.

Full size image

Although the long-term magma budget indicates a general accumulation of magma, the trend shows mid- and short-term variations of ΔVm that can be compared with the deformation data reported for the same period. In this regard, we have used the published baseline distance between two CGPS stations (located on the west side of Etna; inset in Fig. 4b), which highlight the deformation pattern characterizing Mt. Etna activity between 2004–201038 (Fig. 4b).

This comparison shows an excellent correlation between the two datasets, suggesting that the magma budget retrieved from SO2 and thermal data is closely linked to the volcano deformation. All the main stages of inflation (positive ΔVm) are actually corresponding to periods of “excess degassing” produced by magma accumulation at depth, without net extrusion of magma. These stages essentially correspond to the open-vent activity, or to periods characterized by magmatic intrusion, like the one occurred in the winter 2005–2006 (yellow field in Fig. 4). On the other hand, all periods of magma drainage (negative ΔVm) occurred during deflation stages, and in particular during specific periods of the main effusive eruptions (grey fields in Fig. 4a).

By using similar GPS analysis, other authors39 identified 6 stages of inflation/deflation occurred between 2004 and 2007 (named from T5 to T10; Table 1). For each stage they estimated the depth (D) of the deformation source as well as the magma chamber volume changes (ΔVc) that better explain the ground deformation pattern (Fig. 5a). These data indicate the presence of a magma chamber at 3.5–5.5 km b.s.l. where the pressurization/depressurization occurs. The volumes changes (ΔVc) inferred for each stage span from −8.4 × 106 m3 (T5) to +6.4 × 106 m3 (T7), and compare very well with the volumes calculated from the magma budget approach (ΔVm; Table 1). This excellent correlation (R2 = 0.989; Fig. 5b) indicates a ΔVm/ΔVc ratio spanning from 2.4 to 6.5, with a best-fit value equal to 3.6 (Fig. 5b). As described previously this ratio describes the compressibility of the system (eq. 3), which for bubble poor basaltic magmas stored at shallow crustal levels spans from 1.2 to 7.710,11. These results are thus consistent with the occurrence of efficient magma convection in the upper magmatic systems of Mt. Etna allowing degassed magma to sinks back and accumulate within the deforming magma chamber.

Table 1 Synthesis of magma budget volumes (ΔVm) and subsurface volume changes (ΔVc) for the six inflation/deflation stages occurred at Mt. Etna between 2004 and 2007
Full size table
Figure 5
figure 5

(a) Time series of cumulative ΔVm, retrieved from Qin and Qout (red line), and cumulative ΔVc, inferred from deformation data39 (black dashed line) for the 2004–2007 period. Six stages of inflation/deflation are identified and named (from T5 to T10) after Palano et al.39. (b) Correlation between ΔVm and ΔVc for the six analysed stages. The ratio ΔVm/ΔVc = 3.6 is consistent with a magma chamber filled by bubble poor basaltic magma10,11.

Full size image

These results have a series of important implications for the long- and short- term monitoring of volcanoes showing persistent activity.

Firstly, we demonstrated that the endogenous growth of Mt. Etna volcano can be quantified in great detail by means of satellite-derived thermal and SO2 flux data, and that the resulting magma budget (ΔVm) is compatible with the volume changes (ΔVc) inferred by deformation measurements, and with the bulk compressibility of the magmatic system.

Starting from this evidence the following considerations can be drawn for any open-vent volcano:

  • each open-vent volcano that degasses more magma than it emits, grows in an endogenous way;

  • this growth can be monitored by means of satellite-derived thermal and SO2 measurements (allowing the calculation of ΔVm; eqs 1 and 2), or by means of deformation measurements (allowing the calculation of ΔVc; eq. 3);

  • The ratio between ΔVm and ΔVc is a function of the compressibility of the system and can be used to to infer depth of the accumulation zone as well as the magma and the crustal parameters;

  • The continuous monitoring of ΔVm and ΔVc allows to quantify the shallow magma budget of the system. Each temporal decorrelation between these two volumes is indicative of a change in (i) position of the magma accumulation zone (ii) initial volatile’s content (iii) thermo-elastic properties of the system.

  • if open-vent activity is not accompanied by long-term detectable deformation (i.e. Merapi, Popocatépetl, Colima14) the endogenous growth possibly occurs within a very compliant crust or at shallow depths, or in correspondence of a bubble-rich, highly compressible magma chamber, characterized by a high ΔVm/ΔVc ratio13.

We also demonstrated that thermal and gas emissions are not necessarily correlated throughout effusive activity3, nor during open-vent activity (Fig. 2). This implies that, even at persistently degassing volcanoes, the high-temperature thermal anomalies measured by satellite57 (i.e. Te > 600 K), are not necessarily correlated to the emission of gas itself, as hypothesized by58. In fact, the magmatic intrusions occurred between December 2005 and January 2006, although it produced an evident pulsation of SO2 flux from the summit craters (>20 ktonnes day−1), it was not accompanied by evident thermal anomalies, just because the magma remained at depth (>3 km b.s.l.45). Accordingly, we here stress that also at persistently degassing volcanoes, the detection of MIROVA thermal anomalies, advise that the magma column has reached the surface, or is at very shallow depth.

A first recent comparison of thermal anomalies, degassing, and surface deformation, derived from satellite data at 47 of the most active volcanoes in Latin America, provides clear evidence of the potential of applying this approach at regional scale59. Equations 1, 2 and 3 can be applied in near real time if appropriate parametrization has been validated such as for Mt. Etna. We believe that in the future, the technological advancement represented by the new infrared, ultraviolet and microwave satellite’s sensors will allow a sufficient degree of detail for monitoring on a daily basis the endogenous growth of open-vent volcanoes and, more generally, the efficiency of magma transport within the magmatic plumbing systems.

Methods

Volcanic Radiative Power (VRP) via MODIS-MIROVA data

Thermal emissions of Mt. Etna, have been obtained by analysing the data provided by the Moderate Resolution imaging Spectroradiometer (MODIS). Two MODIS sensors, in orbit since February 2000 and May 2002 (on board of Terra and Aqua NASA’s satellites, respectively) provide, on average, 4 images per day of the entire globe, with a spatial resolution of 1 km in the infrared. The data were processed through the MIROVA system57, an automatic volcanic hotspot detection system, based on the analysis of Middle Infrared (MIR) images. This system allows to detect, locate and quantify the thermal emissions sourced by volcanic activity by calculating the Volcanic Radiative Power (VRP). The VRP is a measurement of the heat flux radiated almost exclusively by hot lava surfaces (i.e. having effective temperature above 600 K) and is calculated via the MIR method60 as:

$$VRP=18.9\cdot {A}_{pixel}\cdot \sum _{i=1}^{npix}{({R}_{\mathrm{MIR},alert}-{R}_{{\rm{M}}IR,bk})}_{i}$$
(4)

where RMIR,alert is the pixel integrated MIR radiance of the ith alerted pixel, RMIR,bk is the MIR radiance of the background, Apixel is the pixel size (1 km2 for the resampled MODIS pixels), and 18.9 is a constant of proportionality60. The VRP has an uncertainty of ±30%60 which cover the possible insulation conditions (integrated surface temperature from 600 K to 1500 K) of the observed lava flow (not shown in Fig. 2a for graphical clarity).

In this work, we analysed 8903 images acquired over Mt. Etna between 1 August 2004 and 31 January 2010. Within this dataset MIROVA detected a thermal anomaly in 3320 images (∼37%) allowing the construction of the VRP time series shown in Fig. 2a. The presence of clouds (or ash plumes), as well as the poor viewing geometry conditions may prevent or affect the thermal signal detected by the satellite57. In volcanoes that exhibit persistent thermal anomalies (i.e more than one a day; as for Etna) a practical way to overcome this attenuation is to considered the maximum daily VRP (Supplementary Dataset) as the most reliable value to calculate the daily magma output rate57. The latter has been calculated by using the eq. (2), where a single coefficient, called radiant density (crad in in J m−3) describes the relationship between volumetric and radiant flux appropriate for the observed eruption8. An exhaustive review of the application of this method over basaltic lava flows is provided by61.

Here we used a radiant density of 2.5 × 108 J m−3 which has been already calibrated for Etnean lava and provides estimates of magma output rate with an uncertainty of ±30%8,57. According to57, this uncertainty embeds all the possible emplacement conditions (rheological, topographic and insulation) that characterize the Etnean lava flows. In order to obtain dense rock equivalent magma output rates (Qout), we assumed ρm = 2600 kg m−3, and ρlava = 2350 kg m−3 to give a bulk flow vescicularity of about 10%62.

SO2 flux (ϕSO2) via Ozone Monitoring Instrument (OMI)

The OMI is one of the four instruments on board of AURA platform dedicated to monitor solar backscatter radiation at wavelengths spanning from 270 to 500 nm (visible and ultraviolet). It provides a daily global coverage in 14 orbits since the 1st October 2004. Each image has a complete swath of 2600 km and a nominal pixel spatial resolution of 13 × 24 km at nadir. Here we used the OMSO2 Level 2G (0.125° × 0.125°) global product63 that contains estimates of the total SO2 vertical column density (VCDSO2; in D.U.), assuming different centres of mass altitudes (CMAs), at 0.9 km (Planetary Boundary Layer, PBL), 3 km (Lower tropospheric, TRL), 8 km (Middle tropospheric, TRM), and 17 km (Lower stratospheric, STL), respectively. Since 2010, a technical issue on the instrument’s field of view makes certain rows of the OMI swath unusable for SO2 retrieval. This led the occurrence of 2–3 days-long gaps between consecutive OMI observations that corrupt the quantification of the long-term degassing budget. Here, we processed OMI data acquired between 1st October 2004 to 1st February 2010, consisting of 1831 images.

To detect SO2 emitted from Mt. Etna we adopted the same procedure described in64, composed by the following steps:

  1. (i)

    Extraction and cropping of mask of 20° × 20° centred on Mt. Etna, for any CMA product;

  2. (ii)

    Calculation of a contextual threshold at PBL level, defined by Tso2 = μ + 3σ, where μ and σ represent the mean and the standard deviation of those pixels within the mask having VCDSO2 < 1 D.U (at PBL level);

  3. (iii)

    Recognition and definition of groups of adjacent pixels (clusters) that positively exceed this threshold;

  4. (iv)

    Visual inspection and manual selection of all the clusters attributed to the Etna’s plume.

For any image where at least one cluster was selected, we calculated the SO2 mass burden (MSO2, in tonnes), at PBL, TRL, TRM and STL layers, by using the following equation, as proposed by65:

$${M}_{SO2}=0.0285\cdot {A}_{pix}\sum _{i=1}^{npix}VC{D}_{SO2}$$
(5)

where npix, Apix and VCDSO2 represent, the number of alerted pixels, the pixel area (in km2) and the vertical column density of SO2 (in D.U.), of the selected plume.

For Mt. Etna plume we used the SO2 mass retrieved for PBL and TRL layers, because the information on the typical altitude reached by the volcanic plume related to the activity of the analysed volcanoes (less than 5 km). In particular, we used the PBL level for the most of the cases which provide good results to quantify passive degassing emitted by open vent activity66. However, during more powerful plume emissions, hereby defined as plumes producing more than 20 ktonnes in the PBL, we used the mass burden calculated for TRL layer.

To convert the mass burden (MSO2) into SO2 flux (ϕSO2) we used the “delta-M” method65, according which:

$${\varphi }_{SO2}=\frac{1}{\tau }\cdot \frac{{M}_{i}-({M}_{i-1}\cdot {e}^{-\frac{{\rm{\Delta }}t}{\tau }})}{1-{e}^{-\frac{{\rm{\Delta }}t}{\tau }}}$$
(6)

where Δt is the time interval (days) between two consecutive mass estimates Mi and Mi−1, and τ is the SO2 e-folding time, an SO2 loss term that takes into account chemical, transport and dilution processes inside the plume67. We settled τ equal to 2 days, which has been proved to be an optimized value for a continuously degassing volcano in a stable wind regime68. The resulting time series consists of 754 discrete measurements of ϕSO2 (Supplementary Dataset) which are compared with ground measurements (Fig. 6) obtained with the FLAME network and traverse method for the same period55. The uncertainty in the estimation of ϕSO2 using eq. 6 derives from the complex combination of the uncertainties in the selection of the proper center of mass altitudes (hence MSO2) as well as on the assumed SO2 loss term (τ). Although the exact error of each of these parameters remains difficult to quantify (since it may vary from image to image) the excellent agreement with ground measurements, in terms of average values (3259 tonnes day−1 from OMI; 3530 tonnes day−1 from Flame network; 3250 tonnes day−1 from traverse method) and trend (Fig. 6), provides a first order validation for ϕSO2 derived from OMI whose uncertainty is considered comparable with ground measurements (i.e. ±30%55).

Figure 6
figure 6

Comparison between OMI-derived SO2 flux (eqs 5 and 6) and ground measurements provided by Flame network and traverse method, respectively (modified from Salerno et al.55).

Full size image

Finally, to convert the ϕSO2 into magma supply rate (Qin) we used the eq. (2) by assuming a total sulfur loss ΔS, equal to 3600 ppm. This value is derived from the maximum initial S content measured on melt inclusion of Mt Etna69 and assuming a complete volatile loss during magma ascent. Note that the assumption of a lower initial sulphur content or an incomplete loss of the volatile during the rise of the magma, produces higher values of Qin. Consequently, the results from eq. 1 are to be considered minimum values on which the uncertainty of ϕSO2 (±30%) is propagated.