Abstract
We report that in an in-plane magnetised magnetic film the in-plane direction of a propagating spin wave can be changed by up to 90 degrees using an externally induced magnetic gradient field. We have achieved this result using a reconfigurable, laser-induced magnetisation gradient created in a conversion area, in which the backward volume and surface spin-wave modes coexist at the same frequency. Shape and orientation of the gradient control the conversion efficiency. Experimental data and numerical calculations agree very well. Our findings open the way to magnonic circuits with in-plane steering of the spin-wave modes.
Similar content being viewed by others
Introduction
Spin waves, and their quanta magnons, are considered as the information carriers for next generation data-processing devices1,2,3,4,5,6. Here, in-plane magnetised films are preferred due to the minimised demagnetisation energy. Important building blocks for magnonic circuits have already been realised, e. g. magnon transistors7, majority gates8,9 or multiplexers10. However, the dispersion relations of the corresponding spin waves, namely backward volume (BVMSWs) and surface spin-waves (MSSWs) are strongly anisotropic and do not coexist at the same frequency. BVMSWs propagate along the static magnetisation and MSSWs propagate perpendicularly, respectively. For a fixed saturation magnetisation and a fixed external magnetic field, the frequencies of MSSWs lay above the ferromagnetic resonance (FMR) frequency whereas the frequencies of BVMSWs lay below. This approximation is valid for dipolar magnetostatic systems in a limited range of the wavevectors and frequencies, the exchange contribution to the spin-wave energy can be neglected in the studies. A change in the propagation direction requires mode conversion between MSSWs and BVMSWs, which energy and momentum conservation obviously prohibit.
To overcome this problem two roads are commonly followed: The frequency gap is adjusted via non-linear multi-magnon11,12,13,14 or multi-particle scattering processes (e.g. Brillouin or Raman scattering)15,16, via non-stationary mechanisms as in dynamic photonic/magnonic crystals17,18, and in the Doppler effect19,20,21. Or, the spin-wave momentum (the propagation direction) is changed via non-uniform magnetic media10,22,23,24,25,26,27 or via lateral confinement of the magnetic structures28,29. In the latter case, the locally varying magnetic parameters shift the spin-wave dispersion relations and, hence, allow for the conversion between different spin-wave modes. However, the usual dimensions δx of such inhomogeneities are small in order to provide a large change in the spin-wave momentum \(|{\overrightarrow{k}}_{{\rm{inh}}}|\propto 1/\delta x\). Such highly localised inhomogeneities are associated with undesirable spin-wave reflections and offer no flexibility in spin-wave steering.
Here, we present an gradual and reflection-less approach to change the spin-wave propagation using graded index spin-wave media extending over sizes δx much larger than the spin-wave wavelength \(\lambda =2\pi /|\overrightarrow{k}|\ll \delta x\). A continuous transformation of spin-wave momentum in magnetisation gradients leads to highly-efficient mode conversion over a wide frequency range. We demonstrate the control of spin-wave propagation by optically reconfiguring the media to tune the transformation of spin-wave momentum.
Results
Conversion principle
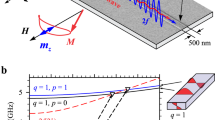
Let us discuss the mechanism behind the two-dimensional magnon guiding for the case of converting a pure BVMSW into a pure MSSW, propagating perpendicularly to each other. Figure 1a depicts this configuration. The pure initial and final modes propagate in regions of the magnetic waveguide with saturation magnetisation MS,1 (blue region) and MS,2 (red region), respectively. To obey energy conservation, the dispersion relations of the two modes have to overlap, requiring MS,2 < MS,1 (see solid blue line and dashed red line in Fig. 2b, overlap is found in the grey marked conversion area). To additionally fulfil momentum conservation, the wavevectors have to be transformed into each other. This smooth transformation is enabled by a gradient of the saturation magnetisation perpendicular to the initial propagation direction (grey bordered area in Fig. 1a) and spatially represents the area in which the dispersion relations overlap. To illustrate the flow of spin-wave energy during the conversion process, the local spin-wave group velocities \({\overrightarrow{v}}_{{\rm{gr}}}\) are depicted in Fig. 1a by black arrows. This process is initiated by refraction of the spin wave30 at the edge of the magnetisation gradient (orange line in Fig. 1a, modified wavelength but no change in the direction of \(\overrightarrow{k}\) for our simple example but important later on) and continues with smooth transformation of the spin-wave wavevector31 (highlighted with coloured arrows at certain positions). This scheme also holds for more general cases in which spin waves propagate under arbitrary angles \(\phi =\angle (\overrightarrow{k},\,{\overrightarrow{H}}_{{\rm{ext}}})\) with respect to the biasing magnetic field \({\overrightarrow{H}}_{{\rm{ext}}}\).
Scheme of the mode conversion and dispersion relations for different local values of the saturation magnetisation. (a) The mode conversion process transforms BVMSW (blue) to MSSW (red) modes. Both types of spin waves propagate in the sketched areas at different values of the saturation magnetisation MS. A magnetisation landscape – a temperature-induced arbitrary shaped magnetisation gradient \(\overrightarrow{\nabla }{M}_{{\rm{S}}}(x,y)\) – enables the mode conversion. A two–step process changes the direction of the wavevector \(\overrightarrow{k}\) and the group velocity \({\overrightarrow{v}}_{{\rm{gr}}}\). First, the spin-wave is refracted at the interface to the gradient decreasing the wavenumber. Next, a smooth transformation inside the magnetisation landscape occurs. The external magnetic field \({\overrightarrow{H}}_{{\rm{ext}}}\) is oriented parallel to the waveguide (in-plane orientation). (b) The dispersion relations of MSSW (propagating in the y direction) and BVMSW modes (propagating in the x direction) are shown versus the wavevector k. Due to the saturation magnetisation gradient, a wide band for both modes is realised. The intersection of these bands forms the conversion area (grey), where the mode conversion is allowed. The values MS,1 and MS,2 correspond to the temperatures T1 at antenna 1 and T2 at antenna 2 and are chosen according to the infrared camera measurements in Fig. 2. The conversion area is limited by the ferromagnetic resonance frequencies (FMR) for MS,1 and MS,2, respectively. A temperature-induced change in the minimal and maximal value of the saturation magnetisation leads to a shift of both frequencies and consequently of the conversion area. Thus, a similar behaviour of the conversion process is expected for different ranges of the saturation magnetisation. The maximal wavenumber of 500 rad/cm corresponds to the spin wave of the wavelength 125 µm. (c) The experimental set-up is shown schematically. A light pattern which creates a magnetisation gradient is formed close to the centre in x-direction of the waveguide at antenna 2 (illumination area). With respect to the externally applied magnetic field \({\overrightarrow{H}}_{{\rm{ext}}}\), antennas 1 and 3 can excite and detect BVMSWs. Antenna 2 is used for the detection of MSSWs. Thus, spin waves excited at MS,1 are converted and afterwards measured at MS,2.
Transmission characteristics for different light intensity distributions and the corresponding local saturation magnetisation. A rectangular and triangular graded intensity distribution is applied in the centre between antennas 1 and 3 – at the position of antenna 2 (“illumination area” in Fig. 1c) – and the corresponding spin-wave transmission S31 (a) and S21 (b) is measured (rectangle: blue line, triangle: red line) and compared with the reference measurement without heating (black line). The light-induced temperature distributions are shown as colour code (bottom right corner of Fig. 2, blue: cold, red: hot). The S31 (S21) parameter depicts the transmission of spin waves form antenna 1 to 3 (1 to 2). The grey area (7.10–7.15 GHz) is above the ferromagnetic resonance frequency for an unheated sample (see the S31 parameter) and is not of interest in this work. The strongest conversion (up to −22 dB) is achieved by a triangular light pattern with an intensity gradient. The small frequency shift between the triangle’s and the rectangle’s spectrum in Fig. 2b of (11 ± 1) MHz, exemplarily measured at a −40 dB level, is due to an incomplete match to the temperatures near antenna 2. (c) The profiles of the saturation magnetisation along the y coordinate (over the waveguide width) at the centre of the illumination area (grey dotted line) are shown for the different light distributions. Gradients in the saturation magnetisation with a negative slope cause the mode conversion. The slope of the linear fit (from 0.0 to 2.75 mm) for the triangle with an intensity gradient is −3.58 kA/m per mm.
We realise the different saturation magnetisations for an experimental demonstration of this mode-conversion process by laser-induced local heating recently introduced by us32. This method allows for generating almost arbitrary saturation magnetisation landscapes (see methods) to influence the spin-wave propagation. For the simple example discussed above (Fig. 1a) the BVMSW is launched in a cold region (blue) and converted into the MSSW in a hot region (red). For launching and detecting the respective spin waves, we use the microwave antenna configuration depicted in Fig. 1c.
Experimental studies of mode conversion in magnetisation gradients
We perform our experiments on a 6.6 µm thick ferrimagnetic Yttrium Iron Garnet (YIG) film acting as spin-wave waveguide. The dependency of its saturation magnetisation MS on the local temperature T(x,y) is well known: MS(T) decreases for increasing T 31,32,33,34 (see equation 3 in the methods part). To heat the magnon waveguide, arbitrary intensity distributions are realised via computer-generated holograms. Green laser light from a continuous-wave laser (λ = 532 nm) illuminates a phase-only spatial light modulator on which the computer-generated holograms are displayed, resulting in a controlled intensity distribution on the spin-wave waveguide. As the optical set-up is similar to the one presented in32, we refer to the methods part and supplementary materials for further details. The YIG film absorbs approximately 55% of the green light. Remaining light impinges onto a black absorber layer which further increases the thermal contrast inside the spin-wave waveguide32. Since YIG/GGG have relatively high thermal conductivities, it is assumed that a possible out-of-plane thermal gradient is small and does not influence the phenomena under investigation. An infrared camera records the resulting temperature distribution over the sample. The corresponding local saturation magnetisation is calculated using the given spatial temperature distribution (see methods). To excite and detect spin waves, a vector network analyser is connected to the three-microstrip-antenna configuration shown in Fig. 1c to determine the scattering parameters S21 and S31.
Let us discuss now the experimental results for the exemplary cases shown in Fig. 2. Figure 2a depicts transmission spectra for spin-wave transmission from antenna 1 to 3 (parameter S31). Without any intensity distribution, S31 follows a typical BVMSW transmission spectrum35 with a maximum of about −24 dB. This value is defined by the excitation/detection efficiency of the antennas and by the damping of spin waves propagating over 10 mm distance. The grey shaded area (7.10–7.15 GHz) marks the spectral region above the ferromagnetic resonance frequency for an unheated sample. In this frequency range, only higher width-modes (pronounced peak) can be excited by antenna 1. However, spin-wave modes with frequencies above 7.1 GHz are not of interest in this work. The picture dramatically changes, if we shine either one of the two exemplary intensity distributions onto the sample: for a rectangular (solid blue curve) as well as for a triangular distribution (solid red curve) the transmission from antenna 1 to antenna 3 vanishes completely. In Fig. 2b, we observe the opposite behaviour. Transmission from antenna 1 to 2 (parameter S21) for the reference measurement (black solid line) shows practically no signal: The constant background around −50 dB over a wide frequency range up to 7.1 GHz is due to the electromagnetic leakage between the antennas. The absence of the S21 transmission shows that no excitation of waves with \(\overrightarrow{k}\perp {\overrightarrow{H}}_{{\rm{ext}}}\) occurred and, consequently, no BVMSW-to-MSSW mode conversion is possible, as expected. For the rectangular (blue solid line) and triangular (red solid lines) pattern, a strong transmission signal is observed, comparable in strength to the one measured for the BVMSW in the reference measurement (Fig. 2a). This behaviour is consistent with mode-conversion from BVMSW to MSSW modes. To explain this further let us discuss the resulting saturation magnetisation gradient in more detail. Both light patterns are intensity gradients reaching from the maximum intensity (upper waveguide edge at antenna 2) down to 20% of this value (lower waveguide edge, see bottom part of Fig. 2c). Due to the intrinsic thermal conductivity of YIG a temperature gradient \(\overrightarrow{\nabla }T(x,y)\) and, thus, a magnetisation gradient \(\overrightarrow{\nabla }{M}_{{\rm{S}}}(x,y)\) evolves inside the sample (the gradient in z direction is neglected). We keep the saturation magnetisation MS,2(T) at antenna 2 approximately constant by adjusting the laser power appropriately. This is done to allow a quantitative comparison of the two cases. Antenna 1 and antenna 3 are kept far away from the heated area, thus MS,1 and MS,3 are almost equal and correspond approximately to the value at room temperature. By non-uniformly heating the sample, the S21-parameter maximum increases up to −23 dB for the rectangular pattern and −22 dB for the triangular pattern. Compared to the maximum of the BVMSW spectrum S31 (−24 dB), the mode conversion in magnetisation gradients is very effective. Furthermore, the bandwidth of the converted spectrum at −30 dB increases from 38 MHz in the rectangular case to 84 MHz in the triangular case. In contrast to the unheated sample, now the S31 parameter shows practically no transmission since the spin waves are converted and partially reflected in the heated region. The temperature profiles – recorded using an infrared camera – are used to calculate the local saturation magnetisation in dependency of the y coordinate (Fig. 2c) at the centre of the illumination area (grey dotted lines). To allow for an effective mode conversion the MS gradient needs a negative slope (away from antenna 2) and has to reach all over the waveguide width. This is consistent with the band structure model described above: MSSW with the same frequency as BVMSW can propagate only in a region of YIG with decreased MS. So, the crucial requirement for the conversion is that antenna 2 should detect spin waves from a part of the waveguide at lower saturation magnetisation compared to antenna 1. Moreover, smooth and linear magnetisation transitions are needed to avoid reflections34. The experimental temperature distribution is the solution of the heat equation regarding given boundary conditions. The lower (y = 0.0 mm) and upper (y = 3.0 mm) edges of the waveguide are thermally isolated (von Neumann boundary conditions). Here, the temperature drops down and, thus, the saturation magnetisation increases. Moreover, antenna 2 acts as a heat sink (Dirichlet boundary condition).
Conversion area and efficiency maximum
To experimentally check the limits of the conversion area shown in Fig. 1b, the sample is illuminated with the triangular light distribution with an intensity gradient (see Fig. 2). With increasing laser power, the spin-wave waveguide heats up. Accordingly, MS decreases at antenna 2 and, thus, the difference between the saturation magnetisations \({\rm{\Delta }}{M}_{{\rm{S}}}\) at antenna 1 and 2 increases. \({\rm{\Delta }}{M}_{{\rm{S}}}\) depicts qualitatively the gradient of the saturation magnetisation. Figure 3a and b show the corresponding transmission spectra as a colour map for the S21 and S31 parameters, respectively. Stronger heating and, therefore, reduction of MS(T) leads to a wider frequency bandwidth of the conversion area, as expected from our reasoning above (the conversion area in Fig. 1b depends on the difference in the saturation magnetisations MS,1 and MS,2). Spin waves cannot propagate to antenna 3 by increasing the MS gradient at antenna 2 (Fig. 3b).
Experimental magnetisation dependence of the conversion area and the conversion efficiency’s maximum. The frequency f is shown versus the difference between the saturation magnetisations \(\Delta {M}_{{\rm{S}}}={M}_{{\rm{S}},1}-{M}_{{\rm{S}},2}\) at antenna 1 and 2 for the S21 (a) and S31 (b) parameters, respectively. The spin-wave transmission is colour coded (red: strong transmission, blue: no transmission). White squares (triangles) are the experimentally determined FMR frequencies using the reflection parameters S11 (S22) at antenna 1 (antenna 2). The width of the conversion area increases with decreasing saturation magnetisation (a) and the FMR frequency at antenna 2 shifts to lower frequencies. On the other hand, spin waves with a frequency larger than this FMR frequency cannot travel through the magnetisation landscape to reach antenna 3 (b). (c) The normalised efficiency of the mode conversion (black line) of BVMSWs to MSSWs is shown versus the frequency f. Here, the saturation magnetisation difference \({\rm{\Delta }}{M}_{{\rm{S}}}\) is about 16 kA⁄m. The position of the maximum is determined via fitting the experimental data with an empirical bi-Gaussian function (grey dotted line). (d) The frequency of the numerical conversion efficiency peak (red squares) is plotted versus \({\rm{\Delta }}{M}_{{\rm{S}}}\). Black circles indicate the theoretically expected position of the crossing points of the BVMSW (at antenna 1) and MSSW (at antenna 2) dispersion relations for the corresponding saturation magnetisations. The error bars result from the peak fitting error of the experimental data and the error in the external magnetic field (\({\mu }_{0}{\rm{\Delta }}{H}_{{\rm{ext}}}=\pm \,1\,{\rm{mT}}\)), respectively.
Furthermore, we calculate the conversion efficiency qualitatively (Fig. 3c) using the S21 parameter, the frequency range of the antenna excitation and detection, and the propagation parameters of the spin waves (see methods). The corresponding frequency of the efficiency maximum is determined via numerical peak fitting. The conversion takes place in between the ferromagnetic resonance frequencies at antenna 1 and 2 (FMR1 and FMR2). The frequency position of the efficiency maxima can be compared to the dispersion relations of the BVMSW and MSSW modes (Fig. 3d). The strongest conversion is observed at the crossing point of the dispersion relation of the MSSW mode at antenna 2 and the dispersion relation of the BVMSW at antenna 1 (see Fig. 1b). For this particular frequency, the magnitude of the momentum is conserved.
Isofrequency curves and micromagnetic simulations
In order to obtain deeper insight into the conversion mechanism, we perform numerical micromagnetic calculations for magnetisation variations reaching from MS,1 = 141kA/m (at room temperature) to MS,2 = 123 kA/m (T2 ≈ 353K, largest temperature investigated in the experiments, for details of the calculations see the methods section). If the spin waves are excited at f = 7.0 GHz the mode conversion is energetically allowed (see dispersion relations in the supplementary materials). As a reference, additional simulations for 6.8 GHz are shown in the supplementary materials (pure BVMSW behaviour). The propagation of spin waves in gradients of the saturation magnetisation is visualised in Fig. 4 and can be understood via the according isofrequency curves36,37. In general, mode conversion can occur if the angle between wavevector and external magnetic field \(\phi =\angle (\overrightarrow{k},\,{\overrightarrow{H}}_{{\rm{ext}}})\) is larger than a critical angle φc38. MSSWs are described by isofrequency curves, which lie completely above the line corresponding to φc (\({\phi }_{{\rm{c}}}\approx 46^\circ \), see supplementary materials). Curves below or even crossing this limit are BVMSW modes propagating under the angle φ. We identify the two mechanisms changing the direction of the wave propagation: first, refraction at the interface or at the shape of the magnetisation gradient30 and, second, the gradient \(\overrightarrow{\nabla }{M}_{{\rm{S}}}(x,y)\) itself31. For a rectangular magnetisation landscape (Fig. 4a), the waveguide is divided into two regions: one with saturation magnetisation below MS,FMR and one with a value above. As example, we choose MS,FMR (green dotted line) as the value of the saturation magnetisation at which the FMR frequency equals 7.0 GHz (excitation frequency in the micromagnetic simulations). In this case, the propagating spin waves (black dotted line) at MS ≈ 141 kA/m enter the gradient at a certain value MS,in defined by the coordinate in the direction perpendicular to the propagation direction. The spin waves cannot enter the gradient region where MS,in < MS,FMR (see region above green dotted line) and, thus, they are reflected. For MS,in ≥ MS,FMR the wave propagates into the magnetisation gradient and only its wavelength is modified (no change in the direction of \(\overrightarrow{k}\)). Afterwards, mode conversion takes place: the spin-wave wavevector and, respectively, its wavelength change differently in different positions over the waveguide’s width resulting in a bending of the phase fronts and in an adjustment of the group velocity’s direction. The spin waves propagate into regions with decreased MS. The corresponding isofrequency curves illustrate how the conversion process is happening: the spin waves at MS ≈ 141 kA/m propagate into the gradient region at MS,in. The tangential component of \(\overrightarrow{k}\) (with respect to the interface) is conserved and only kx changes. After entering \(\overrightarrow{\nabla }{M}_{{\rm{S}}}(x,y)\), kx is conserved and only ky is modified since the translational symmetry of the system is broken due to the magnetisation gradient in the y direction. The change Δky is due to refraction of the spin waves in the gradient area, whose extend is much larger than the wavelength31. Eventually, \(\overrightarrow{k}\) rotates in the x-y-plane.
Micromagnetic calculations (left) and corresponding isofrequency curves (right). The numerical calculations are performed for f = 7.0 GHz. Two gradient shapes are analysed: a rectangle (a) and a triangle (b). Figure 4a is shown schematically in Fig. 1a. The isofrequency curves illustrate how the wavevector \(\overrightarrow{k}\) is transformed while the spin wave propagates (dotted line) into and through the magnetisation gradient region. The spin wave enters the gradient at a certain local value of the saturation magnetisation MS,in. The isofrequency curves visualise the 2-step conversion process: first, the spin waves are refracted at the interface. Next, the transformation inside the gradient takes place. The direction of \(\overrightarrow{k}\) can be directly compared to the micromagnetic simulations. Here, the spin wave is excited at the left of each picture and propagates to the right into the magnetisation gradient region (grey rectangle or triangle) where the conversion can take place. The dynamic magnetisation mz is colour coded (red: max, blue: min).
A similar behaviour is observed in the triangular case (Fig. 4b) for \({M}_{{\rm{S}},{\rm{in}}}\ge {M}_{{\rm{S}},{\rm{FMR}}}\). The spin wave is converted smoothly while propagating towards the region of lower saturation magnetisation. For the triangle, additional refraction30 due to the shape of the \(\overrightarrow{\nabla }{M}_{{\rm{S}}}(x,y)\) area occurs. The wave propagates towards the interface under an angle of 45° and, thus, kx and ky are modified. Next, \(\overrightarrow{\nabla }{M}_{{\rm{S}}}(x,y)\) leads to an increase in ky. The triangular case (Fig. 4b) for f = 7.0 GHz shows minor reflections at values of the magnetisation in the range of \({M}_{{\rm{S}},{\rm{FMR}}}\le {M}_{{\rm{S}}}\le 124\,{\rm{kA}}/{\rm{m}}\). In the \(\overrightarrow{k}\)-space, the black dotted line corresponding to the refraction now crosses the MSSW isofrequency curves (above the grey dotted line associated to φc) and a direct conversion from BVMSWs to MSSWs due to the geometrical shape is possible. The gradient of MS is used afterwards to tune the propagation direction of MSSWs to the demanded angle that is perpendicular to the spin-wave waveguide. Thus, the efficiency of the mode conversion is improved. This is in direct agreement with our experimental results. All the simulations show reflections at the top of the waveguide due to the change in the y-component of the group velocity. For more clarity, further calculations (no upper waveguide edge) have been performed and can be found in the supplementary materials. Also, additional movies are presented in the supplementary materials, showing the excitation of BVMSWs for different frequencies and the spin-wave propagation into and through the rectangular and triangular magnetisation landscape, respectively.
Conclusion
We proposed and demonstrated experimentally as well as numerically mode conversion between backward volume and magnetostatic surface spin-wave modes using gradients of the saturation magnetisation MS. The magnetisation landscapes are achieved via reconfigurable laser-induced thermal patterns. The gradients perpendicular to the spin-wave propagation direction allow for the 90 degree rotation of the spin-wave wavevector via breaking the translational symmetry of the waveguide. As opposite to the spin-wave momentum, the energy of spin waves is conserved in this process due to the intersection of frequency bands of both spin-wave modes allowed in a non-uniform media. Since the proposed conversion is a smooth process (the characteristic length scales of the gradients are much larger than the spin-wave wavelength) it allows for the minimisation of undesirable reflections and ensures high efficiency of the conversion in a wide range of spin-wave frequencies. Moreover, it is shown that the conversion mechanism can be further enhanced via the exploiting of refraction at the edge of the magnetisation gradient. Thus, the mode conversion using a triangle-shaped area of the magnetisation gradient has shown even higher efficiency in a wider frequency range. The concept of spin-wave guiding in in-plane magnetised films using magnetisation gradients can be extended to the nanoscale (using e.g. ionic implantation39,40,41) and is promising for the realisation of magnonic networks for novel computing concepts. Moreover, the proposed approach of gradually changing the material’s properties is not only applicable for the guiding of spin waves in anisotropic media but can be also extended to waves in strongly-anisotropic media in general.
Methods
Sample
The W = 3 mm wide sample consists of a multilayer structure. The substrate is a 500 µm thick Gadolinium Gallium Garnet (GGG, paramagnetic) layer. On top, a 6.6 µm thin Yttrium Iron Garnet (YIG, ferrimagnetic) film was deposited via liquid phase epitaxy. A several 10 µm thick black lacquer is used as additional absorber of the light intensity to increase the thermal contrast of the temperature profile – due to an approximately one order of magnitude smaller thermal conductivity compared to YIG32. Furthermore, the lacquer is used as glue. The dielectric spacer is an adhesive tape with a thickness of about 50 µm. This spacer separates the antennas – otherwise they would act as a heat sink – thermally from the sample. GGG is close-to-transparent for green light (532 nm wavelength). Most of the absorption takes place in the YIG film (approx. 55%). Remaining light impinges onto the black absorber. A scheme of the multilayer system is shown in the supplementary materials.
Experimental setup
The experimental setup is divided into two parts – optics and microwave technology. The illumination of the sample is realised using a green continuous wave laser (Coherent Verdi G7, 532 nm, maximal power of 7.4 W). The beam is expanded/confined via a combination of a Galilean and a Keplerian telescope. Finally, a Fourier lens reconstructs the hologram on the sample. About thirty-two percent (32%) of the input laser power is available for the heating process (Pholo = 0.32 Plaser). The intensity distributions on the sample are achieved by a spatial light modulator (Hamamatsu X10468-01; phase only modulation). Multi foci42 are used to realise the applied rectangular and triangular intensity distributions. The intensity gradients used in the experiment range from the highest intensity down to 20% of this value. The resulting temperature distributions are recorded using an infrared camera (FLIR SC-655). A scheme of the optical set-up can be found in the supplementary materials.
The microwave excitation and detection is performed by a vector network analyser (Anritsu MS4642B). To avoid nonlinearities, the microwave power for the spin-wave excitation was set to −5 dBm. The copper antennas are about 18 µm thick and 50 µm wide along the excitation/detection area of 3 mm (fabricated on a Duroid ceramic substrate). The distance L between antenna 1 and 3 is 10 mm. The centre of antenna 2 is located in between (5 mm distance to the other antennas). The fabrication uses standard lithography and etching processes. The external magnetic field Hext is created via an electromagnet (Lakeshore EM4-HVA, Hext,max ≈ 400 mT @ 110 mm pole distance).
Dispersion relations
To discuss the spin-wave mode conversion analytically, we use the respective dispersion relations for the BVMSW and MSSW modes:
where ωH = γμ0Hext, ωM(T) = γμ0MS(T), and d = 6.6 μm is the YIG film thickness. Here, γ = 2π⋅28.0 GHz/T is the electron gyromagnetic ratio and μ0 = 4π⋅10−7N/A2 the permeability in vacuum. μ0Hext = (180 ± 1) mT is the external applied magnetic field (Hall probe measurement). The local modification of the saturation magnetisation (MS,298K ≈ 140 kA/m at room temperature) is included via the temperature distribution T(x,y)32:
The temperature – and therefore MS – is approximately uniform over the film thickness. The native crossing point of both dispersion relations for the same MS and Hext at kx = ky = 0 rad/cm is called the ferromagnetic resonance (FMR) frequency:
Conversion efficiency
To determine the frequency of the conversion efficiency’s maximum of spin waves propagating in magnetisation gradients we choose the following simplified approach (all the given parameters are frequency dependent). S21 describes the normalised power which is transmitted between the antennas 1 and 2 (0 ≤ S21 ≤ 1). A spin wave propagating between these antennas is damped (parameter β) and converted (parameter γ):
Here, δantenna describes the excitation/detection efficiency of the regarded antenna. L is the distance from antenna 1 to antenna 3 and W is the waveguide width. Neglecting the microwave absorption of the microstrip antennas, the excitation and detection frequency range at antenna 1 and 2 is given by
and
The propagation or damping parameter β is determined via (x is the propagation distance):
The propagation length lprop can be calculated using the group velocity \({v}_{{\rm{gr}}}=2\pi \frac{\partial f}{\partial k}\) and the lifetime τ of the particular spin-wave mode:
Here,
α = 0.5⋅10−4 is the Gilbert damping constant, ω the angular frequency, and \({P}_{{\rm{A}}}=\sqrt{1+{(\frac{\gamma {\mu }_{0}{M}_{{\rm{S}}}(T)}{4\pi f})}^{2}}\) the ellipticity correction factor43. Finally, the conversion spectrum γ is given by equation (5):
Isofrequency curves
The isofrequency curves shown in figure 4 are calculated numerically using the equation37:
The parameters μ and ν are given by:
Equation (7) connects the two components kx and ky with each other. Furthermore, the cotangent was substituted with a Laurent series:
We took the first ten terms of the series to approximate the fundamental thickness mode. The B2n are the Bernoulli numbers.
Simulation
The micromagnetic simulations are performed using the open-source software mumax³ (v3.9.1)44. The influence of the temperature on the saturation magnetisation was implemented via equation (3). The respective dimensions in the x, y, and z directions are 10 mm × 3 mm × 6.6 µm (1024 × 512 × 1 cells). So, the thickness of the whole spin-wave waveguide is modelled by one cell. The achieved cell sizes are 9.8 µm × 5.9 µm × 6.6 µm. The excitation of the spin waves is done using the Oersted field of a microstrip antenna (50 µm width). The RF current was chosen to be 2 mA to avoid nonlinearities. The excitation signal was applied at the centre of the waveguide (at x = 5 mm). In Fig. 4, extracts of the propagating wave for x > 5 mm are shown (4 mm × 3 mm, 120 ns after the excitation, stationary regime). To avoid reflections at the edges of YIG (at x = 0 and 10 mm), a 1 mm wide absorbing region was used. Here, the Gilbert damping constant α smoothly and linearly increases from 10−4 to 10−1 .
Data availability
All data generated or analysed during this study are included in this published article (and its Supplementary Information files).
References
Chumak, A. V. et al. Magnon Spintronics. Nature Phys. 11, 453–461 (2015).
Kruglyak, V. V., Demokritov, S. O. & Grundler, D. Magnonics. J. Phys. D: Appl. Phys. 43, 264001 (2010).
Khitun, A., Bao, M. & Wang, K. L. Magnonic logic circuits. J. Phys. D: Appl. Phys. 43, 264005 (2010).
Lenk, B., Ulrichs, H., Garbs, F. & Münzenberg, M. The building blocks of magnonics. Phys. Rep. 507, 107136 (2011).
Nikonov, D. I. E. & Young, I. A. Benchmarking of beyond-CMOS exploratory devices for logic integrated circuit. IEEE J. Explor. Sol.-State Comput. Devices and Circ. 1, 3 (2015).
Krawczyk, M. & Grundler, D. Review and prospects of magnonic crystals and devices with reprogrammable band structure. J. Phys.-Cond. Matt. 26, 123202 (2014).
Chumak, A. V., Serga, A. A. & Hillebrands, B. Magnon transistor for all-magnon data processing. Nature Commun. 5, 4700 (2014).
Klingler, S. et al. Design of a spin-wave majority gate employing mode selection. Appl. Phys. Lett. 105, 152410 (2014).
Fischer, T. et al. Experimental prototype of a spin-wave majority gate. Appl. Phys. Lett. 110, 152401 (2017).
Vogt, K. et al. Realization of a spin-wave multiplexer. Nature Commun. 5, 3727 (2014).
L’vov, V. S. Wave Turbulence Under Parametric Excitation (Springer, 1994).
Pirro, P. et al. Non-Gilbert-damping Mechanism in a Ferromagnetic Heusler Compound Probed by Nonlinear Spin Dynamics. Phys. Rev. Lett. 113, 227601 (2014).
Sebastian, T. et al. Nonlinear Emission of Spin-Wave Caustics from an Edge Mode of a Microstructured Co2Mn0.6Fe0.4Si Waveguide. Phys. Rev. Lett. 110, 067201 (2013).
Gurevich, A. G. & Melkov, G. A. Magnetization Oscillations and Waves (CRC Press, 1996).
Jersch, J. et al. Mapping of localized spin-wave excitations by near-field Brillouin light scattering. Appl. Phys. Lett. 97, 152502 (2010).
Sebastian, T. et al. Micro-focused Brillouin light scattering: imaging spin waves at the nanoscale. Front. Phys. 3, 35 (2015).
Tanaka, Y. et al. Dynamic control of the Q factor in a photonic crystal nanocavity. Nature Mater. 6(11), 862–865 (2007).
Chumak, A. V. et al. All-linear time reversal by a dynamic artificial crystal. Nature Commun. 1, 141 (2010).
Vlaminck, V. & Bailleul, M. Current-Induced Spin-Wave Doppler Shift. Science 322(5900), 410–413 (2008).
Stancil, D. D. et al. Observation of an inverse Doppler shift from left-handed dipolar spin waves. Phys. Rev. B 74, 060404 (2006).
Chumak, A. V. et al. Reverse Doppler effect of magnons with negative group velocity scattered from a moving Bragg grating. Phys. Rev. B 81, 140404 (2010).
Vogt, K. et al. Spin waves turning a corner. Appl. Phys. Lett. 101, 042410 (2012).
Davies, C. S. et al. Towards graded-index magnonics: steering spin waves in magnonic networks. Phys. Rev. B 92, 020408 (2015).
Davies, C. S. & Kruglyak, V. V. Graded-index magnonics. Low Temperature Physics 41, 760–766 (2015).
Sadovnikov, A. V. et al. Spin wave propagation in a uniformly biased curved magnonic waveguide. Phys. Rev. B 96, 060401(R) (2017).
Tkachenko, V. S., Kuchko, A. N., Dvornik, M. & Kruglyak, V. V. Propagation and scattering of spin waves in curved magnonic waveguides. Appl. Phys. Lett. 101, 152402 (2012).
Gruszecki, P. & Krawczyk, M. Spin wave beam propagation through media with the graded refractive index: Mirage effect and prospective applications. Phys. Rev. B 97, 094424 (2018).
Sadovnikov, A. V. et al. Magnonic beam splitter: the building block of parallel magnonic circuitry. Appl. Phys. Lett. 106, 192406 (2015).
Brächer, T. et al. Generation of propagating backward volume spin waves by phase-sensitive mode conversion in two-dimensional microstructures. Appl. Phys. Lett. 102, 132411 (2013).
Stigloher, J. et al. Snell’s Law for Spin Waves. Phys. Rev. Lett. 117, 037204 (2016).
Dzyapko, O. et al. Reconfigurable heat-induced spin wave lenses. Appl. Phys. Lett. 109, 232407 (2016).
Vogel, M. et al. Optically reconfigurable magnetic materials. Nature Phys. 11, 487–491 (2015).
Stancil, D. D. & Prabhakar, A. Spin waves: theory and applications (Springer, 2009).
Obry, B. et al. Spin-wave propagation and transformation in a thermal gradient. Appl. Phys. Lett. 101, 192406 (2012).
Serga, A. A., Chumak, A. V. & Hillebrands, B. YIG magnonics. J. Phys. D: Appl. Phys. 43, 264002 (2010).
Lock, E. H. The properties of isofrequency dependences and the laws of geometrical optics. Phys. Usp. 51, 375–393 (2008).
Vashkovsky, A. V. & Lock, E. H. Properties of backward electromagnetic waves and negative reflection in ferrite films. Phys. Usp. 49, 389–399 (2006).
Hurben, M. J. & Patton, C. E. Theory of magnetostatic waves for in-plane magnetized isotropic films. J. Magn. Magn. Mater. 139, 263–291 (1995).
Carter, R. L. et al. lon-implanted magnetostatic wave reflective array filters. J. Appl. Phys. 53, 2655 (1982).
Obry, B. et al. A micro-structured ion-implanted magnonic crystal. Appl. Phys. Lett. 102, 202403 (2013).
Algra, H. A. & Hansen, P. Temperature Dependence of the Saturation Magnetization of Ion-Implanted YIG Films. Appl. Phys. A 29, 83–86 (1982).
Waller, E. H. & von Freymann, G. Multi foci with diffraction limited resolution. Opt. Express 21, 21708–21713 (2013).
Kalarickal, S. S. et al. Ferromagnetic resonance linewidth in metallic thin films: Comparison of measurement methods. J. Appl. Phys. 99, 093909 (2006).
Vansteenkiste, A. et al. The design and verification of mumax3. AIP Advances 4, 107133 (2014).
Acknowledgements
Financial support by the DFG transregional collaborative research center (SFB/TRR) 173 “Spin + X – Spin in its collective environment” (project B04) is gratefully acknowledged.
Author information
Authors and Affiliations
Contributions
M.V. and G.v.F. devised and planned the project. R.A. started the first experiments during his bachelor thesis. M.V. realised the experimental setup, performed the final measurements, analysed the data, performed the simulations, and wrote a first draft of the manuscript. P.P. supported the simulations. A.V.C., B.H. and G.v.F. led the project. All authors discussed the results and contributed to writing the manuscript.
Corresponding author
Ethics declarations
Competing Interests
The authors declare no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Vogel, M., Aßmann, R., Pirro, P. et al. Control of Spin-Wave Propagation using Magnetisation Gradients. Sci Rep 8, 11099 (2018). https://doi.org/10.1038/s41598-018-29191-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-018-29191-2
This article is cited by
-
Reconfigurable magnon interference by on-chip dynamic wavelength conversion
Scientific Reports (2023)
-
Sensitivity enhancement in magnetic sensor using CoFeB/Y3Fe5O12 resonator
Scientific Reports (2022)
-
Advances in coherent magnonics
Nature Reviews Materials (2021)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.