Abstract
High-speed magnetization control of ferromagnetic films using light pulses is attracting considerable attention and is increasingly important for the development of spintronic devices. Irradiation with a nearly monocyclic terahertz pulse, which can induce strong electromagnetic fields in ferromagnetic films within an extremely short time of less than ~1 ps, is promising for damping-free high-speed coherent control of the magnetization. Here, we successfully observe a terahertz response in a ferromagnetic-semiconductor thin film. In addition, we find that a similar terahertz response is observed even in a non-magnetic semiconductor and reveal that the electric-field component of the terahertz pulse plays a crucial role in the magnetization response through the spin-carrier interactions in a ferromagnetic-semiconductor thin film. Our findings will provide new guidelines for designing materials suitable for ultrafast magnetization reversal.
Similar content being viewed by others
Introduction
In ferromagnetic materials, typically more than a few hundred picoseconds are necessary to reverse the magnetization when using spin-transfer torque or light irradiation1,2,3,4,5,6,7,8,9,10,11,12,13, limiting the operational speed of magnetic memory devices. Meanwhile, using a terahertz light pulse, a strong electromagnetic field can be induced within an extremely short time of less than ~1 picosecond in ferromagnetic thin films, in which the spin-lattice relaxation is too slow to follow the electromagnetic fields11. Thus, terahertz pulse control of the magnetization is promising for ultrafast magnetization reversal within a few picoseconds. In the previous studies on terahertz pump-probe measurements, a tiny modulation in the magnetization by a terahertz pulse was demonstrated for ferromagnetic-metal thin films such as Co, Ni, Fe and CoFeB11,12,13. Until now, the origin of this phenomenon has been attributed to the Landau-Lifschitz-Gilbert (LLG) torque11,12,13, which is induced by the magnetic-field component of the terahertz pulse, and to the demagnetization caused by the heating11,13.
Recently, static-electric-field control of the magnetization vector14,15,16, Curie temperature and coercivity17,18 has been reported for magnetic metal thin films; however, thus far, the electric-field component of the terahertz pulse has not often been associated with the magnetization modulation13. In non-magnetic materials, optical properties are known to be influenced by the modulation of the spatial carrier distribution induced by the electric field of light19,20,21, which is called the Franz-Keldysh effect (FKE). The FKE has been investigated mainly for semiconductors rather than metals because semiconductors have a low carrier density and are sensitive to electric fields. GaAs is a suitable semiconductor for our study because it becomes ferromagnetic when doped with Mn and because Mn-doped GaAs (GaMnAs) is fairly sensitive to an external optical stimulus5,22. Comparing GaAs samples doped with non-magnetic atoms and with Mn atoms can demonstrate how the electric field of the terahertz pulse influences the magnetization in ferromagnetic GaMnAs.
Results
Samples and experimental setup
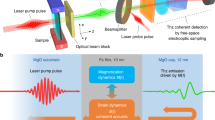
In our terahertz pump-probe measurements, we used thin films so that the terahertz pump beam can penetrate them. We use a non-magnetic semiconductor Be-doped GaAs thin film (referred to as GaAs:Be, Be acceptor concentration: 1019 cm−3, thickness: 40 nm) and a ferromagnetic semiconductor Ga0.94Mn0.06As thin film (thickness: 20 nm) with a perpendicular easy magnetization axis (see Methods). We utilize strong terahertz-pump pulses (~400 kV/cm) with the centered frequency of 1 THz, whose electric field ETHz is aligned along the [110] axis in the film plane (Fig. 1a). We detect the change Δθ in the polarization rotation of the reflected probe pulse with a delay time t relative to the terahertz-pump pulse. All measurements are carried out at 10 K (see Methods).
Overview of the experiment and the observation of Δθ induced by the Franz-Keldysh effect. (a) Schematic illustration of the experimental setup. The terahertz pump pulse (yellow) is focused on the GaAs:Be or GaMnAs sample surface, and the following probe pulse with a delay time t detects the excited dynamics. Both the pump and probe pulses are linearly polarized. The strong terahertz-pump pulse with a centred frequency of 1 THz, whose electric field ETHz is along the [110] axis, is generated by optical rectification using a LiNbO3 crystal. The incident angle of the probe pulse is tilted by 10° from the sample normal towards the in-plane [110] axis. The magnetization (pale blue arrow) is tilted from the sample normal by the terahertz pump pulse. The angle of the probe polarization plane from the [110] axis towards the \([1\bar{1}0]\,\) axis is defined as α. (b) Time evolution of Δθ (red circles) measured at 10 K without an external magnetic field for GaAs:Be when applying ETHz expressed by the green dotted curve. Δθ is normalized by its maximum value. The blue circles express Δθ when the intensity ETHz2 of the terahertz pump pulse is 25% of the green dotted curve (i.e., the maximum ETHz is 200 kV/cm). α is set to 30°. (c,d) Maximum value of the time evolution of |Δθ| obtained for GaAs:Be (c) and GaMnAs (d) plotted as a function of the maximum value of ETHz2 at 10 K. These measurements are carried out without an external magnetic field when α is set to 30°.
Observation of Δθ induced by the FKE
Here, we show that the strong FKE is induced by the terahertz pump pulses both in GaAs:Be and GaMnAs. The FKE induces magnetization modulation and birefringence. In the following discussion, the subscript “max” refers to the maximum value of the transient. In GaAs:Be, evidence of the FKE can be seen in the (ETHz2)max dependence of (−ΔR/R)max and in the time evolution of −ΔR/R (Supplementary Information Fig. S1); (−ΔR/R)max increases and saturates with increasing (ETHz2)max and the peak positions of −ΔR/R depend on (ETHz2)max (see Supplementary Information Sec. A). These are distinctive features of the FKE23. Owing to the polarization of the terahertz pulse along [110], the FKE induces the anisotropy of the reflectivities between [110] and \([1\bar{1}0]\,\) polarized light beams24. This anisotropy leads to the birefringence and thus the polarization rotation. These effects are actually observed in non-magnetic GaAs:Be, as shown in Fig. 1b, where the angle α between the electric field vector Eprobe of the probe and ETHz (//[110]) is set to 30° (Fig. 1a). As shown in Fig. 1c, |Δθ|max tends to saturate as (ETHz2)max increases, confirming that the observed Δθ is induced by the FKE23. For GaMnAs, we see that similar saturating behaviour appears in the (ETHz2)max dependence of |Δθ|max (Fig. 1d). Here, α is also set to 30°. This result indicates that Δθ is mainly attributed to the birefringence due to the FKE. The Δθ value observed for GaMnAs is smaller than that for GaAs:Be because the carrier density of GaMnAs is larger than that of GaAs:Be. Note that the small drop of |Δθ|max at high electric fields is due to the shift of the absorption edge by the FKE25. Because the signal induced by the birefringence is not directly related to the magnetization dynamics, this component should be removed.
Coherent magnetization modulation by E THZ
By setting Eprobe//ETHz(//[110]) (i.e., α = 0°), the effect of the birefringence can be minimized, and the magnetic signal becomes dominant (Supplementary Information Sec. B). In fact, the sign of Δθ is almost perfectly inverted when the magnetic field (=30 mT), which is applied perpendicular to the sample surface to align the magnetization, is reversed (orange and blue circles in Fig. 2). This means that the observed signal in Fig. 2 is purely a magnetic signal and is almost proportional to the change ΔMperp in the perpendicular magnetization Mperp, i.e., Δθ is mainly attributed to the polarization rotation ΔθMOKE induced by the magneto-optical Kerr effect. Note that the terahertz modulation of the magnetization is not caused by the magnetic field of the terahertz pulse, because the observed Δθ is three orders of magnitude larger than that calculated by the LLG-torque model (Supplementary Information Sec. D); rather, it is explained by the electric field of the terahertz pulse, i.e., the FKE. As shown below, in addition to this component, Δθ incorporates a small polarization rotation Δθbir induced by the birefringence, which remains owing to the small deviation from the ideal alignment of Eprobe//ETHz, and a component of the magnetic linear dichroism (ΔθMLD), which is proportional to the square of the in-plane magnetization ΔMin26.
Terahertz response of the GaMnAs film. Blue and orange open circles represent Δθ measured at 10 K for the Ga0.94Mn0.06As thin film when Eprobe//ETHz (α = 0°) with the magnetic field of 30 mT is applied in the [001] and \([00\bar{1}]\) directions, respectively. |ETHz| is shown by the green solid curve.
To quantitatively understand the magnetization dynamics, we perform the analysis using the dielectric tensor27,
Here, x// \([1\bar{1}0]\,\), y//[110] and z//[001] (Fig. 1a). Using this tensor and the Onsager relations, we can express Δθbir, ΔθMOKE and ΔθMLD using ε xx , ε yy , ε xy and ε zz , as described in Supplementary Information Sec. B. ΔθMOKE, which is proportional to ΔMperp, is obtained by \(({\rm{\Delta }}{\theta }_{M//[001]}-{\rm{\Delta }}{\theta }_{M//[00\bar{1}]})/2\), where \({\rm{\Delta }}{\theta }_{M//[001]}\) and \({\rm{\Delta }}{\theta }_{M//[00\bar{1}]}\) denote Δθ measured with the magnetic field applied in the [001] and \([00\bar{1}]\) directions shown in Fig. 2, respectively. Because ΔθMOKE is influenced by not only the change in ε xy but also the change in ε yy (Supplementary Information Sec. B), we derive −Δε xy/ ε xy (Fig. 3a), which is purely proportional to ΔMperp divided by the initial perpendicular magnetization before the pump pulse irradiation (Supplementary Information Sec. B). Here, Δε xy is the change in ε xy . Figure 3a shows that Mperp is indeed modulated by up to 1% and that it coherently follows the terahertz electric field.
For coherent magnetization control, the magnetization must tilt following the electric field of the terahertz pulse. We therefore examine the in-plane magnetization response. We derive ΔθMLD, which is proportional to ΔMin2, using the relation ΔθMLD = Δθ − Δθbir − ΔθMOKE, where Δθbir is derived from experimental ΔR/R (Supplementary Information Sec. B). In Fig. 3b, the dynamics of ΔMin2 (purple plot) show a time evolution similar to that of the perpendicular magnetization dynamics (dark blue plot in Fig. 3a), i.e., ΔMin2 increases when Mperp decreases (or when −Δε xy /ε xy increases). The geometrical calculation demonstrates that −ΔMperp is proportional to ΔMin2 (Supplementary Information Sec. C). Therefore, our results indicate that the magnetization is indeed tilted by the terahertz pulse. These results clearly indicate that the magnetization coherently follows the ultrafast oscillation of the electric field of the terahertz pulse via the FKE.
Discussion
We now discuss the physical mechanism of the magnetization modulation by the terahertz pulse. In the FKE, the anisotropy of the refractive index is induced by the anisotropic modulation of the band structure; owing to the application of a terahertz electric field along [110], the band structure is spatially tilted along [110] (Fig. 4a). This enables optical transitions with energies smaller than the band gap for [110]-polarized light, thus modulating the reflectivity of the material. In GaMnAs, while ETHz is applied, not only the valence band (VB) but also the electrochemical potential (EP) is tilted because carriers cannot diffuse within a few picoseconds (Fig. 4b). However, electrons can move between the VB and the impurity band (IB) (Fig. 4b), leading to the modulation in the local carrier concentration in the IB. Because ferromagnetism is induced by the double-exchange interaction between the IB holes in GaMnAs, the modulation in the carrier density of the IB induces the modulation of the magnetization28,29 and its direction via spin-orbit interaction5 or the inducement of orbital angular momentum30.
Magnetization modulation via the modulation of the spatial carrier distribution induced by the electric field of light. (a) Modulation of the band structure by the FKE. The band structure is spatially tilted by ETHz, enabling optical transitions with an energy smaller than the band gap. The red curves represent the electron wave functions. (b) Spatial band diagram of GaMnAs while ETHz is applied. The green and red regions are filled by electrons and holes, respectively. The IB and the VB overlap. Owing to the electric field, electrons in the VB can move to the IB.
At first glance, the electric field of the terahertz pulse appears to be not related to the magnetization; however, our results strongly suggest that it plays a crucial role in the terahertz response of the magnetization via the FKE. This new mechanism, the magnetization modulation via the modulation of the spatial carrier distribution induced by the electric field of light, will provide guidelines for designing materials that are suitable for coherent control of the magnetization using terahertz pulses and will provide a new approach to the ultrafast magnetization reversal.
Methods
Samples
The GaMnAs sample consists of (from top to bottom) Ga0,94Mn0.06As (20 nm)/In0.2Al0.8As (500 nm)/GaAs (100 nm), which was grown on a semi-insulating GaAs (001) substrate via low-temperature molecular beam epitaxy. After growth, this sample was annealed at 180 °C for 68 h. The Curie temperature of the film is 125 K. The GaMnAs film has a coercivity of 15 mT at 10 K. The GaAs:Be sample consists of GaAs:Be (Be: 1019 cm−3, 40 nm)/In0.2Al0.8As (500 nm)/GaAs (100 nm) grown on a semi-insulating GaAs (001) substrate.
Terahertz-pump probe measurements
The terahertz-pump probe measurements were performed using a pulsed-light source with a repetition rate of 1 kHz. Both pump and probe pulses were linearly polarized. The strong terahertz-pump pulse with a centred frequency of 1 THz, whose electric field ETHz was aligned along [110] in the film plane (Fig. 1a), was generated by tilted-pulse-front optical rectification in a LiNbO3 crystal with a tilted-pump-pulse-front scheme31,32. The measurement of ETHz is described in detail in ref.33. The terahertz intensity was adjusted by rotating two wire-grid polarizers. The time duration of the probe pulse was 90 fs, and the wavelength was 800 nm. The delay time of the probe pulse relative to the pump pulse was controlled by changing the length of the optical path of the probe pulse. Δθ was detected by a balanced detection technique using a half-wave plate, a polarizing beam splitter, a pair of balanced silicon photodiodes and a boxcar integrator. We defined the time origin (t = 0 ps) of the terahertz pulse as the time when the terahertz electric field reaches a maximum. All measurements are carried out at 10 K.
References
Beaurepaire, E., Merle, J.-C., Daunois, A. & Bigot, J.-Y. Ultrafast spin dynamics in ferromagnetic nickel. Phys. Rev. Lett. 76, 4250–4253 (1996).
Hohlfeld, J., Matthias, E., Knorren, R. & Bennemann, K. H. Nonequilibrium magnetization dynamics of nickel. Phys. Rev. Lett. 78, 4861–4864 (1997).
Koopmans, B., van Kampen, M., Kohlhepp, J. T. & de Jonge, W. J. Ultrafast magneto-optics in nickel: magnetism or optics? Phys. Rev. Lett. 85, 844–847 (2000).
van Kampen, M. et al. All-optical probe of coherent spin waves. Phys. Rev. Lett. 88, 227201 (2002).
Hashimoto, Y., Kobayashi, S. & Munekata, H. Photoinduced precession of magnetization in ferromagnetic (Ga, Mn)As. Phys. Rev. Lett. 100, 067202 (2008).
Kirilyuk, A., Kimel, A. V. & Rasing, T. H. Laser-induced magnetization dynamics and reversal in ferrimagnetic alloys. Rep. Prog. Phys. 76, 026501 (2013).
Gerrits, T. et al. Picosecond control of coherent magnetisation dynamics in permalloy thin films by picosecond magnetic field pulse shaping. J. Magn. Magn. Mater. 240, 283–286 (2002).
Gerrits, T., van den Berg, H. A. M., Hohlfeld, J., Bär, L. & Rasing, T. Ultrafast precessional magnetization reversal by picosecond magnetic field pulse shaping. Nature 418, 509–512 (2002).
Baierl, S. et al. Nonlinear spin control by terahertz-driven anisotropy fields. Nat. Photon. 10, 715–718 (2016).
Shalaby, M. et al. Terahertz macrospin dynamics in insulating ferrimagnets. Phys. Rev. B 88, 140301 (2013).
Vicario, C. et al. Off-resonant magnetization dynamics phase-locked to an intense phase-stable terahertz transient. Nat. Photon. 7, 720–723 (2013).
Bonetti, S. et al. THz-driven ultrafast spin-lattice scattering in amorphous metallic ferromagnets. Phys. Rev. Lett. 117, 087205 (2016).
Shalaby, M., Vicario, C. & Hauri, C. P. Simultaneous electronic and the magnetic excitation of a ferromagnet by intense THz pulses. New J. Phys. 18, 013019 (2016).
Chiba, D. et al. Magnetization vector manipulation by electric fields. Nature 455, 515–518 (2008).
Chiba, D. et al. Electrical control of the ferromagnetic phase transition in cobalt at room temperature. Nat. Mat. 10, 853–856 (2011).
Chiba, D., Yamanouch, M., Matsukura, F. & Ohno, H. Electrical manipulation of magnetization reversal in a ferromagnetic semiconductor. Science 301, 943–945 (2003).
Chiba, D., Matsukura, F. & Ohno, H. Electric-field control of ferromagnetism in (Ga, Mn)As. Appl. Phys. Lett. 89, 162505 (2006).
Sawicki, M. et al. Experimental probing of the interplay between ferromagnetism and localization in (Ga, Mn)As. Nature Phys. 6, 22–25 (2010).
Franz, W. Einfluß eines elektrischen feldes auf eine optische Absorptionskante. Z. Naturforsch. 13a, 484 (1958).
Keldysh, L. V. The effect of a strong electric field in the optical properties of insulating crystals. Soviet Physics JETP 7, 788 (1958).
Kampfrath, T., Tanaka, K. & Nelson, K. A. Resonant and nonresonant control over matter and light by intense terahertz transients. Nat. Photon. 7, 680–690 (2013).
Ishii, T. et al. Electronic structure near the Fermi level in the ferromagnetic semiconductor GaMnAs studied by ultrafast time-resolved light-induced reflectivity measurements. Phys. Rev. B 93, 241303 (2016).
Novelli, F., Fausti, D., Giusti, F., Parmigiani, F. & Hoffmann, M. Mixed regime of light-matter interaction revealed by phase sensitive measurements of the dynamical Franz-Keldysh effect. Sci. Rep. 3, 1227 (2013).
Pfeifer, T., Kütt, W., Kurz, H. & Scholz, R. Generation and detection of coherent optical phonons in germanium. Phys. Rev. Lett. 69, 3248 (1992).
Alping, A. & Coldren, L. A. Electrorefraction in GaAs and InGaAsP and its application to phase modulators. J. Appl. Phys. 61, 2430 (1987).
Kimel, A. V. et al. Observation of giant magnetic linear dichroism in (Ga, Mn)As. Phys. Rev. Lett. 94, 227203 (2005).
Kahn, F. J., Pershan, P. S. & Remeika, J. P. Ultraviolet magneto-optical properties of single-crystal orthoferrites, garnets, and other ferric oxide compounds. Phys. Rev. 186, 891–918 (1969).
Ohno, H. et al. Electric-field control of ferromagnetism. Nature 408, 944–946 (2000).
Sawicki, M. et al. Experimental probing of the interplay between ferromagnetism and localization in (Ga, Mn)As. Nat. Phys. 6, 22–25 (2010).
Matsuda, T. & Munekata, H. Mechanism of photoexcited precession of magnetization in (Ga, Mn)As on the basis of time-resolved spectroscopy. Phys. Rev. B 93, 075202 (2016).
Hebling, J., Almási, G., Kozma, I. Z. & Kuhl, J. Velocity matching by pulse front tilting for large area THz-pulse generation. Opt. Express 10, 1161–1166 (2002).
Hirori, H., Doi, A., Blanchard, F. & Tanaka, K. Single-cycle terahertz pulses with amplitudes exceeding 1 MV/cm generated by optical rectification in LiNbO3. Appl. Phys. Lett. 98, 091106 (2011).
Miyamoto, T., Yada, H., Yamakawa, H. & Okamoto, H. Ultrafast modulation of polarization amplitude by terahertz fields in electronic-type organic ferroelectrics. Nat. Commun. 4, 2586 (2013).
Acknowledgements
This work was partly supported by Grants-in-Aid for Scientific Research (No. 26249039, 18H03860, ISHO1011, 16H02095), the Project for Developing Innovation Systems of MEXT, Spintronics Research Network of Japan, and CREST, Japan Science and Technology Agency (Grant No. JPMJCR1661). T.I., H.Y. and T.K. were supported by the Japan Society for the Promotion of Science (JSPS) through the Program for Leading Graduate Schools (MERIT). T.I., H.Y. and T.K. thank the JSPS Research Fellowship Program for Young Scientists for support.
Author information
Authors and Affiliations
Contributions
T.I. and H.Y. conceived the experiment. T.K. and T.I. grew and characterized the samples. H.Y., T.M. and N.K. constructed the terahertz-pump optical-probe systems. T.I. and H.Y. performed the measurements. T.I. analysed the data. H.O., M.T. and S.O. coordinated the study. T.I. and S.O. wrote the paper with inputs from all authors.
Corresponding authors
Ethics declarations
Competing Interests
The authors declare no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Ishii, T., Yamakawa, H., Kanaki, T. et al. Ultrafast magnetization modulation induced by the electric field component of a terahertz pulse in a ferromagnetic-semiconductor thin film. Sci Rep 8, 6901 (2018). https://doi.org/10.1038/s41598-018-25266-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-018-25266-2
This article is cited by
-
Effects of Cobalt Nanoisland Geometry on Terahertz Negative Refraction: a Numerical Analysis
Journal of Superconductivity and Novel Magnetism (2021)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.