Abstract
The abundance variations of near surface atmospheric CO2 isotopologues (primarily 16O12C16O, 16O13C16O, 17O12C16O, and 18O12C16O) represent an integrated signal from anthropogenic/biogeochemical processes, including fossil fuel burning, biospheric photosynthesis and respiration, hydrospheric isotope exchange with water, and stratospheric photochemistry. Oxygen isotopes, in particular, are affected by the carbon and water cycles. Being a useful tracer that directly probes governing processes in CO2 biogeochemical cycles, Δ17O (=ln(1 + δ17O) − 0.516 × ln(1 + δ18O)) provides an alternative constraint on the strengths of the associated cycles involving CO2. Here, we analyze Δ17O data from four places (Taipei, Taiwan; South China Sea; La Jolla, United States; Jerusalem, Israel) in the northern hemisphere (with a total of 455 measurements) and find a rather narrow range (0.326 ± 0.005‰). A conservative estimate places a lower limit of 345 ± 70 PgC year−1 on the cycling flux between the terrestrial biosphere and atmosphere and infers a residence time of CO2 of 1.9 ± 0.3 years (upper limit) in the atmosphere. A Monte Carlo simulation that takes various plant uptake scenarios into account yields a terrestrial gross primary productivity of 120 ± 30 PgC year−1 and soil invasion of 110 ± 30 PgC year−1, providing a quantitative assessment utilizing the oxygen isotope anomaly for quantifying CO2 cycling.
Similar content being viewed by others
Introduction
The net growth rate and level of CO2 in the atmosphere represents a dynamic balance between anthropogenic activities and natural sources and sinks1. The diurnal and seasonal cycles, however, are largely affected by terrestrial photosynthesis and respiration2,3,4. The oxygen isotopic composition of atmospheric CO2 has been shown to be a powerful tracer for improving understanding of carbon and water cycles involving CO2 (ref.2,3,4,5,6,7,8,9), providing a unique way to estimate terrestrial gross primary productivity2,5,8,9. Oxygen has three stable isotopes (16O,17O, and18O). The18O/16O isotope ratio is used widely for studying aspects of the carbon and water cycles of natural systems2,3,5,6,7,8,9, but17O/16O has rarely been used owing to added analytical difficulty. Here, we compare and analyze the results of the triple-oxygen isotope composition of surface air CO2 from northern hemisphere sites in the western Pacific (South China Sea and Taipei, Taiwan)10 and available data from the middle east (Jerusalem, Israel)11 and western North America (La Jolla, United States)12, to provide deeper insight into the global CO2 cycle of the atmosphere, biosphere, and hydrosphere (The data from Göttingen, Germany13 are not included because of the presence of unknown drift in their two-year 17O data, though their first year of data agree well with the data reported in this work. Our approach is discussed in detail in a following section). We derive the residence time of CO2 in the atmosphere and gross primary production (GPP) from the integrated data set and discuss how interhemispheric transport affects these quantities. Given that the tropospheric mixing time in each hemisphere is much shorter than the interhemispheric mixing time14,15 and the latter shorter than the CO2 residence time derived here (see below), the compiled data should be a valid approximation of the global average.
We show that the terrestrial flux (the CO2 cycling flux between the atmosphere and terrestrial biosphere) can be quantified using the Δ17O values of CO2, where
and we set λ = 0.516 (a value commonly used in the literature16,17,18) and δ’s are referenced with respect to a commonly used scale, V-SMOW. Here, we follow typical notation (equation 1) to report the values of Δ17O. However, for the budget calculation that involves multiple-component mixing, Δ17O is not a conserved quantity19, and instead, the linear form of Δ17O, Δ, is used:
The budget formulation is then identical to that using δ’s. We note that for describing sources in the budget calculation, Δ17O and Δ are equally valid. For example, global meteoric water20 obeys the relation λ = 0.528, and the reported Δ17O value for δ18O greater than −10‰ is 0.032 ± 0.017‰. In contrast, Δ = 0.026 ± 0.017‰. The means are different but the standard deviations are the same, demonstrating that over the range of the δ18O values considered, there is no advantage of utilization of either equation (1) or (2) for source representation.
The advantage of using Δ17O (orΔ) over δ18O measurements is that Δ17O directly probes the associated processes in the carbon and water cycles11,20,21,22,23,24,25, as discussed in the next section. Moreover, λ, unlike δ, is insensitive to temperature, and both δ17O and δ18O are affected following the canonical mass-dependent relation23,26. Exchanging oxygen isotopes with water is the major process that we consider in determining CO2 fluxes between the atmosphere and biosphere/hydrosphere; the associated λ is well defined experimentally11,23, and the fluxes (e.g., the terrestrial flux - the cycling flux between the terrestrial biosphere and atmosphere, inferred from the oxygen isotopic composition of CO2) are robustly constrained (cf. ref.8).
A classic application of the triple isotope approach is the measurement of Δ17O in dissolved O2 in waters25,27,28. The biologically produced Δ17O value of O2 is balanced by the anomaly produced in the middle atmosphere as a consequence of O2-O3-CO2 photochemistry. Since the signal has a millennium time scale, it can be used to study biospheric changes during the past thousand years. In addition, there is a potentially analogous application for CO2, provided processes that affect Δ17O in CO2 are quantified. Similar to O2, the atmospheric Δ17O of CO2 is controlled by CO2-O2-O3 photochemistry and various anthropogenic, biospheric, and hydrospheric processes, including fossil fuel burning, photosynthesis, respiration, and exchange with leaf and soil water, oceans and other bodies of water11,16,18,19,29,30,31,32,33. The primary enhancing source of the oxygen isotopic anomaly resides in the middle atmosphere, as a consequence of the exchange reaction between CO2 and O3 via the excited state oxygen atom O(1D) (ref.29,30,31,32). Along with various sources19 and processes5,9,11,23,33 that determine the “net” Δ17O of CO2 emitted from the Earth’s surface, Hoag and co-authors16 investigated the contribution of stratospheric CO2 to the tropospheric CO2 mass-independent isotopic composition and predicted an anomaly of Δ17O ≈ 0.15‰, above the mean value of emissions/fluxes from the surface.
Sources and processes defining Δ17O values
The size of the anomaly is dependent upon the choice of λ and the reference scale. Here, we choose 0.516 for the slope and the most commonly used scale for oxygen, V-SMOW. We note that the selection of reference scale does not affect interpretation, provided the variation and partitioning among the three oxygen isotopes are properly accounted for. Given that the carbon flux estimation presented in this paper is based on the deviations of the oxygen anomalies of reservoirs/processes from that measured in atmospheric CO2, it is natural to take the λ value describing the variation of the triple-oxygen isotopic partitioning in tropospheric CO2. Processes that affect CO2 isotopologues in the troposphere are terrestrial, oceanic and anthropogenic, with the first being dominant. In the terrestrial biosphere, leaf water transpiration governs the variation of oxygen isotopes in CO2; the mean, however, is largely determined by water-CO2 equilibration catalyzed by carbonic anhydrase2,3,5,6,7,9,33. It has been found previously that the transpiration λ value is a function of air relative humidity21, whereas dependence on other meteorological variables such as temperature and soil water isotopic composition has not been observed. We set λ = 0.516, as it represents the transpiration λ at 75% relative humidity, a globally averaged humidity near the surface34. This λ value is essentially the same as that of CO2 we obtained (0.518 ± 0.004, see below) for the western Pacific, which had an average relative humidity of 76 ± 4% during 2010–2015 (data obtained from the Center for Weather Bureau, Taiwan; site code: 466920; the value changes slightly to 72 ± 11% if considering only day time between 6 AM to 6 PM). However, given the sparse spatiotemporal coverage of the data, the governing slope cannot be firmly decided. More data taken under a variety of environmental conditions are needed to set a better constraint. From the current understanding of the processes occurring, we consider plant transpiration the most important process affecting the variation of atmopsheric CO2 (e.g., see Landais et al.21 and Cuntz et al.3 and references contained therein). We stress that the value of λ does not affect the flux interpretation shown below, as long as equation (2) is used, but the selection must best represent the variation of atmospheric CO2.
A schematic diagram (not to scale) that describes various sources and processes modifying CO2 isotopologues is shown in Fig. 1, which summarizes the oxygen isotope transport at steady state. On a global scale, equilibrium processes are the major controllers in oxygen isotope dynamics; we show below in the Box model section that kinetic fractionations are insignificant. (Previous work13 utilized a time-dependent model showing a measurable seasonal cycle of Δ17O at an amplitude of ~0.05‰. The present work assesses the global carbon budget at steady state, leaving the assessment of spatiotemporal variability, including seasonality, to a latter paper when data covering a variety of spatial and temporal scales are available). Three sources/processes are considered: terrestrial (meteoric) water, ocean water, and anthropogenic CO2, with the last inheriting the atmospheric O2 isotopic composition. The variation of the triple oxygen isotopic composition of meteoric water follows a slope of 0.528 (ref.20). Subsequent isotopic exchange between water and atmospheric CO2 modifies the oxygen isotopic composition of CO2 following a slope of 0.5229 (ref.11). In addition, in the terrestrial biosphere, plant transpiration changes the source water following a slope of 0.516, a value chosen to represent the average slope at the globally averaged relative humidity of 75% (ref.21,34), as discussed above. These three values (0.528, 0.5229, and 0.516) determine the Δ17O value of CO2 mediated by processes involving water. We use the scheme in Fig. 1 to follow changes in oxygen isotopic composition, starting with meteoric water (point A). Transpiration that affects leaf water changes source meteoric water from A to B; the line AB follows the slope of 0.516. Subsequent water-CO2 isotopic exchange determines the composition of oxygen in leaf CO2, following the slope of 0.5229 to point C. For exchange with ocean water, only water-CO2 equilibration is involved, that changes CO2 to the blue “X.” When the CO2 enters the stratosphere, the coupled CO2-O2-O3 photochemistry moves the tropospheric CO2 to D; this path goes through mean tropospheric CO2 (diamond) and has slope of >1 (ref.29,30,31,32). Large-scale circulation and synoptic eddy mixing bring the modified CO2 back to the troposphere. The tropospheric CO2 (diamond) represents a balance among stratospheric (grey), terrestrial (yellow), oceanic (blue “X”), and the final component - anthropogenic CO2 (red symbol). Figure 1B, plotting Δ17O versus ln(1 + δ18O), shows that our reference λ is chosen (0.516) so that plant transpiration does not change the Δ17O value of terrestrial water. This is a rotation of Fig. 1A such that a slope of 0.516 becomes 0, and this removes the dominant variation along the correlation in Fig. 2A, as shown in Fig. 2B.
Schematic diagram (not to scale) of the sources and transport of CO2 considered in this paper. (A) ln(1 + δ17O) vs. ln(1 + δ18O) plot for meteoric water (blue), transpiration water (green), plant equilibrated CO2 (yellow), and stratospheric modified CO2 (grey). Ocean water equilibrated CO2 is shown by the blue “X” and averaged tropospheric CO2 value by the diamond. Anthropogenic CO2 is denoted by the explosive red starburst symbol. Arrows indicate transport. The slopes (m) for the lines AB, BC, and diamond-D are 0.516, 0.5229, and > 1, respectively. See text for details. (B) Similar to (A) but for Δ17O vs. ln(1 + δ18O). The corresponding slopes have been decreased by 0.516. Δ17Omw is the meteoric Δ17O, the y-intercept of the line AB. α18Owater-CO2 represents the fractionation in δ18O of water and CO2.
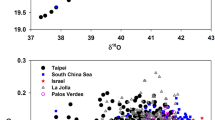
(A) ln(1 + δ17O) vs. ln(1 + δ18O) plot for atmospheric CO2 collected from Taipei (Taiwan), South China Sea, La Jolla (United States), and Jerusalem (Israel). Values in ‰ are referenced to V-SMOW. The geometric mean regression of the Taipei data gives ln(1 + δ17O) = (0.519 ± 0.005)× ln(1 + δ18O) + (0.2 ± 0.2‰). (B) The reported Δ17O values vs. ln(1 + δ18O). Note that the Δ17O values for the last two datasets have been re-scaled following equation (1). The error bars are smaller than the symbol size, with an error of ~0.05‰ for δ18O and ~0.01‰ for Δ17O. The two points (give values or reference to table where the data are given) from La Jolla beyond the plotting range of Δ17O are not shown.
Δ17O values of source CO2
In addition to well-quantified photochemical processes in the middle atmosphere, there are two known processes that modify Δ17O: combustion and isotope exchange with water. The former produces CO2 with Δ17O = −0.21‰, the same as air O2 (ref.19), used for the anthropogenic component below.
Isotopic exchange with water can be estimated using water-CO2 equilibrium11,23. Under the assumed definition of λ derived from equation (1), Δ17O of CO2 emitted from sources involving water-CO2 equilibration processes like respiration and soil invasion, following the slope of 0.5229 (ref.11), is (0.5229 − 0.516) × ln \(({{\alpha }^{18}}_{{\rm{w}}{\rm{a}}{\rm{t}}{\rm{e}}{\rm{r}}-{{\rm{C}}{\rm{O}}}_{2}})\,+\,{{\rm{\Delta }}}^{17}{O}_{{\rm{m}}{\rm{w}}}\) (see the previous section and Fig. 1), where \({{\alpha }^{18}}_{{\rm{w}}{\rm{a}}{\rm{t}}{\rm{e}}{\rm{r}}-{{\rm{C}}{\rm{O}}}_{2}}\) and Δ17Omw are, respectively, the fractionation factor for water equilibrated 18O in CO2 and Δ17O of water. See Fig. 1B for the schematics. We adopt \({{\alpha }^{18}}_{{\rm{w}}{\rm{a}}{\rm{t}}{\rm{e}}{\rm{r}}-{{\rm{C}}{\rm{O}}}_{2}}\,=\,1.043\) at a globally averaged land temperature of 15 °C (taken over 60° south to 75° north, where most biological activities occur; ref.34). Globally averaged meteoric water has Δ17Omw = −0.046 ± 0.005‰ (1 standard error; or 0.032 ± 0.003‰ at λ = 0.528; ref.20), excluding highly depleted waters having δ18O less than −10‰ in high latitude regions covered by snow and/or ice. (Here, standard error represents the error of a sample mean; standard deviation describes the error of a single measurement, the spread of replicate analyses of a single sample, or the spread of an ensemble). Figure 3 shows that the values of Δ17Omw from various regions are comparable.
The Δ17O value for terrestrial CO2 (Δ17Oland) is calculated to be 0.244 ± 0.005‰ ((0.5229 − 0.516) × ln(1.043) − 0.046‰ = 0.000244 = 0.244‰). We note that since water transpiration in plants follows λ = 0.516 at 75% relative humidity21, this process does not change Δ17O values of waters originating from meteoric water. This equivalence is a major advantage of choosing λ = 0.516 in equation (1). (We note that 5% variation in relative humidity results in ~0.015‰ change in the Δ17O of leaf water-equilibrated CO2, through water transpiration21).
The other largest water reservoir is the oceans. The globally averaged Δ17O of ocean waters (Δ17Oow) is 0.000 ± 0.001‰ (or −0.005 ± 0.001‰ at λ = 0.528; ref.20), and the resulting Δ17O of oceanic CO2 at 20 °C (ref.35) is 0.284 ± 0.001‰. The observation that Δ17O of meteoric water is lower than that of oceanic water suggests that the Δ17O approach has greater sensitivity to terrestrial processes than to oceanic ones.
Below we combine the values calculated above with data from several locations around the world (La Jolla, CA, USA; Jerusalem, Israel; Taipei, Taiwan; and the South China Sea) to put constraints on GPP and the oxygen isotope residence time of CO2 in the atmosphere. The oxygen isotope residence time is defined by the ratio of the atmospheric CO2 mass loading (M) and the CO2 mass flux between the atmosphere and biosphere/hydrosphere (Fsur). The flux is inferred from the mass balance calculation obtained using the triple oxygen isotopic composition of tropospheric CO2 shown below.
Methods
In addition to using data available in the literature from Jerusalem11, La Jolla12, and western Pacific regions10, we have continuously collected air for isotopic analysis of CO2 in three locations: (1) Academia Sinica campus (abbreviated AS; 121°36′51″ E, 25°02′27″ N; ~10 m above ground level or 60 m above sea level) in Taipei, Taiwan and (2) the campus of National Taiwan University (NTU; 121°32′21″ E, 25°00′53″ N; ~10 m above ground level or 20 m above sea level; ~10 km southwest of Academia Sinica). To check the reported Δ17O values in the eastern Pacific12, we have also collected and analyzed CO2 from Los Angeles, California at a latitude slightly higher than La Jolla, along the coast on Palos Verdes peninsula (118°10.9′ W, 33° 44.7′ N; PVD). Data reported in this work and analyzed in Taiwan are provided in full in the supplementary material.
Analytical methods are described in detail elsewhere10,18,36,37,38 and summarized here. Air from western Pacific regions for isotope analysis was collected in pre-conditioned 1-liter Pyrex bottles, achieved by passing dry, high purity nitrogen through the bottles overnight. The sampling bottles used for concentration (~350-ml bottle) and isotope (1-liter) analyses were connected in series. Samples were collected and compressed to 2-bar after flushing the bottles for 5 minutes with ambient air at a flow rate of ~2 liter per min. Moisture was removed during sampling using magnesium perchlorate to minimize subsequent isotope exchange between CO2 and water39. Concentration of CO2 is measured using a LI-COR infrared gas analyzer (model 840A, LI-COR, USA), with reproducibility better than 1 ppmv. The PVD samples were collected on Saturday afternoons at about 14:00 PST, into 2-liter evacuated Pyrex flasks after passing through Mg(ClO4)2. Carbon dioxide was separated from the air samples cryogenically and measured, following the method described in Newman et al.38. The CO2-O2 oxygen isotope exchange method developed previously36,37 was used to measure the Δ17O of CO2 samples. Isotopic analyses were done using a FINNIGAN MAT 253 mass spectrometer in the dual inlet mode. The analytical precision obtained for a single measurement of the Δ17O value of CO2 is better than 0.01‰ (1-σ standard deviation).
Results
The concentrations of the isotopologues of CO2 at South China Sea (SCS) are close to those reported at Mauna Loa measured by the National Oceanic and Atmospheric Administration - Earth System Research Laboratory (data available online at http://www.esrl.noaa.gov/gmd/dv/iadv/), with [CO2] 395.4 ± 7.3 ppmv and δ13C −8.47 ± 0.22‰ (1-σ standard deviation). In Taipei (AS + NTU), the averaged [CO2] is 416.2 ± 18.3 ppmv, δ13C is −9.22 ± 0.83‰, and δ18O is 40.65 ± 0.82‰. [CO2] varies between ~350 and 475 ppmv, with low values during the day and high at night, representing the combined effect of natural biogeochemical cycle (photosynthesis and respiration), anthropogenic emissions, and boundary mixed layer height diurnal variation38. During day time hours, photosynthesis dominates, resulting in reduction of CO2 concentration and less negative δ13C and δ18O values. The CO2 content is lower during the day than night also due to dilution as the boundary layer deepens. The mean values obtained for [CO2] and δ13C, as compared to SCS, show that in Taipei a clear contribution from anthropogenic emissions is seen. Given the proximity of the AS and NTU stations (~10 km apart), air transport time is shorter than the CO2 oxygen isotope residence time shown below, resulting in, on a yearly basis, similar levels of CO2 isotopologues, including Δ17O.
Figure 2A compiles tropospheric CO2 data in a plot of ln(1 + δ17O) vs. ln(1 + δ18O). The least square linear regression analysis of the data obtained in Taipei yields a slope of 0.518 ± 0.004 (excluding some outliers at δ18O < 38.5‰) and intercept of 0.3 ± 0.2‰ (1 standard error, R2 = 0.99). The observation that the same slope is obtained as for transpiration at ~75% relative humidity (close to the value at the sampling sites in western Pacific, 76 ± 4% averaged between 2010 and 2015) suggests that transpiration is likely a controlling process affecting the variation of the triple oxygen isotopic composition of near surface CO2. As the variation of oxygen isotopic compositions in CO2 at the two Taipei sampling sites is biogeochemically mediated, one may use Δ17O to estimate the actual “flux” between the atmosphere and soil/leaf, which in turn gives the value for GPP. The overall ratio of ln(1 + δ17O)/ln(1 + δ18O) for Taipei is 0.524 ± 0.001, consistent with that of a water-CO2 equilibrium value of 0.5229 ± 0.0001 (ref.11,23), further verifying that the oxygen of near-surface CO2 in this region is primarily affected by biogeochemistry with minor influences from the stratosphere and human activities. The Δ17O values in Taipei vary from 0.216 to 0.415‰ (Fig. 2B) with an average of 0.335 ± 0.039‰ (1-σ standard deviation of the range), a value similar to 0.31 ± 0.06‰ obtained in La Jolla12 and 0.321 ± 0.007‰ in Jerusalem11. (Note that the values for the last two have been re-scaled with respect to λ = 0.516, for the sake of consistency among the data sets). The value at SCS is 0.335 ± 0.033‰. The value reported at La Jolla is the lowest among the four. We then check the possibility that the difference between La Jolla and Taiwan is caused by the difference in Δ17O scale between the two labs. By taking CO2 samples collected also in the eastern Pacific region but at a higher latitude near a southwest facing beach on the Palos Verdes peninsula (PVD). The obtained values for 2015 are 0.317 ± 0.032‰, essentially the same as the decadal mean from La Jolla. The average of the mean Δ17O values from La Jolla, Jerusalem, Taipei, and the South China Sea (0.326 ± 0.005‰) is used below in the box model calculation of GPP and oxygen isotope residence time. The averaged Δ17O value decreases by only 0.004‰ if just the two largest datasets, from Taiwan and La Jolla, are averaged. Therefore, we note that whether the SCS, Jerusalem, and PVD data are included does not change the conclusion presented below. For example, a 0.01‰ reduction in Δ17O results in ~0.2-year decrease in the oxygen isotope residence time. Moreover, given that the oxygen isotope residence time is on the order of one year only and sources and processes that change the isotopologues of the tropospheric CO2 are variable spatially and temporally, spatiotemporal inhomogeneity in Δ17O is expected. The average of the mean values from more locations should be more representative for the global Δ17O.
Box model
A box model is employed to assess various contributing processes for Δ17O. At steady state, the mass balance equation for δ (where δ is either δ17O or δ18O), following Cuntz et al.3, can be written as follows:
where C is the volume mixing ratio of CO2, M is the mass of the atmosphere, subscripts “a”, “st”, “anth”, “l”, “r”, “s”, and “o” of δ’s represent the δ values of the sampled air, the stratosphere, anthropogenic emissions, and leaf, respiration, soil, and ocean water, respectively, ε’s are the associated kinetic fractionation factors, and F is the flux in and out of a reservoir such that the subscript “la” refers to leaf-to-air, “al” air-to-leaf, and “ao” air-to-ocean. We then rewrite the equation (3) in terms of Δ in steady state as follows.
where the kinetic terms ((Fla − Fal) × εl × (λl − λ0) + Fr × εs × (λs − λ0) + Fao × εo × (λo − λ0)) become negligible (even with extreme values for F at 500 PgC year−1 or λl,s,o at 0.529, the value for equilibrium water between condensed and vapor phases, the isoflux is found to be less than 1‰ PgC year−1). We note that Δ in the linear definition in equation (4) obeys mass conservation whereas Δ17O in the logarithmic definition does not. The use of Δ17O in equation (4) results in an error about 10% in each term derived, i.e., ~40 PgC year−1 biased too high in Fla + Fr + Fs and ~0.2 year too short in the resident time of CO2 in the atmosphere (though still within the error of the estimation). Parameters and values used in the box modeling are summarized in Table 1.
Finally, we have Δ l = −0.009 ± 0.006‰, Δ r = Δ s = 0.019 ± 0.006‰, Δ o = 0.075 ± 0.001‰, and Δ anth = −0.286 ± 0.001‰ (or Δ17Ol = Δ17Or = Δ17Os = Δ17Oland = 0.244 ± 0.005‰, Δ17Oo = 0.284 ± 0.001‰, and Δ17Oanth = −0.213 ± 0.001‰). The oceanic flux Foa is 90 ± 6 PgC year−1 (an average of IPCC 2001, 2007, and 2013; ref.1,2,3,40), Fanth is 9.4 ± 0.8 PgC year−1 for year 2011 (ref.1,41). We further take Fla ≈ 2Fr ≈ 2Fs (from the fact that global net productivity is much less than the gross productivity, and the assumption of catalyzed soil invasion7), and with this, the terrestrial flux Fland equal to Fla + Fr + Fs. Then equation (4) can be reduced to
For stratospheric flux, global model simulations17 that consider various atmospheric transports yield an averaged isoflux from the stratosphere, Fst × (Δ st − Δ a), of 50 ± 3‰ PgC year−1, consistent with that obtained and used previously (~43‰ PgC year−1; ref.16,42). Figure 4 summarizes the derived terrestrial CO2 flux and residence time in the atmosphere; in this particular model, the cross-hemispheric transport and mixing are not included, as the hemispheric difference in Δ17O was predicted to be small (<0.01‰; ref.16). Sensitivities of the derived quantities with respect to the variations of the relative importance of ocean flux, cross-tropopause exchange flux, soil invasion, and Δ17O value in the southern hemisphere are presented in Fig. 5. See below for the detail on the error assessment.
(A) CO2 recycling time (pink) as a function of Δ17O in atmospheric CO2 measured near the surface, calculated using equation (5), the box model. The corresponding oceanic (blue) and terrestrial (green) fluxes (the cycling flux between the atmosphere and terrestrial biosphere) are also shown (right-hand axis). The vertical dashed line represents the measured Δ17O in the tropospheric CO2. Shaded zones represent 1-σ error. (B) The isoflux of Δ17O from anthropogenic (gold), terrestrial (green), oceanic (blue), and stratospheric (black) sources, as a function of Δ17O in atmospheric CO2, with 1-σ error shown by the shaded areas. At Δ17O = 0.326‰, the terrestrial isoflux (42‰ PgC year−1) is a factor of 10 higher than the oceanic isoflux (5‰ PgC year−1), suggesting that the Δ17O approach has greater sensitivity to terrestrial processes than oceanic processes. For easier comparison, we have multiplied the oceanic and terrestrial isofluxes by −1.
(A) The calculated recycling time of CO2 (pink line), as a function of Fland/Fsur. Stratospheric isoflux is adopted to be 50‰ PgC year−1; anthropogenic flux is 9.4 PgC year−1. The required fluxes from the land and ocean to reproduce the observed Δ17O = 0.326‰ in air CO2 are also shown, in green and blue, respectively. The vertical dashed line is drawn through the generally adopted oceanic flux of 90 PgC year−1. (B) The calculated recycling time of CO2, as a function of the stratospheric isoflux, assuming an oceanic flux of 90 PgC year−1 and Δ17O = 0.325‰ in air CO2. The nominal flux adopted in (A) is indicated by the vertical dashed line. The associated terrestrial flux is also shown. (C) Estimated GPP as a function of soil invasion flux, for two photosynthetic scenarios (θ eq represents the degree of hydration of CO2 in stomata and κ c is a measure of stomatal conductance). The stratospheric isoflux is set to be 50‰ PgC year−1, anthropogenic flux is 9.4 PgC year−1, and air CO2 Δ17O = 0.326‰. (D) Estimated GPP as a function of southern hemisphere (SH) CO2 Δ17O. The stratospheric isoflux is set to be 50‰ PgC year−1, anthropogenic flux is 9.4 PgC year−1, and northern hemisphere air CO2 Δ17O = 0.326‰ (marked by the vertical dashed line).
The current steady state box model is an updated version of Hoag et al.16; the major surface resetting processes are included explicitly to distinguish the terrestrial (re-)cycling fluxes from the oceanic. Previous works (Cuntz et al.3 and Hofmann et al.13) solve time-dependent equations (equation 3). In this case, kinetic fractionations becomes important, and spatiotemporal variabilities in all components of carbon/oxygen cycling and recycling fluxes are significant. As a result, spatial and temporal inhomogeneity in sampling has to be considered and evaluated critically. When more data are available, natural variability in carbon fluxes can be assessed. Therefore, for examining the global carbon flux at steady state, we take measurements spanning as much space and time as possible. For error analysis, a standard error from each component of measurements is adopted, leaving standard deviation for representing spatiotemporal variability assessment in time-dependent models.
Assessing gross primary productivity
Plant uptake scenarios affect the estimates of GPP and soil invasion. GPP can be estimated as follows:
where θ eq represents the degree of hydration of CO2 in stomata and κ c is a measure of stomatal conductance which can be expressed by
where C c is the CO2 concentration in chloroplasts at the site of CO2 hydration and C a is the atmospheric concentration. The factor 0.88 is used to account for leaf respiration43. For C3 plants, C c /C a = 2/3; for C4 plants, C c /C a = 1/3, assuming that C c is equal to intracellular CO2 concentration44. A generally averaged C c /C a is 0.57 or κ c = 1.33 (ref.8,9). Overall, the value of κ c varies between 1.33 and 2.93 (ref.3,9). θ eq is also variable33,45. Careful error assessment is made to quantify the effect of this variation on the value of GPP (Fig. 5C). See Table 1 for the summary of the parameters and the associated errors and variations used in this work.
Errors resulted from incomplete understanding of sources and the Δ17O values in various water bodies (meteoric, soil, and oceanic waters) and global atmospheric CO2 (i.e., tropospheric and stratosphere-to-troposphere stream Δ17O). The corresponding errors are summarized in Table 1. There are, however, other related processes whose λ values remain poorly known, of which transpiration and plant uptake scenarios (i.e., chloroplast CO2 concentration and enzyme catalyzed oxygen exchange efficiency) are the most uncertain. Transpiration is sensitive to plant species and ambient air relative humidity21. If we assume an average considered in Landais et al.21, the variation in λ results in a variation of 0.017‰ for Δ17O for a 0.05 change in relative humidity; this term contributes ~30% of the error (~1/3 from atmospheric CO2 measurements and the remaining from the cross-tropopause exchange Δ17O flux) in estimating the global oxygen isotope residence time and terrestrial carbon flux. Proper error propagation is made to assess the error in the terrestrial flux (Fland) derived in equation (5), followed by a Monte Carlo approach to evaluate the errors from κ c and θ eq in equation (6) for GPP estimate.
A function f with n independent variables is expressed as:
The error of each quantity x i is given by the standard deviation (σ i ) or standard error \((\overline{{\sigma }_{i}})\). The former refers to the error of a single measurement while the latter is used to represent the error of a sample mean. With a large set of measurements, we use \(\overline{{\sigma }_{i}}\) to represent the error of the sample mean \(\overline{{x}_{i}}\). The errors in equation (4) refer to such an error. Following standard error propagation, the error of the function f is
For a single measurement, i.e., one measurement only for each of the variable x i , the final error σ f is the square root of \(\sum _{i=1}^{i=n}{(\frac{\partial f}{\partial {x}_{i}})}^{2}{\sigma }_{i}^{2}.\,\) Therefore, for a set of many measurements reported in this work, the estimated terrestrial flux Fland is 345 PgC year−1, with 1 standard error being 70 PgC year−1. However, for each of the variables in equation (4) (Δ17 a, Δ17 l or Δ17Omw, Foa, Fanth, and Fst × (Δ17 st − Δ17 a)), if we measure them once, the error, or sample inhomogeneity due to inhomogeneity in sources and processes, increases to 600 PgC year−1. For errors resulting from global carbon cycling scenarios, a Monte Carlo simulation is employed. The final error is estimated from 500 × 500 × 500 = 125 M (500 samplings for the terrestrial flux and 500 each for two variables in the carbon model in equation (6); the errors are converged with >300 random samplings) calculations of the model using repeated random sampling of each of the three variables. From the literature, κ c ranges from 1.33 to 2.97 (depending critically on C c ; ref.3,9) and θ eq from 0.7 to 0.78 (affected by enzyme carbonic anhydrase activity in C4 plants; ref.9,33,44). To consider final total errors in GPP and Fs, we perform Monte Carlo simulations to randomly determine values for κ c and θ eq over the aforementioned ranges. The distribution of these two parameters is assumed to be uniform, with the measurement error of Δ17O assumed to be following normal distribution.
Oxygen isotope residence time and gross primary productivity
Figure 4 summarizes the model results calculated using equation (5) and a value for Δ17O of 0.326 ± 0.005‰ for the troposphere, the average for the four locations discussed above. The current mass loading of atmospheric CO2 (M) is 828 ± 10 PgC (ref.1,45). The CO2 oxygen isotope residence time τ is given by M/Fsur, where Fsur = Fland + Fao. Taking into account the aforementioned uncertainties of the parameters in equation (5), the terrestrial flux Fland is determined to be 345 ± 70 PgC year−1, and τ is 1.9 ± 0.3 years, consistent with previous estimates2,3,8,9. The estimate is insensitive to the partitioning between ocean and terrestrial fluxes because of the sensitivity of the Δ17O approach to the terrestrial processes (Fig. 4). However, we show below that we cannot constrain the value for GPP better than other methods, because of unknown quantities for soil invasion and degree of isotopic equilibrium between leaf water and stomatal CO2.
No significant advancement toward quantifying soil invasion has been made since Wingate et al.7. The reported flux can be as low as <10 PgC year−1 (ref.6) to as high as 450 PgC year−1 (ref.7), depending on the catalyzed hydration activity (via enzyme carbonic anhydrase). Recently, the hydration activity has been found to likely be high, resulting in an invasion flux as high as respiration7, and we choose this as our best estimate, i.e., Fs = Fr. By definition, GPP is the sum of respiration Fr and NEP (net ecosystem productivity). So equation (6) can be expressed by
The globally estimated NEP is 10 PgC year−1 (ref.46). Once the values of κ c and θ eq are chosen, along with the value of Fland reported above, GPP and Fs can be derived.
With a previously suggested plant uptake scenario (κ c = 1.33 and θ eq = 0.78; ref.8) and an independent constraint for net ecosystem productivity46,47, we derive an estimate of 130 ± 25 PgC year−1 for GPP, with a best guess for soil invasion of 120 ± 20 PgC year−1 (calculated from equation (10)). A Monte Carlo simulation that considers various carbon cycling models3,8,9,33,44, including plant types and degree of oxygen equilibrium with various water bodies in the biosphere and hydrosphere, gives the estimates of GPP and soil invasion to 120 ± 30 and 110 ± 30 PgC year−1, respectively. The estimated GPP is toward the lower end of Welp et al.8 but close to that of Beer et al.4. Given that the value of Δ17O is sensitive to the isofluxes between atmospheric CO2 and water bodies, we expect that extended studies with multiple CO2 isotopologues into C3-dominated regions such as the Amazonian rainforest, C4-dominated lands such as grasslands in North America, and vegetation-sparse areas such as the Canadian arctic could have great potential to refine the partitioning between photosynthesis, respiration and soil invasion, and thus to provide a better estimate of the terrestrial GPP.
Figure 5 shows the sensitivities of the derived quantities with respect to the variations of the ocean flux (expressed as variations in the fraction of flux from land to flux from the total surface) (A), cross-tropopause exchange flux (B), soil invasion (C), and Δ17O value in the southern hemisphere (D). Because of the low value of the oceanic isoflux shown in Fig. 4B, the ocean affects the derived terrestrial flux and CO2 residence time weakly; changing the oceanic flux by 50% changes the residence time by 0.1 year. The stratospheric flux, however, is more sensitive. 10% changes in the stratospheric flux result in ~0.2 year changes in the residence time. The sensitivity to soil invasion (Fig. 5C) is calculated by varying the variable with Fland fixed at 345 PgC year−1. Depending on soil invasion and plant uptake scenarios (the values of κ c and θ eq ), the value of GPP could vary between 0 and 200 PgC year−1. Another important value that remains undetermined is the value of Δ17O of tropospheric CO2 in the southern hemisphere. Figure 5D shows that the GPP and residence time are sensitive to the Δ17O value in the southern hemisphere; the sensitivity is obtained by assuming Fs = 110 PgC year−1, Fland = 345 PgC year−1, κ c = 1.33, and θ eq = 0.78. If Δ17O in the southern hemisphere is 0.01‰ (0.02‰) higher than that in the northern hemisphere, the GPP value increases to 170 (200) PgC year−1; the residence time then reduces to 1.7 (1.5) years. As soon as the value of Δ17O in the southern hemisphere is measured, the global residence time can be determined and GPP can be better quantified.
In summary, the triple-oxygen isotopic composition of CO2 constrains the global oxygen isotopic residence time in the atmosphere to 1.9 ± 0.3 years, compared to 0.9–1.7 years (ref.8) or longer2,3,9 reported previously. The terrestrial flux is quantified to be 345 ± 70 PgC year−1, falling in the range reported in the literature, 200–660 PgC year−1 (ref.2,3,8,9). Because of the isotope recycling time of CO2, the spatial inhomogeneity of Δ17O obtained between localities shows that the commonly used δ values can be applied to Δ17O to refine knowledge of the flux partitioned between respiration/soil invasion, photosynthesis, and air-sea exchange. CO2 sampling campaigns in the remote Pacific and southern hemisphere oceans can better remove interference from terrestrial processes, to quantify the oceanic flux. High-resolution global and regional models assimilating CO2 isotopologues (Δ17O in particular) with online carbon and water cycle modules can potentially strengthen our understanding of the associated processes at molecular scales. We expect that existing models3 coupled with a cross-tropopause exchange module extending surface biogeochemical models to include stratospheric processes will greatly improve our estimates and provide extraordinary precision to probe the associated fluxes in the global carbon and water cycles involving CO2.
References
Stocker, T. F., Dahe, Q., & Plattner, G. K. Climate Change 2013: The Physical Science Basis, Working Group I Contribution to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change. Summary for Policymakers (IPCC, 2013).
Ciais, P. et al. A three-dimensional synthesis study of δ18O in atmospheric CO2. 1. Surface fluxes. Journal of Geophysical Research-Atmospheres 102, 5857–5872 (1997).
Cuntz, M. et al. A comprehensive global three-dimensional model of δ18O in atmospheric CO2: 2. Mapping the atmospheric signal. J. Geophys. Res. 108(D17), 4528 (2003).
Beer, C. et al. Terrestrial Gross Carbon Dioxide Uptake: Global Distribution and Covariation with Climate. Science 329, 834–838 (2010).
Francey, R. J. & Tans, P. P. Latitudinal variation in O18 of atmospheric CO2. Nature 327, 495–497 (1987).
Stern, L. A., Amundson, R. & Baisden, W. T. Influence of soils on oxygen isotope ratio of atmospheric CO2. Global Biogeochemical Cycles 15, 753–759 (2001).
Wingate, L. et al. The impact of soil microorganisms on the global budget of δ18O in atmospheric CO2. Proceedings of the National Academy of Sciences of the United States of America 106, 22411–22415 (2009).
Welp, L. P. et al. Interannual variability in the oxygen isotopes of atmospheric CO2 driven by El Niño. Nature 477(7366), 579–582 (2011).
Farquhar, G. D. et al. Vegetation effects on the isotope composition of oxygen in atmospheric CO2. Nature 363(6428), 439–443 (1993).
Liang, M. C., Mahata, S., Laskar, A. H. & Bhattacharya, S. K. Spatiotemporal Variability of Oxygen Isotope Anomaly in near Surface Air CO2 over Urban, Semi-Urban and Ocean Areas in and around Taiwan. Aerosol and Air Quality Research 17(3), 706–720 (2017).
Barkan, E. & Luz, B. High‐precision measurements of 17O/16O and 18O/16O ratios in CO2. Rapid communications in mass spectrometry 26, 2733–2738 (2012).
Thiemens, M. H., Chakraborty, S. & Jackson, T. L. Decadal Δ17O record of tropospheric CO2: Verification of a stratospheric component in the troposphere. Journal of Geophysical Research-Atmospheres 119, 6221–6229 (2014).
Hofmann, M. E. G., Horváth, B., Schneider, L., Peters, W., Schützenmeister, K. & Pack, A. Atmospheric measurements of Δ17O in CO2 in Göttingen, Germany reveal a seasonal cycle driven by biospheric uptake. Geochimica et Cosmochimica Acta 199, 143–163 (2017).
Jacob, D. J., Prather, M. J., Wofsy, S. C. & McElroy, M. B. Atmospheric distribution of 85Kr simulated with a general circulation model. Journal of Geophysical Research 92(D6), 6614–6626 (1987).
Lal, D. & Rama, Characteristics of global tropospheric mixing based on man-made C14, H3, and Sr90. Journal of Geophysical Research 71(12), 2865–2874, https://doi.org/10.1029/JZ071i012p02865 (1966).
Hoag, K., Still, C., Fung, I. & Boering, K. A. Triple oxygen isotope composition of tropospheric carbon dioxide as a tracer of terrestrial gross carbon fluxe. Geophys. Res. Lett. 32(2), L02802 (2005).
Liang, M. C., Blake, G. A. & Yung, Y. L. Seasonal cycle of C16O16O, C16O17O, and C16O18O in the middle atmosphere: Implications for mesospheric dynamics and biogeochemical sources and sinks of CO2. Journal of Geophysical Research-Atmospheres 113, https://doi.org/10.1029/2007jd008392 (2008).
Liang, M. C. & Mahata, S. Oxygen anomaly in near surface carbon dioxide reveals deep stratospheric intrusion. Scientific Reports 5, 11352 (2015).
Laskar, A. H., Mahata, S. & Liang, M. C. Identification of anthropogenic CO2 using triple oxygen and clumped isotopes. Environmental Science & Technology 50(21), 11806–11814 (2016).
Luz, B. & Barkan, E. Variations of 17O/16O and 18O/16O in meteoric waters. Geochimica et Cosmochimica Acta 74, 6276–6286 (2010).
Landais, A., Barkan, E., Yakir, D. & Luz, B. The triple isotopic composition of oxygen in leaf water. Geochimica et Cosmochimica Acta 70, 4105–4115 (2006).
Barkan, E. & Luz, B. Diffusivity fractionations of H2 16O/H2 17O and H2 16O/H2 18O in air and their implications for isotope hydrology. Rapid Communications in Mass Spectrometry 21, 2999–3005 (2007).
Hofmann, M. E. G., Horvath, B. & Pack, A. Triple oxygen isotope equilibrium fractionation between carbon dioxide and water. Earth and Planetary Science Letters 319, 159–164 (2012).
Luz, B. & Barkan, E. The isotopic ratios 17O/16O and 18O/16O in molecular oxygen and their significance in biogeochemistry. Geochimica et Cosmochimica Acta 69, 1099–1110 (2005).
Luz, B., Barkan, E., Bender, M. L., Thiemens, M. H. & Boering, K. A. Triple-isotope composition of atmospheric oxygen as a tracer of biosphere productivity. Nature 400, 547–550 (1999).
Cao, X. & Liu, Y. Equilibrium mass-dependent fractionation relationships for triple oxygen isotopes. Geochimica et Cosmochimica Acta 75, 7435–7445 (2011).
Luz, B. & Barkan, E. Assessment of oceanic productivity with the triple-isotope composition of dissolved oxygen. Science 288, 2028–2031 (2000).
Jurikova, H., Guha, T., Abe, O., Shiah, F. K., Wang, C. H. & Liang, M. C. Variations in triple isotope composition of dissolved oxygen and primary production in a subtropical reservoir. Biogeosciences 13(4), 6683–6698 (2016).
Thiemens, M. H., Jackson, T., Zipf, E. C., Erdman, P. W. & Vanegmond, C. Carbon-dioxide and oxygen-isotope anomalies in the mesosphere and stratosphere. Science 270, 969–972 (1995).
Liang, M. C., Blake, G. A., Lewis, B. R. & Yung, Y. L. Oxygen isotopic composition of carbon dioxide in the middle atmosphere. Proceedings of the National Academy of Sciences of the United States of America 104, 21–25 (2007).
Thiemens, M. H., Jackson, T., Mauersberger, K., Schueler, B. & Morton, J. Oxygen isotope fractionation in stratospheric CO2. Geophysical Research Letters 18, 669–672 (1991).
Yung, Y. L., DeMore, W. B. & Pinto, J. P. Isotopic exchange between carbon-dioxide and ozone via O(1D) in the stratosphere. Geophysical Research Letters 18, 13–16 (1991).
Gillon, J. & Yakir, D. Influence of carbonic anhydrase activity in terrestrial vegetation on the 18O content of atmospheric CO2. Science 291, 2584–2587 (2001).
Dai, A. Recent Climatology, Variability, and Trends in Global Surface Humidity. Journal of Climate 19, 3589–3606 (2006).
Brenninkmeijer, C. A. M., Kraft, P. & Mook, W. G. Oxygen isotope fractionation between CO2 and H2O. Isotope Geoscience 1(2), 181–190 (1983).
Mahata, S., Bhattacharya, S. K., Wang, C. H. & Liang, M. C. Oxygen isotope exchange between O2 and CO2 over hot platinum: An innovative technique for measuring Δ17O in CO2. Anal. Chem. 85(14), 6894–6901 (2013).
Mahata, S., Bhattacharya, S. K. & Liang, M. C. An improved method of high‐precision determination of Δ17O of CO2 by catalyzed exchange with O2 using hot platinum. Rapid Communications in Mass Spectrometry 30, 119–131 (2016).
Newman, S. et al. Changes in mixing ratio and isotopic composition of CO2 in urban air from the Los Angeles basin, California, between 1972 and 2003. Journal of Geophysical Research-Atmospheres 113, doi:https://doi.org/10.1029/2008JD00999 (2008).
Gemery, P. A., Trolier, M. & White, J. W. Oxygen isotope exchange between carbon dioxide and water following atmospheric sampling using glass flasks. Journal of Geophysical Research: Atmospheres 101, 14415–14420 (1996).
Solomon, S. Climate change 2007-the physical science basis: Working group I contribution to the fourth assessment report of the IPCC, Cambridge University Press (2007).
Peters, G. P., Marland, G., Le Quéré, C., Boden, T., Canadell, J. G. & Raupach, M. R. Rapid growth in CO2 emissions after the 2008-2009 global financial crisis. Nature Climate Change 2(1), 2–4 (2012).
Boering, K. A. et al. Observations of the anomalous oxygen isotopic composition of carbon dioxide in the lower stratosphere and the flux of the anomaly to the troposphere. Geophysical Research Letters 31, L03109 (2004).
Pearcy, R. & Ehleringer, J. Comparative ecophysiology of C3 and C4 plants. Plant, Cell & Environment 7, 1–13 (1984).
Cousins, A. B., Badger, M. R. & von Caemmerer, S. C4 photosynthetic isotope exchange in NAD-ME- and NADP-ME-type grasses. Journal of Experimental Botany 59, 1695 (2008).
Joos, F. et al. Carbon dioxide and climate impulse response functions for the computation of greenhouse gas metrics: a multi-model analysis. Atmospheric Chemistry and Physics 13, 2793–2825 (2013).
Saugier, B., Roy, J. & Mooney, H. A. Estimations of global terrestrial productivity: converging toward a single number. Terrestrial global productivity, 543–557 (Academic Press, 2001).
Houghton, J. T. et al. Climate change 2001: the scientific basis. (2001).
Li, S., Levin, N. E. & Chesson, L. A. Continental scale variation in 17O-excess of meteoric waters in the United States. Geochimica et Cosmochimica Acta 164, 110–126 (2015).
Landais, A., Risi, C., Bony, S., Vimeux, F., Descroix, L., Falourd, S. & Bouygues, A. Combined measurements of 17Oexcess and d-excess in African monsoon precipitation: Implications for evaluating convective parameterizations. Earth and Planetary Science Letters 298(1), 104–112 (2010).
Miller, J. B., Yakir, D., White, J. W. C. & Tans, P. P. Measurement of 18O/16O in the soil-atmosphere CO2 flux. Global Biogeochemical Cycles 13, 761–774, https://doi.org/10.1029/1999gb900028 (1999).
Vogel, J. C., Grootes, P. M. & Mook, W. G. Isotopic fractionation between gaseous and dissolved carbon dioxide. Zeitschrift Fur Physik 230, 225, https://doi.org/10.1007/bf01394688 (1970).
Acknowledgements
Special thank is due Chung-Ho Wang and Institute of Earth Sciences for providing lab space to accommodate our instruments, Chien-Chang Yen for discussion on error analysis, and S. K. Bhattacharya and Yuk Yung for helpful discussion. This work was supported in part by a MOST grant 105–2111-M-001-006-MY3 to Academia Sinica.
Author information
Authors and Affiliations
Contributions
M.C.L. analysed the data and wrote the paper. S.M., A.H.L., and S.N. collected samples and performed analyses. M.H.T. provided calibrations. All authors discussed the results and commented on the manuscript.
Corresponding author
Ethics declarations
Competing Interests
The authors declare that they have no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Liang, MC., Mahata, S., Laskar, A.H. et al. Oxygen isotope anomaly in tropospheric CO2 and implications for CO2 residence time in the atmosphere and gross primary productivity. Sci Rep 7, 13180 (2017). https://doi.org/10.1038/s41598-017-12774-w
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-017-12774-w
This article is cited by
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.