Abstract
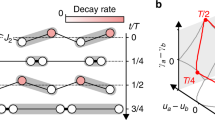
Thouless pumping enables topological transport and the direct measurement of topological invariants. So far, realizations of Thouless pumping rely on the adiabatic evolution of a physical system following a non-degenerate band, but it has been predicted that pumping can become non-Abelian in nature when degenerate bands exist. The resulting non-Abelian gauge fields and associated non-commutative operations would be promising for applications related to unitary matrices, such as photonic quantum logic. Here we propose the experimental realization of non-Abelian Thouless pumping in an on-chip photonic platform. By modulating the coupling coefficients within photonic waveguides with degenerate flat bands, we observe non-Abelian Thouless pumping in a three-step pumping device where the outcomes depend on the sequence of the pumping operations. We anticipate our versatile platform to reveal more complex non-Abelian topological physics and realize on-chip non-Abelian photonic devices in the future.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
Source data are provided with this paper. All other data that support the plots within this paper and other findings of this study are available from the corresponding authors upon reasonable request.
Code availability
The codes used for performing the theoretical analysis and numerical simulations are available from X.-L.Z. upon reasonable request.
References
Thouless, D. J. Quantization of particle transport. Phys. Rev. B 27, 6083–6087 (1983).
Wang, Z., Chong, Y., Joannopoulos, J. D. & Soljai, M. Observation of unidirectional backscattering-immune topological electromagnetic states. Nature 461, 772–775 (2009).
Qi, X. L. & Zhang, S. C. Topological insulators and superconductors. Rev. Mod. Phys. 83, 1057–1110 (2011).
Kraus, Y. E., Lahini, Y., Ringel, Z., Verbin, M. & Zilberberg, O. Topological states and adiabatic pumping in quasicrystals. Phys. Rev. Lett. 109, 106402 (2012).
Rechtsman, M. C. et al. Photonic Floquet topological insulators. Nature 496, 196–200 (2013).
Jotzu, G. et al. Experimental realization of the topological Haldane model with ultracold fermions. Nature 515, 237–240 (2014).
Nalitov, A., Solnyshkov, D. & Malpuech, G. Polariton Z topological insulator. Phys. Rev. Lett. 114, 116401 (2015).
Lohse, M., Schweizer, C., Price, H. M., Zilberberg, O. & Bloch, I. Exploring 4D quantum Hall physics with a 2D topological charge pump. Nature 553, 55–58 (2018).
Privitera, L., Russomanno, A., Citro, R. & Santoro, G. E. Nonadiabatic breaking of topological pumping. Phys. Rev. Lett. 120, 106601 (2018).
Fedorova, Z. et al. Limits of topological protection under local periodic driving. Light. Sci. Appl. 8, 63 (2019).
Aidelsburger, M. et al. Measuring the Chern number of Hofstadter bands with ultracold bosonic atoms. Nat. Phys. 11, 162–166 (2015).
Wimmer, M., Price, H., Carusotto, I. & Peschel, U. Experimental measurement of the Berry curvature from anomalous transport. Nat. Phys. 13, 545–550 (2017).
Zilberberg, O. et al. Photonic topological boundary pumping as a probe of 4D quantum Hall physics. Nature 553, 59–62 (2018).
Wang, Y. et al. Direct observation of topology from single-photon dynamics. Phys. Rev. Lett. 122, 193903 (2019).
Niu, Q. & Thouless, D. J. Quantised adiabatic charge transport in the presence of substrate disorder and many-body interaction. J. Phys. A 17, 2453 (1984).
Lohse, M., Schweizer, C., Zilberberg, O., Aidelsburger, M. & Bloch, I. A Thouless quantum pump with ultracold bosonic atoms in an optical superlattice. Nat. Phys. 12, 350–354 (2016).
Nakajima, S. et al. Topological Thouless pumping of ultracold fermions. Nat. Phys. 12, 296–300 (2016).
Ma, W. et al. Experimental observation of a generalized Thouless pump with a single spin. Phys. Rev. Lett. 120, 120501 (2018).
Fedorova, Z., Qiu, H., Linden, S. & Kroha, J. Observation of topological transport quantization by dissipation in fast Thouless pumps. Nat. Commun. 11, 3758 (2020).
Cerjan, A., Wang, M., Huang, S., Chen, K. P. & Rechtsman, M. C. Thouless pumping in disordered photonic systems. Light. Sci. Appl. 9, 178 (2020).
Jürgensen, M., Mukherjee, S. & Rechtsman, M. C. Quantized nonlinear Thouless pumping. Nature 596, 63–67 (2021).
Ke, Y. et al. Topological phase transitions and Thouless pumping of light in photonic waveguide arrays. Laser Photon. Rev. 10, 995–1001 (2016).
Ke, Y., Qin, X., Kivshar, Y. S. & Lee, C. Multiparticle Wannier states and Thouless pumping of interacting bosons. Phys. Rev. A 95, 063630 (2017).
Kolodrubetz, M. H., Nathan, F., Gazit, S., Morimoto, T. & Moore, J. E. Topological floquet Thouless energy pump. Phys. Rev. Lett. 120, 150601 (2018).
Schnyder, A. P., Ryu, S., Furusaki, A. & Ludwig, A. W. W. Classification of topological insulators and superconductors in three spatial dimensions. Phys. Rev. B 78, 195125 (2008).
Chiu, C.-K., Teo, J. C. Y., Schnyder, A. P. & Ryu, S. Classification of topological quantum matter with symmetries. Rev. Mod. Phys. 88, 035005 (2016).
Wu, Q., Soluyanov, A. A. & Bzdušek, T. Non-Abelian band topology in noninteracting metals. Science 365, 1273–1277 (2019).
Tiwari, A. & Bzdušek, T. Non-Abelian topology of nodal-line rings in PT-symmetric systems. Phys. Rev. B 101, 195130 (2020).
Yang, E. et al. Observation of non-Abelian nodal links in photonics. Phys. Rev. Lett. 125, 033901 (2020).
Guo, Q. et al. Experimental observation of non-Abelian topological charges and edge states. Nature 594, 195–200 (2021).
Brosco, V., Pilozzi, L., Fazio, R. & Conti, C. Non-Abelian Thouless pumping in a photonic lattice. Phys. Rev. A 103, 063518 (2021).
Carolan, J. et al. Universal linear optics. Science 349, 711–716 (2015).
Imany, P. et al. High-dimensional optical quantum logic in large operational spaces. npj Quantum Inf. 5, 59 (2019).
Zhang, X. L. et al. Non-Abelian braiding on photonic chips. Nat. Photon. 16, 390–395 (2022).
Wu, S., Gao, Z., Wu, T., Zhang, Z. & Feng, L. Ultrafast heterodyne mode imaging and refractive index mapping of a femtosecond laser written multimode waveguide. Opt. Lett. 47, 214–217 (2022).
Yu, F., Zhang, X. L., Tian, Z. N., Chen, Q. D. & Sun, H. B. General rules governing the dynamical encircling of an arbitrary number of exceptional points. Phys. Rev. Lett. 127, 253901 (2021).
Boross, P., Asbóth, J. K., Széchenyi, G., Oroszlány, L. & Pályi, A. Poor man’s topological quantum gate based on the Su-Schrieffer-Heeger model. Phys. Rev. B 100, 045414 (2019).
Wu, Y., Liu, H., Liu, J., Jiang, H. & Xie, X. C. Double-frequency Aharonov-Bohm effect and non-Abelian braiding properties of Jackiw-Rebbi zero-mode. Natl Sci. Rev. 7, 572–578 (2020).
Wilczek, F. & Zee, A. Appearance of gauge structure in simple dynamical systems. Phys. Rev. Lett. 52, 2111–2114 (1984).
Chen, Z. G., Zhang, R. Y., Chan, C. T. & Ma, G. Classical non-Abelian braiding of acoustic modes. Nat. Phys. 18, 179–184 (2022).
Zhao, H. et al. Non-Hermitian topological light steering. Science 365, 1163–1166 (2019).
Shalaev, M. I., Walasik, W. & Litchinitser, N. M. Optically tunable topological photonic crystal. Optica 6, 839–844 (2019).
Yu, F. et al. Resetting directional couplers for high-fidelity quantum photonic integrated chips. Opt. Lett. 46, 5181–5184 (2021).
You, O. et al. Observation of non-Abelian Thouless pump. Phys. Rev. Lett. 128, 244302 (2022).
Acknowledgements
This work was supported by the National Natural Science Foundation of China (NSFC) under grant nos. 61590930, 11974140, 61805098, 61825502 and 61827826, as well as China Postdoctoral Science Foundation grant no. 2019M651200. X.-L.Z. thanks G. Ma for fruitful discussions.
Author information
Authors and Affiliations
Contributions
X.-L.Z. conceived the idea, performed the theoretical analysis and designed the experiment. Y.-K.S. and F.Y. carried out the experimental measurements under the supervision of Z.-N.T. and Q.-D.C. The manuscript was written by X.-L.Z. with input from all the authors. The project was supervised by H.-B.S.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Peer review
Peer review information
Nature Physics thanks Liang Feng and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Notes 1–4, Figs. 1–16, Table 1 and refs. 1–6.
Source data
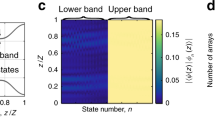
Source Data Fig. 1c–e
Source data for Fig. 1c–e.
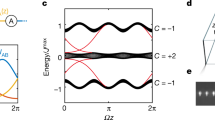
Source Data Fig. 2a,b
Source data for Fig. 2a,b.
Rights and permissions
About this article
Cite this article
Sun, YK., Zhang, XL., Yu, F. et al. Non-Abelian Thouless pumping in photonic waveguides. Nat. Phys. 18, 1080–1085 (2022). https://doi.org/10.1038/s41567-022-01669-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-022-01669-x
This article is cited by
-
Laser manufacturing of spatial resolution approaching quantum limit
Light: Science & Applications (2024)
-
Ultrafast laser-induced self-organized nanostructuring in transparent dielectrics: fundamentals and applications
PhotoniX (2023)
-
Hybrid topological photonic crystals
Nature Communications (2023)
-
Floquet non-Abelian topological insulator and multifold bulk-edge correspondence
Nature Communications (2023)
-
A second wave of topological phenomena in photonics and acoustics
Nature (2023)