Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Letter
  • Published:

Correlated insulating states at fractional fillings of the WS2/WSe2 moiré lattice

Nature Physics volume 17, pages 715–719 (2021)Cite this article

Subjects

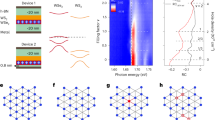
Abstract

The strong electron interactions in the minibands formed in moiré superlattices of van der Waals materials, such as twisted graphene and transition metal dichalcogenides, make such systems a fascinating platform with which to study strongly correlated states1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19. In most systems, the correlated states appear when the moiré lattice is filled by an integer number of electrons per moiré unit cell. Recently, correlated states at fractional fillings of 1/3 and 2/3 holes per moiré unit cell have been reported in the WS2/WSe2 hetero-bilayer, hinting at the long-range nature of the electron interaction16. Here we observe a series of correlated insulating states at fractional fillings of the moiré minibands on both electron- and hole-doped sides in angle-aligned WS2/WSe2 hetero-bilayers, with certain states persisting at temperatures up to 120 K. Simulations reveal that these insulating states correspond to ordering of electrons in the moiré lattice with a periodicity much larger than the moiré unit cell, indicating a surprisingly strong and long-range interaction beyond the nearest neighbours.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Spectrum of the correlated insulating states in device D1.
Fig. 2: Ordering patterns at fractional fillings of the moiré lattice.
Fig. 3: Temperature dependence of the correlated insulating states in device D1.
Fig. 4: Correlated insulating states in device D2.

Similar content being viewed by others

Data availability

Data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request. Source data are provided with this paper.

Code availability

The source code for the Monte Carlo simulations is available from the corresponding author upon reasonable request.

References

  1. Bistritzer, R. & MacDonald, A. H. Moiré bands in twisted double-layer graphene. Proc. Natl Acad. Sci. USA 108, 12233–12237 (2011).

    Article  ADS  Google Scholar 

  2. Cao, Y. et al. Correlated insulator behaviour at half-filling in magic-angle graphene superlattices. Nature 556, 80–84 (2018).

    Article  ADS  Google Scholar 

  3. Cao, Y. et al. Unconventional superconductivity in magic-angle graphene superlattices. Nature 556, 43–50 (2018).

    Article  ADS  Google Scholar 

  4. Shen, C. et al. Correlated states in twisted double bilayer graphene. Nat. Phys. 16, 520–525 (2020).

    Article  Google Scholar 

  5. Cao, Y. et al. Tunable correlated states and spin-polarized phases in twisted bilayer–bilayer graphene. Nature 583, 215–220 (2020).

    Article  ADS  Google Scholar 

  6. Liu, X. et al. Tunable spin-polarized correlated states in twisted double bilayer graphene. Nature 583, 221–225 (2020).

    Article  ADS  Google Scholar 

  7. Chen, G. et al. Evidence of a gate-tunable Mott insulator in a trilayer graphene moiré superlattice. Nat. Phys. 15, 237–241 (2019).

    Article  Google Scholar 

  8. Chen, G. et al. Signatures of gate-tunable superconductivity in trilayer graphene/boron nitride moiré superlattice. Nature 572, 215–219 (2019).

    Article  Google Scholar 

  9. Sharpe, A. L. et al. Emergent ferromagnetism near three-quarters filling in twisted bilayer graphene. Science 365, 605–608 (2019).

    Article  ADS  Google Scholar 

  10. Serlin, M. et al. Intrinsic quantized anomalous Hall effect in a moiré heterostructure. Science 367, 900–903 (2020).

    Article  ADS  Google Scholar 

  11. Chen, G. et al. Tunable correlated Chern insulator and ferromagnetism in a moiré superlattice. Nature 579, 56–61 (2020).

    Article  ADS  Google Scholar 

  12. Chen, S. et al. Electrically tunable correlated and topological states in twisted monolayer–bilayer graphene. Nat. Phys. https://doi.org/10.1038/s41567-020-01062-6 (2020).

  13. Polshyn, H. et al. Electrical switching of magnetic order in an orbital Chern insulator. Nature 588, 66–70 (2020).

    Article  ADS  Google Scholar 

  14. Shi, Y. et al. Tunable van Hove singularities and correlated states in twisted trilayer graphene. Preprint at https://arxiv.org/pdf/2004.12414.pdf (2020).

  15. Wu, F., Lovorn, T., Tutuc, E. & MacDonald, A. H. H. Hubbard model physics in transition metal dichalcogenide moiré bands. Phys. Rev. Lett. 121, 26402 (2018).

    Article  ADS  Google Scholar 

  16. Regan, E. C. et al. Mott and generalized Wigner crystal states in WSe2/WS2 moiré superlattices. Nature 579, 359–363 (2020).

    Article  ADS  Google Scholar 

  17. Tang, Y. et al. Simulation of Hubbard model physics in WSe2/WS2 moiré superlattices. Nature 579, 353–358 (2020).

    Article  ADS  Google Scholar 

  18. Shimazaki, Y. et al. Strongly correlated electrons and hybrid excitons in a moiré heterostructure. Nature 580, 472–477 (2020).

    Article  ADS  Google Scholar 

  19. Wang, L. et al. Correlated electronic phases in twisted bilayer transition metal dichalcogenides. Nat. Mater. 19, 861–866 (2020).

    Article  Google Scholar 

  20. Mott, N. F. The basis of the electron theory of metals, with special reference to the transition metals. Proc. Phys. Soc. A 62, 416–422 (1949).

    Article  ADS  Google Scholar 

  21. Imada, M., Fujimori, A. & Tokura, Y. Metal–insulator transitions. Rev. Mod. Phys. 70, 1039–1263 (1998).

    Article  ADS  Google Scholar 

  22. Sheng, D. N., Gu, Z.-C., Sun, K. & Sheng, L. Fractional quantum Hall effect in the absence of Landau levels. Nat. Commun. 2, 389 (2011).

    Article  ADS  Google Scholar 

  23. Grüner, G. The dynamics of charge-density waves. Rev. Mod. Phys. 60, 1129–1181 (1988).

    Article  ADS  Google Scholar 

  24. Emery, V. J., Kivelson, S. A. & Tranquada, J. M. Stripe phases in high-temperature superconductors. Proc. Natl Acad. Sci. USA 96, 8814–8817 (1999).

    Article  ADS  Google Scholar 

  25. Hoffman, J. E. A four unit cell periodic pattern of quasi-particle states surrounding vortex cores in Bi2Sr2CaCu2O8 + δ. Science 295, 466–469 (2002).

    Article  ADS  Google Scholar 

  26. Wigner, E. On the interaction of electrons in metals. Phys. Rev. 46, 1002–1011 (1934).

    Article  ADS  Google Scholar 

  27. Hubbard, J. Generalized Wigner lattices in one dimension and some applications to tetracyanoquinodimethane (TCNQ) salts. Phys. Rev. B 17, 494–505 (1978).

    Article  ADS  Google Scholar 

  28. Grimes, C. C. & Adams, G. Evidence for a liquid-to-crystal phase transition in a classical, two-dimensional sheet of electrons. Phys. Rev. Lett. 42, 795–798 (1979).

    Article  ADS  Google Scholar 

  29. Andrei, E. Y. et al. Observation of a magnetically induced Wigner solid. Phys. Rev. Lett. 60, 2765–2768 (1988).

    Article  ADS  Google Scholar 

  30. Shapir, I. et al. Imaging the electronic Wigner crystal in one dimension. Science 364, 870–875 (2019).

    Article  ADS  Google Scholar 

  31. Xu, Y. et al. Correlated insulating states at fractional fillings of moiré superlattices. Nature 587, 214–218 (2020).

    Article  ADS  Google Scholar 

  32. Jin, C. et al. Stripe phases in WSe2/WS2 moiré superlattices. Preprint at https://arxiv.org/pdf/2007.12068.pdf (2020).

  33. Jin, C. et al. Observation of moiré excitons in WSe2/WS2 heterostructure superlattices. Nature 567, 76–80 (2019).

    Article  ADS  Google Scholar 

  34. Zhang, Z. et al. Flat bands in twisted bilayer transition metal dichalcogenides. Nat. Phys. 16, 1093–1096 (2020).

    Article  Google Scholar 

  35. Lai, K., Kundhikanjana, W., Kelly, M. & Shen, Z. X. Modeling and characterization of a cantilever-based near-field scanning microwave impedance microscope. Rev. Sci. Instrum. 79, 063703 (2008).

    Article  ADS  Google Scholar 

  36. Cui, Y.-T., Ma, E. Y. & Shen, Z.-X. Quartz tuning fork based microwave impedance microscopy. Rev. Sci. Instrum. 87, 063711 (2016).

    Article  ADS  Google Scholar 

  37. Chu, Z. et al. Nanoscale conductivity imaging of correlated electronic states in WSe2/WS2 moiré superlattices. Phys. Rev. Lett. 125, 186803 (2020).

    Article  ADS  Google Scholar 

  38. Li, Z. et al. Revealing the biexciton and trion–exciton complexes in BN encapsulated WSe2. Nat. Commun. 9, 3719 (2018).

    Article  ADS  Google Scholar 

  39. Rademaker, L., Pramudya, Y., Zaanen, J. & Dobrosavljević, V. Influence of long-range interactions on charge ordering phenomena on a square lattice. Phys. Rev. E 88, 032121 (2013).

    Article  ADS  Google Scholar 

  40. Swendsen, R. H. & Wang, J.-S. Replica Monte Carlo simulation of spin-glasses. Phys. Rev. Lett. 57, 2607–2609 (1986).

    Article  ADS  MathSciNet  Google Scholar 

  41. Toukmaji, A. Y. & Board, J. A. Ewald summation techniques in perspective: a survey. Comput. Phys. Commun. 95, 73–92 (1996).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

We thank D. Chen, L. Yan, L. Ma and K. Li for help with device fabrication. We are grateful to R. Swendsen and M. Widom for their help with the Monte Carlo simulation. C.W. and D.X. thank W. Duan for providing part of the computational resources. X.H. and Y.-T.C. acknowledge support from the NSF under award no. DMR-2004701, a Hellman Fellowship award and a seed fund from SHINES, an EFRC funded by the US Department of Energy (DOE), Basic Energy Sciences (BES) under award no. SC0012670. S.M., Z. Li and S.-F.S. acknowledge support by AFOSR through grant no. FA9550-18-1-0312. T.W. and S.-F.S. acknowledge support from ACS PRF through grant no. 59957-DNI10. Z. Lian and S.-F.S. acknowledge support from NYSTAR through Focus Center-NY–RPI contract C150117. Device fabrication was supported by the Micro and Nanofabrication Clean Room (MNCR) at Rensselaer Polytechnic Institute (RPI). S.-F.S. also acknowledges support from the NSF through Career grant no. DMR-1945420. The research by S.O. is supported by the US Department of Energy, Office of Science, Basic Energy Sciences, Materials Sciences and Engineering Division. C.W. and D.X. acknowledge support from DOE, BES grant no. DE-SC0012509. K.W. and T.T. acknowledge support from the Elemental Strategy Initiative conducted by the MEXT (Japan; grant no. JPMXP0112101001), JSPS (KAKENHI grant no. JP20H00354) and the CREST (JPMJCR15F3), JST. We acknowledge computing time provided by BRIDGES at the Pittsburgh Supercomputing Center (award no. TG-DMR190080) under the Extreme Science and Engineering Discovery Environment (XSEDE) supported by the NSF (ACI-1548562).

Author information

Author notes

  1. These authors contributed equally: Xiong Huang, Tianmeng Wang, Shengnan Miao, Chong Wang.

Authors and Affiliations

  1. Department of Physics and Astronomy, University of California, Riverside, CA, USA

    Xiong Huang & Yong-Tao Cui

  2. Department of Materials Science and Engineering, University of California, Riverside, CA, USA

    Xiong Huang

  3. Department of Chemical and Biological Engineering, Rensselaer Polytechnic Institute, Troy, NY, USA

    Tianmeng Wang, Shengnan Miao, Zhipeng Li, Zhen Lian & Su-Fei Shi

  4. Department of Physics, Carnegie Mellon University, Pittsburgh, PA, USA

    Chong Wang & Di Xiao

  5. International Center for Materials Nanoarchitectonics, National Institute for Materials Science, Tsukuba, Japan

    Takashi Taniguchi

  6. Research Center for Functional Materials, National Institute for Materials Science, Tsukuba, Japan

    Kenji Watanabe

  7. Materials Science and Technology Division, Oak Ridge National Laboratory, Oak Ridge, TN, USA

    Satoshi Okamoto

  8. Department of Electrical, Computer and Systems Engineering, Rensselaer Polytechnic Institute, Troy, NY, USA

    Su-Fei Shi

Authors
  1. Xiong Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tianmeng Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Shengnan Miao
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Chong Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Zhipeng Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Zhen Lian
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Takashi Taniguchi
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Kenji Watanabe
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Satoshi Okamoto
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. Di Xiao
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Su-Fei Shi
    View author publications

    You can also search for this author in PubMed Google Scholar

  12. Yong-Tao Cui
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

S.-F.S. and Y.-T.C. initiated the research. T.W., S.M., Z. Li and Z. Lian fabricated the heterostructure devices. X.H. performed the MIM measurements. Y.-T.C., S.-F.S., D.X., S.M., T.W., C.W. and X.H. analysed the data. C.W., S.O. and D.X. performed numerical simulations. Y.-T.C., S.-F.S. and D.X. wrote the manuscript with input from all authors.

Corresponding authors

Correspondence to Di Xiao, Su-Fei Shi or Yong-Tao Cui.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Physics thanks Fengcheng Wu and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Extended data

Extended Data Fig. 1 Sample information.

a,b Optical microscope images of devices (a) D1 and (b) D2 with different flakes outlined. c,d Angular dependence of the SHG signal in (c) device D1 and (d) device D2.

Extended Data Fig. 2 Additional MIM data in device D1.

a, MIM-Im vs gate voltage traces for both sweeping directions in device D1 at T = 4 K. b, MIM-Im vs gate voltage traces with extended gate range from −7 V to 6 V taken at T = 3 K. The feature near −5 V likely correponds to n = −2 state. However, due to poor electrical contact at high hole doping, this feature is not stable and its gate position is not repeatable at different spots.

Extended Data Fig. 3 Spatial uniformity of sample conductivity in device D1.

a,b Optical image and atomic force microscopy (AFM) image of device D1. c, MIM-Im image at Vg = −2 V scanned over the region marked by the rectangles in (a) and (b). d, MIM-Im images taken at different gate voltages marked along the top axis in (e). e, MIM-Im vs gate traces taken at spots A-F as indicated in (c). All MIM data are taken at T = 10 K. Scale bars are 2 μm.

Extended Data Fig. 4 Ordering patterns simulated on a 48 by 48 lattice for n = 1/3, 1/4, 1/7, and 1/9.

The column on the right plots the fast Fourier transform (FFT) image of the ordering pattern.

Extended Data Fig. 5 Ordering patterns simulated on a 48 by 48 lattice for n = 1/2, 1/6, 4/9, and 2/9.

The column on the right plots the FFT image of the ordering pattern.

Extended Data Fig. 6 Simulated specific heat for different fillings.

The temperature scale, kBT, is normalized by the nearest neighbor Coulomb interaction. The identified ordering temperatures, kBTc, are listed in the figure.

Extended Data Fig. 7 The Fourier transformed configurations in the reciprocal space as kBT varies for filling 1/7.

The lowest kBT is 0.017; the highest kBT is 0.031; kBTc = 0.021 is labeled in the picture. To visualize the transition around the critical temperature, we select eight configurations from the simulations of 1/7 filling, on which we perform Fourier transformations. The absolute values of the eight Fourier amplitudes are added and plotted in the reciprocal space. It is clear the Fourier amplitudes changes abruptly around Tc, which justifies our approach of identifying the peak in the specific heat as the transition temperature.

Extended Data Fig. 8 Temperature dependence of MIM-Im for gate sweeping down from 5 V to -5 V in device D2.

The black markers indicate the positions of the n = −1/3 state, which can be resolved at temperatures of 20 K and above.

Extended Data Fig. 9 Spatial variation and hysteresis of the MIM-Im spectrum in device D2.

a, Optical microscope of D2. b, AFM scan around the area indicated by the solid square in (a). The scale bar is 5 μm. c, MIM spectra taken at spots A-E as marked in (b). d, Sweep up and down traces at spot B. e, Sweep up and down traces at spot E. All data taken at T = 2.8 K.

Source data

Source Data Fig. 1

Data for Fig. 1c–e.

Source Data Fig. 3

Data for Fig. 3, all panels.

Source Data Fig. 4

Data for Fig. 4a,c.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Huang, X., Wang, T., Miao, S. et al. Correlated insulating states at fractional fillings of the WS2/WSe2 moiré lattice. Nat. Phys. 17, 715–719 (2021). https://doi.org/10.1038/s41567-021-01171-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-021-01171-w

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing