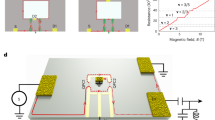
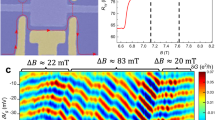
Abstract
Anyons are quasiparticles that, unlike fermions and bosons, show fractional statistics when two of them are exchanged. Here, we report the experimental observation of anyonic braiding statistics for the ν = 1/3 fractional quantum Hall state by using an electronic Fabry–Perot interferometer. Strong Aharonov–Bohm interference of the edge mode is punctuated by discrete phase slips that indicate an anyonic phase θanyon = 2π/3. Our results are consistent with a recent theory that describes an interferometer operated in a regime in which device charging energy is small compared to the energy of formation of charged quasiparticles, which indicates that we have observed anyonic braiding.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
Source data are available for this paper. All other data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.
References
Leinaas, J. M. & Myrheim, J. On the theory of identical particles. Nuovo Ciment. B 37, 1–23 (1977).
Wilczek, F. Quantum mechanics of fractional-spin particles. Phys. Rev. Lett. 49, 957–959 (1982).
Tsui, D. C., Stormer, H. L. & Gossard, A. C. Two-dimensional magnetotransport in the extreme quantum limit. Phys. Rev. Lett. 48, 1559–1562 (1982).
Laughlin, R. B. Anomolous quantum Hall effect: An incompressible quantum fluid with fractionally charged excitation. Phys. Rev. Lett. 50, 1395–1398 (1983).
Nayak, C., Simon, S. H., Stern, A., Freedman, M. & Das Sarma, S. Non-Abelian anyons and topological quantum computation. Rev. Mod. Phys. 80, 1083–1159 (2008).
Arovas, D., Schrieffer, J. R. & Wilczek, F. Fractional statistics and the quantum Hall effect. Phys. Rev. Lett. 53, 722–723 (1984).
Halperin, B. I. Statistics of quasiparticles and the hierarchy of fractional quantized Hall states. Phys. Rev. Lett. 52, 1583–1586 (1984).
Kjonsberg, H. & Leinaas, J. M. Charge and statistics of quantum Hall quasi-particles – numerical study of mean values and fluctuations. Nucl. Phys. B 559, 705–742 (1999).
Jeon, G. S., Graham, K. L. & Jain, J. K. Fractional statistics in the fractional quantum Hall effect. Phys. Rev. Lett. 91, 036801 (2003).
Jain, J. K. Composite Fermions (Cambridge Univ. Press, 2007).
Das Sarma, S., Freedman, M. & Nayak, C. Topologically protected qubits from a possible non-Abelian fractional quantum Hall state. Phys. Rev. Lett. 94, 166802 (2005).
Stern, A. & Lindner, N. H. Topological quantum computation – from basic concepts to first experiments. Science 339, 1179–1184 (2013).
Bartolomei, H. et al. Fractional statistics in anyon collisions. Science 368, 173–177 (2020).
Rosenow, B. & Stern, A. Flux superperiods and periodicity transitions in quantum Hall interferometers. Phys. Rev. Lett. 124, 106805 (2020).
Chamon, C. de C., Freed, D. E., Kivelson, S. A., Sondhi, S. L. & Wen, X. G. Two point-contact interferometer for quantum Hall systems. Phys. Rev. B 55, 2331–2342 (1997).
Halperin, B. I. & Rosenow, B. Influence of interactions on flux and back-gate period of quantum Hall interferometers. Phys. Rev. Lett. 98, 106801 (2007).
Halperin, B. I., Stern, A., Neder, I. & Rosenow, B. Theory of the Fabry–Perot quantum Hall interferometer. Phys. Rev. B 83, 155440 (2011).
Rosenow, B. & Simon, S. H. Telegraph noise and the Fabry–Perot quantum Hall interferometer. Phys. Rev. B 85, 201302 (2012).
Levkivskyi, I. P., Frohlich, J. & Sukhorukov, E. B. Theory of fractional quantum Hall interferometers. Phys. Rev. B 86, 245105 (2012).
von Keyserlingk, C. W., Simon, S. H. & Rosenow, B. Enhanced bulk–edge Coulomb coupling in fractional Fabry–Perot interferometers. Phys. Rev. Lett. 115, 126807 (2015).
Goldstein, M. & Gefen, Y. Suppression of interference in quantum Hall Mach–Zehnder geometry by upstream neutral modes. Phys. Rev. Lett. 117, 276804 (2016).
Litvin, L. V., Helzel, A., Tranitz, H. -P., Wegscheider, W. & Strunk, C. Edge–channel interference controlled by Landau level filling. Phys. Rev. B. 78, 075303 (2008).
Deviatov, E. V. & Lorke, A. Experimental realization of a Fabry–Perot type interferometer by copropogating edge states in the quantum Hall regime. Phys. Rev. B 77, 161302 (2008).
Zhang, Y. et al. Distinct signatures for Coulomb blockade and interference in electronic Fabry–Perot interferometers. Phys. Rev. B 79, 241304 (2009).
McClure, D. T. et al. Edge-state velocity and coherence in a quantum Hall Fabry–Perot interferometer. Phys. Rev. Lett. 103, 206806 (2009).
Lin, P. V., Camino, F. E. & Goldman, V. J. Electron interferometry in the quantum Hall regime: Aharonov–Bohm effect of interacting electrons. Phys. Rev. B 80, 125310 (2009).
An, S., et al., Braiding of Abelian and non-Abelian anyons in the fractional quantum Hall effect. Preprint at https://arxiv.org/abs/1112.3400 (2011).
Ofek, N. et al. Role of interactions in an electron Fabry–Perot interferometer operating in the quantum Hall effect regime. Proc. Natl Acad. Sci. USA 107, 5276–5281 (2010).
Choi, H. K. et al. Robust electron pairing in the integer quantum Hall effect. Nat. Comm. 6, 7435 (2015).
Gurman, I., Sabo, R., Heiblum, M., Umansky, V. & Mahalu, D. Dephasing of an electronic two-path interferometer. Phys. Rev. B 93, 121412 (2016).
Sivan, I. et al. Observation of interaction-induced modulations of a quantum Hall liquid’s area. Nat. Comm. 7, 12184 (2016).
Sabo, R. et al. Edge reconstruction in fractional quantum Hall states. Nat. Phys. 13, 491 (2017).
Duprez, H. et al. Macroscopic electron quantum coherence in a solid-state circuit. Phys. Rev. X 9, 021030 (2019).
Bhattacharyya, R., Mitali, B., Heiblum, M., Mahalu, D. & Umansky, V. Melting of interference in the fractional quantum Hall effect: appearance of neutral modes. Phys. Rev. Lett. 122, 246801 (2019).
Roosli, M. P. et al. Observation of quantum Hall interferometer phase jumps due to a change in the number of localized bulk quasiparticles. Phys. Rev. B 101, 125302 (2020).
Kivelson, S. Semiclassical theory of localized many-anyon states. Phys. Rev. Lett. 65, 3369 (1990).
Kim, E. Aharonov–Bohm interference and fractional statistics in a quantum Hall interferometer. Phys. Rev. Lett. 97, 216404 (2006).
Stern, A. & Halperin, B. I. Proposed experiments to probe the non-Abelian ν = 5/2 quantum Hall state. Phys. Rev. Lett. 96, 016802 (2006).
Bonderson, P., Kitaev, A. & Shtengel, K. Detecting non-Abelian statistics in the ν = 5/2 fractional quantum Hall state. Phys. Rev. Lett. 96, 016803 (2006).
Bishara, W., Bonderson, P., Nayak, C., Shtengel, K. & Slingerland, J. K. Interferometric signature of non-Abelian anyons. Phys. Rev. B 80, 155303 (2009).
Willett, R. L., Pfeiffer, L. N. & West, K. W. Measurement of filling factor 5/2 quasiparticle interference with observation of charge e/4 and e/2 period oscillations. Proc. Natl Acad. Sci. USA 106, 8853–8858 (2009).
Stern, A., Rosenow, B., Ilan, R. & Halperin, B. I. Interference, Coulomb blockade, and the identification of non-Abelian quantum Hall states. Phys. Rev. B 82, 085321 (2010).
Willett, R. L., Nayak, C., Shtengel, K., Pfeiffer, L. N. & West, K. W. Magnetic-field-tuned Aharonov–Bohm oscillations and evidence for non-Abelian anyons at ν = 5/2. Phys. Rev. Lett. 111, 186401 (2013).
Willett, R.L., et al., Interference measurements of non-Abelian e/4 & Abelian e/2 quasiparticle braiding. Preprint at https://arxiv.org/abs/1905.10248v1 (2019).
McClure, D. T., Chang, W., Marcus, C. M., Pfeiffer, L. N. & West, K. W. Fabry–Perot interferometry with fractional charges. Phys. Rev. Lett. 108, 256804 (2012).
Nakamura, J. et al. Aharonov–Bohm interference of fractional quantum Hall edge modes. Nat. Phys. 15, 563–569 (2019).
Halperin, B. I. Quantized Hall conductance, current-carrying edge states, and the existence of extended states in a two-dimensional disordered potential. Phys. Rev. B. 25, 2185–2190 (1982).
Rezayi, E. H. & Haldane, F. D. M. Incompressible states of the fractionally quantized Hall effect in the presence of impurities: a finite-size study. Phys. Rev. B. 32, 6924–6927 (1985).
MacDonald, A. H., Liu, K. L., Girvin, S. M. & Platzman, P. M. Disorder and the fractional quantum Hall effect: activation energies and the collapse of the gap. Phys. Rev. B. 33, 4014–4020 (1986).
Goldman, V. J. & Su, B. Resonant tunneling in the quantum Hall regime: measurement of fractional charge. Science 267, 1010–1012 (1995).
de-Picciotto, R. et al. Direct observation of a fractional charge. Nature 389, 162–164 (1997).
Saminadayar, L., Glattli, D. C., Lin, Y. & Etienne, B. Observation of the e/3 fractionally charged Laughlin quasiparticle. Phys. Rev. Lett. 79, 2526–2529 (1997).
Du, R. R., Stormer, H. L., Tsui, D. C., Pfeiffer, L. N. & West, K. W. Experimental evidence for new particles in the fractional quantum Hall effect. Phys. Rev. Lett. 70, 2944–2947 (1993).
Hu, Z., Rezayi, E. H., Wan, X. & Yang, K. Edge-mode velocities and thermal coherence of quantum Hall interferometers. Phys. Rev. B 80, 235330 (2009).
Park, J., Gefen, Y. & Sim, H. Topological dephasing in the ν = 2/3 fractional quantum Hall regime. Phys. Rev. B 92, 245437 (2015).
Bid, A. et al. Observation of neutral modes in the fractional quantum Hall regime. Nature 466, 585–590 (2010).
Inoue, H. et al. Proliferation of neutral modes in fractional quantum Hall states. Nat. Comm. 5, 4067 (2014).
Manfra, M. J. Molecular beam epitaxy of ultra-high-quality AlGaAs/GaAs heterostructures: enabling physics in low-dimensional electronic systems. Annu. Rev. Condens. Matter Phys. 5, 347–373 (2014).
Gardner, G. C., Fallahi, S., Watson, J. D. & Manfra, M. J. Modified MBE hardware and techniques and role of gallium purity for attainment of two dimensional electron gas mobility > 35 × 106 cm2/Vs in AlGaAs/GaAs quantum wells grown by MBE. J. Cryst. Growth 441, 71–77 (2016).
Sahasrabudhe, H. et al. Optimization of edge state velocity in the integer quantum Hall regime. Phys. Rev. B 97, 085302 (2018).
Jeon, G. S., Graham, K. L. & Jain, J. K. Berry phases for composite fermions: effective magnetic field and fractional statistics. Phys. Rev. B 70, 125316 (2004).
Acknowledgements
This work is supported by the US Department of Energy, Office of Science and Office of Basic Energy Sciences (award no. DE-SC0020138). G.C.G. acknowledges support from Microsoft Quantum. We thank B. Rosenow for valuable comments on an early version of this manuscript.
Author information
Authors and Affiliations
Contributions
J.N. and M.J.M. designed the heterostructures and experiments. S.L. and G.C.G. conducted the molecular beam epitaxy growth. J.N. fabricated the devices, performed the measurements and analysed the data with input from M.J.M. J.N. and M.J.M wrote the manuscript with input from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data
Extended Data Fig. 1 Layer stack of the GaAs/AlGaAs heterostructure used for the experiments.
This structure utilizes three GaAs quantum wells: a primary 30nm well flanked by two 13nm screening wells to reduce the bulk-edge interaction in the interferometer. There are 25nm AlGaAs barriers between the main well and screening wells, and the total center-to-center setback of the screening wells from the main well is 48nm.
Extended Data Fig. 2 Repeatability of discrete phase jumps.
a, First scan measurement of conductance versus B and δVg. This is the same data in Fig. 2 of the main text. b, Second scan across the same range of magnetic field using the same QPC gate voltages. As can be seen from the data, the same pattern of discrete jumps appear in the second scan. The second scan was taken approximately one hour after the first scan. Values of Δθ/2π extracted from least squares fits are shown for both scans, and show similar values for each phase jump in both scans.
Extended Data Fig. 3 Measurement of the energy gap for the ν= 1/3 fractional quantum Hall state.
The inset shows longitudinal resistance Rxx measured in a bulk region away from the interferometer at different temperatures. A linear fit of the data yields a gap of Δ = 5.5K. This is consistent with values measured in previous experiments at similar magnetic field.
Extended Data Fig. 4 Conductance oscillations at different magnetic fields.
a, Conductance oscillations δG versus side gate voltage δVg in the low-field region at B = 8.4T (blue), in the central region at B = 8.85T (black), and in the high-field region at B = 9.3T (red). The side gate oscillation period δVg is significantly smaller in the low field and high field regions than in the central region, with δVg = 5.8mV at 8.4T, δVg = 8.5mV at 8.85T, and δVg = 5.4mV at 9.3T. The QPCs are tuned to approximately 90% transmission. b, Conductance G versus side gate voltage at zero magnetic field with the device operated in the Coulomb blockade regime. Unlike other data presented in this work, the oscillations shown here are due to resonant tunneling of electrons rather than interference, and the QPCs are tuned weak tunneling, G << e2/h. The Coulomb blockade oscillations have a period of 5.3mV, which is used to obtain the total lever arm αtotal of the gates to the interferometer. c, Aharonov-Bohm interference oscillations at ν = 1. The oscillations period of 8.0mV is used to obtain the lever arm αedge of the gates to the edge.
Extended Data Fig. 5 Simulations of interferometer behavior at ν = 1/3.
Conductance values are computed as a function of magnetic field B and side gate voltages δVg, taking into account both the Aharonov-Bohm phase and the contribution θanyon from braiding around localized quasiparticles inside the bulk of the interferometer. Simulations are performed at different ratios of the temperature kBT to the interferometer charging energy Ec = e2/2C a) 0.002 b) 0.02 and c) 0.1. d, Plot of the thermal expectation value of the number of localized quasiparticles inside the interferometer for different ratios of kBT/Ec; in this context a negative quasiparticle number indicates a population of quasiholes. In each case in the middle of the state there are no quasiparticles, resulting in conventional Aharonov-Bohm interference with 3Φ0 period, while at higher fields quasiholes form and at lower fields quasiparticles form, resulting in phase slips with Φ0 period. As temperature is elevated, the quasiparticle number is thermally smeared, making the Φ0 period phase slips unobservable and reducing the amplitude of the oscillations that occur as a function of δVg. e, Qualitative plot of the density of states versus energy.
Extended Data Fig. 6 Measurements of interference at ν = 1.
a, Bulk quantum Hall transport showing the zero in Rxx and plateau in Rxy corresponding to the ν = 1 integer quantum Hall state. For this integer state, the bulk excitations and edge state current carrying particles are simply electrons, which obey fermionic statistics. b, Conductance oscillations versus magnetic field, showing an oscillation period ΔB =11mT. From this period the effective area AI of the interferometer can be extracted: AI = Φ0 ΔB. In c), d), and e) we show conductance versus B and δVg across the interferometer in the low field region of the plateau, near the center of the plateau, and on the high-field side of the plateau; the region on the plateau corresponding to each pajama plot is shown in a). In each of these regions the device exhibits negatively sloped Aharonov-Bohm oscillations. This contrasts with the data shown in the main text for the ν = 1/3 state where lines of constant phase flatten out at high and low fields. This is consistent with the fact that electrons, which carry current and form localized states at ν = 1, are fermions who obey trivial braiding statistics, θfermion = 2π, making braiding unobservable and leading to no change in interference behavior.
Extended Data Fig. 7 Differential conductance measurements at ν = 1/3.
a, Differential conductance ∂I/∂Vsd as a function of side gate voltage δVg and source-drain bias Vsd at B = 8.4T in the low-field region. b, Conductance oscillation amplitude from a Fourier transform of the conductance versus side gate voltage data as a function of Vsd. The oscillation amplitude shows a node pattern as a function of Vsd from which the edge velocity may be extracted, yielding vedge = 8.3 × 103m/s. c, Differential conductance and d) oscillation amplitude versus Vsd at 8.85T giving vedge = 9.7 × 103m/s. e, Differential conductance and f) oscillation amplitude versus Vsd at 9.3T giving vedge = 9.3 × 103m/s. Evidently, the edge velocity does not change significantly across the ν = 1/3 quantum Hall plateau.
Extended Data Fig. 8 Measurements of interference for a second device, taken from a different chip fabricated on the same wafer.
a, Conductance across the interferometer versus magnetic field B and side gate voltage δVg; δVg is relative to -1.0V. Behavior is similar to that observed in the device described in the main text: in a finite region with width ≈ 430mT, the device exhibits negatively sloped Aharonov-Bohm oscillations, which flatten out at higher and lower magnetic fields, consistent with the creation of quasipaticles and quasiholes. b, Bulk magnetotransport showing Rxx (red) and Rxy (blue) for device B. The region near the center of the ν = 1/3 state where the negatively sloped Aharonov-Bohm oscillations occur is highlighted. c, zoomed-in view of a clear phase jump in the data (this jump is also visible in b), but the data in c) is a different scan intended to improve signal to noise). Least-squares fits of the conductance on either side of the phase jump yields an extracted phase jump Δθ/2π = -0.32, yielding an anyonic phase θanyon = 2π × 0.32, consistent with theory.
Supplementary information
Supplementary Information
Supplementary Discussion 1–4.
Source data
Source Data Fig. 2
Numerical source data.
Source Data Fig. 3
Numerical source data.
Source Data Fig. 4
Numerical source data.
Source Data Extended Data Fig. 2
Numerical source data.
Source Data Extended Data Fig. 3
Numerical source data.
Source Data Extended Data Fig. 4
Numerical source data.
Source Data Extended Data Fig. 5
Numerical source data.
Source Data Extended Data Fig. 6
Numerical source data.
Source Data Extended Data Fig. 7
Numerical source data.
Source Data Extended Data Fig. 8
Numerical source data.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Nakamura, J., Liang, S., Gardner, G.C. et al. Direct observation of anyonic braiding statistics. Nat. Phys. 16, 931–936 (2020). https://doi.org/10.1038/s41567-020-1019-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-020-1019-1
This article is cited by
-
Non-Abelian topological order and anyons on a trapped-ion processor
Nature (2024)
-
Clarification of braiding statistics in Fabry–Perot interferometry
Nature Physics (2024)
-
Evidence for Topological Protection Derived from Six-Flux Composite Fermions
Nature Communications (2024)
-
Fractional quantum anomalous Hall effect in multilayer graphene
Nature (2024)
-
Measuring statistics-induced entanglement entropy with a Hong–Ou–Mandel interferometer
Nature Communications (2024)