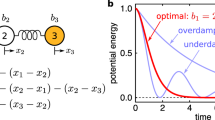
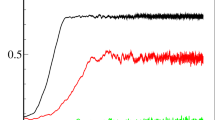
Abstract
Symmetry breaking—the phenomenon in which the symmetry of a system is not inherited by its stable states—underlies pattern formation, superconductivity and numerous other effects. Recent theoretical work has established the possibility of converse symmetry breaking, a phenomenon in which the stable states are symmetric only when the system itself is not. This includes scenarios in which interacting entities are required to be non-identical in order to exhibit identical behaviour, such as in reaching consensus. Here we present an experimental demonstration of this phenomenon. Using a network of alternating-current electromechanical oscillators, we show that their ability to achieve identical frequency synchronization is enhanced when the oscillators are tuned to be suitably non-identical and that converse symmetry breaking persists for a range of noise levels. These results have implications for the optimization and control of network dynamics in a broad class of systems whose function benefits from harnessing uniform behaviour.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
Code availability
The custom code used for the analysis of the data from the experiment is available from the corresponding author upon reasonable request.
References
Pikovsky, A., Rosenblum, M. & Kurths, J. Synchronization: A Universal Concept in Nonlinear Sciences (Cambridge Univ. Press, 2001).
Arenas, A., Díaz-Guilera, A., Kurths, J., Moreno, Y. & Zhou, C. Synchronization in complex networks. Phys. Rep. 469, 93–153 (2008).
Dörfler, F. & Bullo, F. Synchronization in complex networks of phase oscillators: a survey. Automatica 50, 1539–1564 (2014).
Pecora, L. M. & Carroll, T. L. Synchronization of chaotic systems. Chaos 25, 097611 (2015).
Yamaguchi, S. et al. Synchronization of cellular clocks in the suprachiasmatic nucleus. Science 302, 1408–1412 (2003).
Yamaguchi, Y. et al. Mice genetically deficient in vasopressin V1a and V1b receptors are resistant to jet lag. Science 342, 85–90 (2013).
Lu, Z. et al. Resynchronization of circadian oscillators and the east–west asymmetry of jet-lag. Chaos 26, 094811 (2016).
Ranta, E., Kaitala, V., Lindström, J. & Linden, H. Synchrony in population dynamics. Proc. R. Soc. Lond. B 262, 113–118 (1995).
Schwartz, M. K., Mills, L. S., McKelvey, K. S., Ruggiero, L. F. & Allendorf, F. W. DNA reveals high dispersal synchronizing the population dynamics of Canada lynx. Nature 415, 520–522 (2002).
McClintock, M. K. Menstrual synchrony and suppression. Nature 229, 244–245 (1971).
Strogatz, S. H., Abrams, D. M., McRobie, A., Eckhardt, B. & Ott, E. Theoretical mechanics: crowd synchrony on the Millennium Bridge. Nature 438, 43–44 (2005).
Rosin, D. P., Rontani, D., Gauthier, D. J. & Schöll, E. Control of synchronization patterns in neural-like Boolean networks. Phys. Rev. Lett. 110, 104102 (2013).
Fischer, I. et al. Zero-lag long-range synchronization via dynamical relaying. Phys. Rev. Lett. 97, 123902 (2006).
Zamora-Munt, J., Masoller, C., García-Ojalvo, J. & Roy, R. Crowd synchrony and quorum sensing in delay-coupled lasers. Phys. Rev. Lett. 105, 264101 (2010).
Argyris, A., Bourmpos, M. & Syvridis, D. Experimental synchrony of semiconductor lasers in coupled networks. Opt. Express 24, 5600–5614 (2016).
Kiss, I. Z., Zhai, Y. & Hudson, J. L. Emerging coherence in a population of chemical oscillators. Science 296, 1676–1678 (2002).
Kiss, I. Z., Rusin, C. G., Kori, H. & Hudson, J. L. Engineering complex dynamical structures: sequential patterns and desynchronization. Science 316, 1886–1889 (2007).
Fon, W. et al. Complex dynamical networks constructed with fully controllable nonlinear nanomechanical oscillators. Nano Lett. 17, 5977–5983 (2017).
Hill, D. J. & Chen, G. Power systems as dynamic networks. In Proceedings of the 2006 IEEE International Symposium on Circuits and Systems 722–725 (IEEE, 2006).
Motter, A. E., Myers, S. A., Anghel, M. & Nishikawa, T. Spontaneous synchrony in power-grid networks. Nat. Phys. 9, 191–197 (2013).
Dörfler, F., Chertkov, M. & Bullo, F. Synchronization in complex oscillator networks and smart grids. Proc. Natl Acad. Sci. USA 110, 2005–2010 (2013).
Nishikawa, T. & Motter, A. E. Symmetric states requiring system asymmetry. Phys. Rev. Lett. 117, 114101 (2016).
Okuda, K. & Kuramoto, Y. Mutual entrainment between populations of coupled oscillators. Prog. Theor. Phys. 86, 1159–1176 (1991).
Golubitsky, M., Stewart, I. & Schaeffer, D. G. Singularities and Groups in Bifurcation Theory Vol. 2 (Springer, 1988).
Golubitsky, M. & Stewart, I. Symmetry and pattern formation in coupled cell networks. In Pattern Formation in Continuous and Coupled Systems 65–82 (Springer, 1999).
Nicosia, V., Valencia, M., Chavez, M., Díaz-Guilera, A. & Latora, V. Remote synchronization reveals network symmetries and functional modules. Phys. Rev. Lett. 110, 174102 (2013).
Pecora, L. M., Sorrentino, F., Hagerstrom, A. M., Murphy, T. E. & Roy, R. Cluster synchronization and isolated desynchronization in complex networks with symmetries. Nat. Commun. 5, 4079 (2014).
Whalen, A. J., Brennan, S. N., Sauer, T. D. & Schiff, S. J. Observability and controllability of nonlinear networks: the role of symmetry. Phys. Rev. X 5, 011005 (2015).
Sorrentino, F., Pecora, L. M., Hagerstrom, A. M., Murphy, T. E. & Roy, R. Complete characterization of the stability of cluster synchronization in complex dynamical networks. Sci. Adv. 2, e1501737 (2016).
Zhang, L., Motter, A. E. & Nishikawa, T. Incoherence-mediated remote synchronization. Phys. Rev. Lett. 118, 174102 (2017).
Cho, Y. S., Nishikawa, T. & Motter, A. E. Stable chimeras and independently synchronizable clusters. Phys. Rev. Lett. 119, 084101 (2017).
Barrett, W., Francis, A. & Webb, B. Equitable decompositions of graphs with symmetries. Linear Algebra Appl. 513, 409–434 (2017).
MacArthur, B. D., Sánchez-García, R. J. & Anderson, J. W. Symmetry in complex networks. Discrete Appl. Math. 156, 3525–3531 (2008).
Kuramoto, Y. & Battogtokh, D. Coexistence of coherence and incoherence in nonlocally coupled phase oscillators. Nonlinear Phenom. Complex Syst. 5, 380–385 (2002).
Abrams, D. M. & Strogatz, S. H. Chimera states for coupled oscillators. Phys. Rev. Lett. 93, 174102 (2004).
Panaggio, M. J. & Abrams, D. M. Chimera states: coexistence of coherence and incoherence in networks of coupled oscillators. Nonlinearity 28, R67 (2015).
Hagerstrom, A. M. et al. Experimental observation of chimeras in coupled-map lattices. Nat. Phys. 8, 658–661 (2012).
Tinsley, M. R., Nkomo, S. & Showalter, K. Chimera and phase-cluster states in populations of coupled chemical oscillators. Nat. Phys. 8, 662–665 (2012).
Martens, E. A., Thutupalli, S., Fourrière, A. & Hallatschek, O. Chimera states in mechanical oscillator networks. Proc. Natl Acad. Sci. USA 110, 10563–10567 (2013).
Hart, J. D., Bansal, K., Murphy, T. E. & Roy, R. Experimental observation of chimera and cluster states in a minimal globally coupled network. Chaos 26, 094801 (2016).
Zhang, Y., Nishikawa, T. & Motter, A. E. Asymmetry-induced synchronization in oscillator networks. Phys. Rev. E 95, 062215 (2017).
Zhang, Y. & Motter, A. E. Identical synchronization of nonidentical oscillators: when only birds of different feathers flock together. Nonlinearity 31, R1 (2017).
Grainger, J. & Stevenson, W. Power System Analysis (McGraw-Hill, 1994).
Anderson, P. M. & Fouad, A. A. Power System Control and Stability (IEEE Press, 2003).
Nishikawa, T. & Motter, A. E. Comparative analysis of existing models for power-grid synchronization. New J. Phys. 17, 015012 (2015).
Susuki, Y., Mezić, I. & Hikihara, T. Coherent swing instability of power grids. J. Nonlinear Sci. 21, 403–439 (2011).
Lozano, S., Buzna, L. & Díaz-Guilera, A. Role of network topology in the synchronization of power systems. Eur. Phys. J. B 85, 231–238 (2012).
Menck, P. J., Heitzig, J., Kurths, J. & Schellnhuber, H. J. How dead ends undermine power grid stability. Nat. Commun. 5, 3969 (2014).
Auer, S., Kleis, K., Schultz, P., Kurths, J. & Hellmann, F. The impact of model detail on power grid resilience measures. Eur. Phys. J. Special Topics 225, 609–625 (2016).
Schäfer, B., Beck, C., Aihara, K., Witthaut, D. & Timme, M. Non-Gaussian power grid frequency fluctuations characterized by Lévy-stable laws and superstatistics. Nat. Energy 3, 119–126 (2018).
Burke, J. V., Lewis, A. S. & Overton, M. L. A robust gradient sampling algorithm for nonsmooth, nonconvex optimization. SIAM J. Optimiz. 15, 751–779 (2005).
Freitas, P. & Lancaster, P. On the optimal value of the spectral abscissa for a system of linear oscillators. SIAM J. Matrix Anal. A 21, 195–208 (1999).
Kirillov, O. N. & Overton, M. L. Robust stability at the swallowtail singularity. Front. Phys. 1, 1–9 (2013).
Boyd, S. Convex optimization of graph Laplacian eigenvalues. Proc. ICM 3, 1311–1319 (2006).
De Abreu, N. M. M. Old and new results on algebraic connectivity of graphs. Linear Algebra Appl. 423, 53–73 (2007).
Zou, W. & Zhan, M. Splay states in a ring of coupled oscillators: from local to global coupling. SIAM J. Appl. Dyn. Syst. 8, 1324–1340 (2009).
Uhlenbeck, G. E. & Ornstein, L. S. On the theory of the Brownian motion. Phys. Rev. 36, 823–841 (1930).
Gillespie, D. T. Markov Processes: An Introduction for Physical Scientists (Academic Press, 1991).
Doob, J. L. The Brownian movement and stochastic equations. Ann. Math. 43, 351–369 (1942).
Orfanidis, S. J. Introduction to Signal Processing (Prentice Hall, 1996).
Acknowledgements
We thank J.B. Ketterson for insightful discussions about this research. This research was funded by ARO Grant No. W911NF-15-1-0272 and by Northwestern University’s Finite Earth Initiative (supported by L. McQuown and M. McQuown).
Author information
Authors and Affiliations
Contributions
F.M., T.N. and A.E.M. designed the research and contributed to the modelling. F.M. performed the experiments and simulations. F.M., T.N. and A.E.M. analysed the results and wrote the paper. All authors approved the final manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Physics thanks Eckehard Schöll and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary sections 1 and 2 and Figs. 1–5.
Supplementary Video 1
Animated versions of Fig. 2b,c of the main text, visualizing the symmetric (top row) and asymmetric (bottom row) oscillations of the dominant eigenmodes.
Source Data Fig. 1
Data represented in Fig. 1e of the main text.
Source Data Fig. 2
Data represented in Fig. 2 of the main text.
Source Data Fig. 3
Data represented in Fig. 3 of the main text.
Rights and permissions
About this article
Cite this article
Molnar, F., Nishikawa, T. & Motter, A.E. Network experiment demonstrates converse symmetry breaking. Nat. Phys. 16, 351–356 (2020). https://doi.org/10.1038/s41567-019-0742-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-019-0742-y
This article is cited by
-
Heterogeneity-stabilized homogeneous states in driven media
Nature Communications (2021)
-
Asymmetry underlies stability in power grids
Nature Communications (2021)