Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Letter
  • Published:

Extreme magnetic field-boosted superconductivity

Nature Physics volume 15, pages 1250–1254 (2019)Cite this article

Subjects

Abstract

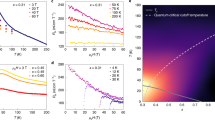
Applied magnetic fields underlie exotic quantum states, such as the fractional quantum Hall effect1 and Bose–Einstein condensation of spin excitations2. Superconductivity, however, is inherently antagonistic towards magnetic fields. Only in rare cases3,4,5 can these effects be mitigated over limited fields, leading to re-entrant superconductivity. Here, we report the coexistence of multiple high-field re-entrant superconducting phases in the spin-triplet superconductor UTe2 (ref. 6). We observe superconductivity in the highest magnetic field range identified for any re-entrant superconductor, beyond 65 T. Although the stability of superconductivity in these high magnetic fields challenges current theoretical models, these extreme properties seem to reflect a new kind of exotic superconductivity rooted in magnetic fluctuations7 and boosted by a quantum dimensional crossover8.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Magnetic field-induced superconducting and polarized phases of UTe2.
Fig. 2: Re-entrance of superconductivity in UTe2.
Fig. 3: Angle dependence of the field-induced superconducting and polarized phases of UTe2.
Fig. 4: Temperature dependence of SCFP in UTe2.

Similar content being viewed by others

Data availability

The data represented in Figs. 1–4 are available as source data in Supplementary Data 1–4. All other data that support the plots within this paper and other findings of this study are available from the corresponding authors on reasonable request.

References

  1. Stormer, H. L. Nobel lecture: the fractional quantum Hall effect. Rev. Mod. Phys. 71, 875–889 (1999).

    Article  ADS  MathSciNet  Google Scholar 

  2. Zapf, V., Jaime, M. & Batista, C. D. Bose–Einstein condensation in quantum magnets. Rev. Mod. Phys. 86, 563–614 (2014).

    Article  ADS  Google Scholar 

  3. Meul, H. W. et al. Observation of magnetic-field-induced superconductivity. Phys. Rev. Lett. 53, 497–500 (1984).

    Article  ADS  Google Scholar 

  4. Uji, S. et al. Magnetic-field-induced superconductivity in a two-dimensional organic conductor. Nature 410, 908–910 (2001).

    Article  ADS  Google Scholar 

  5. Konoike, T. et al. Magnetic-field-induced superconductivity in the antiferromagnetic organic superconductor κ−(BETS)2FeBr4. Phys. Rev. B 70, 094514 (2004).

    Article  ADS  Google Scholar 

  6. Ran, S. et al. Nearly ferromagnetic spin-triplet superconductivity. Science 365, 684–687 (2019).

    Article  ADS  Google Scholar 

  7. Mineev, V. P. Reentrant superconductivity in URhGe. Phys. Rev. B 91, 014506 (2015).

    Article  ADS  Google Scholar 

  8. Lebed, A. G. & Sepper, O. Quantum limit in a magnetic field for triplet superconductivity in a quasi-one-dimensional conductor. Phys. Rev. B 90, 024510 (2014).

    Article  ADS  Google Scholar 

  9. Jaccarino, V. & Peter, M. Ultra-high-field superconductivity. Phys. Rev. Lett. 9, 290–292 (1962).

    Article  ADS  Google Scholar 

  10. Lévy, F., Sheikin, I., Grenier, B. & Huxley, A. D. Magnetic field-induced superconductivity in the ferromagnet URhGe. Science 309, 1343–1346 (2005).

    Article  ADS  Google Scholar 

  11. Lévy, F., Sheikin, I. & Huxley, A. Acute enhancement of the upper critical field for superconductivity approaching a quantum critical point in URhGe. Nat. Phys. 3, 460–463 (2007).

    Article  Google Scholar 

  12. Aoki, D. et al. Unconventional superconductivity in heavy fermion UTe2. J. Phys. Soc. Jpn 88, 043702 (2019).

    Article  ADS  Google Scholar 

  13. Aoki, D. et al. Extremely large and anisotropic upper critical field and the ferromagnetic instability in UCoGe. J. Phys. Soc. Jpn 78, 113709 (2009).

    Article  ADS  Google Scholar 

  14. Huxley, A. D., Yates, S. J. C., Lévy, F. & Sheikin, I. Odd-parity superconductivity and the ferromagnetic quantum critical point. J. Phys. Soc. Jpn 76, 051011 (2007).

    Article  ADS  Google Scholar 

  15. Hattori, T. et al. Relationship between ferromagnetic criticality and the enhancement of superconductivity induced by transverse magnetic fields in UCoGe. J. Phys. Soc. Jpn 83, 073708 (2014).

    Article  ADS  Google Scholar 

  16. Knafo, W. et al. High-field moment polarization in the ferromagnetic superconductor UCoGe. Phys. Rev. B 86, 184416 (2012).

    Article  ADS  Google Scholar 

  17. De Boer, F. R. et al. High-magnetic-field and high-pressure effects in monocrystalline URu2Si2. Phys. B+C. 138, 1–6 (1986).

    Article  ADS  Google Scholar 

  18. Balicas, L. et al. Superconductivity in an organic insulator at very high magnetic fields. Phys. Rev. Lett. 87, 067002 (2001).

    Article  ADS  Google Scholar 

  19. Hattori, K. & Tsunetsugu, H. p-Wave superconductivity near a transverse saturation field. Phys. Rev. B 87, 064501 (2013).

    Article  ADS  Google Scholar 

  20. Sherkunov, Y., Chubukov, A. V. & Betouras, J. J. Effects of Lifshitz transitions in ferromagnetic superconductors: the case of URhGe. Phys. Rev. Lett. 121, 097001 (2018).

    Article  ADS  Google Scholar 

  21. Dupuis, N. & Montambaux, G. Superconductivity of quasi-one-dimensional conductors in a high magnetic field. Phys. Rev. B 49, 8993–9008 (1994).

    Article  ADS  Google Scholar 

  22. Sato, M. & Ando, Y. Topological superconductors: a review. Rep. Prog. Phys. 80, 076501 (2017).

    Article  ADS  MathSciNet  Google Scholar 

  23. Kallin, C. & Berlinsky, J. Chiral superconductors. Rep. Prog. Phys. 79, 054502 (2016).

    Article  ADS  Google Scholar 

  24. Sarma, S. D., Freedman, M. & Nayak, C. Majorana zero modes and topological quantum computation. npj Quant. Inf. 1, 15001 (2015).

    Article  ADS  Google Scholar 

  25. Karzig, T. et al. Scalable designs for quasiparticle-poisoning-protected topological quantum computation with Majorana zero modes. Phys. Rev. B 95, 235305 (2017).

    Article  ADS  Google Scholar 

  26. Miyake, A. et al. Metamagnetic transition in heavy fermion superconductor UTe2. J. Phys. Soc. Jpn 88, 063706 (2019).

    Article  ADS  Google Scholar 

  27. Knebel, G. et al. Field-reentrant superconductivity close to a metamagnetic transition in the heavy-fermion superconductor UTe2. J. Phys. Soc. Jpn 88, 063707 (2019).

    Article  ADS  Google Scholar 

  28. Palm, E. C. & Murphy, T. P. Very low friction rotator for use at low temperatures and high magnetic fields. Rev. Sci. Instrum. 70, 237–239 (1999).

    Article  ADS  Google Scholar 

  29. Altarawneh, M. M., Mielke, C. H. & Brooks, J. S. Proximity detector circuits: an alternative to tunnel diode oscillators for contactless measurements in pulsed magnetic field environments. Rev. Sci. Instrum. 80, 066104 (2009).

    Article  ADS  Google Scholar 

  30. Ghannadzadeh, S. et al. Measurement of magnetic susceptibility in pulsed magnetic fields using a proximity detector oscillator. Rev. Sci. Instrum. 82, 113902 (2011).

    Article  ADS  Google Scholar 

  31. Singleton, J. et al. Observation of the Fulde–Ferrell–Larkin–Ovchinnikov state in the quasi-two-dimensional organic superconductor κ–(BEDT–TTF)2Cu(NCS)2. J. Phys. Condens. Matter 12, L641 (2000).

    Article  Google Scholar 

  32. Goddard, P. A. et al. Experimentally determining the exchange parameters of quasi-two-dimensional Heisenberg magnets. New J. Phys. 10, 083025 (2008).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

We acknowledge helpful discussions with A. Lebed and V. Yakovenko. W.T.F. is grateful for the support of the Schmidt Science Fellows programme in partnership with the Rhodes Trust. Research at the University of Maryland was supported by the US National Science Foundation Division of Materials Research Award No. DMR-1610349 (support for sample preparation), the US Department of Energy (DOE) Award No. DE-SC-0019154 (support for experimental measurements) and the Gordon and Betty Moore Foundation’s EPiQS Initiative through Grant No. GBMF4419 (support for materials synthesis). Work performed at NHMFL was supported by NSF Cooperative Agreement No. DMR-1644779, the State of Florida, DOE and through the DOE Basic Energy Sciences Field Work Project Science in 100 T. A portion of this work was supported by the NHMFL User Collaboration Grants Program. Identification of commercial equipment does not imply recommendation or endorsement by NIST.

Author information

Authors and Affiliations

  1. Center for Nanophysics and Advanced Materials, Department of Physics, University of Maryland, College Park, MD, USA

    Sheng Ran, I-Lin Liu, Yun Suk Eo, Daniel J. Campbell, Paul M. Neves, Wesley T. Fuhrman, Shanta R. Saha, Christopher Eckberg, Hyunsoo Kim, Johnpierre Paglione & Nicholas P. Butch

  2. NIST Center for Neutron Research, National Institute of Standards and Technology, Gaithersburg, MD, USA

    Sheng Ran, I-Lin Liu, Shanta R. Saha, Johnpierre Paglione & Nicholas P. Butch

  3. Department of Materials Science and Engineering, University of Maryland, College Park, MD, USA

    Sheng Ran & I-Lin Liu

  4. National High Magnetic Field Laboratory, Florida State University, Tallahassee, FL, USA

    David Graf

  5. National High Magnetic Field Laboratory, Los Alamos National Laboratory, Los Alamos, NM, USA

    Fedor Balakirev & John Singleton

  6. Department of Physics, The Clarendon Laboratory, University of Oxford, Oxford, UK

    John Singleton

Authors
  1. Sheng Ran
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. I-Lin Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yun Suk Eo
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Daniel J. Campbell
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Paul M. Neves
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Wesley T. Fuhrman
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Shanta R. Saha
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Christopher Eckberg
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Hyunsoo Kim
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. David Graf
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Fedor Balakirev
    View author publications

    You can also search for this author in PubMed Google Scholar

  12. John Singleton
    View author publications

    You can also search for this author in PubMed Google Scholar

  13. Johnpierre Paglione
    View author publications

    You can also search for this author in PubMed Google Scholar

  14. Nicholas P. Butch
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

N.P.B. directed the project. S.R., W.T.F. and S.R.S. synthesized the single crystalline samples. S.R., I.-L.L., J.S. and F.B. performed the magnetoresistance, PDO and magnetization measurements in the pulsed field. Y.S.E., D.J.C., P.M.N. and D.G. performed the magnetoresistance measurements in the d.c. field. C.E. and H.K. performed magnetoresistance measurements in low magnetic fields. S.R. and N.P.B. wrote the manuscript with contributions from all authors.

Corresponding authors

Correspondence to Sheng Ran or Nicholas P. Butch.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Supplementary Figs. 1–5.

Supplementary Data 1

Source data for Fig. 1.

Supplementary Data 2

Source data for Fig. 2.

Supplementary Data 3

Source data for Fig. 3.

Supplementary Data 4

Source data for Fig. 4.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ran, S., Liu, IL., Eo, Y.S. et al. Extreme magnetic field-boosted superconductivity. Nat. Phys. 15, 1250–1254 (2019). https://doi.org/10.1038/s41567-019-0670-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-019-0670-x

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing