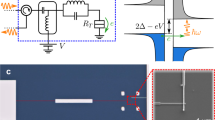
Abstract
The shift of the energy levels of a quantum system owing to broadband electromagnetic vacuum fluctuations—the Lamb shift—has been central for the development of quantum electrodynamics and for the understanding of atomic spectra1,2,3,4,5,6. Identifying the origin of small energy shifts is still important for engineered quantum systems, in light of the extreme precision required for applications such as quantum computing7,8. However, it is challenging to resolve the Lamb shift in its original broadband case in the absence of a tuneable environment. Consequently, previous observations1,2,3,4,5,9 in non-atomic systems are limited to environments comprising narrowband modes10,11,12. Here, we observe a broadband Lamb shift in high-quality superconducting resonators, a scenario also accessing static shifts inaccessible in Lamb’s experiment1,2. We measure a continuous change of several megahertz in the fundamental resonator frequency by externally tuning the coupling strength to the engineered broadband environment, which is based on hybrid normal-metal–insulator–superconductor tunnel junctions13,14,15. Our results may lead to improved control of dissipation in high-quality engineered quantum systems and open new possibilities for studying synthetic open quantum matter16,17,18 using this hybrid experimental platform.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
Data availability
The data that support the findings of this study are available at https://doi.org/10.5281/zenodo.1995361.
References
Lamb, W. E. & Retherford, R. C. Fine structure of the hydrogen atom by a microwave method. Phys. Rev. 72, 241–243 (1947).
Bethe, H. A. The electromagnetic shift of energy levels. Phys. Rev. 72, 339–341 (1947).
Heinzen, D. J. & Feld, M. S. Vacuum radiative level shift and spontaneous-emission linewidth of an atom in an optical resonator. Phys. Rev. Lett. 59, 2623–2626 (1987).
Brune, M. et al. From Lamb shift to light shifts: vacuum and subphoton cavity fields measured by atomic phase sensitive detection. Phys. Rev. Lett. 72, 3339–3342 (1994).
Marrocco, M., Weidinger, M., Sang, R. T. & Walther, H. Quantum electrodynamic shifts of Rydberg energy levels between parallel metal plates. Phys. Rev. Lett. 81, 5784–5787 (1998).
Carmichael, H. J. Statistical Methods in Quantum Optics 1 (Springer, Berlin, 1999).
Gisin, N. & Thew, R. Quantum communication. Nat. Photon. 1, 165–171 (2007).
Ladd, T. D. et al. Quantum computers. Nature 464, 45–53 (2010).
Rentrop, T. et al. Observation of the phononic Lamb shift with a synthetic vacuum. Phys. Rev. X 6, 041041 (2016).
Fragner, A. et al. Resolving vacuum fluctuations in an electrical circuit by measuring the Lamb shift. Science 322, 1357–1360 (2008).
Yoshihara, F. et al. Inversion of qubit energy levels in qubit-oscillator circuits in the deep-strong-coupling regime. Phys. Rev. Lett. 120, 183601 (2018).
Mirhosseini, M. et al. Superconducting metamaterials for waveguide quantum electrodynamics. Nat. Commun. 9, 3706 (2018).
Partanen, M. et al. Quantum-limited heat conduction over macroscopic distances. Nat. Phys. 12, 460–464 (2016).
Tan, K. Y. et al. Quantum-circuit refrigerator. Nat. Commun. 8, 15189 (2017).
Masuda, S. et al. Observation of microwave absorption and emission from incoherent electron tunneling through a normal-metal–insulator–superconductor junction. Sci. Rep. 8, 3966 (2018).
Houck, A. A., Türeci, H. E. & Koch, J. On-chip quantum simulation with superconducting circuits. Nat. Phys. 8, 292–299 (2012).
Fitzpatrick, M., Sundaresan, N. M., Li, A. C., Koch, J. & Houck, A. A. Observation of a dissipative phase transition in a one-dimensional circuit QED lattice. Phys. Rev. X 7, 011016 (2017).
Ma, R. et al. A dissipatively stabilized Mott insulator of photons. Nature 556, 51–57 (2019).
Gramich, V., Solinas, P., Möttönen, M., Pekola, J. P. & Ankerhold, J. Measurement scheme for the Lamb shift in a superconducting circuit with broadband environment. Phys. Rev. A 84, 052103 (2011).
Paraoanu, G. S. Microwave-induced coupling of superconducting qubits. Phys. Rev. B 74, 140504 (2006).
Rigetti, C. & Devoret, M. Fully microwave-tunable universal gates in superconducting qubits with linear couplings and fixed transition frequencies. Phys. Rev. B 81, 134507 (2010).
Kerckhoff, J., Nurdin, H. I., Pavlichin, D. S. & Mabuchi, H. Designing quantum memories with embedded control: photonic circuits for autonomous quantum error correction. Phys. Rev. Lett. 105, 040502 (2010).
Kapit, E., Chalker, J. T. & Simon, S. H. Passive correction of quantum logical errors in a driven, dissipative system: a blueprint for an analog quantum code fabric. Phys. Rev. A 91, 062324 (2015).
Geerlings, K. et al. Demonstrating a driven reset protocol for a superconducting qubit. Phys. Rev. Lett. 110, 120501 (2013).
Partanen, M. et al. Optimized heat transfer at exceptional points in quantum circuits. Preprint at https://arxiv.org/abs/1812.02683 (2018).
Weiss, U. Quantum Dissipative Systems. (World Scientific, Singapore, 2012).
Frisk Kockum, A., Delsing, P. & Johansson, G. Designing frequency-dependent relaxation rates and Lamb shifts for a giant artificial atom. Phys. Rev. A 90, 013837 (2014).
Silveri, M., Grabert, H., Masuda, S., Tan, K. Y. & Möttönen, M. Theory of quantum-circuit refrigeration by photon-assisted electron tunneling. Phys. Rev. B 96, 094524 (2017).
Ingold, G.-L. & Nazarov, Y. V. in Single Charge Tunneling: Coulomb Blockade Phenomena in Nanostructures (eds Grabert, H. & Devoret, M. H.) (Plenum, New York, 1992).
Dynes, R. C., Narayanamurti, V. & Garno, J. P. Direct measurement of quasiparticle-lifetime broadening in a strong-coupled superconductor. Phys. Rev. Lett. 41, 1509–1512 (1978).
Landau, L. D. & Lifshitz, E. M. Statistical Physics Part 1 (Pergamon, Oxford, 1980).
Caldeira, A. O. & Leggett, A. J. Quantum tunnelling in a dissipative system. Ann. Phys. 149, 374–456 (1983).
Gao, J. et al. Equivalence of the effects on the complex conductivity of superconductor due to temperature change and external pair breaking. J. Low Temp. Phys. 151, 557–563 (2008).
Goetz, J. et al. Loss mechanisms in superconducting thin film microwave resonators. J. Appl. Phys. 119, 015304 (2016).
Forn-Díaz, P. et al. Ultrastrong coupling of a single artificial atom to an electromagnetic continuum in the nonperturbative regime. Nat. Phys. 13, 39–43 (2017).
Catelani, G., Schoelkopf, R. J., Devoret, M. H. & Glazman, L. I. Relaxation and frequency shifts induced by quasiparticles in superconducting qubits. Phys. Rev. B 84, 064517 (2011).
Abramowitz, M. & Stegun, I. A. Handbook of Mathematical Functions (Dover, New York, 1972).
Acknowledgements
We acknowledge discussions with G. Catelani, A. Clerk, J. Govenius, H. Grabert and J. Tuorila. This research was financially supported by the European Research Council under grant no. 681311 (QUESS) and Marie Skłodowska-Curie grant no. 795159; by the Academy of Finland under its Centres of Excellence Program grant nos. 312300 and 312059 and grant nos. 265675, 305237, 305306, 308161, 312300, 314302, 316551 and 316619; JST ERATO grant no. JPMJER1601, JSPS KAKENHI grant no. 18K03486 and by the Alfred Kordelin Foundation, the Emil Aaltonen Foundation, the Vilho, Yrjö and Kalle Väisälä Foundation, the Jane and Aatos Erkko Foundation and the Technology Industries of Finland Centennial Foundation. We are grateful for the provision of facilities and technical support by Aalto University at OtaNano – Micronova Nanofabrication Centre.
Author information
Authors and Affiliations
Contributions
M.S. carried out the theoretical analysis and wrote the manuscript with input from all the authors. S.M., V.S. and M.J. conducted the experiments and analysed the data. S.M. and K.Y.T. fabricated the samples. R.E.L., M.P. and J.G. contributed to the fabrication, development of the devices and the measurement scheme. L.G. fabricated the niobium layers. E.H., M.P. and J.G. contributed to the data analysis. E.H. and F.H. gave theory support. M.M. supervised the work in all respects.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Figures.
Rights and permissions
About this article
Cite this article
Silveri, M., Masuda, S., Sevriuk, V. et al. Broadband Lamb shift in an engineered quantum system. Nat. Phys. 15, 533–537 (2019). https://doi.org/10.1038/s41567-019-0449-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-019-0449-0
This article is cited by
-
Optical scattering imaging with sub-nanometer precision based on position-ultra-sensitive giant Lamb shift
Science China Physics, Mechanics & Astronomy (2024)
-
Quantum bath engineering of a high impedance microwave mode through quasiparticle tunneling
Nature Communications (2022)
-
A low-noise on-chip coherent microwave source
Nature Electronics (2021)
-
Energy of a free Brownian particle coupled to thermal vacuum
Scientific Reports (2021)
-
Observation of quantum many-body effects due to zero point fluctuations in superconducting circuits
Nature Communications (2019)