Abstract
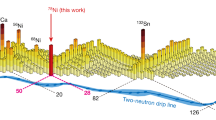
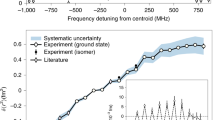
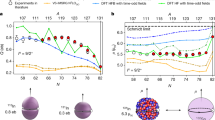
In rare cases, the removal of a single proton (Z) or neutron (N) from an atomic nucleus leads to a dramatic shape change. These instances are crucial for understanding the components of the nuclear interactions that drive deformation. The mercury isotopes (Z = 80) are a striking example1,2: their close neighbours, the lead isotopes (Z = 82), are spherical and steadily shrink with decreasing N. The even-mass (A = N + Z) mercury isotopes follow this trend. The odd-mass mercury isotopes 181,183,185Hg, however, exhibit noticeably larger charge radii. Due to the experimental difficulties of probing extremely neutron-deficient systems, and the computational complexity of modelling such heavy nuclides, the microscopic origin of this unique shape staggering has remained unclear. Here, by applying resonance ionization spectroscopy, mass spectrometry and nuclear spectroscopy as far as 177Hg, we determine 181Hg as the shape-staggering endpoint. By combining our experimental measurements with Monte Carlo shell model calculations, we conclude that this phenomenon results from the interplay between monopole and quadrupole interactions driving a quantum phase transition, for which we identify the participating orbitals. Although shape staggering in the mercury isotopes is a unique and localized feature in the nuclear chart, it nicely illustrates the concurrence of single-particle and collective degrees of freedom at play in atomic nuclei.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout


Similar content being viewed by others
Data availability
All of the relevant data that support the findings of this study are available from the corresponding author upon reasonable request.
Change history
24 January 2022
A Correction to this paper has been published: https://doi.org/10.1038/s41567-022-01503-4
References
Bonn, J., Huber, G., Kluge, H.-J., Kugler, L. & Otten, E. Sudden change in the nuclear charge distribution of very light mercury isotopes. Phys. Lett. B 38, 308–311 (1972).
Kühl, T. et al. Nuclear shape staggering in very neutron-deficient Hg isotopes detected by laser spectroscopy. Phys. Rev. Lett. 39, 180–183 (1977).
Andreyev, A. N. et al. A triplet of differently shaped spin-zero states in the atomic nucleus 186Pb. Nature 405, 430–433 (2000).
Wood, J. L. & Heyde, K. A focus on shape coexistence in nuclei. J. Phys. G 43, 020402 (2016).
Campbell, P., Moore, I. & Pearson, M. Laser spectroscopy for nuclear structure physics. Prog. Part. Nucl. Phys. 86, 127–180 (2016).
De Witte, H. et al. Nuclear charge radii of neutron-deficient lead isotopes beyond n= 104 midshell investigated by in-source laser spectroscopy.Phys. Rev. Lett. 98, 112502 (2007).
Heyde, K. & Wood, J. L. Shape coexistence in atomic nuclei. Rev. Mod. Phys. 83, 1467–1521 (2011).
Wrzosek-Lipska, K. & Gaffney, L. P. Unique and complementary information on shape coexistence in the neutron-deficient Pb region derived from Coulomb excitation. J. Phys. G 43, 024012 (2016).
Julin, R., Grahn, T., Pakarinen, J. & Rahkila, P. In-beam spectroscopic studies of shape coexistence and collectivity in the neutron-deficient z ≈ 82 nuclei. J. Phys. G 43, 024004 (2016).
Borge, M. J. G. & Blaum, K. Focus on exotic beams at ISOLDE: A laboratory portrait. J. Phys. G 45, 010301 (2017).
Otsuka, T., Honma, M., Mizusaki, T., Shimizu, N. & Utsuno, Y. Monte Carlo shell model for atomic nuclei. Prog. Part. Nucl. Phys. 47, 319–400 (2001).
Day Goodacre, T. Developments of the ISOLDE RILIS for Radioactive Ion Beam Production and the Results of their Application in the Study of Exotic Mercury Isotopes. PhD thesis, University of Manchester (2016).
Sels, S. Laser Spectroscopy of Neutron-Deficient Mercury Isotopes and Commissioning of a Gas-jet Based RFQ Ion Guide. PhD thesis, Instituut voor Kern en Stralingsfysica, KU Leuven (2018).
Otsuka, T. & Tsunoda, Y. The role of shell evolution in shape coexistence. J. Phys. G 43, 024009 (2016).
Sachdev, S. Quantum Phase Transitions 2nd edn (Cambridge Univ Press, Cambridge, 2011).
Carr, L. D. Understanding Quantum Phase Transitions (CRC, Boca Raton, 2010).
Togashi, T., Tsunoda, Y., Otsuka, T. & Shimizu, N. Quantum phase transition in the shape of Zr isotopes. Phys. Rev. Lett. 117, 172502 (2016).
Bree, N. et al. Shape coexistence in the neutron-deficient even–even 182–186 Hg isotopes studied via Coulomb excitation. Phys. Rev. Lett. 112, 162701 (2014).
Ulm, G. et al. Isotope shift of 182Hg and an update of nuclear moments and charge radii in the isotope range 181Hg-206Hg. Z. Phys. A. 325, 247–259 (1986).
Reimann, R. J. & McDermott, M. N. Precision magnetic moment determinations for 43-min 199mHg and other isomers of mercury. Phys. Rev. C 7, 2065–2079 (1973).
Bieroń, J., Pyykkö, P. & Jönsson, P. Nuclear quadrupole moment of 201Hg. Phys. Rev. A 71, 012502 (2005).
Kohler, R. H. Detection of double resonance by frequency change: application to 201Hg. Phys. Rev. 121, 1104–1111 (1961).
Day Goodacre, T. et al. Blurring the boundaries between ion sources: the application of the RILIS inside a FEBIAD-type ion source at ISOLDE. Nucl. Instrum. Meth. Phys. Res. B 376, 39–45 (2016).
Tsunoda, Y., Otsuka, T., Shimizu, N., Honma, M. & Utsuno, Y. Novel shape evolution in exotic Ni isotopes and configuration-dependent shell structure. Phys. Rev. C 89, 031301 (2014).
Lettry, J. et al. Release from ISOLDE molten metal targets under pulsed proton beam conditions. Nucl. Instrum. Meth. Phys. Res. B 126, 170–175 (1997).
Lettry, J. et al. Effects of thermal shocks on the release of radioisotopes and on molten metal target vessels. Nucl. Instrum. Meth. Phys. Res. B 204, 251–256 (2003).
Fedosseev, V. et al. Ion beam production and study of radioactive isotopes with the laser ion source at ISOLDE. J. Phys. G 44, 084006 (2017).
Day Goodacre, T. et al. RILIS-ionized mercury and tellurium beams at ISOLDE/CERN. Hyperfine Interact. 238, 41 (2017).
Marsh, B. A. Resonance ionization laser ion sources for on-line isotope separators (invited). Rev. Sci. Instrum. 85, 02B923 (2014).
Fedosseev, V. N., Kudryavtsev, Y. & Mishin, V. I. Resonance laser ionization of atoms for nuclear physics. Physica Scripta 85, 058104 (2012).
Rothe, S. et al. Narrow linewidth operation of the RILIS titanium: Sapphire laser at ISOLDE/CERN. Nucl. Instrum. Meth. Phys. Res. B 317, 561–564 (2013).
Wolf, R. N., Eritt, M., Marx, G. & Schweikhard, L. A multi-reflection time-of-flight mass separator for isobaric purification of radioactive ion beams. Hyperfine Interact. 199, 115–122 (2011).
Wienholtz, F. et al. Towards ultra high-resolution multi-reflection time-of-flight mass spectrometry at ISOLTRAP. Phys. Scr. T166, 014068 (2015).
Brown, B. A. Double-octupole states in 208Pb. Phys. Rev. Lett. 85, 5300–5303 (2000).
Otsuka, T. et al. Novel features of nuclear forces and shell evolution in exotic nuclei.Phys. Rev. Lett. 104, 012501 (2010).
Bohr, A. & Mottelson, B. R. Nuclear Structure (World Scientific, Singapore, 1998).
Berdichevsky, D. & Tondeur, F. Nuclear core densities isotope shifts, and the parametrization of the droplet model. Z. Phys. A 322, 141–147 (1985).
Acknowledgements
This work has received funding through the following channels: the Max-Planck-Society, IMPRS-PTFS; BMBF (German Federal Ministry for Education and Research) Nos. 05P12HGCI1 and 05P15HGCIA; STFC Grant Nos. ST/L005727, ST/M006433, ST/M006434, ST/L002868/1, ST/L005794/1; Slovak Research and Development Agency, contract No. APVV-14-0524; European Unions Seventh Framework Programme for Research and Technological Development under Grant Agreements 267194 (COFUND) and 289191 (LA3NET). FWO-Vlaanderen (Belgium), by GOA/2015/010 (BOF KU Leuven); the Inter-university Attraction Poles Programme initiated by the Belgian Science Policy Office (BriX network P7/12), by the European Commission within the Seventh Framework Programme through I3-ENSAR (contract no. RII3-CT-2010-262010) and by a grant from the European Research Council (ERC-2011-AdG-291561-HELIOS). S.S acknowledges the Agency for Innovation by Science and Technology in Flanders (IWT). L.P.G acknowledges FWO-Vlaanderen (Belgium) as an FWO Pegasus Marie Curie Fellow. A.W and K.Z acknowledge the Wolfgang-Gentner scholarship and the BMBF (German Federal Ministry for Education and Research) no. 05P12ODCIA. This work was supported by JSPS and FWO under the Japan-Belgium Research Cooperative Program. The MCSM calculations were performed on the K computer at RIKEN AICS (hp160211, hp170230). This work was also supported in part by Priority Issue on Post-K computer (Elucidation of the Fundamental Laws and Evolution of the Universe) from MEXT and JICFuS. J.D. and A.P. acknowledge partial support from the STFC grant No. ST/P003885/1. The density functional theory calculations were performed using the DiRAC Data Analytic system at the University of Cambridge, operated by the University of Cambridge High Performance Computing Service on behalf of the STFC DiRAC HPC Facility (www.dirac.ac.uk). This equipment was funded by BIS National E-infrastructure capital grant (ST/K001590/1), STFC capital grants ST/H008861/1 and ST/H00887X/1, and STFC DiRAC Operations grant ST/K00333X/1. DiRAC is part of the National e-Infrastructure.
Author information
Authors and Affiliations
Contributions
A.N.A, A.E.B, T.D.G, D.V.F, V.N.F, L.P.G, M.H, B.A.M, M.D.S. and P.V.D. conceived the experiment and prepared the proposal; T.D.G, D.V.F, V.N.F, B.A.M, Y.M.P, P.L.M, R.E.R, S.R. and M.V. carried out laser ionization scheme and/or ion source developments; A.N.A, K.B, T.E.C, V.N.F, M.H, S.K, B.A.M, L.S, P.V.D. and K.Z. supervised the participants; B.A, N.A.A, D.A, A.E.B, J.B, T.E.C, J.G.C, T.D.G, G.J.F.-S., D.V.F, V.N.F, K.T.F, L.P.G, L.G, M.H, K.M.L, V.M, B.A.M, Y.M.P, P.L.M, R.E.R, S.S, P.S, C.V.B, P.V.D, M.V, E.V, A.W, F.W. and A.Z. participated in data taking; T.O. and Y.T. performed MCSM calculations; J.D. and A.P. performed density functional theory calculations; A.E.B, T.D.G, D.V.F, B.A.M, P.L.M, R.E.R, S.R. and M.V. took part in laser set-up and operation; B.A, D.A, T.E.C, J.G.C, K.T.F, L.P.G, L.G, K.M.L, V.M, M.R, R.E.R, L.S, S.S, C.V.B, A.W, F.W. and R.N.W. set up and operated the detection and data acquisition systems; A.N.A, A.E.B, K.B, T.E.C, T.D.G, J.D, D.V.F, L.P.G, M.H, B.A.M, T.O, L.S, M.D.S, S.S, P.V.D, E.V. and K.W. contributed to the data analysis and interpretation; A.N.A, A.E.B, K.B, T.E.C, J.G.C, T.D.G, J.D, V.N.F, L.P.G, M.H, D.L, B.A.M, T.O, L.S, S.S, Y.T. and P.V.D. contributed to the manuscript preparation.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Marsh, B.A., Day Goodacre, T., Sels, S. et al. Characterization of the shape-staggering effect in mercury nuclei. Nature Phys 14, 1163–1167 (2018). https://doi.org/10.1038/s41567-018-0292-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-018-0292-8
This article is cited by
-
Predictions of nuclear charge radii based on the convolutional neural network
Nuclear Science and Techniques (2023)
-
α-Clustering in atomic nuclei from first principles with statistical learning and the Hoyle state character
Nature Communications (2022)
-
Reassigning the shapes of the 0+ states in the 186Pb nucleus
Communications Physics (2022)
-
Sterile neutrinos and the global reactor antineutrino dataset
Journal of High Energy Physics (2021)