Abstract
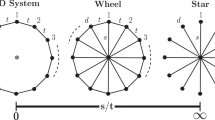
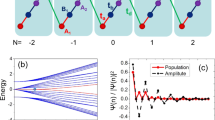
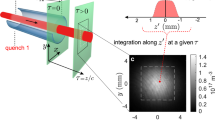
Quantum phase transitions, transitions between many-body ground states, are of extensive interest in research ranging from condensed-matter physics to cosmology1,2,3,4. Key features of the phase transitions include a stage with rapidly growing new order, called inflation in cosmology5, followed by the formation of topological defects6,7,8. How inflation is initiated and evolves into topological defects remains a hot topic of debate. Ultracold atomic gas offers a pristine and tunable platform to investigate quantum critical dynamics9,10,11,12,13,14,15,16,17,18,19,20,21. We report the observation of coherent inflationary dynamics across a quantum critical point in driven Bose–Einstein condensates. The inflation manifests in the exponential growth of density waves and populations in well-resolved momentum states. After the inflation stage, extended coherent dynamics is evident in both real and momentum space. We present an intuitive description of the quantum critical dynamics in our system and demonstrate the essential role of phase fluctuations in the formation of topological defects.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Sachdev, S. Quantum Phase Transitions 1st edn (Cambridge Univ. Press, Cambridge, 2011).
Morikawa, M. Cosmological inflation as a quantum phase transition. Progr. Theoret. Phys. 93, 685–709 (1995).
Kibble, T. W. B. Some implications of a cosmological phase transition. Phys. Rep. 67, 183–199 (1980).
Vojta, T. Quantum phase transitions. AIP Conf. Proc. 1550, 288–247 (2013).
Guth, A. H. Inflationary universe: A possible solution to the horizon and flatness problems. Phys. Rev. D 23, 347–356 (1981).
Kibble, T. W. B. Topology of cosmic domains and strings. J. Phys. A 9, 1387–1398 (1976).
Zurek, H. W. Cosmological experiments in superfluid helium? Nature 317, 505–508 (1985).
del Campo, A. & Zurek, W. H. Universality of phase transition dynamics: Topological defects from symmetry breaking. Int. J. Mod. Phys. A 29, 1430018 (2014).
Sadler, L. E., Higbie, J. M., Leslie, S. R., Vengalattore, M. & Stamper-Kurn, D. M. Spontaneous symmetry breaking in a quenched ferromagnetic spinor Bose–Einstein condensate. Nature 443, 312–315 (2006).
Polkovnikov, A., Sengupta, K., Silva, A. & Vengalattore, M. Nonequilibrium dynamics of closed interacting quantum systems. Rev. Mod. Phys. 83, 863–883 (2011).
Bloch, I., Dalibard, J. & Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 80, 885–964 (2008).
Dziarmaga, J. Dynamics of a quantum phase transition and relaxation to a steady state. Adv. Phys. 59, 1063–1189 (2010).
Baumann, K., Mottl, R., Brennecke, F. & Esslinger, T. Exploring symmetry breaking at the Dicke quantum phase transition. Phys. Rev. Lett. 107, 140402 (2011).
Barnett, R., Polkovnikov, A. & Vengalattore, M. Prethermalization in quenched spinor condensates. Phys. Rev. A 84, 023606 (2011).
Lamporesi, G., Donadello, S., Serafini, S., Dalfovo, F. & Ferrari, G. Spontaneous creation of Kibble–Zurek solitons in a Bose–Einstein condensate. Nat. Phys. 9, 656–660 (2013).
Nicklas, E. et al. Observation of scaling in the dynamics of a strongly quenched quantum gas. Phys. Rev. Lett. 115, 245301 (2015).
Navon, N., Gaunt, A. L., Smith, R. P. & Hadzibabic, Z. Critical dynamics of spontaneous symmetry breaking in a homogeneous Bose gas. Science 347, 167–170 (2015).
Klinder, J., Keler, H., Wolke, M., Mathey, L. & Hemmerich, A. Dynamical phase transition in the open Dicke model. Proc. Natl Acad. Sci. USA 112, 3290–3295 (2015).
Meldgin, C. et al. Probing the Bose glass–superfluid transition using quantum quenches of disorder. Nat. Phys. 12, 646–649 (2016).
Anquez, M. et al. Quantum Kibble–Zurek mechanism in a spin-1 Bose–Einstein condensate. Phys. Rev. Lett. 116, 155301 (2016).
Clark, L. W., Feng, L. & Chin, C. Universal space-time scaling symmetry in the dynamics of bosons across a quantum phase transition. Science 354, 606–610 (2016).
Parker, C. V., Ha, L.-C. & Chin, C. Direct observation of effective ferromagnetic domains of cold atoms in a shaken optical lattice. Nat. Phys. 9, 769–774 (2013).
Ha, L.-C., Clark, L. W., Parker, C. V., Anderson, B. M. & Chin, C. Roton-maxon excitation spectrum of bose condensates in a shaken optical lattice. Phys. Rev. Lett. 114, 055301 (2015).
Shvarchuck, I. et al. Bose–Einstein condensation into nonequilibrium states studied by condensate focusing. Phys. Rev. Lett. 89, 270404 (2002).
Hung, C. L. et al. Extracting density–density correlations from in situ images of atomic quantum gases. Phys. Rev. A 59, 4595–4607 (1999).
Morsch, O. & Oberthaler, M. Dynamics of Bose–Einstein condensates in optical lattices. Rev. Mod. Phys. 78, 179–215 (2006).
Anglin, J. R. Second-quantized Landau–Zener theory for dynamical instabilities. Phys. Rev. A 67, 051601 (2003).
Acknowledgements
We thank E. Berg, Q. Zhou and B. M. Anderson for helpful discussions. L.W.C. was supported by a Grainger fellowship. A.G. is supported by a Kadanoff–Rice fellowship. This work was supported by the University of Chicago Materials Research Science and Engineering Center, which is funded by the National Science Foundation under award number DMR-1420709, NSF grant PHY-1511696 and Army Research Office-Multidisciplinary Research Initiative grant W911NF-14-1-0003.
Author information
Authors and Affiliations
Contributions
L.F. and L.W.C. designed the experiments. L.F. performed the experiments and analysed the data. L.F. and C.C. prepared the manuscript. All authors contributed discussions on experiments and the manuscript. C.C. supervised.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Information, Supplementary Figures 1–3, Supplementary References
Rights and permissions
About this article
Cite this article
Feng, L., Clark, L.W., Gaj, A. et al. Coherent inflationary dynamics for Bose–Einstein condensates crossing a quantum critical point. Nature Phys 14, 269–272 (2018). https://doi.org/10.1038/s41567-017-0011-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-017-0011-x
This article is cited by
-
Crystallization of bosonic quantum Hall states in a rotating quantum gas
Nature (2022)
-
A driven three-dimensional electric lattice for polar molecules
Frontiers of Physics (2022)
-
Quantum thermodynamics of single particle systems
Scientific Reports (2020)
-
Quantum simulation of Unruh radiation
Nature Physics (2019)
-
Spin current generation and relaxation in a quenched spin-orbit-coupled Bose-Einstein condensate
Nature Communications (2019)