Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Nonlinear helical dichroism in chiral and achiral molecules

Nature Photonics volume 17, pages 82–88 (2023)Cite this article

Subjects

Abstract

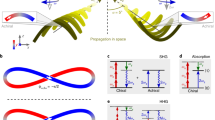
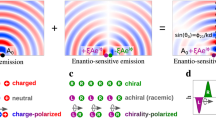
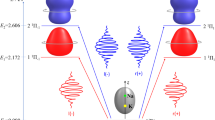
Chiral interactions are prevalent in nature, driving a variety of biochemical processes. Discerning the two non-superimposable mirror images of a chiral molecule, known as enantiomers, requires interaction with a chiral reagent with known handedness. Circularly polarized light beams are often used as a chiral reagent. Here we demonstrate efficient chiral sensitivity with linearly polarized helical light beams carrying an orbital angular momentum of ±lħ, in which the handedness is defined by the twisted wavefront structure tracing a left- or right-handed corkscrew pattern as it propagates in space. By probing the nonlinear optical response, we show that helicity-dependent nonlinear absorption occurs even in achiral molecules and can be controlled. We model this effect by considering induced multipole moments in light–matter interactions. Design and control of light–matter interactions with helical light may open new opportunities in chiroptical spectroscopy, light-driven molecular machines, optical switching and in situ ultrafast probing of chiral systems and magnetic materials.

This is a preview of subscription content, access via your institution

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Transmission of circularly polarized (s = ±1) femtosecond vortex pulses in fenchone.
Fig. 2: Helical dichroism (Type II) in fenchone with linearly polarized light (s = 0) as a function of peak laser fluence.
Fig. 3: Helicity-dependent absorption, HD(Type I), in achiral molecules.
Fig. 4: Helical dichroism with linearly polarized asymmetric OAM beams (l = ±1) in limonene.
Fig. 5: Helical dichroism (Type I) with asymmetric OAM beams in R(+)-limonene.

Data availability

The minimum dataset necessary to interpret the results can be obtained from the corresponding authors upon reasonable request.

Code availability

The simulation data were obtained by evaluating the equations using standard technical software. The code is available upon reasonable request to the corresponding authors.

References

  1. Caldwell, D. J. & Eyring, H. The Theory of Optical Activity (Univ. of Utah, 1971).

  2. Nakanishi, K., Berova, N. & Woody, R. Circular Dichroism: Principles and Applications (Wiley, 2000).

  3. Castiglioni, E., Abbate, S. & Longhi, G. Experimental methods for measuring optical rotatory dispersion: survey and outlook. Chirality 23, 711–716 (2011).

    Google Scholar 

  4. Kuball, H.-G. in Encyclopedia of Analytical Science 2nd edn (eds Worsfold, P. et al.) 60–79 (Elsevier, 2005).

  5. Janssen, M. H. M. & Powis, I. Detecting chirality in molecules by imaging photoelectron circular dichroism. Phys. Chem. Chem. Phys. 16, 856–871 (2014).

    Google Scholar 

  6. Tang, Y. & Cohen, A. E. Enhanced enantioselectivity in excitation of chiral molecules by superchiral light. Science 332, 333–336 (2011).

    ADS  Google Scholar 

  7. Collins, J. T. et al. Chirality and chiroptical effects in metal nanostructures: fundamental and current trends. Adv. Opt. Matter 5, 1700182 (2017).

    Google Scholar 

  8. Wang, X. & Tang, Z. Circular dichroism studies on plasmonic nanostructures. Small 13, 1601115 (2017).

    Google Scholar 

  9. Cireasa, R. et al. Probing molecular chirality on a sub-femtosecond timescale. Nat. Phys. 11, 654–658 (2015).

    Google Scholar 

  10. Bégin, J.-L., Alsaawy, M. & Bhardwaj, R. Chiral discrimination by recollision enhanced femtosecond laser mass spectrometry. Sci. Rep. 10, 14074 (2020).

    ADS  Google Scholar 

  11. Lux, C. et al. Circular dichroism in the photoelectron angular distributions of camphor and fenchone from multiphoton ionization with femtosecond laser pulses. Angew. Chem. Int. Ed. 51, 5001–5005 (2012).

    Google Scholar 

  12. Powis, I. Photoelectron circular dichroism of the randomly oriented chiral molecules glyceraldehyde and lactic acid. J. Chem. Phys. 112, 301 (2000).

    ADS  Google Scholar 

  13. Allen, L. et al. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 45, 8185–8189 (1992).

    ADS  Google Scholar 

  14. Torres, J. P. & Torner, L. Twisted Photons: Applications of Light with Orbital Angular Momentum (Wiley, 2011).

  15. Andrews, D. L. & Babiker, M. The Angular Momentum of Light (Cambridge Univ. Press, 2013).

  16. Surzhykov, A., Seipt, D. & Fritzsche, S. Probing the energy flow in Bessel light beams using atomic photoionization. Phys. Rev. A 94, 033420 (2016).

    ADS  Google Scholar 

  17. Wätzel, J. & Berakdar, J. Discerning on a sub-optical-wavelength the attosecond time delays in electron emission from magnetic sublevels by optical vortices. Phys. Rev. A 94, 033414 (2016).

    ADS  Google Scholar 

  18. Picón, A. et al. Transferring orbital and spin angular momenta of light to atoms. New J. Phys. 12, 083053 (2010).

    ADS  Google Scholar 

  19. Peshkov, A. A., Fritzsche, S. & Surzhykov, A. Ionization of H2+ molecular ions by twisted Bessel light. Phys. Rev. A 92, 043415 (2015).

    ADS  Google Scholar 

  20. Franke-Arnold, S., Allen, L. & Padgett, M. Advances in optical angular momentum. Laser Photon. Rev. 2, 299–313 (2008).

    ADS  Google Scholar 

  21. Andersen, M. F. et al. Quantized rotation of atoms from photons with orbital angular momentum. Phys. Rev. Lett. 97, 170406 (2006).

    ADS  Google Scholar 

  22. He, H. et al. Direct observation of transfer of angular momentum to absorptive particles from a laser beam with a phase singularity. Phys. Rev. Lett. 75, 826–829 (1995).

    ADS  Google Scholar 

  23. Schmiegelow, C. T. et al. Transfer of optical orbital angular momentum to a bound electron. Nat. Commun. 7, 129998 (2016).

    Google Scholar 

  24. Picón, A. et al. Photoionization with orbital angular momentum beams. Opt. Express 18, 3660–3671 (2010).

    ADS  Google Scholar 

  25. Loffler, W., Broer, D. J. & Woerdman, J. P. Circular dichroism of cholesteric polymers and the orbital angular momentum of light. Phys. Rev. A 83, 065801 (2011).

    ADS  Google Scholar 

  26. Araoka, F. et al. Interactions of twisted light with chiral molecules: an experimetal investigation. Phys. Rev. A 71, 055401 (2005).

    ADS  Google Scholar 

  27. Andrews, D. L., Romero, L. D. & Babiker, M. On optical vortex interactions with chiral matter. Opt. Commun. 237, 133–139 (2004).

    ADS  Google Scholar 

  28. Brullot, W. et al. Resolving enantiomers using the optical angular momentum of twisted light. Sci. Adv. 2, 150134 (2016).

    Google Scholar 

  29. Ni, J. et al. Giant helical dichroism of single chiral nanostructures with photonic orbital angular momentum. ACS Nano 15, 2893–2900 (2021).

    Google Scholar 

  30. Zambrana-Puyalto, X., Vidal, X. & Molina-Terriza, G. Angular momentum-induced circular dichroism in non-chiral nanostructures. Nat. Commun. 5, 4922 (2014).

    ADS  Google Scholar 

  31. Forbes, K. A. & Andrews, D. L. Spin–orbit interactions and chiroptical effects engaging orbital angular momentum of twisted light in chiral and achiral media. Phys. Rev. A 99, 023837 (2019).

    ADS  Google Scholar 

  32. Forbes, K. A. & Andrews, D. L. Optical orbital angular momentum: twisted light and chirality. Opt. Lett. 43, 435–438 (2018).

    ADS  Google Scholar 

  33. Marrucci, L., Manzo, C. & Paparo, D. Optical spin-to-orbital angular momentum conversion in inhomogeneous anisotropic media. Phys. Rev. Lett. 96, 163905 (2006).

    ADS  Google Scholar 

  34. Hugo, L. et al. Arbitrary optical wavefront shaping via spin-to-orbit coupling. J. Opt. 18, 124002 (2016).

    Google Scholar 

  35. Temnov, V. V. et al. Multiphoton ionization in dielectrics: comparison of circular and linear polarization. Phys. Rev. Lett. 97, 237403 (2006).

    ADS  Google Scholar 

  36. Vivas, M. G. et al. Polarization effect on the two-photon absorption of a chiral compound. Opt. Express 20, 18600–18608 (2012).

    ADS  Google Scholar 

  37. Gong, S.-H. & Penzkofer, A. Two-photon absorption and two-photon-induced absorption of some organic liquids at 347.15 nm. Opt. Quantum Electron. 31, 269–290 (1999).

    Google Scholar 

  38. Oosterbeek, R., Ashforth, S., Bodley, O. & Simpson, M. Measuring the ablation threshold fluence in femtosecond laser micromachining with vortex and Bessel pulses. Opt. Express 26, 34558–34568 (2018).

    ADS  Google Scholar 

  39. Rahimian, M. G. et al. Spatially controlled nano-structuring of silicon with femtosecond vortex pulses. Sci. Rep. 10, 12643 (2020).

    ADS  Google Scholar 

  40. Tang, Y. & Cohen, A. E. Optical chirality and its interaction with matter. Phys. Rev. Lett. 104, 163901 (2010).

    ADS  Google Scholar 

  41. Ohkubo, J. et al. Molecular alignment in a liquid induced by a nonresonant laser field: molecular dynamics simulation. J. Chem. Phys. 120, 9123–9132 (2004).

    ADS  Google Scholar 

  42. Dooley, P. W. et al. Direct imaging of rotation wave-packet dynamics of diatomic molecules. Phys. Rev. A 68, 023406 (2003).

    ADS  Google Scholar 

  43. Fleischer, S. et al. Molecular alignment induced by ultrashort laser pulses and its impact on molecular motion. Is. J. Chem. 52, 414–437 (2012).

    Google Scholar 

  44. Leibscher, M., Averbukh, I. S. & Rabitz, H. Molecular alignment by trains of short laser pulses. Phys. Rev. Lett. 90, 213001 (2003).

    ADS  Google Scholar 

  45. Leibscher, M., Averbukh, I. S. & Rabitz, H. Enhanced molecular alignment by short laser pulses. Phys. Rev. A 69, 013402 (2004).

    ADS  Google Scholar 

  46. Toro, C. et al. Two-photon absorption circular dichroism: a new twist in nonlinear spectroscopy. Chem. Eur. J. 16, 3504–3509 (2010).

    Google Scholar 

  47. Yang, N. & Cohen, A. E. Local geometry of electromagnetic fields and its role in molecular multipole transitions. J. Phys. Chem. B 115, 5304–5311 (2011).

    Google Scholar 

  48. Barron, L. D. Molecular Light Scattering and Optical Activity (Cambridge Univ. Press, 2004).

  49. Buckingham, A. D. & Dunn, M. B. Optical activity of oriented molecules. J. Chem. Soc. A 1971, 1988–1991 (1971).

    Google Scholar 

  50. Andrews, S. S. Using rotational averaging to calculate the bulk response of isotropic and anisotropic samples from molecular parameters. J. Chem. Educ. 81, 877 (2004).

    Google Scholar 

  51. Andrews, D. L. & Allcock, P. Optical Harmonics in Molecular Systems: Quantum Electrodynamical Theory (Wiley, 2005).

  52. Lipkin, D. Existence of a new conservation law in electromagnetic theory. J. Math. Phys. (N.Y.) 5, 696–700 (1964).

    ADS  MathSciNet  MATH  Google Scholar 

  53. Wang, Z. et al. A novel chiral metasurface with controllable circular dichroism induced by coupling localized and propagating modes. Adv. Opt. Mater. 4, 883–888 (2016).

    ADS  Google Scholar 

  54. Guo, Y. et al. Orbital angular momentum dichroism caused by the interaction of electric and magnetic dipole moments and the geometrical asymmetry of chiral metal nanoparticles. Phys. Rev. A 102, 033525 (2020).

    ADS  Google Scholar 

  55. Loudon, R. The Quantum Theory of Light (Oxford Univ. Press, 2003).

  56. Forbes, K. A. & Andrews, D. L. Orbital angular momentum of twisted light: chirality and optical activity. J. Phys. Photon. 3, 022007 (2021).

    Google Scholar 

  57. Bradshaw, D. S. & Andrews, D. L. Manipulating particles with light: radiation and gradient forces. Eur. J. Phys. 38, 034008 (2017).

    Google Scholar 

  58. Cerjan, A. et al. Orbital angular momentum of Laguerre-Gaussian beams beyond the paraxial approximation. J. Opt. Soc. Am. A 28, 2253–2260 (2011).

    ADS  Google Scholar 

  59. Forbes, K. A. et al. Relevance of longitudinal fields of paraxial optical vortices. J. Opt. 23, 075401 (2021).

    ADS  Google Scholar 

Download references

Acknowledgements

We acknowledge financial support from the Natural Science and Engineering Council of Canada, Canada Research Chairs and Canadian Foundation for Innovation.

Author information

Author notes

  1. These authors contributed equally: Jean-Luc Bégin, Ashish Jain.

Authors and Affiliations

  1. Department of Physics, University of Ottawa, Ottawa, Ontario, Canada

    Jean-Luc Bégin, Ashish Jain, Andrew Parks, Felix Hufnagel, Paul Corkum, Ebrahim Karimi, Thomas Brabec & Ravi Bhardwaj

Authors
  1. Jean-Luc Bégin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ashish Jain
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Andrew Parks
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Felix Hufnagel
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Paul Corkum
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Ebrahim Karimi
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Thomas Brabec
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Ravi Bhardwaj
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

J-L.B., A.J. and R.B. conceived the experiments. J-L.B., A.J. and R.B. designed and planned the experiments. J-L.B. and A.J. conducted the experiments and analysed the results. J-L.B., A.J., A.P., T.B. and R.B. worked on the theory and conducted numerical simulations. F.H. fabricated the q-plates, P.C., E.K. and R.B. supervised the project. J-L.B., A.J., T.B. and R.B. prepared the first draft, and all authors reviewed the manuscript.

Corresponding author

Correspondence to Ravi Bhardwaj.

Ethics declarations

Competing interests

The authors declare no competing interests.

Peer review

Peer review information

Nature Photonics thanks David Andrews and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Additional information

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Supplementary Information, including ten sections. Additional results are provided in sections 1 to 7, including Figs. 1–8. The Discussion includes three sections: fluence calculations with Fig. 9, optical force dipole with Fig. 10 and the theory on extension to multiphotons.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Bégin, JL., Jain, A., Parks, A. et al. Nonlinear helical dichroism in chiral and achiral molecules. Nat. Photon. 17, 82–88 (2023). https://doi.org/10.1038/s41566-022-01100-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41566-022-01100-0

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing