Abstract
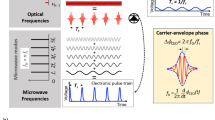
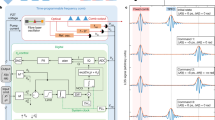
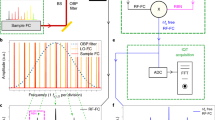
Optical frequency measurements are among the most precise tools available to science. With the rapid advances in optical clocks now achieving a low 10−17 stability at 1 s and averaging down to the 10−19 level in a few hundred seconds, real-time sensing of subtle phenomena becomes essential. To render possible such measurements, we introduce real-time optical phase tracking with ultra-low-noise frequency combs as a fundamental means to constantly monitor frequency offsets. This enables the characterization of optical frequency synthesis with stability and accuracy at the 20th decimal place within a measurement time of <100 s. To enable comb operation at this level of performance, dichroic heterodyne detection is used to compensate phase drifts occurring in the generation and dissemination of widely spaced optical frequencies. We qualify an example set-up by comparison with a reference system, measuring an offset between two combs of (5.4 ± 5.3) × 10−21 in one single measurement run of 1 × 105 s.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data that support the findings of this study are available from the corresponding author upon reasonable request.
References
Hall, J. L. Nobel lecture: Defining and measuring optical frequencies. Rev. Mod. Phys. 78, 1279–1295 (2006).
Hänsch, T. W. Nobel lecture: Passion for precision. Rev. Mod. Phys. 78, 1297–1309 (2006).
Udem, T., Reichert, J., Holzwarth, R. & Hänsch, T. W. Absolute optical frequency measurement of the cesium D1 line with a mode-locked laser. Phys. Rev. Lett. 82, 3568–3571 (1999).
Diddams, S. A. et al. Direct link between microwave and optical frequencies with a 300 THz femtosecond laser comb. Phys. Rev. Lett. 84, 5102–5105 (2000).
Udem, T., Holzwarth, R. & Hänsch, T. W. Optical frequency metrology. Nature 416, 233–237 (2002).
Keilmann, F., Gohle, C. & Holzwarth, R. Time-domain mid-infrared frequency-comb spectrometer. Opt. Lett. 29, 1542–1544 (2004).
Barmes, I., Witte, S. & Eikema, K. S. E. Spatial and spectral coherent control with frequency combs. Nat. Photon. 7, 38–42 (2013).
Bernhardt, B. et al. Cavity-enhanced dual-comb spectroscopy. Nat. Photon. 4, 55–57 (2010).
Coddington, I., Newbury, N. & Swann, W. Dual-comb spectroscopy. Optica 3, 414–426 (2016).
Lomsadze, B., Smith, B. C. & Cundiff, S. T. Tri-comb spectroscopy. Nat. Photon. 12, 676–680 (2018).
Picqué, N. & Hänsch, T. W. Frequency comb spectroscopy. Nat. Photon. 13, 146–157 (2019).
Baltuška, A. et al. Attosecond control of electronic processes by intense light fields. Nature 421, 611–615 (2003).
Fortier, T. M. et al. Generation of ultrastable microwaves via optical frequency division. Nat. Photon. 5, 425–429 (2011).
Xie, X. et al. Photonic microwave signals with zeptosecond-level absolute timing noise. Nat. Photon. 11, 44–47 (2016).
Zobel, J. W. et al. Comparison of optical frequency comb and sapphire loaded cavity microwave oscillators. IEEE Photon. Technol. Lett. 31, 1323–1326 (2019).
Coddington, I., Swann, W. C., Nenadovic, L. & Newbury, N. R. Rapid and precise absolute distance measurements at long range. Nat. Photon. 3, 351–356 (2009).
Lezius, M. et al. Space-borne frequency comb metrology. Optica 3, 1381–1387 (2016).
Diddams, S. A. et al. An optical clock based on a single trapped 199Hg+ ion. Science 293, 825–828 (2001).
Udem, Th., Holzwarth, R. & Hänsch, T. W. Optical frequency metrology. Nature 416, 233–237 (2002).
Schioppo, M. et al. Ultrastable optical clock with two cold-atom ensembles. Nat. Photon. 11, 48–52 (2017).
Campbell, S. L. et al. A Fermi-degenerate three-dimensional optical lattice clock. Science 358, 90–94 (2017).
Marti, G. E. et al. Imaging optical frequencies with 100 µHz precision and 1.1 µm resolution. Phys. Rev. Lett. 120, 103201 (2017).
Ushijima, I., Takamoto, M., Das, M., Ohkubo, T. & Katori, H. Cryogenic optical lattice clocks. Nat. Photon. 9, 185–189 (2015).
Nicholson, T. L. et al. Systematic evaluation of an atomic clock at 2 × 10−18 total uncertainty. Nat. Commun. 6, 6896 (2015).
Huntemann, N., Sanner, C., Lipphardt, B., Tamm, C. & Peik, E. Single-ion atomic clock with 3 × 10−18 systematic uncertainty. Phys. Rev. Lett. 116, 063001 (2016).
McGrew, W. F. et al. Atomic clock performance enabling geodesy below the centimetre level. Nature 564, 87–90 (2018).
Brewer, S. M. et al. 27Al+ quantum-logic clock with a systematic uncertainty below 10−18. Phys. Rev. Lett. 123, 033201 (2019).
Kessler, T. et al. A sub-40-mHz-linewidth laser based on a silicon single-crystal optical cavity. Nat. Photon. 6, 687–692 (2012).
Häfner, S. et al. 8 × 10−17 fractional laser frequency instability with a long room-temperature cavity. Opt. Lett. 40, 2112–2115 (2015).
Matei, D. G. et al. 1.5 μm lasers with sub-10 mHz linewidth. Phys. Rev. Lett. 118, 263202 (2017).
Robinson, J. M. et al. Crystalline optical cavity at 4 K with thermal-noise-limited instability and ultralow drift. Optica 6, 240–243 (2019).
Oelker, E. et al. Demonstration of 4.8 × 10−17 stability at 1 s for two independent optical clocks. Nat. Photon. 13, 714–719 (2019).
Takano, T. et al. Geopotential measurements with synchronously linked optical lattice clocks. Nat. Photon. 10, 662–666 (2016).
Grotti, J. et al. Geodesy and metrology with a transportable optical clock. Nat. Phys. 14, 437–441 (2018).
Takamoto, M. et al. Frequency ratios of Sr, Yb and Hg based optical lattice clocks and their applications. C. R. Phys. 16, 489–498 (2015).
Nemitz, N. et al. Frequency ratio of Yb and Sr clocks with 5 × 10−17 uncertainty at 150 seconds averaging time. Nat. Photon. 10, 258–261 (2016).
Ma, L. et al. Optical frequency synthesis and comparison with uncertainty at the 10−19 level. Science 303, 1843–1845 (2004).
Nicolodi, D. et al. Spectral purity transfer between optical wavelengths at the 10−18 level. Nat. Photon. 8, 219–223 (2014).
Johnson, L. A. M., Gill, P. & Margolis, H. S. Evaluating the performance of the NPL femtosecond frequency combs: agreement at the 10−21 level. Metrologia 52, 62–71 (2015).
Yao, Y., Jiang, Y., Yu, H., Bi, Z. & Ma, L. Optical frequency divider with division uncertainty at the 10−21 level. Natl Sci. Rev. 3, 463–469 (2016).
Leopardi, H. et al. Single-branch Er:fiber frequency comb for precision optical metrology with 10−18 fractional instability. Optica 4, 879–885 (2017).
Rolland, A. et al. Ultra-broadband dual-branch optical frequency comb with 10−18 instability. Optica 5, 1070–1077 (2018).
Barbieri, P., Clivati, C., Pizzocaro, M., Levi, F. & Calonico, D. Spectral purity transfer with 5 × 10−17 instability at 1 s using a multibranch Er:fiber frequency comb. Metrologia 56, 045008 (2019).
Telle, H. R., Lipphardt, B. & Stenger, J. Kerr-lens, mode-locked lasers as transfer oscillators for optical frequency measurements. Appl. Phys. B 74, 1–6 (2002).
Santarelli, G. et al. Frequency stability degradation of an oscillator slaved to a periodically interrogated atomic resonator. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 45, 887–894 (1998).
Marra, G., Margolis, H. S. & Richardson, D. J. Dissemination of an optical frequency comb over fiber with 3 × 10−18 fractional accuracy. Opt. Express 20, 1775–1782 (2012).
Riehle, F. Towards a redefinition of the second based on optical atomic clocks. C. R. Phys. 16, 506–515 (2015).
Grebing, C. et al. Realization of a timescale with an accurate optical lattice clock. Optica 3, 563–569 (2016).
Riehle, F. Optical clock networks. Nat. Photon. 11, 25–31 (2017).
Bize, S. The unit of time: present and future directions. C. R. Phys. 20, 153–168 (2019).
Milner, W. R. et al. Demonstration of a time scale based on a stable optical carrier. Phys. Rev. Lett. (in the press); preprint at https://arxiv.org/abs/1907.03184 (2019).
Hänsel, W. et al. All polarization-maintaining fiber laser architecture for robust femtosecond pulse generation. Appl. Phys. B 123, 41 (2017).
Hänsel, W., Giunta, M., Lezius, M., Fischer, M. & Holzwarth, R. Electro-optic modulator for rapid control of the carrier-envelope offset frequency. In Conference on Lasers and Electro-Optics SF1C.5 (OSA, 2017).
Nazarova, T., Riehle, F. & Sterr, U. Vibration-insensitive reference cavity for an ultra-narrow-linewidth laser. Appl. Phys. B 83, 531–536 (2006).
Kramer, G. & Klische, W. Extra high precision digital phase recorder. In 18th European Frequency and Time Forum 595–602 (Institution of Engineering and Technology, 2004).
Acknowledgements
We acknowledge funding from the European Union’s 7th Framework Programme (EU FP7) Marie Skłodowska-Curie Initial Training Network Future Atomic Clock Technology (FACT), the Defense Advanced Research Projects Agency’s (DARPA) Program in Ultrafast Laser Science and Engineering (PULSE, PμreComb project) under contract no. W31P4Q-14-C-0050 and the German Space Agency (DLR) projects ‘Faser-optischer Kammgenerator für angewandte LIDAR-Spektroskopie’ (FOKAL), ‘Faser-optischer Kammgenerator unter Schwerelosigkeit’ (FOKUS and FOKUS II) and ‘InfraRed Astronomy Satellite Swarm Interferometry’ (IRASSI). We thank H. Katori and J. Ye for insightful discussions and colleagues from Menlo Systems for technical support.
Author information
Authors and Affiliations
Contributions
M.G. and W.H. performed the experiments, conceived and realized the two frequency combs and the optical set-up. M.G. analysed the data. M.G. and W.H. wrote the manuscript. M.F. and M.L. managed the project. T.U. provided deep insight into the interpretation of the results and aided with the optical set-up. R.H. initiated and led the activities. All co-authors commented on and improved the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Giunta, M., Hänsel, W., Fischer, M. et al. Real-time phase tracking for wide-band optical frequency measurements at the 20th decimal place. Nat. Photonics 14, 44–49 (2020). https://doi.org/10.1038/s41566-019-0520-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41566-019-0520-5