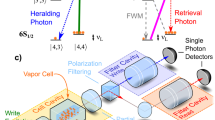
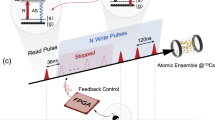
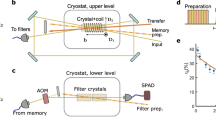
Abstract
Photonic quantum information technologies rely on quantum memory for long-lived storage and coherent manipulation of short pulses of non-classical light. The optical quantum memories explored over the past two decades are based on various coherent light–matter interaction schemes, but despite impressive progress, practical memories featuring efficient, broadband and long-lived operation remain elusive, due to the technical demands and inherent limitations of the established schemes. Here, we introduce a technique for high-speed quantum memory and manipulation that overcomes these obstacles. This scheme relies on dynamically controlled absorption of light via the ‘Autler–Townes effect’, which mediates reversible transfer between photonic coherence and the collective ground-state coherence of the storage medium. We experimentally demonstrate proof-of-concept storage and signal processing capabilities of our protocol in a laser-cooled gas of rubidium atoms, including storage of nanoseconds-long single-photon-level laser pulses for up to a microsecond. This approach opens up new avenues in quantum optics, with immediate applications on atomic and solid-state platforms.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on SpringerLink
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout






Similar content being viewed by others
Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding authors upon reasonable request.
References
Autler, S. H. & Townes, C. H. Stark effect in rapidly varying fields. Phys. Rev. 100, 703–722 (1955).
Picque, J. L. & Pinard, J. Direct observation of the Autler–Townes effect in the optical range. J. Phys. B 9, L77–L81 (1976).
He, X. F., Fisk, P. T. H. & Manson, N. B. Autler–Townes effect of the photoexcited diamond nitrogen-vacancy center in its triplet ground state. J. Appl. Phys. 72, 211–217 (1992).
Zhu, Y. F. et al. Vacuum Rabi splitting as a feature of analysis and experimental-observations. Phys. Rev. Lett. 64, 2499–2502 (1990).
Thompson, R. J., Rempe, G. & Kimble, H. J. Observation of normal-mode splitting for an atom in an optical cavity. Phys. Rev. Lett. 68, 1132–1135 (1992).
Bernadot, F., Nussenzveig, P., Brune, M., Raimond, J. M. & Haroche, S. Vacuum Rabi splitting observed on a microscopic atomic sample in a microwave cavity. Europhys. Lett. 17, 33–38 (1992).
Wade, C. G. et al. Probing an excited-state atomic transition using hyperfine quantum-beat spectroscopy. Phys. Rev. A 90, 033424 (2014).
Holloway, C. L. et al. Sub-wavelength imaging and field mapping via electromagnetically induced transparency and Autler–Townes splitting in Rydberg atoms. App. Phys. Lett. 104, 244012 (2014).
Ghafoor, F. Autler–Townes multiplet spectroscopy. Laser. Phys. 24, 035702 (2014).
Lukin, M. D. Colloquium: trapping and manipulating photon states in atomic ensembles. Rev. Mod. Phys. 75, 457–472 (2003).
Fleischhauer, M., Imamoglu, A. & Marangos, J. P. Electromagnetically induced transparency: optics in coherent media. Rev. Mod. Phys. 77, 633–673 (2005).
Abi-Salloum, T. Y. Electromagnetically induced transparency and Autler-–Townes splitting: two similar but distinct phenomena in two categories of three-level atomic systems. Phys. Rev. A 81, 053836 (2010).
Anisimov, P. M., Dowling, J. P. & Sanders, B. C. Objectively discerning Autler–Townes splitting from electromagnetically induced transparency. Phys. Rev. Lett. 107, 163604 (2011).
Giner, L. et al. Experimental investigation of the transition between Autler–Townes splitting and electromagnetically-induced-transparency models. Phys. Rev. A 87, 013823 (2013).
Tan, C. & Huang, G. Crossover from electromagnetically induced transparency to Autler–Townes splitting in open ladder systems with Doppler broadening. J. Opt. Soc. Am. B 31, 704–715 (2014).
Peng, B., Chen, W., Nori, F., Ozdemir, K. O. & Yang, L. What is and what is not electromagnetically induced transparency in whispering-gallery microcavities. Nat. Commun. 5, 5082 (2014).
Lu, X. et al. Transition from Autler–Townes splitting to electromagnetically induced transparency based on the dynamics of decaying dressed states. J. Phys. B 48, 055003 (2015).
He, L.-Y., Wang, T.-J., Gao, Y.-P., Cao, C. & Wang, C. Discerning electromagnetically induced transparency from Autler–Townes splitting in plasmonic waveguide and coupled resonators system. Opt. Express 23, 23817–23826 (2015).
Liao, W.-T., Keitel, C. H. & Pálffy, A. All-electromagnetic control of broadband quantum excitations using gradient photon echoes. Phys. Rev. Lett. 113, 123602 (2014).
Liu, C., Dutton, Z., Behroozi, C. H. & Hau, L. V. Observation of coherent optical information storage in an atomic medium using halted light pulses. Nature 409, 490–493 (2001).
Hosseini, M. et al. Coherent optical pulse sequencer for quantum applications. Nature 461, 241–245 (2009).
Afzelius, M. et al. Demonstration of atomic frequency comb memory for light with spin-wave storage. Phys. Rev. Lett. 104, 040503 (2010).
Reim, K. F. et al. Towards high-speed optical quantum memories. Nat. Photon. 4, 218–221 (2010).
Hedges, M. P., Longdell, J. J., Li, Y. & Sellars, M. J. Efficient quantum memory for light. Nature 465, 1052–1056 (2010).
Clausen, C. et al. Quantum storage of photonic entanglement in a crystal. Nature 469, 508–512 (2011).
Saglamyurek, E. et al. Broadband waveguide quantum memory for entangled photons. Nature 469, 513–518 (2011).
Mohapatra, A. K., Jackson, T. R. & Adams, C. S. Coherent optical detection of highly excited Rydberg states using electromagnetically induced transparency. Phys. Rev. Lett. 98, 113003 (2007).
Pritchard, J. D. et al. Cooperative atom–light interaction in a blockaded Rydberg ensemble. Phys. Rev. Lett. 105, 193603 (2010).
Zhou, Y. et al. Coherent control of a strongly driven silicon vacancy optical transition in diamond. Nat. Commun. 8, 14451 (2017).
Siyushev, P. et al. Optical and microwave control of germanium-vacancy center spins in diamond. Phys. Rev. B 96, 081201(R) (2017).
Agarwal, G. S. & Huang, S. Electromagnetically induced transparency in mechanical effects of light. Phys. Rev. A 81, 041803 (2010).
Huang, J. Y. et al. In situ observation of the electrochemical lithiation of a single SnO2 nanowire electrode. Science 330, 1515–1520 (2010).
Teufel, J. D. et al. Circuit cavity electromechanics in the strong-coupling regime. Nature 471, 204–208 (2011).
Safavi-Naeini, A. H. et al. Electromagnetically induced transparency and slow light with optomechanics. Nature 472, 69–73 (2011).
Sillanpää, M. A. et al. Autler–Townes effect in a superconducting three-level system. Phys. Rev. Lett. 103, 193601 (2009).
Abdumalikov, A. A. et al. Electromagnetically induced transparency on a single artificial atom. Phys. Rev. Lett. 104, 193601 (2010).
Novikov, S. et al. Autler–Townes splitting in a three-dimensional transmon superconducting qubit. Phys. Rev. B 88, 060503 (2013).
Sun, H.-C. et al. Electromagnetically induced transparency and Autler–Townes splitting in superconducting flux quantum circuits. Phys. Rev. A 89, 063822 (2014).
Gorshkov, A. V., André, A., Sørensen, A. S. & Lukin, M. D. Universal approach to optimal photon storage in atomic media. Phys. Rev. Lett. 98, 123601 (2007).
Gorshkov, A. V., André, A., Lukin, M. D. & Sørensen, A. S. Photon storage in Λ-type optically dense atomic media. II. Free-space model. Phys. Rev. A 76, 033804 (2007).
Heshami, K. et al. Quantum memories: emerging applications and recent advances. J. Mod. Opt. 63, 2005–2028 (2016).
Afzelius, M., Simon, C., de Riedmatten, H. & Gisin, N. Multimode quantum memory based on atomic frequency combs. Phys. Rev. A 79, 052329 (2009).
Tittel, W. et al. Photon-echo quantum memory in solid state system. Laser Photon. Rev. 4, 244–267 (2010).
Riedl, S. et al. Bose–Einstein condensate as a quantum memory for a photonic polarization qubit. Phys. Rev. A 85, 022318 (2012).
Cho, Y.-W. et al. Highly efficient optical quantum memory with long coherence time in cold atoms. Optica 3, 100–107 (2016).
Hsiao, Y.-F. et al. Highly efficient coherent optical memory based on electromagnetically induced transparency. Phys. Rev. Lett. 120, 183602 (2018).
Saglamyurek, E. et al. An integrated processor for photonic quantum states using a broadband light–matter interface. New J. Phys. 16, 065019 (2014).
Reim, K. F. et al. Multipulse addressing of a raman quantum memory: configurable beam splitting and efficient readout. Phys. Rev. Lett. 108, 263602 (2013).
Campbell, G. T. et al. Configurable unitary transformations and linear logic gates using quantum memories. Phys. Rev. Lett. 113, 063601 (2014).
Lukin, M. D. & Imamoglu, A. Controlling photons using electromagnetically induced transparency. Nature 413, 273–276 (2001).
Nunn, J. et al. Mapping broadband single-photon wave packets into an atomic memory. Phys. Rev. A 75, 011401(R) (2007).
Novikova, I., Phillips, N. B. & Gorshkov, A. V. Optimal light storage with full pulse-shape control. Phys. Rev. A 78, 021802(R) (2008).
Nillson, M. & Kroll, S. Solid-state quantum memory using complete absorption and re-emission of photons by tailored and externally controlled inhomogeneous absorption profiles. Opt. Commun. 247, 393–403 (2005).
Alexander, A. L., Longdell, J. J., Sellars, M. J. & Manson, N. B. Photon echoes produced by switching electric fields. Phys. Rev. Lett. 96, 043602 (2006).
Kraus, J. B. et al. Quantum memory for nonstationary light fields based on controlled reversible inhomogeneous broadening. Phys. Rev. A 73, 020302(R) (2006).
Hetet, G., Longdell, J. J., Alexander, A. L., Lam, P. K. & Sellars, M. J. Electro-optic quantum memory for light using two-level atoms. Phys. Rev. Lett. 100, 023601 (2008).
Nunn, J. et al. Multimode memories in atomic ensembles. Phys. Rev. Lett. 101, 260502 (2008).
Bustard, P. J., England, D. G., Heshami, K., Kupchak, C. & Sussman, B. J. Reducing noise in a Raman quantum memory. Opt. Lett. 41, 5055–5058 (2016).
Nunn, J. et al. Theory of noise suppression in Λ-type quantum memories by means of a cavity. Phys. Rev. A 96, 012338 (2017).
Khan, S., Bharti, V. & Natarajan, V. Role of dressed-state interference in electromagnetically induced transparency. Phys. Lett. A 380, 4100–4104 (2016).
Acknowledgements
We appreciate generous technical support from G. Popowich, P. Davis, S. Wilson, S. Hubele, L. Cooke and the following groups for lending us equipment for our initial measurements: J. Beamish, J. P. Davis, F. Hegmann, A. Lvovsky, W. Tittel, R. Wolkow. We also thank B. Sanders, Y.-C. Chen and C. O’Brien for useful discussions. We gratefully acknowledge funding from the Natural Science and Engineering Research Council of Canada (NSERC RGPIN-2014-06618, STPGP 494024–16), Canada Foundation for Innovation (CFI), Canada Research Chairs Program (CRC), Canadian Institute for Advanced Research (CIFAR), Alberta Innovates — Technology Futures (AITF) and the University of Alberta.
Author information
Authors and Affiliations
Contributions
The ATS memory approach was proposed by E.S. with feedback from K.H. and L.J.L. The project was supervised by L.J.L. and E.S. The ultracold atom apparatus was designed by L.J.L., and it was built and commissioned by L.J.L., T.H. and E.S. The design of the experiments, the measurements and the analysis of the results were performed by E.S. and T.H. The numerical modelling of the ATS memory was performed by K.H. with input from E.S. The simulations and numerical analysis were performed by E.S. and A.R. with the guidance of K.H. The manuscript was written by E.S. and L.J.L. with feedback from all co-authors.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary notes and figures.
Rights and permissions
About this article
Cite this article
Saglamyurek, E., Hrushevskyi, T., Rastogi, A. et al. Coherent storage and manipulation of broadband photons via dynamically controlled Autler–Townes splitting. Nature Photon 12, 774–782 (2018). https://doi.org/10.1038/s41566-018-0279-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41566-018-0279-0
This article is cited by
-
Fast, noise-free atomic optical memory with 35-percent end-to-end efficiency
Communications Physics (2023)
-
Coherent optical two-photon resonance tomographic imaging in three dimensions
Communications Physics (2023)
-
Slowing down x-ray photons in a vibrating recoilless resonant absorber
Scientific Reports (2022)
-
High-performance cavity-enhanced quantum memory with warm atomic cell
Nature Communications (2022)
-
Experimental investigation of light storage of diffraction-free and quasi-diffraction-free beams in hot atomic gas cell
Frontiers of Physics (2022)