Abstract
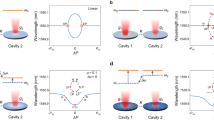
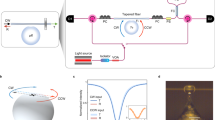
Non-Hermitian physical systems have attracted considerable attention lately for their unconventional behaviour around exceptional points (EPs)—spectral singularities at which eigenvalues and eigenvectors coalesce. In particular, many new EP-related concepts such as unidirectional lasing and invisibility, as well as chiral transmission, have been realized. Given the progress in understanding the physics of EPs in various photonic structures, it is surprising that one of the oldest theoretical predictions associated with them, a remarkable broadening of the laser linewidth at an EP, has been probed only indirectly so far. Here, we fill this gap by steering a phonon laser through an EP in a compound optomechanical system formed by two coupled resonators. We observe a pronounced linewidth broadening of the mechanical lasing mode generated in one of the resonators when the system approaches the EP.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Bender, C. M. Making sense of non-Hermitian Hamiltonians. Rep. Prog. Phys. 70, 947–1018 (2007).
Moiseyev, N. Non-Hermitian Quantum Mechanics (Cambridge Univ. Press, Cambridge, 2011).
Doppler, J. et al. Dynamically encircling an exceptional point for asymmetric mode switching. Nature 537, 76–79 (2016).
Dembowski, C. et al. Experimental observation of the topological structure of exceptional points. Phys. Rev. Lett. 86, 787–790 (2001).
Guo, A. et al. Observation of PT-symmetry breaking in complex optical potentials. Phys. Rev. Lett. 103, 093902 (2009).
Rüter, C. E. et al. Observation of parity–time symmetry in optics. Nat. Phys. 6, 192–195 (2010).
Klaiman, S., Günther, U. & Moiseyev, N. Visualization of branch points in PT-symmetric waveguides. Phys. Rev. Lett. 101, 080402 (2008).
Lin, Z. et al. Unidirectional invisibility induced by PT-symmetric periodic structures. Phys. Rev. Lett. 106, 213901 (2011).
Regensburger, A. et al. Parity–time synthetic photonic lattices. Nature 488, 167–171 (2012).
Peng, B. et al. Parity–time-symmetric whispering-gallery microcavities. Nat. Phys. 10, 394–398 (2014).
Chang, L. et al. Parity–time symmetry and variable optical isolation in active–passive-coupled microresonators. Nat. Photon. 8, 524–529 (2014).
Peng, B. et al. Chiral modes and directional lasing at exceptional points. Proc. Natl Acad. Sci. USA 113, 6845–6850 (2016).
Peng, B. et al. Loss-induced suppression and revival of lasing. Science 346, 328–332 (2014).
Feng, L., Wong, Z. J., Ma, R.-M., Wang, Y. & Zhang, X. Single-mode laser by parity–time symmetry breaking. Science 346, 972–975 (2014).
Hodaei, H., Miri, M.-A., Heinrich, M., Christodoulides, D. N. & Khajavikhan, M. Parity–time-symmetric microring lasers. Science 346, 975–978 (2014).
Wiersig, J. Enhancing the sensitivity of frequency and energy splitting detection by using exceptional points: application to microcavity sensors for single-particle detection. Phys. Rev. Lett. 112, 203901 (2014).
Liu, Z.-P. et al. Metrology with PT-symmetric cavities: enhanced sensitivity near the PT-phase transition. Phys. Rev. Lett. 117, 110802 (2016).
Chen, W., Özdemir, S. K., Zhao, G., Wiersig, J. & Yang, L. Exceptional points enhance sensing in an optical microcavity. Nature 548, 192–196 (2017).
Hodaei, H. et al. Enhanced sensitivity at higher-order exceptional points. Nature 548, 187–191 (2017).
Xu, H., Mason, D., Jiang, L. & Harris, G. E. Topological energy transfer in an optomechanical system with exceptional points. Nature 537, 80–83 (2016).
Wenzel, H., Bandelow, U., Wunsche, H. J. & Rehberg, J. Mechanisms of fast self pulsations in two-section DFB lasers. IEEE J. Quantum Electron. 32, 69–78 (1996).
Berry, M. Mode degeneracies and the Petermann excess-noise factor for unstable lasers. J. Mod. Opt. 50, 63–81 (2003).
Schawlow, A. L. & Townes, C. H. Infrared and optical masers. Phys. Rev. 112, 1940–1948 (1958).
Peterman, K. Calculated spontaneous emission factor for double-heterostructure injection lasers with gain-induced waveguiding. IEEE J. Quantum Electron. QE-15, 566–570 (1979).
Siegman, A. E. Excess spontaneous emission in non-Hermitian optical systems. I. Laser amplifiers. Phys. Rev. A 39, 1253–1263 (1989).
Grangier, P. & Poizat, J. P. A simple quantum picture for the Petermann excess noise factor. Eur. Phys. J. D 1, 97–104 (1998).
Hamel, W. A. & Woerdman, J. P. Observation of enhanced fundamental linewidth of a laser due to nonorthogonality of its longitudinal eigenmodes. Phys. Rev. Lett. 64, 1506 (1990).
Cheng, Y.-J., Fanning, C. G. & Siegman, A. E. Experimental observation of a large excess quantum noise factor in the linewidth of a laser oscillator having nonorthogonal modes. Phys. Rev. Lett. 77, 627–630 (1996).
van Eijkelenborg, M. A., Lindberg, Å. M., Thijssen, M. S. & Woerdman, J. P. Resonance of quantum noise in an unstable cavity laser. Phys. Rev. Lett. 77, 4314–4317 (1996).
Schomerus, H., Frahm, K. M., Patra, M. & Beenakker, C. W. J. Quantum limit of the laser line width in chaotic cavities and statistics of residues of scattering matrix poles. Phys. A 278, 469–496 (2000).
Lee, S.-Y. et al. Divergent Petermann factor of interacting resonances in a stadium-shaped microcavity. Phys. Rev. A 78, 015805 (2008).
Yoo, G., Sim, H.-S. & Schomerus, H. Quantum noise and mode nonorthogonality in non-Hermitian PT-symmetric optical resonators. Phys. Rev. A 84, 063833 (2011).
Schomerus, H. Excess quantum noise due to mode nonorthogonality in dielectric microresonators. Phys. Rev. A 79, 061801(R) (2009).
Chong, Y. D. & Douglas Stone, A. General linewidth formula for steady-state multimode lasing in arbitrary cavities. Phys. Rev. Lett. 109, 063902 (2012).
Pick, A. et al. Ab initio multimode linewidth theory for arbitrary inhomogeneous laser cavities. Phys. Rev. A 91, 063806 (2015).
Liertzer, M. et al. Pump-induced exceptional points in lasers. Phys. Rev. Lett. 108, 173901 (2012).
Brandstetter, M. et al. Reversing the pump dependence of a laser at an exceptional point. Nat. Commun. 5, 4034 (2014).
Grudinin, I. S., Lee, H., Painter, O. & Vahala, K. J. Phonon laser action in a tunable two-level system. Phys. Rev. Lett. 104, 083901 (2010).
Jing, H. et al. PT-symmetric phonon laser. Phys. Rev. Lett. 113, 053604 (2014).
Cartarius, H., Main, J. & Wunner, G. Exceptional points in atomic spectra. Phys. Rev. Lett. 99, 173003 (2007).
Acknowledgements
This work was supported by NSF grant no. EFMA1641109, ARO grant no. W911NF1210026, ARO grant no. W911NF1710189 and the European Commission under project NHQWAVE (MSCA-RISE 691209). S.K.O. was supported by ARO grant no. W911NF-16-1-0339. S.K.O thanks J. Mateo for his continuous support. J.Z. is supported by the NSFC under grant nos. 61622306, 11674194. Y.-X.L. is supported by the NSFC under grant no. 61025022. Y.-X.L. and J.Z. are supported by the National Basic Research Program of China (973 Program) under grant no. 2014CB921401, the Tsinghua University Initiative Scientific Research Program and the Tsinghua National Laboratory for Information Science and Technology (TNList) Cross-discipline Foundation. F.N. is partially supported by the MURI Center for Dynamic Magneto-Optics via AFOSR Award no. FA9550-14-1-0040, Asian Office of Aerospace Research and Development (AOARD) (grant no. FA2386-18-1-4045), the IMPACT program of JST, JSPS-RFBR grant no. 17-52-50023, CREST grant no. JPMJCR1676, RIKEN-AIST Joint Research Fund and the Sir John Templeton Foundation. K.P., D.O.K. and S.R. are supported by the Austrian Science Fund (FWF) through project no. SFB NextLite F49-P10. H. Yilmaz prepared the chromium-coated silica nanofibre tip for the experiments.
Author information
Authors and Affiliations
Contributions
S.R., S.K.O, B.P. and L.Y. conceived the idea. B.P., J.Z., S.K.O., S.R. and L.Y. designed the experiments. J.Z and B.P. performed the experiments with help from G.Z. J.Z. analysed the experimental data, J.Z., K.P. and D.O.K. performed the theoretical analysis and numerical simulations, guided by S.K.O, Y.-X.L. and S.R. J.Z., S.K.O., S.R., Y.-X.L. and L.Y. wrote the manuscript with contributions from all authors. L.Y. supervised the research.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary Information
Supplementary Information
Supplementary discussion and experimental details; Supplementary Figures 1–9; Supplementary References 1–15.
Rights and permissions
About this article
Cite this article
Zhang, J., Peng, B., Özdemir, Ş.K. et al. A phonon laser operating at an exceptional point. Nature Photon 12, 479–484 (2018). https://doi.org/10.1038/s41566-018-0213-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41566-018-0213-5
This article is cited by
-
Floquet parity-time symmetry in integrated photonics
Nature Communications (2024)
-
Tracking exceptional points above the lasing threshold
Nature Communications (2023)
-
Nonlinear multi-frequency phonon lasers with active levitated optomechanics
Nature Physics (2023)
-
Scattering exceptional point in the visible
Light: Science & Applications (2023)
-
Theoretical investigation of dynamics and concurrence of entangled \({{\mathcal {P}}}{{\mathcal {T}}}\) and anti-\({{\mathcal {P}}}{{\mathcal {T}}}\) symmetric polarized photons
Scientific Reports (2023)