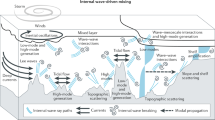
Abstract
Oceanic mesoscale structures such as eddies and fronts can alter the propagation, breaking and subsequent turbulent mixing of wind-generated internal waves. However, it has been difficult to ascertain whether these processes affect the global-scale patterns, timing and magnitude of turbulent mixing, thereby powering the global oceanic overturning circulation and driving the transport of heat and dissolved gases. Here we present global evidence demonstrating that mesoscale features can significantly enhance turbulent mixing due to wind-generated internal waves. Using internal wave-driven mixing estimates calculated from Argo profiling floats between 30° and 45° N, we find that both the amplitude of the seasonal cycle of turbulent mixing and the response to increases in the wind energy flux are larger to a depth of at least 2,000 m in the presence of a strong and temporally uniform field of mesoscale eddy kinetic energy. Mixing is especially strong within energetic anticyclonic mesoscale features compared to cyclonic features, indicating that local modification of wind-driven internal waves is probably one mechanism contributing to the elevated mixing observed in energetic mesoscale environments.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$259.00 per year
only $21.58 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Alford, M. H., MacKinnon, J. A., Simmons, H. L. & Nash, J. D. Near-inertial internal gravity waves in the ocean. Annu. Rev. Mar. Sci. 8, 95–123 (2016).
MacKinnon, J. A. et al. Climate process team on internal-wave driven ocean mixing. Bull. Am. Meteorol. Soc. 98, 2429–2454 (2017).
Rimac, A., Storch, J.-S., Eden, C. & Haak, H. The influence of high-resolution wind stress field on the power input to near-inertial motions in the ocean. Geophys. Res. Lett. 40, 4882–4886 (2013).
Jochum, M. et al. The impact of oceanic near-inertial waves on climate. J. Clim. 26, 2833–2844 (2013).
Weller, R. A. The relation of near-inertial motions observed in the mixed layer during the JASIN (1978) experiment to the local wind stress and to the quasi-geostrophic flow field. J. Phys. Oceanogr. 12, 1122–1136 (1982).
Kunze, E., Schmitt, R. W. & Toole, J. M. Near-inertial wave-propagation in geostrophic shear. J. Phys. Oceanogr. 15, 544–565 (1985).
Young, W. & Jelloul, M. B. Propagation of near-inertial oscillations through a geostrophic flow. J. Mar. Res. 55, 735–766 (1997).
Klein, P., Smith, S. L. & Lapeyre, G. Organization of near-inertial energy by an eddy field. Q. J. R. Meteorol. Soc. 130, 1153–1166 (2004).
Müller, P. On the diffusion of momentum and mass by internal gravity waves. J. Fluid. Mech. 77, 789–823 (1976).
Bühler, O. & McIntyre, M. E. Wave capture and wave-vortex duality. J. Fluid. Mech. 534, 67–95 (2005).
Polzin, K. L. Mesoscale eddy-internal wave coupling. Part II: energetics and results from PolyMode. J. Phys. Oceanogr. 40, 789–801 (2010).
Polzin, K. L. & Lvov, Y. V. Toward regional characterizations of the oceanic internal wavefield. Rev. Geophys. 49, RG4003 (2011).
Mooers, C. N. Several effects of a baroclinic current on the cross-stream propagation of inertial-internal waves. Geophys. Astro. Fluid. 6, 245–275 (1975).
Whitt, D. & Thomas, L. Near-inertial waves in strongly baroclinic currents. J. Phys. Oceanogr. 43, 706–725 (2013).
Whalen, C. B., Talley, L. D. & MacKinnon, J. A. Spatial and temporal variability of global ocean mixing inferred from Argo profiles. Geophys. Res. Lett. 39, L18612 (2012).
Lueck, R. & Osborn, T. The dissipation of kinetic energy in a warm-core ring. J. Geophys. Res. Oceans 91, 803–818 (1986).
Padman, L., Levine, M., Dillon, T., Morison, J. & Pinkel, R. Hydrography and microstructure of an Arctic Cyclonic Eddy. J. Geophys. Res. Oceans 95, 9411–9420 (1990).
Kunze, E., Schmitt, R. W. & Toole, J. M. The energy balance in a warm-core ring’s near-inertial critical layer. J. Phys. Oceanogr. 25, 942–957 (1995).
Sheen, K. L. et al. Modification of turbulent dissipation rates by a deep Southern Ocean eddy. Geophys. Res. Lett. 42, 3450–3457 (2015).
Osborn, T. R. Estimates of the local-rate of vertical diffusion from dissipation measurements. J. Phys. Oceanogr. 10, 83–89 (1980).
Whalen, C. B., MacKinnon, J. A., Talley, L. D. & Waterhouse, A. F. Estimating the mean diapycnal mixing using a finescale strain parameterization. J. Phys. Oceanogr. 45, 1174–1188 (2015).
Henyey, F. S., Wright, J. & Flatte, S. M. Energy and action flow through the internal wave feild: an eikonal approach. J. Geophys. Res. Oceans 91, 8487–8495 (1986).
Gregg, M. & Kunze, E. Shear and strain in Santa Monica basin. J. Geophys. Res. Oceans 96, 16709–16719 (1991).
Polzin, K. L., Toole, J. M. & Schmitt, R. W. Finescale parameterizations of turbulent dissipation. J. Phys. Oceanogr. 25, 306–328 (1995).
Kunze, E., Firing, E., Hummon, J. M., Chereskin, T. K., & Thurnherr, A. M. Global abyssal mixing inferred from lowered ADCP shear and CTD strain profiles. J. Phys. Oceanogr. 36, 1553–1576 (2006).
Polzin, K. L., Naveira Garabato, A. C., Huussen, T. N., Sloyan, B. M. & Waterman, S. N. Finescale parameterizations of turbulent dissipation. J. Geophys. Res. Oceans 119, 1383–1419 (2014).
Alford, M., Cronin, M. & Klymak, J. Annual cycle and depth penetration of wind-generated near-inertial internal waves at Ocean Station Papa in the Northeast Pacific. J. Phys. Oceanogr. 42, 889–909 (2012).
Alford, M. H. & Whitmont, M. Seasonal and spatial variability of near-inertial kinetic energy from historical moored velocity records. J. Phys. Oceanogr. 37, 2022–2037 (2007).
Silverthorne, K. E. & Toole, J. M. Seasonal kinetic energy variability of near-inertial motions. J. Phys. Oceanogr. 39, 1035–1049 (2009).
Zhai, X., Greatbatch, R. J. & Eden, C. Spreading of near-inertial energy in a 1/12 model of the North Atlantic Ocean. Geophys. Res. Lett. 34, L10609 (2007).
Dunphy, M, & Lamb, K. G. Focusing and vertical mode scattering of the first mode internal tide by mesoscale eddy interaction. J. Geophys. Res. Oceans 119, 523–536 (2014).
Kerry, C. G., Powell, B. S. & Carter, G. S. The impact of subtidal circulation on internal tide generation and propagation in the Philippine Sea. J. Phys. Oceanogr. 44, 1386–1405 (2014).
Vanneste, J. Balance and spontaneous wave generation in geophysical flows. Ann. Rev. Fluid Mech. 45, 147–172 (2013).
Danioux, E., Vanneste, J., Klein, P. & Sasaki, H. Spontaneous inertia-gravity-wave generation by surface-intensified turbulence. J. Fluid. Mech. 699, 153–173 (2012).
Reeder, M. J. & Griffiths, M. Stratospheric inertia-gravity waves generated in a numerical model of frontogenesis II: wave sources, generation mechanisms and momentum fluxes.Q. J. R. Meteorol. Soc 122, 1175–1195 (1996).
Alford, M. H., MacKinnon, J. A., Pinkel, R. & Klymak, J. M. Space-time scales of shear in the North Pacific. J. Phys. Oceanogr. 47, 2455–2478 (2017).
Nikurashin, M. & Ferrari, R. Global energy conversion rate from geostrophic flows into internal lee waves in the deep ocean. Geophys. Res. Lett. 38, L08610 (2011).
Xie, J.-H. & Vanneste, J. A generalised-Lagrangian-mean model of the interactions between near-inertial waves and mean flow. J. Fluid. Mech. 774, 143–169 (2015).
Wagner, G. & Young, W. Available potential vorticity and wave-averaged quasi-geostrophic flow. J. Fluid. Mech. 785, 401–424 (2015).
Danioux, E., Klein, P. & Rivière, P. Propagation of wind energy into the deep ocean through a fully turbulent mesoscale eddy field. J. Phys. Oceanogr. 38, 2224–2241 (2008).
Elipot, S., Lumpkin, R. & Prieto, G. Modification of inertial oscillations by the mesoscale eddy field. J. Geophys. Res. Oceans 115, C09010 (2010).
Jones, W. L. Ray tracing for internal gravity waves. J. Geophys. Res. 74, 2028–2033 (1969).
Polzin, K. L. Mesoscale eddy-internal wave coupling. Part I: Symmetry, wave capture, and results from the mid-ocean dynamics experiment. J. Phys. Oceanogr. 38, 2556–2574 (2008).
Billheimer, S. & Talley, L. D. Annual cycle and destruction of eighteen degree water. J. Geophys. Res. Oceans 121, 6604–6617 (2016).
Pollard, R. T. & Millard, R. C. Comparison between observed and simulated wind-generated inertial oscillations. In Deep Sea Research and Oceanographic Abstracts Vol. 17, 813–821 (Elsevier, 1970).
D’Asaro, E. A. The energy flux from the wind to near-inertial motions in the surface mixed layer. J. Phys. Oceanogr. 15, 1043–1059 (1985).
Alford, M. H. Internal swell generation: The spatial distribution of energy flux from the wind to mixed layer near-inertial motions. J. Phys. Oceanogr. 31, 2359–2368 (2001).
Plueddemann, A. & Farrar, J. Observations and models of the energy flux from the wind to mixed-layer inertial currents. Deep-Sea Res. Pt II 53, 5–30 (2006).
Van Meurs, P. Interactions between near-inertial mixed layer currents and the mesoscale: The importance of spatial variabilities in the vorticity field. J. Phys. Oceanogr. 28, 1363–1388 (1998).
Whitt, D. B. & Thomas, L. N. Resonant generation and energetics of wind-forced near-inertial motions in a geostrophic flow. J. Phys. Oceanogr. 45, 181–208 (2015).
Park, J. J., Kim, K. & Schmitt, R. W. Global distribution of the decay timescale of mixed layer inertial motions observed by satellite-tracked drifters. J. Geophys. Res. Oceans 114, C11010 (2009).
D’Asaro, E. A. et al. Upper-ocean inertial currents forced by a strong storm. I: Data and comparisons with linear-theory. J. Phys. Oceanogr. 25, 2909–2936 (1995).
Lumpkin, R. & Garraffo, Z. Evaluating the decomposition of tropical Atlantic drifter observations. J. Atmos. Oceanic Technol. 22, 1403–1415 (2005).
Arbic, B. K., Scott, R. B., Chelton, D. B., Richman, J. G. & Shriver, J. F. Effects of stencil width on surface ocean geostrophic velocity and vorticity estimation from gridded satellite altimeter data. J. Geophys. Res. Oceans 117, C03029 (2012).
Acknowledgements
The authors are grateful for the support of NSF OCE-1259573 and for valuable comments from K. Polzin and E. Kunze.
Author information
Authors and Affiliations
Contributions
C.B.W conceived of the study, conducted the analysis, and wrote the manuscript. Both J.A.M and L.D.T. contributed to the analysis and writing of the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Whalen, C.B., MacKinnon, J.A. & Talley, L.D. Large-scale impacts of the mesoscale environment on mixing from wind-driven internal waves. Nature Geosci 11, 842–847 (2018). https://doi.org/10.1038/s41561-018-0213-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41561-018-0213-6