Introduction

Superconductivity with coexisting electronic orders can be found in various strongly correlated systems. Among these orders electron nematicy, where the electron fluid breaks the discrete rotational symmetry of the underlying lattice, has recently emerged as an ubiquitous phase in many superconductors ranging from cuprates1, to iron-based superconductors2 where superconductivity emerges within a nematic phase, and more recently doped Bi2Se33 and twisted bi-layer graphene4,5 where the superconductivity itself may have a nematic component. In iron-based superconductors (Fe SC), superconductivity is found to coexist with both stripe-like magnetic spin-density-wave (SDW) and nematic orders. BaFe2As2, a member of this family, undergoes a nematic-structural transition from a C4 to a C2 symmetric phase, followed by a SDW transition2,6. The C4 rotational symmetry breaking is triggered by electronic degrees of freedom and has been dubbed nematic for this reason2,7,8. With increasing doping by substitution (e.g., Ba with K or Fe with Co6,9), the C2 symmetric nematic-SDW phase, hereafter called the C2 phase, is weakened and a superconducting (SC) dome forms around a possible quantum critical point. The coexistence with the C2 phase can profoundly impact the nature of SC order, by coupling different nearly degenerate pairing channels like s and d-wave10,11, or inducing an orbitally-selective SC state12,13.

One way to gain insight into the coupling between nematic and SC degrees of freedom is to study the collective modes of the SC state upon entering the C2 SC phase. Theoretically, intertwined electronic orders where superconductivity coexists with other electronic orders can lead to a rich spectrum of SC collective modes14,15,16,17,18,19,20,21,22,23,24. In a single band conventional superconductor the collective mode excitation spectrum consists of two modes: the Nambu-Goldstone phase mode which is shifted to the plasma frequency through the Anderson–Higgs mechanism, and the Higgs amplitude mode located at twice the SC gap energy. The Higgs mode does not couple linearly to light14,25,26. Except in very special cases like charge-density-wave superconductors27,28,29,30, its observation has remained elusive until very recently. In this context, strong terahertz (THz) pulses have emerged as a tool of choice because they can access hidden SC collective modes via nonlinear optical processes31,32,33,34,35,36,37. This has led to the observation of the SC Higgs mode in several SC materials like NbN and Nb3Sn, but also in cuprates and Fe SC38,39,40,41,42,43,44,45. In the case of Fe SC however, little is known experimentally about the impact of nematicity on SC collective modes like the Higgs.

Here we investigate the THz nonlinear response of the Fe SC Ba1−xKxFe2As2 where superconductivity coexists with a nematic order using a THz pump near-infrared (NIR) probe scheme. In the SC state, we observe an instantaneous response which follows the square of the THz electric field which is assigned to the nonlinear THz Kerr effect. In the absence of a coexisting nematic order the THz Kerr signal displays a C4 symmetric polarization dependence consistent with a nonlinear coupling to the SC Higgs mode. In the presence of a coexisting nematic order, the THz Kerr signal displays a drastic change in its polarization dependence: from fully symmetric in the C4 symmetric SC phase to fully nematic in the C2 symmetric phase. We show theoretically that the onset of the THz Kerr Higgs response in the nematic channel can be qualitatively explained by taking into account the anisotropy of the electronic structure in the C2 nematic phase. However, the complete disappearance of the C4 symmetric signal in the C2 SC phase cannot be captured within this simple picture, indicating a non-trivial interplay between the nematic and superconducting order parameters and the emergence of a collective mode, distinct from the Higgs mode. We tentatively assigned this mode to the Bardasis-Schrieffer (BS) mode connecting s-wave and d-wave superconducting ground states which become mixed in the C2 symmetric SC phase.

Results

Non-linear THz Kerr effect

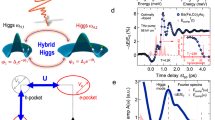
We studied two single crystals of Ba1−xKxFe2As2 with Tc = 26K (UD26) and Tc = 37 K (UD37). The UD26 crystal is slightly underdoped and exhibits a simultaneous nematic/SDW transition at TN ~ TS ~ 90 K. The UD37 crystal only exhibits a superconducting transition and is close to optimal doping. The terahertz-pump optical reflectivity probe (TPOP) measurement scheme is depicted in Fig. 1a. Measurements were carried out with a fixed THz pump polarization along the Fe–Fe direction but two different probe polarizations either parallel or perpendicular to the pump polarization (Fig. 1b). Fe–Fe directions are identified by a 45∘ tilt with respect to the edges of the crystals which are square-shaped.

Fig. 1: Nonlinear THz response in Ba1−xKxFe2As2.
figure 1

a Sketch of the THz pump near-infrared (NIR) probe measurements. b Crystal structure of BaFe2As2 and the two polarization configurations used to determine the C4 symmetric and nematic components of the transient reflectivity. Raman spectra of Ba1−xKxFe2As2 in the B1g symmetry for UD37 (c) and UD26 (d) below Tc. 2Δ indicates superconducting gap from the hole pockets. The gray curve represents the energy spectrum of the THz pump. e For UD37, ΔR/R (blue line) along one of the Fe–Fe axis at 20 K (T < Tc) as a function of delay time between the pump and probe pulses. The instantaneous component that follows \({E}_{{{{\rm{pump}}}}}^{2}\) (red line) component corresponds to the THz Kerr effect.

Full size image

In Fig. 1c, d, we compare the THz pump spectrum with the SC state Raman spectra of the two samples (See Supplementary Note 1 for more details). With an energy centered around ωp = 0.6 THz = 20 cm−1, the THz pump spectrum is located below the lowest superconducting gap 2Δh observed by Raman scattering. Based on previous Raman and angle-resolved photoemission spectroscopy measurements this gap is assigned to the Γ centered hole pockets. The TPOP signal \(\frac{{{\Delta }}R}{R}\) of UD37 below Tc is shown in Fig. 1e. It consists of essentially two components, an instantaneous component that follows the square of the THz electric field (E-field) (red line in Fig. 1e) and a broader decaying component which last several picoseconds after the pump pulse. In the following we will mostly focus on the instantaneous component, the THz Kerr effect, where the strong THz E-field modulates the optical reflectivity in the NIR regime46. We note that in our measurements we only detect an instantaneous component that is proportional to the square of the THz E-field, consistent with the centrosymmetric crystal structure of Ba1−xKxFe2As2. No forbidden odd contribution is observed, as recently reported in the SC state of Nb3Sn47 and attributed to THz field symmetry breaking.

The THz Kerr signal is described by a third-order nonlinear susceptibility χ(3)(ω; ω, + Ω, −Ω)48,49, where ω and Ω are the frequencies of the NIR pulse and THz-pump pulse, respectively. The THz pulse-induced reflectivity change ΔR/R can be expressed in terms of χ(3) (see Supplementary Note 3) as:

$$\frac{{{\Delta }}R}{R}({E}_{i}^{{{{\rm{probe}}}}},{E}_{j}^{{{{\rm{probe}}}}}) \sim \frac{1}{R}\frac{\delta R}{\delta {\epsilon }_{1}}{\epsilon }_{0}[Re{\chi }_{ijkl}^{(3)}]{E}_{k}^{{{{\rm{pump}}}}}{E}_{l}^{{{{\rm{pump}}}}}$$
(1)

where Ei denotes the ith component of the THz-pump or probe E-field and ϵ1 is the real part of the dielectric constant at 1.5 eV. The instantaneous Kerr signal of interest here implies Ω = 0 in χ(3). It is therefore independent of the pump frequency Ω and non-resonant42,49. This is in contrast with the third-harmonic generation (THG) signal which is resonant when the pump frequency Ω equals the superconducting gap Δ31.

In general, the onset of a THz Kerr signal below Tc can be assigned to two different processes: coupling to charge density fluctuations (CDF) like the one observed in Raman experiments, or to the SC Higgs mode. As previously shown in the case of NbN and cuprates important clues about the origin of the THz Kerr signal, and other third-order nonlinear effects like THG, can be obtained by investigating its polarization dependence32,35,36,40,42,43.

Assuming C4 tetragonal symmetry for the normal state of Ba1−xKxFe2As2, we can analyze the polarization dependence of χ(3)(θpump, θprobe) in terms of the irreducible representations of D4h point group as:

$$\begin{array}{l}{\chi }^{(3)}({\theta }_{{{{\rm{pump}}}}},{\theta }_{{{{\rm{probe}}}}})=\frac{1}{2}\left({\chi }_{{{{{\rm{A}}}}}_{1{{{\rm{g}}}}}}^{(3)}+{\chi }_{{{{{\rm{B}}}}}_{1{{{\rm{g}}}}}}^{(3)}\cos 2{\theta }_{{{{\rm{pump}}}}}\cos 2{\theta }_{{{{\rm{probe}}}}}\right.\\ \left.+\,{\chi }_{{{{{\rm{B}}}}}_{2{{{\rm{g}}}}}}^{(3)}\sin 2{\theta }_{{{{\rm{pump}}}}}\sin 2{\theta }_{{{{\rm{probe}}}}}\right)\end{array}$$
(2)

where we have defined the symmetry-resolved nonlinear response functions: \({\chi }_{{{{{\rm{A}}}}}_{1{{{\rm{g}}}}}}^{(3)}={\chi }_{{{{\rm{aaaa}}}}}^{(3)}+{\chi }_{{{{\rm{bbaa}}}}}^{(3)}\), \({\chi }_{{{{{\rm{B}}}}}_{1{{{\rm{g}}}}}}^{(3)}={\chi }_{{{{\rm{aaaa}}}}}^{(3)}-{\chi }_{{{{\rm{bbaa}}}}}^{(3)}\) and \({\chi }_{{{{{\rm{B}}}}}_{{{{\rm{2g}}}}}}^{(3)}={\chi }_{{{{\rm{abab}}}}}^{(3)}+{\chi }_{{{{\rm{abba}}}}}^{(3)}\). and θprobe/pump are the angles between the probe/pump polarization vectors and the a axis of the one Fe unit cell. The A1g is the fully symmetric representation and the B1g/B2g representation transform as x2 − y2 and xy, respectively. The B1g representation has the same symmetry as the C2 symmetric nematic order parameter found in Fe SC. For θpump = 0, the A1g and B1g responses can be accessed using two distinct probe polarization orientations. Indeed making use of Eq. (1) we can write:

$$\begin{array}{l}{\frac{{{\Delta }}R}{R}}^{{{{{\rm{C}}}}}_{4}}=\frac{{{\Delta }}{R}_{{{{\rm{a}}}}}}{{R}_{{{{\rm{a}}}}}}+\frac{{{\Delta }}{R}_{{{{\rm{b}}}}}}{{R}_{{{{\rm{b}}}}}}\propto \scriptsize{Re{\chi }_{{{{{\rm{A}}}}}_{1{{{\rm{g}}}}}}^{(3)}}\\ {\frac{{{\Delta }}R}{R}}^{{{{\rm{nem}}}}}=\frac{{{\Delta }}{R}_{{{{\rm{a}}}}}}{{R}_{{{{\rm{a}}}}}}-\frac{{{\Delta }}{R}_{{{{\rm{b}}}}}}{{R}_{{{{\rm{b}}}}}}\propto \scriptsize{Re{\chi }_{{{{{\rm{B}}}}}_{1{{{\rm{g}}}}}}^{(3)}}\end{array}$$
(3)

where Ri (i = a, b) denotes the reflectivity for a probe polarization along the Fe–Fe axes (a, b) of Fig. 1b and for a fixed pump polarization along the a axis. Here, we have taken Ra = Rb (C4 tetragonal symmetry). Since the notations B1g and A1g are no longer valid in the C2 symmetric orthorhombic phase, we will adopt the notation “C4” for C4 symmetric and “nem” for nematic (or C2 symmetric) when discussing the results below.

Response in the C4 symmetric superconducting state

We start by discussing the C4 symmetric and nematic components of the TPOP signal of the UD37 crystal for which only superconductivity is present. Figure 2a, b show the transient reflectivity obtained for both C4 symmetric and nematic components in the UD37 crystal and at various temperatures ranging from 15 K to 70 K. The decaying part of the ΔR/R signal shows a strong increase in the C4 symmetric channel across Tc indicating the superconducting transition (Inset of Fig. 3a). The instantaneous Kerr component is only observed below Tc, confirming it is linked to the onset of superconductivity. On the other hand, in the nematic channel no significant changes appear in the transient reflectivity at all temperatures. Using the fitting procedure displayed in Fig. 2e, g, we can obtain the temperature dependencies of the instantaneous and decaying components of \({\frac{{{\Delta }}R}{R}}^{{{{{\rm{C}}}}}_{4}}\) and \({\frac{{{\Delta }}R}{R}}^{{{{\rm{nem}}}}}\) (Fig. 3a). The decaying component displays a sharp extremum around Tc and is assigned to the dynamical relaxation of quasi-particles in the SC state (see Supplementary Note 2). The instantaneous component, attributed to the THz Kerr effect, shows a strong enhancement below Tc. The absence of the instantaneous Kerr component in the nematic channel argues in favor of a contribution arising from the Higgs excitation. Indeed, as shown in the case of Bi2Sr2CaCu2O8+x cuprates CDF are expected to contribute to all symmetry channels whereas the Higgs contribution is only active in the fully symmetric, i.e., C4 symmetric for a tetragonal crystal, channel35,36,40,42,50. Interestingly, Raman scattering spectra on the same crystal in the SC state are dominated by the B1g channel11 (see Supplementary Fig. 1), indicating they mostly probe CDF contributions in stark contrast with the THz Kerr signal. We note that the respective weight between Higgs and CDF contributions to third-order nonlinear susceptibilities has been a subject of a debate since BCS calculations indicate dominant CDF contributions for a clean superconductor32. However, there is now an emerging consensus that disorder significantly boosts the Higgs contribution, thus giving a rationale to the dominance of the Higgs contribution observed in THz Kerr and THG experiments in all superconductors studied so far33,35,36,51,52.

Fig. 2: Temperature and symmetry dependence of the THz response.
figure 2

ΔR/R against the delay time in the C4 symmetric (C4) and nematic (Nem) channels at various temperatures for UD37 (a, b) and UD26 (c, d). Fitted curves for the different components of ΔR/R at T < Tc for UD37 (e, g) and UD26 (f, h).

Full size image
Fig. 3: Instantaneous Kerr response.
figure 3

Temperature dependencies of the amplitude of the fitted instantaneous Kerr signals in the C4 symmetric (C4) and nematic (nem) channels for UD37 (a) and UD26 (b). Insets: Temperature dependencies of the amplitude of the fitted SC decaying signals. Calculated temperature dependence of Higgs contribution of the hole pockets to the instantaneous Kerr signals in the C4 symmetric (C4) and nematic (nem) channels for a tetragonal (c) and orthorombic (d) symmetry of the electronic dispersion relation. The shape of the Fermi surfaces of our model is represented in blue and yellow. Inset: Ratio of the nematic and C4 symmetric components of the Higgs response as a function of the nematic order parameter for the hole (blue) and electron pockets (red). Error bars are defined as the standard deviation.

Full size image

Response in the C2 symmetric superconducting state

Having discussed the simple case of the C4 symmetric superconductor case, let us now turn to the sample with a lower doping level, UD26 which display a C2 symmetric SC phase with both nematic and SC orders. Figure 2c, d show the transient reflectivity obtained for both channels and at various temperatures ranging from 9.5 K to 110 K. Above Tc, in contrast to UD37, both C4 symmetric and nematic components show a change in the transient reflectivity below TS/N ~ 90 K indicating the transition to the C2 symmetric nematic phase. The onset of a decaying signal in the nematic channel is consistent with optical pump optical probe measurements on BaFe2(As1−xPx)2 which reported a similar strongly anisotropic signal below TS/N53. In principle, a mixture of C2 domains of different orientation would average out the nematic component of our signal. The fact that we observe a significant non-zero \({\frac{{{\Delta }}R}{R}}^{{{{\rm{nem}}}}}\) shows that one domain orientation prevails under our 250 μm laser spot. We attribute this relatively large domain size to residual strains on the sample due to sample mounting which act as symmetric breaking field and align the nematic domains.

Below Tc, an instantaneous Kerr component of ΔR/R that follows the squared THz-pump E-field is also identified, with however a striking difference compared to UD37. Indeed, while it is essentially absent in \({\frac{{{\Delta }}R}{R}}^{{{{{\rm{C}}}}}_{4}}\), the instantaneous Kerr signal shows a strong enhancement below Tc in the \({\frac{{{\Delta }}R}{R}}^{{{{\rm{nem}}}}}\) channel. Using the fitting procedure displayed in Fig. 2f, h, we obtained the amplitude of the instantaneous Kerr and decaying components (see Fig. 3b and Supplementary Fig. 2). Interestingly, the channel dependencies of the instantaneous Kerr and decaying signal are distinct: while the instantaneous Kerr signal is fully nematic with no C4 symmetric component within our experimental accuracy, the decaying signal is present in both channels with similar amplitudes at all temperatures.

Origin of the nematic response

The complete switch from C4 symmetric to nematic channel of the instantaneous Kerr signal when going from the C4 SC phase to the C2 SC phase is the central finding of the present work. It indicates an unanticipated and profound impact of the C4 symmetry breaking on the THz Kerr nonlinear optical signal of the SC state. We now explore different scenarios to explain this phenomena. First since the structural transition from tetragonal to orthorhombic involves a mixing of the A1g and B1g symmetry into the Ag symmetry, we naturally expect some mixing of the \(\frac{{{\Delta }}R}{R}\) symmetry components due to the anisotropy of the optical constants. Based on optical measurements on detwinned BaFe2As2 samples54 (see Supplementary Note 4), we determined quantitatively how the two symmetries are mixed from the calculation of the \(\frac{1}{R}\frac{\delta R}{\delta {\epsilon }_{1}}\) pre-factor in Eq. (1). We found at most a 25% anisotropy with respect to the a and b axes. As expected this anisotropy causes a non-zero nematic component. However, it leads to a \({\frac{{{\Delta }}R}{R}}^{{{{\rm{nem}}}}}\) signal of at most 10% of the \({\frac{{{\Delta }}R}{R}}^{{{{{\rm{C}}}}}_{4}}\) signal, and therefore plays a marginal role in the C4 symmetric to nematic transition observed in the THz Kerr signal.

Having ruled out a simple effect of anisotropic linear optical constant, we are left with the properties of the nonlinear response χ(3) itself. In the C2 phase, the anisotropy of the electronic dispersion relation will also induce a non-zero component of the Higgs mode response in the nematic channel37. We evaluated the activation of the Higgs response in the nematic channel by calculating the third-order nonlinear Higgs response (see Fig. 3c, d for the contribution of the hole pockets) using a three pocket model (2 hole-like and 1 electron-like) and an s-wave superconducting gap (see Supplementary Note 3 and 4). As expected, in the C4 phase the Higgs response appears below Tc only in the C4 symmetric channel in agreement with our observations in the UD37 sample. In the C2 phase however, the distorted Fermi pockets due to finite nematic order parameter activate the Higgs mode in the nematic channel as observed experimentally. The activation grows with the nematic splitting energy Δnem, but quickly saturates and decreases (see inset of Fig. 3d). We found that for any realistic nematic splitting energy and band parameters, the nematic response of either hole or electron pockets is at most 60% of the C4 symmetric response, thus failing to explain the experimental observation. We note that a dominant contribution from CDF to the THz Kerr signal would be inconsistent with both the fully C4 symmetric Kerr signal observed in UD37 and the fully nematic Kerr signal observed in UD26 (see Supplementary Note 5 for an evaluation of the CDF contribution).

Discussion

From the above discussion, it appears that the strong dominance of the THz Kerr signal in the nematic channel of UD26 cannot be explained simply by the effect of the anisotropy of the optical constant or the electronic structure on the Higgs signal. We are thus left with more speculative scenarios. First, we discuss the possibility of an exotic SC order parameter in the C2 phase. We note that an SC order parameter with lower symmetry like d-wave will not by itself activate a Higgs Kerr signal in non-fully symmetric channels as demonstrated in the case of cuprates37,42, so that our observations cannot be easily linked to a change in SC gap symmetry at least for a single band superconductor. However, in multi-orbital systems like Fe SC it is possible that the internal structure of Cooper pairs in orbital space profoundly affects the anisotropy of the Kerr Higgs signal. An intriguing possibility is the recent proposal of an orbital-selective SC state in the C2 phase of FeSe13. Whether such state would by itself yield a Higgs signal in the nematic channel only is unclear and deserves further theoretical investigations.

Another possibility is that the THz Kerr signal arises from an SC collective mode which couples to the nematic order parameter. In Ba1−xKxFe2As2 s and d-wave pairing channels are close competitors, potentially giving rise to a BS mode in the nematic d wave channel10,55,56. Several spectral features of the Raman spectrum of Ba1−xKxFe2As2 have indeed been interpreted as BS modes, consistent with theoretical evaluations of pairing instabilities in hole-doped BaFe2As210,11,57,58 (see also Supplementary Note 1 for a discussion of the Raman spectra in the SC state). Recently, Muller et al. have argued that in the C2 phase the BS mode will couple to the amplitude mode of the nematic order parameter, giving rise to a single coupled nematic-BS mode below the Higgs mode energy due to the appearance of a strongly mixed s+d SC state24. The stronger decaying signal in the UD26 sample compared to the UD37 sample well-below Tc supports the idea of an increased anisotropy of the SC gap in the C2 SC phase in agreement with a significant d wave admixture. Interestingly, for parameters close to the critical point where the nematic phase terminates Muller et al. found that the coupled nematic-BS mode may become dominant over the Higgs mode in the short-time dynamics after a quench24. Furthermore, we note that the BS mode has the nematic B1g symmetry and will naturally give rise to a signal in the nematic channel59. A computation of the third-order nonlinear susceptibility taking into account both s and d pairing channels in the presence of a finite nematic order parameter is desirable to further assess this scenario.

In conclusion, we have studied the impact of nematicity on the SC collective modes in Ba1−xKxFe2As2 via THz pump optical probe measurements. In the absence of nematicity we observe an instantaneous behavior of the optical reflectivity which we assign to a THz Kerr coupling to the Higgs mode. In the coexisting nematic + SC phase we observe a drastic change in the polarization dependence of the THz Kerr signal from purely C4 symmetric to purely nematic. The change cannot be accounted by the anisotropy of the electronic properties and indicates the emergence of an SC collective mode which couples strongly to the nematic order parameter. The exact identification of this mode requires further investigation, but we suggest the BS mode connecting nearly degenerate s and d wave pairing ground states as a likely candidate.

Methods

Samples

The two single crystals of Ba1−xKxFe2As2 with Tc = 26K (UD26, x ~ 0.23) and Tc = 37 K (UD37, x ~ 0.28) were characterized by SQUID magnetometry, wavelength dispersing spectroscopy and Raman scattering measurements. The samples are square-shaped with sides of ~5 mm. The crystal orientations of both samples were confirmed by polarization-resolved Raman spectroscopy measurements.

Terahertz pump-optical reflectivity probe (TPOP)

Strong single cycle THz pump pulses (0.3–1 THz) with electric field reaching up to 350 kV/cm are generated using optical rectification of 1.5 eV NIR pulses in a LiNbO3 crystal using the tilted pulse front technique60,61. For optical probe measurements 100 fs duration NIR pulses at 1.5 eV are used with a fluence of 10–100 nJ/cm2 and a spot size of 250 μm in diameter. The repetition rate of the NIR laser is 1 kHz.