Abstract
We present a transmon qubit fabrication technique that yields systematic improvements in T1 relaxation times. We encapsulate the surface of niobium and prevent the formation of its lossy surface oxide. By maintaining the same superconducting metal and only varying the surface, this comparative investigation examining different capping materials, such as tantalum, aluminum, titanium nitride, and gold, as well as substrates across different qubit foundries demonstrates the detrimental impact that niobium oxides have on coherence times of superconducting qubits, compared to native oxides of tantalum, aluminum or titanium nitride. Our surface-encapsulated niobium qubit devices exhibit T1 relaxation times 2–5 times longer than baseline qubit devices with native niobium oxides. When capping niobium with tantalum, we obtain median qubit lifetimes above 300 μs, with maximum values up to 600 μs. Our comparative structural and chemical analysis provides insight into why amorphous niobium oxides may induce higher losses compared to other amorphous oxides.
Similar content being viewed by others
Introduction
With massive improvements in device coherence times and gate fidelity over the past two decades, superconducting qubits have emerged as a leading technology platform for quantum computing1,2,3. Although many of these improvements have been driven through optimized device designs and geometries, the presence of defects and impurities at the interfaces and surfaces in the constituent materials continues to limit performance and serve as a critical barrier in achieving scalable quantum systems4,5,6. Specifically, these uncontrolled defect sites can serve as sources of loss by introducing two-level systems (TLS) or nonequilibrium quasiparticles7,8,9,10. As a result, researchers have recently begun to take a materials-oriented approach to understand and eliminate these sources of quantum decoherence in superconducting qubit devices.
Niobium (Nb) has been widely employed as the primary material in superconducting qubits as it possesses the largest critical temperature and superconducting gap of elemental superconductors, making thermal quasiparticle contribution to losses negligible at typical operating temperatures of on the order of tens of mK. It is also highly compatible with industrial-scale processes11. Furthermore, the Fermilab superconducting radio-frequency (SRF) research group has demonstrated in prior detailed studies of 3D cavities in the quantum regime that devices processed from Nb can sustain photon lifetimes as high as 2 s when the surface niobium oxide hosting sources of TLS is removed12. This is ~3 orders of magnitude longer than coherence times reported in the highest-performing transmon qubits13,14, making bare niobium metal an attractive base material for further improvements in 2D superconducting qubits.
These previous measurements have unambiguously identified the surface oxide that forms spontaneously on Nb under ambient conditions as the major source of microwave loss12,15,16,17,18,19,20. Through 3D cavity measurements, we find that the loss tangent of this 5 nm thick oxide is ~0.1, which is orders of magnitude larger than the losses at the metal/substrate interface as well as those in the underlying substrate21,22. As a result, the removal of this oxide has been shown to boost the photon lifetime by 50–200× in 3D Nb SRF cavities in the TLS-dominated, <1 K regime. Other studies on 2D devices have since further confirmed the detrimental effect of this oxide23,24.
Several recent studies have sought to mitigate losses associated with this region. Unfortunately, most methods for avoiding these losses are incompatible with integration into complex or large-scale manufacturing process flows. In one of the successful approaches, the surface oxide was removed by annealing the sample at temperatures at or exceeding 300 °C12, with an almost complete elimination of the TLS-induced losses. While this thermal dissolution method is effective, sustained vacuum is required afterwards to prevent the regrowth of the surface oxide when the cavity or qubit is removed from an ultra-high vacuum (UHV) environment. An alternative oxide removal method involving HF as a wet etchant has been explored as well23,24. This process cannot be performed in vacuum, leading to rapid oxide re-growth afterwards. Furthermore, it can lead to hydrogen incorporation in the underlying niobium which can introduce resistive niobium hydrides25,26,27. Finally, nitrogen plasma passivation techniques have been identified as effective methods to partially suppress oxide formation28,29.
Here, we propose a new strategy based on surface encapsulation to eliminate and prevent the formation of this lossy Nb surface oxide upon exposure to air. The first method involves depositing in situ metal capping layers of Al and Ta onto the Nb films in UHV. The second method involves atomic layer deposition to reduce the native Nb surface oxide by reacting it with a precursor and then depositing a thin metal film (TiN) that exhibits a reduced microwave loss. The third method involves milling away the oxide with Ar+ ions and depositing a thin metal layer of Au with e-beam evaporation. Based on our systematic study, we observe that each of these surface passivation strategies effectively eliminate Nb2O5 and yield a clear improvement in coherence times. Of these capping approaches, we find that the Ta-capped Nb films exhibit the largest improvement and lead to devices with median relaxation times of 300 μs. Finally, we explore the scalability of such an approach by repeating the fabrication of test devices with the Ta capping strategy at a commercial qubit fabrication and measurement facility and are able to replicate the results on Si substrates.
Results and discussion
Qubit fabrication
We fabricated seven sets of qubits for this study as outlined in Table 1 with device geometries provided in Fig. 1a. In terms of surface participation ratio, this is largest for device geometry A, followed by device geometry C, and smallest for device geometry B. Details of the fabrication procedure are provided in the Supplementary Information. Results from structural and chemical analysis of the fabricated qubits are provided in Figs. 1b and 2. Figure 1b shows a low-magnification scanning transmission electron microscopy (STEM) image of a cross-section taken from a superconducting circuit. The superconducting metal (Nb) and metal capping layer (Ta) are labeled. Chemical phase maps generated using STEM energy dispersive spectroscopy are presented in Fig. 2a–d for the Nb films capped with different layers. We find that the capping layers are between 5 and 10 nm thick, as targeted. Additionally, the layers are spatially distinct with minimal intermixing present.
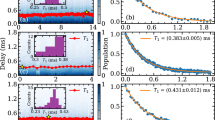
a 8 qubit chip layout consisting of three different geometries. b Low magnification annular dark field scanning transmission electron microscopy (ADF-STEM) image taken from a cross-section of a Nb transmon qubit where the Nb film is capped with a Ta metal layer. c Cryogenic wiring diagram. d, e Pulse scheme for characterization and plots. d Pulse for Rabi experiment used to calibrate π pulses. e Pulse for T1 experiment.
a–d Chemical phase maps generated through STEM energy dispersive spectroscopy of the Nb films capped with Ta, Al, TiN, and Au, respectively. The Ta, Al, and Au capping layers are roughly 10 nm thick and the TiN layer is roughly 5 nm thick. e Plot depicting Nb2O5 counts captured with ToF-SIMS. Each of the capping strategies is effective in mitigating Nb2O5 formation. The Ta capping is particularly effective as a 1000× decrease in Nb2O5− counts is observed with this strategy. f ADF-STEM image of the metal/substrate interface. Minimal intermixing is observed between Nb and the underlying c-plane sapphire substrate. Scale bars in (a)–(d) represent 10 nm. Scale bar in (f) represents 2 nm.
In order to assess the efficacy of the capping layers in preventing Nb2O5 formation, we analyze each film with time-of-flight secondary ion mass spectrometry (ToF-SIMS). This technique combines high mass resolution and sensitivity to both light and heavy elements with <100 nm spatial resolution, and has been employed extensively to identify impurities such as oxides in superconducting qubits16,30. As ToF-SIMS enables the identification of different oxide species based on their mass to charge (M/Z) ratios, we are able to resolve that the surface oxide is primarily composed of Nb2O5 based on the presence of localized signal corresponding to the presence of Nb2O5− ions in this region.
To better understand how this oxide is impacted by metal capping, we compare the Nb2O5− signal counts measured from the surface of the baseline Nb sample to the Nb2O5− signal counts measured at the interface between the capping layer and the Nb metal in the capped Nb samples as indicated in Fig. 2e. We note that this does not refer to total oxide quantity (as aluminum and tantalum themselves oxidize), but specifically to the reduction of the loss channel of particular interest, Nb2O5. The methodology is described in Supplementary Fig. 1 and the results are presented in Fig. 2e. We find that all of the capping strategies are highly effective in mitigating Nb2O5 formation. The Ta capping is particularly effective as a 1000× decrease in measured Nb2O5− is observed with this strategy. The results also suggest that in situ sputter deposition may be slightly more effective at protecting against surface oxidation compared to the other two methods. Finally, we observe the presence of a sharp interface between the Nb film and the underlying sapphire as shown in Fig. 2f. In contrast to Nb films grown on silicon where alloyed regions on the order of 5 nm have been observed, in Nb/sapphire structures we observe minimal intermixing present at the metal/substrate interface31.
Qubit results
To most easily observe the impact of the metal capping layer on the superconducting qubit coherence, we performed measurements using the qubit geometry with the largest surface participation ratio (geometry A). These measurements were performed on the capped devices and on baseline Nb devices that were not capped.
The qubit devices are measured inside a dilution refrigerator at a temperature of around 40 mK via dispersive readout. The cryogenic wiring diagram is shown in Fig. 1c. Both qubit and readout pulses are sent through a single RF line. At the mixing chamber plate, two six-pole-single-throw microwave switches are used to direct the signals to the relevant sample and to extract the outgoing signal. After initial qubit spectroscopy, a Rabi measurement (Fig. 1d) is performed to determine the π-pulse length followed by T1 measurements (Fig. 1e).
Because the qubit energy relaxation time, T1, is largely dependent on the material losses in the qubit device, while the dephasing time, T2, is heavily impacted by many environmental factors such as the thermal noise, IR radiation, and cosmic rays32,33, we focus on qubit T1 characterization. The qubit T1 characterization is widely believed to directly reflect the loss due to TLS, which is the focus of this work. The measurement follows the standard procedure34, i.e., the qubit is driven from the ground to the first-excited state by a calibrated π pulse. The qubit state is then read after a variable delay. The measured signal decay is fitted exponentially to extract the qubit relaxation time, T1. In order to probe both the typical and exceptional T1 times, we benchmark the T1 measurement by continuously collecting data for several hours for each qubit, as described in ref. 4. Using this data set, we extract the average, standard deviation, and best T1 values, which we compare across different devices.
A comparison of the T1 measured for different devices is provided in Fig. 3a and further summarized in Supplementary Table 1. The Nb qubits capped with Ta as well as the Nb qubits capped with Au have the highest average T1 (>100 μs), while the baseline Nb qubits have the lowest average T1. The average T1 value of Nb qubits capped with Al is slightly higher than that of Nb qubits capped with TiN. The improvement in average T1 for all of the capped devices suggests that reducing the native Nb2O5 (a strong TLS host) on the surface of the Nb film improves qubit energy relaxation times. Whiskers or circles in the plot indicate the maximum and minimum T1 values observed during measurement windows on the order of a few hours per qubit [see Fig. 3d for the best T1 decay curve]. The fluctuations of T1 over time [shown in Fig. 3e], and its Gaussian distribution [shown in Fig. 3f] have both been observed in literature4,35, and are presumably considered a signature of qubit lifetime limited by TLS defects residing in the materials.
a T1 comparison of the five sets of qubit devices that were prepared on sapphire substrates. All four Nb/Ta qubits on the chip show T1 > 100 μs, and the largest T1 measured for Nb control qubits is ~50 μs. Boxes mark the 25th percentile and the 75th percentile of the measurement distribution over the course of 10 h of consecutive measurements. The line inside each box represents the median value. Error bars represent 95% confidence interval and circles represent outliers. b Measured T1 values for test devices fabricated on silicon substrates. We observe a clear improvement in terms of the median T1 value following surface capping of Nb with Ta. c Dependence of T1 standard deviation, σT1, on the average T1, μT1. Different colors correspond to the different encapsulation groups shown in (a). Dashed line shows the best fitting of \({\sigma }_{{T}_{1}}\propto {\mu }_{{T}_{1}}^{3/2}\), according to ref. 36. It also shows that the Nb/Ta qubit has 5-10 times improvement of TLS loss compared with the Nb qubit, after converting the number of TLS into the tangent loss, δTLS. d Best T1 = 198 μs. e Statistics for T1 consecutively measured over 10 h, the average T1 (μT1) is 161 μs and the standard deviation (σT1) is 15 μs. The star shows the iteration that yielded the best T1 (see (d) for the decay curve). f Histogram of the T1 values in (e), with a Gaussian fit.
To quantitatively compare TLS densities from different encapsulations, we calculate the average (μT1) vs. standard deviation (σT1) T1 for each of the qubits that were measured continuously for 10 hours. According to TLS theory36, μT1 and σT1 can be represented by the following relations:
where α and β are temperature-dependent constants and ω is the qubit frequency, which is treated as constant in this case as the qubit frequencies all lie within the range of 4 to 6 GHz. N is proportional to the number of TLS present in the qubit. From Eqs. (1) and (2), we find that \({\sigma }_{{T}_{1}}\propto {\mu }_{{T}_{1}}^{3/2}\) and the data is in agreement with this relationship (dashed line in Fig. 3c). Based on this plot, we determine that Nb qubits capped with Ta and Nb qubits capped with Au exhibit the smallest number of TLS and the highest μT1, maximum T1 and fluctuations σT1. Conversely, the baseline Nb qubit exhibits the greatest number of TLS and therefore the lowest average T1. Assuming TLS loss tangent, δTLS, is proportional to the number of TLS9, we plot the relative change of δTLS for each set of devices, as shown in Fig. 3c. We find 5–8× reduction in loss between the Ta-capped Nb devices and the baseline Nb devices. Additionally, we find that the loss associated with Al-capped Nb qubits is slightly lower than TiN-capped Nb qubits. These results are also in agreement with the qubit T1 measurements.
To assess the reproducibility, scalability and applicability of this capping method to other substrates, Ta-capped and Nb-only devices (with the same test geometry) were fabricated in the Rigetti Computing quantum integrated circuit foundry (Fremont, California) on high-resistivity Si(100) substrates. More details of this fabrication process can be found in ref. 6. The Ta-capped devices exhibit a systematic improvement in median T1 (~200 μs) compared to the baseline Nb qubits (~120 μs). A box plot providing information on the measurements performed on both sets of qubits is provided in Fig. 3b with a maximum measured T1 value of 451 μs. The consistent improvement in median T1 for the capped Nb devices for both silicon and sapphire substrates supports a performance limitation imposed by the amorphous Nb2O5 surface oxide in un-capped devices. Meanwhile, the T2, T2 echo, and Tϕ values are provided in Supplementary Fig. 2. The T2, T2 echo, and Tϕ values also increase following capping of Nb with Ta, although the spread in values is larger, as expected given their dependencies on additional extrinsic factors.
Given these promising T1 results, we also fabricated larger footprint qubit geometries of the Ta-capped Nb (Geometry B and C) on sapphire that are comparable to those used by groups that have demonstrated the largest T1 values to date13,14. In Fig. 4, a comparison of the measured T1 values for a Ta-capped device is provided for the three different geometries that were investigated. These results are also summarized in Supplementary Table 2. Geometry B yields T1 values with median qubit relaxation times above 300 μs, which are in line with the median qubit relaxation times reported by these groups. Further, we observe several individual measurements in excess of 550 μs over the course of several days. These are the leading values published to date for transmon qubits. These results, combined with the fact that this approach is reliable with both silicon and sapphire substrates and can be performed at room temperature makes surface encapsulation a very attractive methodology for achieving high coherence qubits. Moreover, future studies will involve gold encapsulation and other similar low-loss capping layers with similar larger footprint geometries to potentially push the envelope of performance even further.
a T1 comparison of Nb/Ta qubits as a function of three different qubit geometries prepared on sapphire substrates. Geometry B yields the largest T1 values. Error bars represent 95% confidence interval. b Statistics for T1 consecutively measured over 70 h for a Geometry B qubit. The average T1 (μT1) is 323 μs. c Decay curve associated with measured T1 value of 586 μs (indicated by star in (b)).
Together, these results clearly demonstrate that eliminating Nb2O5 enhances the T1 relaxation time of Nb transmon qubits. In the case of devices capped with Ta, Al, and TiN, we still observe the presence of amorphous oxides of a few nm thickness such as Ta2O5, AlOx, and TiOx, respectively at the sample surface (Figs. 5 and S3). By linking modifications solely to the metal/air interface to measured T1 values, we observe a trend where Ta2O5 ranks as the least lossy oxide of those measured, followed by Al2O3, TiO2, and finally, Nb2O5. Further, the loss introduced by these various surface oxides does not appear to be directly correlated to their individual thicknesses as illustrated in Table 2. In particular, 1–2 nm TiOx at the surface of the TiN-capped Nb qubit is found to be the thinnest oxide whereas the 5–7 nm Ta2O5 observed at the surface of the Ta-capped Nb qubit prepared on silicon is found to be the thickest.
Oxides for (a) Al-capped Nb, (b) TiN-capped Nb, (c) baseline Nb, and (d) Ta-capped Nb qubits prepared on sapphire are presented. Similar images of surface oxides for (e) baseline Nb, and (f) Ta capped Nb qubits prepared on silicon are also presented. The oxides are identified with arrows and tabulated in Table 2. Scale bars represent 10 nm.
Therefore, our findings help explain why previous experimental studies with qubits prepared from Ta metal exhibited improved T1 values13,14. Namely, our results suggest the improved T1 predominantly arises from the presence of a less lossy surface oxide, as opposed to the tantalum film being less lossy compared to niobium films.
To understand the difference in performance between the capped and baseline samples, we use electron microscopy and, in particular, electron energy loss spectroscopy (EELS) to further evaluate the chemical nature of the Ta oxide. EELS signals captured from points 1-10 on the dark field image of the Ta2O5/Ta interface of the prepared qubit are provided in Fig. 6. From this image, we observe that the oxide thickness of Ta is roughly similar to that observed for Nb oxide (4–5 nm). The Ta oxide is found to be predominantly amorphous based on the presence of diffuse diffraction patterns taken in this region (Fig. S4). Features associated with the tantalum O2,3 edge are labeled with a dotted line in Fig. 6b and those associated with the oxygen K edge are labeled with a dotted line in Fig. 6c. We find there are changes in the shape of both set of features, but the position of oxygen K edge remains constant. This indicates that the Ta predominately exists in a 5+ state. This is in contrast to what has been observed in Nb. For Nb, shifts in the features accompanying the onset of the oxygen K edge are observed as a function of position in the oxide due to spatial variations in the valence state37,38. This suggests that the Ta oxide present in these capped samples is largely free of sub-stoichiometric regions.
a Darkfield STEM image of the Ta2O5/Ta interface. Electron energy loss spectra (EELS) taken from the locations indicated in (a) are provided in (b) and (c). b EELS signal taken from the specified region demonstrating how the tantalum O2,3 edge evolves with position. c EELS signal taken from the specified region demonstrating how oxygen K edge evolves as a function of position. The dotted lines indicate minimal variation in the position of various features of the spectra. This suggests that the oxygen stoichiometry remains consistent throughout the oxide region. Scale bars represent 10 nm.
Finally, the X-ray reflectivity signal captured from both the Nb sample as well as the Ta-capped Nb sample is provided in Fig. S5. Based on the data fits performed using dynamical scattering theory39, we observe that the surface oxide of Nb consists of roughly 4.1 nm of Nb2O5 and 0.5 nm of NbO whereas the surface oxide of Ta consists entirely of 5.9 nm of Ta2O5. This technique suggests that the capped samples are largely free of sub-oxides and is consistent with the TEM findings.
Theoretical and experimental findings have linked the variable oxygen content in the Nb oxide layer to the presence of local paramagnetic moments. These moments are a source of flux noise, dephasing, and energy loss40,41. We hypothesize that the stoichiometric, predominantly Ta2O5 layer of Ta-capped Nb reduces the potential for moment formation compared to the variable oxygen content observed in the Nb oxide layer of uncapped Nb. Additionally, it is possible the Ta oxide layer hosts fewer TLS in the frequency range of the qubit frequency compared to the Nb oxide layer. This is an area of continuing active exploration through experimental and theoretical studies. In summary, we have implemented different passivation strategies to eliminate and prevent the formation of lossy Nb surface oxides in Nb superconducting qubits. By capping Nb films with Ta, Al, TiN, and Au, we are able to systematically improve the average qubit relaxation times. Of these capping strategies, we find that Nb films capped with Ta and Nb films capped with Au yield the highest median T1. We observe similar improvements in median T1 when superconducting qubits are prepared on silicon as well as sapphire. Together, this methodology offers a solution to delivering state-of-the-art devices with median T1 times exceeding 300 μs that is compatible with industrial-level processes. Further, this method offers a pathway for continuing to suppress the dielectric loss associated with the metal/air interface through further exploration of capping Nb film with ambient-stable layers prior to air exposure and is applicable to the field of superconducting devices broadly (quantum information science, detectors for cosmic science, and particle accelerators). Finally, this study provides strong indication that the Nb oxide is more lossy than the tantalum oxide and will help guide future investigations aimed at building a microscopic understanding of TLS sources in superconducting qubits.
Disclaimer
Certain commercial equipment, instruments, or materials are identified in this paper in order to specify the experimental procedure adequately. Such identification is not intended to imply recommendation or endorsement by NIST, nor is it intended to imply that the materials or equipment identified are necessarily the best available for the purpose.
Data availability
The data that support the findings of this study are available from the corresponding author upon reasonable request.
References
Kjaergaard, M. et al. Superconducting qubits: current state of play. Annu. Rev. Condens. Matter Phys. 11, 369–395 (2020).
Wendin, G. Quantum information processing with superconducting circuits: a review. Rep. Prog. Phys. 80, 106001 (2017).
de Leon, N. P. et al. Materials challenges and opportunities for quantum computing hardware. Science 372, eabb2823 (2021).
Burnett, J. J. et al. Decoherence benchmarking of superconducting qubits. npj Quantum Inf. 5, 54 (2019).
Schlör, S. et al. Correlating decoherence in transmon qubits: low frequency noise by single fluctuators. Phys. Rev. Lett. 123, 190502 (2019).
Nersisyan, A. et al. Manufacturing low-dissipation superconducting quantum processors. (2019).
Wilen, C. D. et al. Correlated charge noise and relaxation errors in superconducting qubits. Nature 594, 369–373 (2021).
Simmonds, R. W. et al. Decoherence in Josephson phase qubits from junction resonators. Phys. Rev. Lett. 93, 077003 (2004).
Müller, C., Cole, J. H. & Lisenfeld, J. Towards understanding two-level-systems in amorphous solids: insights from quantum circuits. Rep. Prog. Phys. 82, 124501 (2019).
McDermott, R. Materials origins of decoherence in superconducting qubits. IEEE Trans. Appl. Supercond. 19, 2–13 (2009).
Tolpygo, S. K. et al. Fabrication process and properties of fully-planarized deep-submicron Nb/Al– AlOx/Nb Josephson junctions for VLSI circuits. IEEE Trans. Appl. Supercond. 25, 1–12 (2015).
Romanenko, A. et al. Three-dimensional superconducting resonators at 20 mK with photon lifetimes up to τ = 2 s. Phys. Rev. Appl. 13, 034032 (2020).
Place, A. P. M. et al. New material platform for superconducting transmon qubits with coherence times exceeding 0.3 milliseconds. Nat. Commun. 12, 1779 (2021).
Wang, C. et al. Towards practical quantum computers: transmon qubit with a lifetime approaching 0.5 milliseconds. npj Quantum Inf. 8, 3 (2022).
Romanenko, A. & Schuster, D. I. Understanding quality factor degradation in superconducting niobium cavities at low microwave field amplitudes. Phys. Rev. Lett. 119, 264801 (2017).
Murthy, A. A. et al. Developing a chemical and structural understanding of the surface oxide in a niobium superconducting qubit. ACS Nano 16, 17257–17262 (2022).
Niepce, D., Burnett, J. J., Latorre, M. G. & Bylander, J. Geometric scaling of two-level-system loss in superconducting resonators. Supercond. Sci. Technol. 33, 025013 (2020).
Bafia, D., Grassellino, A. & Romanenko, A. Probing the role of low-temperature vacuum baking on photon lifetimes in superconducting niobium 3-d resonators. Preprint at http://arXiv.org/abs/2108.13352 (2021).
Burnett, J., Faoro, L. & Lindström, T. Analysis of high-quality superconducting resonators: consequences for TLS properties in amorphous oxides. Supercond. Sci. Technol. 29, 044008 (2016).
Premkumar, A. et al. Microscopic relaxation channels in materials for superconducting qubits. Commun. Mater. 2, 72 (2021).
McRae, C. R. H. et al. Materials loss measurements using superconducting microwave resonators. Rev. Sci. Instrum. 91, 091101 (2020).
Checchin, M., Frolov, D., Lunin, A., Grassellino, A. & Romanenko, A. Measurement of the low-temperature loss tangent of high-resistivity silicon using a high-Q superconducting resonator. Phys. Rev. Appl. 18, 034013 (2022).
Verjauw, J. et al. Investigation of microwave loss induced by oxide regrowth in high-Q niobium resonators. Phys. Rev. Appl. 16, 014018 (2021).
Altoé, M. V. P. et al. Localization and mitigation of loss in niobium superconducting circuits. PRX Quantum 3, 020312 (2022).
Knobloch, J. The “Q disease" in superconducting niobium rf cavities. AIP Conf. Proc. 671, 133–150 (2003).
Romanenko, A., Barkov, F., Cooley, L. D. & Grassellino, A. Proximity breakdown of hydrides in superconducting niobium cavities. Supercond. Sci. Technol. 26, 035003 (2013).
Lee, J. et al. Discovery of Nb hydride precipitates in superconducting qubits. Preprint at http://arXiv.org/abs/2108.10385 (2021).
Zheng, K. et al. Nitrogen plasma passivated niobium resonators for superconducting quantum circuits. Appl. Phys. Lett. 120 (2022). https://doi.org/10.1063/5.0082755. 102601.
Fang, X. et al. Understanding mechanism of performance improvement in nitrogen-doped niobium superconducting radio frequency cavity. Mater. Res. Lett. 11, 108–116 (2023).
Murthy, A. A. et al. ToF-SIMS analysis of decoherence sources in superconducting qubits. Appl. Phys. Lett. 120, 044002 (2022).
Lu, X. et al. Stability, metallicity, and magnetism in niobium silicide nanofilms. Phys. Rev. Mater. 6, 064402 (2022).
Clerk, A. A. & Utami, D. W. Using a qubit to measure photon-number statistics of a driven thermal oscillator. Phys. Rev. A 75, 042302 (2007).
Vaaranta, A., Cattaneo, M. & Lake, R. E. Dynamics of a dispersively coupled transmon qubit in the presence of a noise source embedded in the control line. Phys. Rev. A 106, 042605 (2022).
Krantz, P. et al. A quantum engineer’s guide to superconducting qubits. Appl. Phys. Rev. 6, 021318 (2019).
Klimov, P. et al. Fluctuations of energy-relaxation times in superconducting qubits. Phys. Rev. Lett. 121, 090502 (2018).
You, X. et al. Stabilizing and improving qubit coherence by engineering the noise spectrum of two-level systems. Phys. Rev. Appl. 18, 044026 (2022).
Tao, R. et al. Electron energy-loss spectroscopy study of metallic Nb and Nb oxides. J. Appl. Phys. 110, 124313 (2011).
Oh, J.-S. et al. In-Situ Transmission Electron Microscopy Investigation on Surface Oxides Thermal Stability of Niobium. Appl. Surf. Sci. 627, 157297 (2023).
Nelson, A. Co-refinement of multiple-contrast neutron/x-ray reflectivity data using motofit. J. Appl. Crystallogr. 39, 273–276 (2006).
Sheridan, E. et al. Microscopic theory of magnetic disorder-induced decoherence in superconducting Nb films. Preprint at http://arXiv.org/abs/2111.11684 (2022).
Proslier, T., Kharitonov, M., Pellin, M. & Zasadzinski, J. Evidence of surface paramagnetism in niobium and consequences for the superconducting cavity surface impedance. IEEE Trans. Appl. Supercond. 21, 2619–2622 (2011).
Acknowledgements
This material is based upon work supported by the U.S. Department of Energy, Office of Science, National Quantum Information Science Research Centers, Superconducting Quantum Materials and Systems Center (SQMS) under contract no. DE-AC02-07CH11359. This work made use of the Pritzker Nanofabrication Facility of the Institute for Molecular Engineering at the University of Chicago, which receives support from Soft and Hybrid Nanotechnology Experimental (SHyNE) Resource (NSF ECCS-2025633), a node of the National Science Foundation’s National Nanotechnology Coordinated Infrastructure. Ames National Laboratory is operated for the U.S. Department of Energy by Iowa State University under Contract No. DE-AC02-07CH11358. The authors thank members of the SQMS Center for valuable discussions.
Author information
Authors and Affiliations
Contributions
M.B., A.M., S.Z., and F.C. contributed equally to this manuscript. The project was conceived by M.B., A.M., S.Z., F.C., A.R., and A.G. The devices are fabricated by M.B., F.C., I.N., H.C., J.M., K.V. The measurements were performed by A.M., S.Z., E.L., D.M., J.L., J.O., L.Z., D.G., C.G., M.B., and M.H. The experimental data was analyzed by M.B., A.M., S.Z., F.C., X.Y., Z.H., T.R., J.L., D.v.Z., R.P., I.N., A.L., D.B., Y.K., C.K., E.L., D.M., J.M., M.R., H.C., J.M., D.P., K.V., K.Y., J.S., L.Z., M.K., F.Q., D.G., C.T., G.P., V.D., J.R., M.B., M.H., J.Z., J.K., J.S., A.R., and A.G. A.M. wrote the manuscript together with M.B., S.Z., F.C., A.R., and A.G. All authors discussed the results and the manuscript.
Corresponding authors
Ethics declarations
Competing interests
All authors declare no competing interests.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Bal, M., Murthy, A.A., Zhu, S. et al. Systematic improvements in transmon qubit coherence enabled by niobium surface encapsulation. npj Quantum Inf 10, 43 (2024). https://doi.org/10.1038/s41534-024-00840-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41534-024-00840-x
This article is cited by
-
Advanced CMOS manufacturing of superconducting qubits on 300 mm wafers
Nature (2024)
-
Mechanically induced correlated errors on superconducting qubits with relaxation times exceeding 0.4 ms
Nature Communications (2024)