Abstract
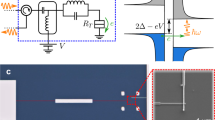
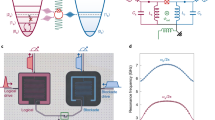
Engineered dissipation can be used for quantum state preparation. This is achieved with a suitably engineered coupling to a dissipative cold reservoir usually formed by an electromagnetic mode. In the field of cavity electro- and optomechanics, the electromagnetic cavity naturally serves as a cold reservoir for the mechanical mode. Here, we realize the opposite scenario and engineer a mechanical oscillator cooled close to its ground state into a cold dissipative reservoir for microwave photons in a superconducting circuit. By tuning the coupling to this dissipative mechanical reservoir, we demonstrate dynamical backaction control of the microwave field, leading to stimulated emission and maser action. Moreover, the reservoir can function as a useful quantum resource, allowing the implementation of a near-quantum-limited phase-preserving microwave amplifier. Such engineered mechanical dissipation extends the toolbox of quantum manipulation techniques of the microwave field and constitutes a new ingredient for optomechanical protocols.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Caldeira, A. O. & Leggett, A. J. Influence of dissipation on quantum tunneling in macroscopic systems. Phys. Rev. Lett. 46, 211–214 (1981).
Poyatos, J. F., Cirac, J. I. & Zoller, P. Quantum reservoir engineering with laser cooled trapped ions. Phys. Rev. Lett. 77, 4728–4731 (1996).
Krauter, H. et al. Entanglement generated by dissipation and steady state entanglement of two macroscopic objects. Phys. Rev. Lett. 107, 080503 (2011).
Barreiro, J. T. et al. An open-system quantum simulator with trapped ions. Nature 470, 486–491 (2011).
Lin, Y. et al. Dissipative production of a maximally entangled steady state of two quantum bits. Nature 504, 415–418 (2013).
Kienzler, D. et al. Quantum harmonic oscillator state synthesis by reservoir engineering. Science 347, 53–56 (2015).
Murch, K. W. et al. Cavity-assisted quantum bath engineering. Phys. Rev. Lett. 109, 183602 (2012).
Shankar, S. et al. Autonomously stabilized entanglement between two superconducting quantum bits. Nature 504, 419–422 (2013).
Leghtas, Z. et al. Confining the state of light to a quantum manifold by engineered two-photon loss. Science 347, 853–857 (2015).
Aspelmeyer, M., Kippenberg, T. J. & Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 86, 1391–1452 (2014).
Kronwald, A., Marquardt, F. & Clerk, A. A. Arbitrarily large steady-state bosonic squeezing via dissipation. Phys. Rev. A 88, 063833 (2013).
Woolley, M. J. & Clerk, A. A. Two-mode squeezed states in cavity optomechanics via engineering of a single reservoir. Phys. Rev. A 89, 063805 (2014).
Wollman, E. E. et al. Quantum squeezing of motion in a mechanical resonator. Science 349, 952–955 (2015).
Pirkkalainen, J.-M., Damskägg, E., Brandt, M., Massel, F. & Sillanpää, M. Squeezing of quantum noise of motion in a micromechanical resonator. Phys. Rev. Lett. 115, 243601 (2015).
Lecocq, F., Clark, J., Simmonds, R., Aumentado, J. & Teufel, J. Quantum nondemolition measurement of a nonclassical state of a massive object. Phys. Rev. X 5, 041037 (2015).
Wang, Y.-D. & Clerk, A. A. Reservoir-engineered entanglement in optomechanical systems. Phys. Rev. Lett. 110, 253601 (2013).
Metelmann, A. & Clerk, A. Quantum-limited amplification via reservoir engineering. Phys. Rev. Lett. 112, 133904 (2014).
Nunnenkamp, A., Sudhir, V., Feofanov, A. K., Roulet, A. & Kippenberg, T. J. quantum-limited amplification and parametric instability in the reversed dissipation regime of cavity optomechanics. Phys. Rev. Lett. 113, 023604 (2014).
Kronwald, A., Marquardt, F. & Clerk, A. A. Dissipative optomechanical squeezing of light. New J. Phys. 16, 063058 (2014).
Metelmann, A. & Clerk, A. Nonreciprocal photon transmission and amplification via reservoir engineering. Phys. Rev. X 5, 021025 (2015).
Teufel, J. D. et al. Circuit cavity electromechanics in the strong-coupling regime. Nature 471, 204–208 (2011).
Braginsky, V. & Manukin, A. Measurement of Weak Forces in Physics Experiments (Univ. Chicago Press, 1977).
Teufel, J. D., Harlow, J. W., Regal, C. A. & Lehnert, K. W. Dynamical backaction of microwave fields on a nanomechanical oscillator. Phys. Rev. Lett. 101, 197203 (2008).
Schliesser, A., Rivière, R., Anetsberger, G., Arcizet, O. & Kippenberg, T. J. Resolved-sideband cooling of a micromechanical oscillator. Nat. Phys. 4, 415–419 (2008).
Cicak, K. et al. Low-loss superconducting resonant circuits using vacuum-gap-based microwave components. Appl. Phys. Lett. 96, 093502 (2010).
Dobrindt, J. M., Wilson-Rae, I. & Kippenberg, T. J. Parametric normal-mode splitting in cavity optomechanics. Phys. Rev. Lett. 101, 263602 (2008).
Braginsky, V., Manukin, A. & Tikhonov, M. Y. Investigation of dissipative ponderomotive effects of electromagnetic radiation. Sov. Phys. JETP 31, 829–831 (1970).
Kippenberg, T. J., Rokhsari, H., Carmon, T., Scherer, A. & Vahala, K. J. Analysis of radiation-pressure induced mechanical oscillation of an optical microcavity. Phys. Rev. Lett. 95, 033901 (2005).
Megrant, A. et al. Planar superconducting resonators with internal quality factors above one million. Appl. Phys. Lett. 100, 113510 (2012).
Clerk, A. A., Devoret, M. H., Girvin, S. M., Marquardt, F. & Schoelkopf, R. J. Introduction to quantum noise, measurement, and amplification. Rev. Mod. Phys. 82, 1155–1208 (2010).
Marquardt, F., Harris, J. G. E. & Girvin, S. M. Dynamical multistability induced by radiation pressure in high-finesse micromechanical optical cavities. Phys. Rev. Lett. 96, 103901 (2006).
Grudinin, I. S., Lee, H., Painter, O. & Vahala, K. J. Phonon laser action in a tunable two-level system. Phys. Rev. Lett. 104, 083901 (2010).
Astafiev, O. et al. Single artificial-atom lasing. Nature 449, 588–590 (2007).
Caves, C. M. Quantum limits on noise in linear amplifiers. Phys. Rev. D 26, 1817–1839 (1982).
Massel, F. et al. Microwave amplification with nanomechanical resonators. Nature 480, 351–354 (2011).
Ockeloen-Korppi, C. F. et al. Low-noise amplification and frequency conversion with a multiport microwave optomechanical device. Phys. Rev. X 6, 041024 (2016).
Bergeal, N. et al. Phase-preserving amplification near the quantum limit with a Josephson ring modulator. Nature 465, 64–68 (2010).
Eichler, C., Salathe, Y., Mlynek, J., Schmidt, S. & Wallraff, A. Quantum-limited amplification and entanglement in coupled nonlinear resonators. Phys. Rev. Lett. 113, 110502 (2014).
Castellanos-Beltran, M. A., Irwin, K. D., Hilton, G. C., Vale, L. R. & Lehnert, K. W. Amplification and squeezing of quantum noise with a tunable Josephson metamaterial. Nat. Phys. 4, 929–931 (2008).
Bloembergen, N. Nonlinear Optics 4th edn (World Scientific, 1996).
Sliwa, K. M. et al. Reconfigurable Josephson circulator/directional amplifier. Phys. Rev. X 5, 041020 (2015).
Andrews, R. W., Reed, A. P., Cicak, K., Teufel, J. D. & Lehnert, K. W. Quantum-enabled temporal and spectral mode conversion of microwave signals. Nat. Commun. 6, 10021 (2015).
Grajcar, M. et al. Sisyphus cooling and amplification by a superconducting qubit. Nat. Phys. 4, 612–616 (2008).
Kerckhoff, J. et al. Tunable coupling to a mechanical oscillator circuit using a coherent feedback network. Phys. Rev. X 3, 093502 (2013).
Wallraff, A. et al. Strong coupling of a single photon to a superconducting qubit using circuit quantum electrodynamics. Nature 431, 162–167 (2004).
Devoret, M. H. & Schoelkopf, R. J. Superconducting circuits for quantum information: an outlook. Science 339, 1169–1174 (2013).
Acknowledgements
We thank A. A. Clerk, V. Sudhir and D. Wilson for helpful comments, E. Glushkov for helping out with the measurement setup and C. Javerzac-Galy for general assistance. This work was funded by the SNF, the NCCR Quantum Science and Technology (QSIT), and the European Union Seventh Framework Program through iQUOEMS (grant no. 323924). L.D.T. is supported by Marie Curie ITN cQOM (grant no. 290161). T.J.K. acknowledges financial support from an ERC AdG (QuREM). A.N. holds a University Research Fellowship from the Royal Society and acknowledges support from the Winton Programme for the Physics of Sustainability. All samples were fabricated in the Center of MicroNanoTechnology (CMi) at EPFL.
Author information
Authors and Affiliations
Contributions
T.J.K. and A.K.F. conceived the idea. L.D.T. fabricated the devices. L.D.T. and N.R.B., under the supervision of A.K.F., performed the measurements. N.R.B. carried out the data analysis. A.N. contributed to the theoretical framework. All authors contributed to writing the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 760 kb)
Rights and permissions
About this article
Cite this article
Tóth, L., Bernier, N., Nunnenkamp, A. et al. A dissipative quantum reservoir for microwave light using a mechanical oscillator. Nature Phys 13, 787–793 (2017). https://doi.org/10.1038/nphys4121
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys4121
This article is cited by
-
High-fidelity and robust optomechanical state transfer based on pulse control
Applied Physics B (2023)
-
Engineered dissipation for quantum information science
Nature Reviews Physics (2022)
-
Topological lattices realized in superconducting circuit optomechanics
Nature (2022)
-
Tunable optical response in a hybrid quadratic optomechanical system coupled with single semiconductor quantum well
Quantum Information Processing (2022)
-
Processing light with an optically tunable mechanical memory
Nature Communications (2021)