Main

Three-dimensional topological insulators (TIs) possess metallic surface states in which the spins of carriers are locked orthogonal to their momenta as a result of the time-reversal invariant. This feature is called ‘spin–momentum locking’, and has been employed as the principal mechanism to induce spin accumulation in the surface states of TIs (refs 1,3,4,5,14,15,16,17). Conceptually, the charge current can fully contribute to the spin current via spin–momentum locking; a C–S conversion efficiency θCS of 100% is expected at the non-TI/TI heterointerface. This highly efficient C–S conversion can be widely applicable to spintronic devices. However, the C–S conversion efficiency deduced from the spin torque measurement can exceed 100% for TIs with EF located in the bulk band, leading to mixed contributions from the surface and bulk bands3,4, when the efficiency is defined as θCS = JS/JC, where JS is the spin current density (A m−2) and JC is the charge current density (A m−2) in the entire TI layer. Here we isolate the contribution of the Dirac electrons in the C–S conversion process and clarify the role of the Fermi level EF and the Fermi velocity vF by employing TI samples with various EF positions. Accordingly, we define the interface C–S conversion coefficient qICS as qICS = JS/jC, where jC is the surface charge current density (A m−1). Based on the concept of spin–momentum locking, the magnitude of JS is governed by that of jC, which is linked with the conductivity of the surface states on the TI layer depending on the Fermi energy and Dirac dispersion: the Fermi velocity vF and the Fermi wavevector kF (refs 15,16,17). In this study, we quantitatively evaluate the interface C–S conversion effect by means of the ST-FMR technique for 8-nm (Bi1−xSbx)2Te3/8-nm Cu/10-nm Ni80Fe20 (Py) trilayer films, as shown in Fig. 1a. Systematic control of the Fermi levels by varying x in (Bi1−xSbx)2Te3 (BST) thin films enables us to investigate the relationship between qICS and the transport properties at the surface state.

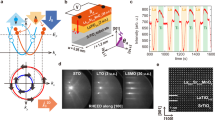
Figure 1: Spin current generation and detection in BST/Cu/Py trilayer structure.
figure 1

a, ST-FMR measurement circuit and device design employing BST/Cu/Py heterostructures. White arrows on the surfaces of the BST layer show the polarization direction of spin accumulation. The static magnetic field (Hext) is tilted by θ = 45°. b, A typical ST-FMR spectrum measured for a BST (x = 0.7)/Cu/Py trilayer film at 10 K. Red plot shows the experimental spectrum, which can be divided into symmetric (VSym, green line) and anti-symmetric (VAnti, blue line) parts. VSym and VAnti correspond to spin-current-induced FMR and Oersted-field-induced FMR, respectively.

Full size image

The ST-FMR technique has been routinely employed to evaluate the spin current induced via the spin Hall effect in paramagnetic metals10. Here we apply this technique to characterize quantitatively the interface C–S conversion effect due to spin–momentum locking in Cu-inserted TI-based trilayer heterostructures, as shown in the top schematic of Fig. 1a. On insertion of a Cu layer between the TI and ferromagnet layers, spin accumulation at the surface states can be separately evaluated owing to suppression of the exchange coupling between the ferromagnet and the surface states of TI (refs 18,19,20). In addition, the deposition of Cu on BST probably plays a minor role in varying the surface state condition, such as through an energy shift of the Dirac point and valence band maximum21, owing to a similar magnitude between work function of Cu and the electron negativity of BST. A photo of the device and measurement circuit is shown at the bottom of Fig. 1a. To evaluate qICS = JS/jC by means of ST-FMR, the charge current distribution in the trilayer should be clarified numerically, because jC in the TI layer is one of the dominant factors in this evaluation technique. When a radiofrequency (rf) current flows in the trilayer film, FMR is excited in the top Py layer under an external static magnetic field Hext. Owing to the presence of the highly conductive Cu layer, the peak of the current density is located towards the outside of the Py layer so that homogeneous rf fields (Hrf) can be applied to the Py layer (see Supplementary Information 1), providing better conditions for characterizing the C–S conversion effect by means of ST-FMR22. Accumulation of spins takes place simultaneously in the surface state of the TI; these accumulated spins generate a spin current JS in the orthogonal direction, diffusing into both Cu and Py layers, and thus exert a spin torque on the Py layer (white arrow in Fig. 1a). Note that the spin pumping effect, as an inverse effect to the charge-to-spin conversion, provides a small contribution in the evaluation of qICS (Supplementary Information 8). A typical ST-FMR spectrum is shown in Fig. 1b: the symmetric voltage VSym is attributable to the spin torque τ∥/ corresponding to the spin current density JS (details discussed later). By quantitative evaluation of VSym, we can deduce the interface C–S conversion coefficient qICS.

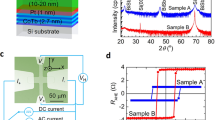
Figure 2a shows the Hall coefficients RH obtained for seven films having different Sb composition x at 10 K. The value of RH is negative for x = 0, with an increasing magnitude as x is increased to 0.82, indicating n-type conduction and a reduction in the electron density. The polarity of RH abruptly reverses its sign when x reaches a value of approximately 0.84, revealing that the Fermi energy traverses the Dirac point (DP). In the range 0.88 ≤ x ≤ 1, the polarity of RH is positive, indicating p-type conduction. The charge carrier densities and mobilities shown in Fig. 2b are estimated from the RH values. Compared to the previous studies3,23, the charge carrier density in the surface state reaches as low as 1012 cm−2, indicating that EF is finely tuned close to the Dirac point. The mobility (μ) in BST films with x = 0.88 reaches a maximum value of 1,900 cm2 V−1 s−1, which is comparable to the previous results24. These transport properties and the temperature dependence of the resistivity (Supplementary Information 10) ensure that the Fermi level of BST is systematically varied from n- to p-type across the DP in a controlled manner, as shown in Fig. 2d.

Figure 2: Transport properties of BST films and detected VSym as a function of Sb composition.
figure 2

a, Hall coefficient RH for BST films measured at 10 K. b, Mobility μ and carrier density n2D, p2D as a function of Sb composition at 10 K. c, Symmetric voltage of ST-FMR as a function of Sb composition. The input rf frequency and power are 7 GHz and 10 dBm, respectively. The error bars are the standard deviation from five samples with different dimensions. d, Schematic of the energy dispersion in BST. e,f, (Top) Spin accumulation due to a Fermi circle shift at the surface state of n-type (e) and p-type (f) BST. Solid and dashed circles are the Fermi circles with Ex and without Ex, respectively. (Bottom) Difference in the Fermi distribution (f − f0) on applying an electric field.

Full size image

Figure 2c shows the dependence of VSym on the Sb composition x obtained from an ST-FMR spectrum measured at an rf power of 8 mW. We confirmed that an rf power of 8 mW is low enough to ensure a linear response, with suppression of heating effects (Supplementary Information 5). The sign of VSym indicates the spin polarization direction of the spin current. We found a positive VSym in both n- and p-type BST films, which is an ideal feature of the C–S conversion via spin–momentum locking1,2,3,4,5,6. In the Dirac dispersion shown in Fig. 2e, f, spins on the Fermi circles of n- and p-type surface states of BSTs rotate clockwise and anti-clockwise, respectively24. When the electric field Ex is applied in the −x-direction, the Fermi circle with the chiral spin structure is shifted from the dashed circles to the solid circles by an amount proportional to Ex along kx, as shown in Fig. 2e. When the Fermi level EF is above the DP, the surface state of BST films has a higher population of down spins, generating spin polarization of the spin current along the −y-direction. When the Fermi level EF is in the valence band of the Dirac dispersion, up spins with momenta along +kx are fewer in number than down spins with −kx. Thus the accumulated spin is oriented along the same direction for both n- and p-type BST films. Note that these results are different from the case of a typical semiconductor such as GaAs25, whose spin Hall effect exhibits a different sign, depending on the carrier type.

The values of qICS and the spin current conductivity σS of BST films are summarized as a function of x in Fig. 3. The value of qICS can be experimentally evaluated from the ratio of VSym to VAnti in the ST-FMR spectrum. By using the conventional evaluation term θCS = JS/JC, with assumption of a uniform JC in the BST film regardless of EF position, large values of θCS are obtained for x = 0.5, 0.7 and 0.9, consistent with previous studies3 (Supplementary Information 9). Here, we propose a scheme for evaluation of qICS making use of jc. In the ST-FMR process, the values of VSym and VAnti correspond, respectively, to the spin-induced torque τ∥ and the Oersted-field-induced torque τ⊥ generated by charge current flow. These two torques per unit moment on the Py are respectively expressed as τ∥ = ℏJS/(2eμ0MStPy) and τ⊥ = ξ{JCCutCu/2 + jC/2}, where MS, t and ξ are the saturation magnetization, film thickness, and reduction factor of the rf field. Note that VAnti shows a sin2θcosθ dependence on the rotation angle of the applied magnetic field (Supplementary Information 6), indicating that VAnti originates purely from the Oersted field. The value of ξ is calculated numerically by means of a finite element method (see Supplementary Information 1). The value of qICS can thus be given by

where a is the ratio of JCCu (A m−2) to jC. The spin current density into Py JSPy (A m−2) is proportional to the spin accumulation at the surface state of the TI, 〈δS0〉, which is expressed as

where kF is the Fermi wavenumber, δkx is the shift of Fermi circle, and τ is the relaxation time. In the two-dimensional system, kF2 is proportional to the carrier density. Therefore, 〈δS0〉 reduces to ℏjC/2evF and qICS = JSPy/jC ∝ vF−1 is obtained. According to angle-resolved photoemission spectra, vF in BST increases slightly from 3.6 to 3.9 × 105 m s−1 as the Sb composition x increases from 0.5 to 0.9 (ref. 26), suggesting that qICS is almost constant in this composition range. For bulk insulating BST films with x = 0.5, 0.7 and 0.9 (apart from two samples in the vicinity of the DP) we observed that the values of qICS indeed lie within the range 0.45–0.57 nm−1, similar in magnitude to the previous results of ST-FMR measurements in Py/Bi2Se3 bilayer film3. Of particular interest is the case x = 0.9; although its VSym value is nearly half those of x = 0.5 and 0.7, as shown in Fig. 2c, the qICS value is still comparable owing to the correction by the small jC. Since we assume the surface thickness responsible for jC to be 1 nm, the value of θCS is estimated to be 45 to 57% for these BST films, yielding a much higher conversion efficiency than those in typical transition metals such as β-Ta (15%) and β-W (33%) (refs 10,11,12,13). Consequently, the interface C–S conversion effect via spin–momentum locking at the surface state is evaluated fairly well using qICS, and consistent with the naive expectation of an at most 100% conversion efficiency. Note that the estimated conversion efficiency θCS is proportional to the conducting channel thickness, which contributes to JC; therefore, the large values of θCS claimed for high conversion efficiencies in previous studies3,4 may be overestimated by assuming a thicker conducting layer. Our estimated values of qICS less than unity appears consistent with the theoretical evaluation, which has proposed a reduction of the in-plane spin polarization due to spin–orbit entanglement in such a material with a strong spin–orbit interaction27. We would also like to note that the product of qICS and the inverse conversion coefficient is expected to be approximately unity for the ideal case where there is no reduction of the in-plane spin polarization in the surface state of the TI28.

Figure 3: Dependence of the charge-to-spin current conversion efficiency of BST on the Sb composition.
figure 3

a, Interface C–S conversion efficiency qICS as a function of Sb composition. Inset shows the band structure and Fermi level position for each Sb composition. Bulk insulating BST with 0.50 ≤ x ≤ 0.90 should have only surface transport. Bi2Te3 (x = 0) and Bi2Sb3 (x = 1) have both bulk and surface conduction paths. Error bars represent the standard deviation over five samples with different dimensions. b, Spin current conductivity as a function of Sb composition.

Full size image

We now discuss the Fermi-level dependence of qICS shown in Fig. 3a. First, in contrast to the almost constant qICS for bulk insulating BST films with x = 0.5, 0.7 and 0.9, the values of qICS show a sharp dip around DP x ∼ 0.82 and 0.88; the value of qICS decreases dramatically when EF is located close to the DP, or equivalently kF becomes approximately zero, originating from the almost zero VSym in our experiments (see Fig. 2c, d). Evaluated from the lowest charge carrier density of approximately 1012 cm−2, the EF position is located within ±60 meV of the DP for x = 0.82 and 0.88, resulting in a small 〈δS0〉 due to the small δkx. In such a situation, with EF close to the DP, a finite amount of scattering may reduce the generated spin polarization, as reported in experiments with spin-resolved angular-dependent photoemission spectroscopy (ARPES)29,30,31 and scanning tunnelling spectroscopy32. Here we give possible reasons for the reduction in the spin polarization. First, when there are inhomogeneities, such as in the Bi/Sb composition, which can be regarded as analogy to electron–hole puddles in graphene33, in the surface state of BST around the Dirac point, charge current can flow in directions other than the electric field direction. As a result, 〈δS0〉 with various spin directions will occur in the surface state of the TI, indicating that the 〈δS0〉 in the y direction will decrease. Furthermore, if there is an additional surface state due to Cu deposition on the TIs, the generated spin polarization will be decreased (Supplementary Information 4). In this situation, it is likely that an ideal constant value of qICS cannot be recovered due to the almost zero Vsym with finite jC. Second, for bulk conductive BST films with x = 0 (Bi2Te3) and x = 1 (Sb2Te3), when we apply the same analytical method as used for bulk insulating films with an assumption of a 1 nm conducting surface layer, the estimated values of qICS are found to be roughly equal to, or even twice as much as, those for the bulk insulating BST films. However, a quantitative evaluation of qICS and θCS in bulk conducting samples is fairly difficult for the following reasons. The first reason is estimation of the effects of parasitic currents: here we assume that the surface is as conductive as the bulk (see Supplementary Information 1). Considering that the surface is expected to be more conductive, the present value of qICS might be overestimated. Although the assumption of a completely insulating bulk is simplistic, a small contribution from a bulk current provides a small perturbation to the present results; we have shown an insignificant effect of the current ratio between the surface and the bulk on the evaluated values of qICS (Supplementary Information 2). The second reason is the Rashba effect: if the charge current in the bulk band also contributes to JS via a Rashba-split band, as in Bi2Se3 (ref. 20), the opposite spin polarization may cancel part of the surface spin accumulation (see Supplementary Information 4). The final reason is the bulk spin Hall effect: the bulk charge current can also contribute to JS via the ordinary spin Hall effect, of which the sign is as yet unclear.

Finally we show the spin current conductivity σS, defined as σS = qICSσsurf, where σsurf is the conductivity of the surface state of the TI, as a function of the Sb composition x in Fig. 3b. The values of σS in bulk insulating BST (x = 0.5 and 0.7), excluding the DP and bulk conductive BST, take values close to 1.8 × 105 Ω−1 m−1, which are comparable to those reported for three-dimensional processes originating from the spin Hall effect in paramagnetic metals such as Pt (3.4 × 105 Ω−1 m−1) (ref. 10) and β-W (1.3 × 105 Ω−1 m−1) (ref. 11). This high value of σS is certainly beneficial, not only for realizing highly efficient magnetization switching, but also for realizing non-volatile spin switching for Boolean and non-Boolean logic initially based on metal spin Hall effects34.

Methods

Sample fabrication.

We grew 8-nm-thick BST films on semi-insulating InP(111) substrates by molecular beam epitaxy. The detailed growth conditions are described in a previous paper23. The Bi/Sb ratio was tuned by adjusting the ratio of the beam equivalent pressures of Bi and Sb. Resistivity and Hall effect measurements were carried out using small chips derived from the same samples as used for the ST-FMR measurements (see Supplementary Information 2). Thin films of 8-nm Cu/10-nm Ni80Fe20 (Py)/5-nm Al2O3 were grown on the BST films by e-beam evaporation at a pressure of 5 × 10−5 Pa. Al2O3 is used as an insulating capping layer. The resistivities of Cu and Py are measured to be 10 and 60 μΩ cm at 10 K. The BST/Cu/Py trilayer films were patterned into rectangular elements (10 × 30, 15 × 45, 20 × 60, 30 × 90, 40 × 120 μm2) using optical lithography and an Ar-ion etching technique. A co-planar waveguide of 5-nm Ti/200-nm Au was deposited on both sides of the rectangular elements.

ST-FMR measurement set-up.

An rf current with an input power of 8 mW is applied along the long edge of the rectangle by means of a microwave analog signal generator (Keysight: MXG N5183A). An external static magnetic field Hext in the range from 0 to 2.0 kOe is also applied in the film plane at an angle of θ = 45 ° with respect to the current flow direction. We demonstrated the rf power dependence of VSym, the heating effect, the dc current dependence of the resonance field, the frequency dependence of the half-width at half-maximum of Δ for VSym and the dependence on magnetic field angle of VAnti, and concluded that the detected VSym and VAnti are primarily due to the charge-to-spin conversion effect (Supplementary Information). All the experiments were performed at 10 K to measure the surface-dominant properties of TI.

Data availability.

The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.