Abstract
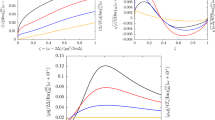
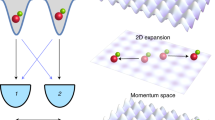
In dilute gases, a set of universal relations, known as the contact relations, directly connects thermodynamics and microscopic properties. So far, they have been established only for interactions with s-wave symmetry—that is, without relative angular momentum. Here we report measurements of two new physical quantities, the p-wave contacts, and, using recently proposed relations, present evidence that they encode the universal aspects of p-wave interactions. Our experiments use an ultracold Fermi gas of 40K, in which s-wave interactions are suppressed by polarizing the sample, whereas p-wave interactions are enhanced by working near a scattering resonance. Using time-resolved spectroscopy, we study how correlations in the system develop after quenching the atoms into an interacting state. By combining quasi-steady-state measurements with new contact relations, we infer an attractive p-wave interaction energy as large as half the Fermi energy. Our results reveal new ways to understand and characterize the properties of a resonant p-wave quantum gas.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Tan, S. Energetics of a strongly correlated Fermi gas. Ann. Phys. 323, 2952–2970 (2008).
Tan, S. Large momentum part of a strongly correlated Fermi gas. Ann. Phys. 323, 2971–2986 (2008).
Tan, S. Generalized virial theorem and pressure relation for a strongly correlated Fermi gas. Ann. Phys. 323, 2987–2990 (2008).
Werner, F., Tarruell, L. & Castin, Y. Number of closed-channel molecules in the BEC–BCS crossover. Eur. Phys. J. B 68, 401–415 (2009).
Zhang, S. & Leggett, A. J. Universal properties of the ultracold Fermi gas. Phys. Rev. A 79, 023601 (2009).
Braaten, E. in The BCS–BEC Crossover and the Unitary Fermi Gas (ed. Zwerger, W.) 193–231 (Springer, 2012).
Werner, F. & Castin, Y. General relations for quantum gases in two and three dimensions: two-component fermions. Phys. Rev. A 86, 013626 (2012).
Werner, F. & Castin, Y. General relations for quantum gases in two and three dimensions. II. Bosons and mixtures. Phys. Rev. A 86, 053633 (2012).
Wild, R. J., Makotyn, P., Pino, J. M., Cornell, E. A. & Jin, D. S. Measurements of Tan’s contact in an atomic Bose–Einstein condensate. Phys. Rev. Lett. 108, 145305 (2012).
Olshanii, M. & Dunjko, V. Short-distance correlation properties of the Lieb–Liniger system and momentum distributions of trapped one-dimensional atomic gases. Phys. Rev. Lett. 91, 090401 (2003).
Combescot, R., Alzetto, F. & Leyronas, X. Particle distribution tail and related energy formula. Phys. Rev. A 79, 053640 (2009).
Fröhlich, B. et al. Two-dimensional Fermi liquid with attractive interactions. Phys. Rev. Lett. 109, 130403 (2012).
Barth, M. & Zwerger, W. Tan relations in one dimension. Ann. Phys. 326, 2544–2565 (2011).
Weiss, R., Bazak, B. & Barnea, N. Nuclear neutron–proton contact and the photoabsorption cross section. Phys. Rev. Lett. 114, 012501 (2015).
DeMarco, B., Bohn, J. L., Burke, J. P., Holland, M. & Jin, D. S. Measurement of p-wave threshold law using evaporatively cooled fermionic atoms. Phys. Rev. Lett. 82, 4208–4211 (1999).
Regal, C. A., Ticknor, C., Bohn, J. L. & Jin, D. S. Tuning p-wave interactions in an ultracold Fermi gas of atoms. Phys. Rev. Lett. 90, 053201 (2003).
Zhang, J. et al. p-wave Feshbach resonances of ultracold 6Li. Phys. Rev. A 70, 030702 (2004).
Schunck, C. H. et al. Feshbach resonances in fermionic Li-6. Phys. Rev. A 71, 045601 (2005).
Kallin, C. Chiral p-wave order in Sr2RuO4 . Rep. Prog. Phys. 75, 042501 (2012).
Read, N. & Green, D. Paired states of fermions in two dimensions with breaking of parity and time-reversal symmetries and the fractional quantum Hall effect. Phys. Rev. B 61, 10267–10297 (2000).
Levinsen, J., Cooper, N. R. & Gurarie, V. Strongly resonant p-wave superfluids. Phys. Rev. Lett. 99, 210402 (2007).
Elliott, S. R. & Franz, M. Majorana fermions in nuclear, particle, and solid-state physics. Rev. Mod. Phys. 87, 137–163 (2015).
Inotani, D., Watanabe, R., Sigrist, M. & Ohashi, Y. Pseudogap phenomenon in an ultracold Fermi gas with a p-wave pairing interaction. Phys. Rev. A 85, 053628 (2012).
Yoshida, S. M. & Ueda, M. Universal high-momentum asymptote and thermodynamic relations in a spinless Fermi gas with a resonant p-wave interaction. Phys. Rev. Lett. 115, 135303 (2015).
Yu, Z., Thywissen, J. H. & Zhang, S. Universal relations for a Fermi gas close to a p-wave interaction resonance. Phys. Rev. Lett. 115, 135304 (2015).
He, M.-Y., Zhang, S.-L., Chan, H. M. & Zhou, Q. Concept of contact spectrum and its applications in atomic quantum Hall states. Phys. Rev. Lett. 116, 045301 (2016).
Zhang, P., Naidon, P. & Ueda, M. Scattering amplitude of ultracold atoms near the p-wave magnetic Feshbach resonance. Phys. Rev. A 82, 062712 (2010).
Ticknor, C., Regal, C. A., Jin, D. S. & Bohn, J. L. Multiplet structure of Feshbach resonances in nonzero partial waves. Phys. Rev. A 69, 042712 (2004).
Chin, C., Grimm, R., Julienne, P. & Tiesinga, E. Feshbach resonances in ultracold gases. Rev. Mod. Phys. 82, 1225–1286 (2010).
Jona-Lasinio, M., Pricoupenko, L. & Castin, Y. Three fully polarized fermions close to a p-wave Feshbach resonance. Phys. Rev. A 77, 043611 (2008).
Günter, K., Stöferle, T., Moritz, H., Köhl, M. & Esslinger, T. p-wave interactions in low-dimensional fermionic gases. Phys. Rev. Lett. 95, 230401 (2005).
Peng, S.-G., Tan, S. & Jiang, K. Manipulation of p-wave scattering of cold atoms in low dimensions using the magnetic field vector. Phys. Rev. Lett. 112, 250401 (2014).
Hazlett, E. L., Zhang, Y., Stites, R. W. & O’Hara, K. M. Realization of a resonant Fermi gas with a large effective range. Phys. Rev. Lett. 108, 045304 (2012).
Kohstall, C., Zaccanti, M., Jag, M. & Trenkwalder, A. Metastability and coherence of repulsive polarons in a strongly interacting Fermi mixture. Nature 485, 615–618 (2012).
Gaebler, J. P., Stewart, J. T., Bohn, J. L. & Jin, D. S. p-wave Feshbach molecules. Phys. Rev. Lett. 98, 200403 (2007).
Chin, C. & Julienne, P. S. Radio-frequency transitions on weakly bound ultracold molecules. Phys. Rev. A 71, 012713 (2005).
Pieri, P., Perali, A. & Strinati, G. C. Enhanced paraconductivity-like fluctuations in the radiofrequency spectra of ultracold Fermi atoms. Nature Phys. 5, 736–740 (2009).
Schneider, W. & Randeria, M. Universal short-distance structure of the single-particle spectral function of dilute Fermi gases. Phys. Rev. A 81, 021601 (2010).
Braaten, E., Kang, D. & Platter, L. Short-time operator product expansion for rf spectroscopy of a strongly interacting Fermi gas. Phys. Rev. Lett. 104, 223004 (2010).
Stewart, J. T., Gaebler, J. P., Drake, T. E. & Jin, D. S. Verification of universal relations in a strongly interacting Fermi gas. Phys. Rev. Lett. 104, 235301 (2010).
Chevy, F. et al. Resonant scattering properties close to a p-wave Feshbach resonance. Phys. Rev. A 71, 062710 (2005).
Inada, Y. et al. Collisional properties of p-wave Feshbach molecules. Phys. Rev. Lett. 101, 100401 (2008).
Nakasuji, T., Yoshida, J. & Mukaiyama, T. Experimental determination of p-wave scattering parameters in ultracold 6Li atoms. Phys. Rev. A 88, 012710 (2013).
Ohashi, Y. BCS–BEC crossover in a gas of Fermi atoms with a p-wave Feshbach resonance. Phys. Rev. Lett. 94, 050403 (2005).
Gurarie, V. & Radzihovsky, L. Resonantly paired fermionic superfluids. Ann. Phys. 322, 2–119 (2007).
Sagi, Y., Drake, T. E., Paudel, R. & Jin, D. S. Measurement of the homogeneous contact of a unitary Fermi gas. Phys. Rev. Lett. 109, 220402 (2012).
Pricoupenko, L. Modeling interactions for resonant p-wave scattering. Phys. Rev. Lett. 96, 050401 (2006).
Shenoy, V. B. & Ho, T.-L. Nature and properties of a repulsive Fermi gas in the upper branch of the energy spectrum. Phys. Rev. Lett. 107, 210401 (2011).
Fuchs, J. et al. Binding energies of 6Li p-wave Feshbach molecules. Phys. Rev. A 77, 053616 (2008).
Gubbels, K. B. & Stoof, H. T. C. Theory for p-wave Feshbach molecules. Phys. Rev. Lett. 99, 190406 (2007).
Jin, D. S., Gaebler, J. P. & Stewart, J. T. in Proceedings of the XVIII International Conference on Laser Spectroscopy (eds Hollberg, L., Bergquist, J. & Kasevich, M.) 127–137 (World Scientific, 2008).
Partridge, G. B., Strecker, K. E., Kamar, R. I., Jack, M. W. & Hulet, R. G. Molecular probe of pairing in the BEC–BCS crossover. Phys. Rev. Lett. 95, 020404 (2005).
Kuhnle, E. D. et al. Universal behavior of pair correlations in a strongly interacting Fermi gas. Phys. Rev. Lett. 105, 070402 (2010).
Navon, N., Nascimbène, S., Chevy, F. & Salomon, C. The equation of state of a low-temperature Fermi gas with tunable interactions. Science 328, 729–732 (2010).
Kuhnle, E. D. et al. Temperature dependence of the universal contact parameter in a unitary Fermi gas. Phys. Rev. Lett. 106, 170402 (2011).
Levinsen, J., Cooper, N. R. & Gurarie, V. Stability of fermionic gases close to a p-wave Feshbach resonance. Phys. Rev. A 78, 063616 (2008).
Acknowledgements
We thank F. Chevy for discussion and shared notes concerning F versus δBm. We also thank N. Zuber for experimental assistance, and J. Bohn, B. Ruzic, S. Tan, E. Taylor, P. Zhang and Q. Zhou for discussion. This work was supported by AFOSR under FA9550-13-1-0063, ARO under W911NF-15-1-0603, the Croucher Foundation, RGC under under 17306414, NKBRSFC, NSERC and NSFC under 11474179, and the Tsinghua University Initiative Scientific Research Program.
Author information
Authors and Affiliations
Contributions
C.L., S.S. and S.T. performed the experiments. C.L., S.S., S.T. and J.H.T. analysed the data. All authors contributed to the interpretation of the spectra. Z.Y., S.T. and S.Z. developed the two-channel model of the dynamics. All authors contributed to the preparation of the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 544 kb)
Rights and permissions
About this article
Cite this article
Luciuk, C., Trotzky, S., Smale, S. et al. Evidence for universal relations describing a gas with p-wave interactions. Nature Phys 12, 599–605 (2016). https://doi.org/10.1038/nphys3670
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys3670
This article is cited by
-
Thermally stable p-wave repulsive Fermi polaron without a two-body bound state
AAPPS Bulletin (2023)
-
Heuristic machinery for thermodynamic studies of SU(N) fermions with neural networks
Nature Communications (2021)
-
Spectroscopic probes of quantum gases
Nature Physics (2021)
-
Degenerate Bose gases near a d-wave shape resonance
Nature Physics (2019)
-
Fermion superfluid with hybridized s- and p-wave pairings
Science China Physics, Mechanics & Astronomy (2017)