Abstract
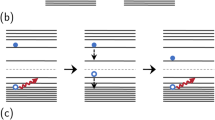
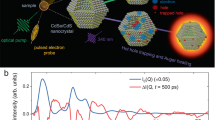
The rate at which excited charge carriers relax to their equilibrium state affects many aspects of the performance of nanoscale devices, including switching speed, carrier mobility and luminescence efficiency. A better understanding of the processes that govern carrier relaxation therefore has important technological implications. A significant increase in carrier–carrier interactions caused by strong spatial confinement of electronic excitations in semiconductor nanostructures leads to a considerable enhancement of Auger effects, which can further result in unusual, Auger-process-controlled recombination and energy relaxation regimes. Here, we report the first experimental observation of efficient Auger heating in CdSe quantum rods at high pump intensities, leading to a strong reduction of carrier cooling rates. In this regime, the carrier temperature is determined by the balance between energy outflow through phonon emission and energy inflow because of Auger heating. This equilibrium results in peculiar carrier cooling dynamics that closely correlate with recombination dynamics, an effect never seen before in bulk or nanoscale semiconductors.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Alivisatos, A. P. Semiconductor clusters, nanocrystals, and quantum dots. Science 271, 933–937 (1996).
Efros, Al. L., Kharchenko, V. A. & Rosen, M. Breaking the phonon bottleneck in nanometer quantum dots: Role of Auger-like processes. Solid State Commun. 93, 281–284 (1995).
Klimov, V. I. & McBranch, D. W. Femtosecond 1P-to-1S electron relaxation in strongly confined semiconductor nanocrystals. Phys. Rev. Lett. 80, 4028–4031 (1998).
Guyot-Sionnest, P., Shim, M., Matranga, C. & Hines, M. Intraband relaxation in CdSe quantum dots. Phys. Rev. B 60, R2181–R2184 (1999).
Mohamed, M. B., Burda, C. & El-Sayed, M. A. Shape dependent ultrafast relaxation dynamics of CdSe nanocrystals: Nanorods vs nanodots. Nano Lett. 1, 589–593 (2001).
Chepic, D. I. et al. Auger ionization of semiconductor quantum drops in a glass matrix. J. Lumin. 47, 113–127 (1990).
Klimov, V. I., Mikhailovsky, A. A., McBranch, D. W., Leatherdale, C. A. & Bawendi, M. G. Quantization of multiparticle Auger rates in semiconductor quantum dots. Science 287, 1011–1013 (2000).
Htoon, H., Hollingsworth, J. A., Dickerson, R. & Klimov, V. I. Effect of zero-dimensional to one-dimensional transformation on Auger recombination in semiconductor quantum rods. Phys. Rev. Lett. 91, 227401 (2003).
Wang, L.-W., Califano, M., Zunger, A. & Franceschetti, A. Pseudopotential theory of Auger processes in CdSe quantum dots. Phys. Rev. Lett. 91, 056404 (2003).
Downer, M. C. & Shank, C. V. Ultrafast heating of silicon on sapphire by femtosecond optical pulses. Phys. Rev. Lett. 56, 761–764 (1986).
Borri, P., Ceccherini, S., Gurioli, M. & Bogani, F. Auger heating of carriers in GaAs/AlAs heterostructures. Solid State Commun. 103, 77–81 (1997).
Klimov, V., Haring Bolivar, P. & Kurz, H. Hot-phonon effects in femtosecond luminescence spectra of electron-hole plasmas in CdS. Phys. Rev. B 52, 4728–4731 (1995).
Murray, C. B., Norris, D. J. & Bawendi, M. G. Synthesis and characterization of nearly monodisperse CdE (E=S,Se,Te) semiconductor nanocrystallites. J. Am. Chem. Soc. 115, 8706–8715 (1993).
Manna, L., Scher, E. C. & Alivisatos, A. P. Synthesis of soluble and processable rod-, arrow-, teardrop-, and tetrapod-shaped CdSe nanocrystals. J. Am. Chem. Soc. 122, 12700–12706 (2000).
Peng, Z. A. & Peng, X. Mechanisms of the shape evolution of CdSe nanocrystals. J. Am. Chem. Soc. 123, 1389–1395 (2001).
Shah, J. Ultrafast luminescence spectroscopy using sum frequency generation. IEEE J. Quantum Electron. 24, 276–288 (1988).
Achermann, M., Hollingsworth, J. A. & Klimov, V. I. Multiexcitons confined within a subexcitonic volume: Spectroscopic and dynamical signatures of neutral and charged biexcitons in ultrasmall semiconductor nanocrystals. Phys. Rev. B 68, 245302 (2003).
Xu, S., Mikhailovsky, A. A., Hollingsworth, J. A. & Klimov, V. I. Hole intraband relaxation in strongly confined quantum dots: Revisiting the 'phonon bottleneck' problem. Phys. Rev. B 65, 045319 (2002).
Pötz, W. & Kocevar, P. Electronic power transfer in pulsed laser excitation of polar semiconductors. Phys. Rev. B 28, 7040–7047 (1983).
Kocevar, P. Hot phonon dynamics. Physica B 134, 155–163 (1985).
Acknowledgements
The authors thank Dr. H. Htoon for useful discussions. This work was supported by Los Alamos LDRD Funds and the Chemical Sciences, Biosciences, and Geosciences Division of the Office of Basic Energy Sciences, Office of Science, U.S. Department of Energy.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
About this article
Cite this article
Achermann, M., Bartko, A., Hollingsworth, J. et al. The effect of Auger heating on intraband carrier relaxation in semiconductor quantum rods. Nature Phys 2, 557–561 (2006). https://doi.org/10.1038/nphys363
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys363
This article is cited by
-
Anisotropic carrier dynamics and laser-fabricated luminescent patterns on oriented single-crystal perovskite wafers
Nature Communications (2024)
-
Gain roll-off in cadmium selenide colloidal quantum wells under intense optical excitation
Scientific Reports (2022)
-
Prospects and challenges of colloidal quantum dot laser diodes
Nature Photonics (2021)
-
Colloidal quantum dot lasers
Nature Reviews Materials (2021)
-
Dynamic lattice distortions driven by surface trapping in semiconductor nanocrystals
Nature Communications (2021)