Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Towards quantum thermodynamics in electronic circuits

Nature Physics volume 11, pages 118–123 (2015)Cite this article

Subjects

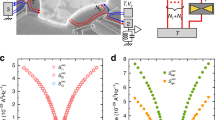
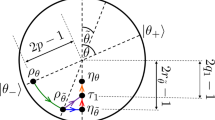
Abstract

Electronic circuits operating at sub-kelvin temperatures are attractive candidates for studying classical and quantum thermodynamics: their temperature can be controlled and measured locally with exquisite precision, and they allow experiments with large statistical samples. The availability and rapid development of devices such as quantum dots, single-electron boxes and superconducting qubits only enhance their appeal. But although these systems provide fertile ground for studying heat transport, entropy production and work in the context of quantum mechanics, the field remains in its infancy experimentally. Here, we review some recent experiments on quantum heat transport, fluctuation relations and implementations of Maxwell’s demon, revealing the rich physics yet to be fully probed in these systems.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Dissipation and relaxation in electronic circuits at low temperatures.
Figure 2: Testing the fluctuation theorem in equation (1) experimentally.
Figure 3: Experimental realization of the Jarzynski fluctuation relation.
Figure 4: Experimental implementation of Maxwell’s demon.

References

  1. Seifert, U. Stochastic thermodynamics, fluctuation theorems, and molecular machines. Rep. Prog. Phys. 75, 126001 (2012).

    Article  ADS  Google Scholar 

  2. Alemany, A. & Ritort, F. Fluctuation theorems in small systems: Extending thermodynamics to the nanoscale. Europhys. News 41, 27–30 (2010).

    Article  Google Scholar 

  3. Alemany, A., Ribezzi, M. & Ritort, F. in Nonequilibrium Statistical Physics of Small Systems: Fluctuation Relations and Beyond (eds Klages, R., Just, W. & Jarzynski, C.) (Wiley-VCH, 2012).

    Google Scholar 

  4. Giazotto, F., Heikkilä, T. T., Luukanen, A., Savin, A. M. & Pekola, J. P. Opportunities for mesoscopics in thermometry and refrigeration: Physics and applications. Rev. Mod. Phys. 78, 217–274 (2006).

    Article  ADS  Google Scholar 

  5. Courtois, H., Hekking, F. W. J., Nguyen, H. Q. & Winkelmann, C. Electronic coolers based on superconducting tunnel junctions: Fundamentals and applications. J. Low Temp. Phys. 175, 799–812 (2014).

    Article  ADS  Google Scholar 

  6. Prance, J. R. et al. Electronic refrigeration of a two-dimensional electron gas. Phys. Rev. Lett. 102, 146602 (2009).

    Article  ADS  Google Scholar 

  7. Pendry, J. B. Quantum limits to flow of information and entropy. J. Phys. A 16, 21612171 (1983).

    Article  MathSciNet  Google Scholar 

  8. Schwab, K., Henriksen, E. A., Worlock, J. M. & Roukes, M. L. Measurement of the quantum of thermal conductance. Nature 404, 974–977 (2000).

    Article  ADS  Google Scholar 

  9. Meschke, M., Guichard, W. & Pekola, J. P. Single-mode heat conduction by photons. Nature 444, 187–190 (2006).

    Article  ADS  Google Scholar 

  10. Timofeev, A. V., Helle, M., Meschke, M., Möttönen, M. & Pekola, J. P. Electronic refrigeration at the quantum limit. Phys. Rev. Lett. 102, 200801 (2009).

    Article  ADS  Google Scholar 

  11. Ciliberto, S., Imparato, A., Naert, A. & Tanase, M. Heat flux and entropy produced by thermal fluctuations. Phys. Rev. Lett. 110, 180601 (2013).

    Article  ADS  Google Scholar 

  12. Jezouin, S. et al. Quantum limit of heat flow across a single electronic channel. Science 342, 601–604 (2013).

    Article  ADS  MathSciNet  Google Scholar 

  13. Blanter, Y. M. & Büttiker, M. Shot noise in mesoscopic conductors. Phys. Rep. 336, 1–166 (2000).

    Article  ADS  Google Scholar 

  14. Averin, D. V. & Pekola, J. P. Violation of the fluctuation-dissipation theorem in time-dependent mesoscopic heat transport. Phys. Rev. Lett. 104, 220601 (2010).

    Article  ADS  Google Scholar 

  15. Sergi, D. Energy transport and fluctuations in small conductors. Phys. Rev. B 83, 033401 (2011).

    Article  ADS  Google Scholar 

  16. Zhan, F., Denisov, S. & Hänggi, P. Power spectrum of electronic heat current fluctuations. Phys. Status Solidi B 250, 2355–2364 (2013).

    Article  ADS  Google Scholar 

  17. Evans, D. J., Cohen, E. G. D. & Morriss, G. P. Probability of second law violations in shearing steady states. Phys. Rev. Lett. 71, 2401–2404 (1993).

    Article  ADS  Google Scholar 

  18. Utsumi, Y. et al. Bidirectional single-electron counting and the fluctuation theorem. Phys. Rev. B 81, 125331 (2010).

    Article  ADS  Google Scholar 

  19. Küng, B. et al. Irreversibility on the level of single-electron tunneling. Phys. Rev. X 2, 011001 (2012).

    Google Scholar 

  20. Cuetara, G. B., Esposito, M., Schaller, G. & Gaspard, P. Effective fluctuation theorems for electron transport in a double quantum dot coupled to a quantum point contact. Phys. Rev. B 88, 115134 (2013).

    Article  ADS  Google Scholar 

  21. Golubev, D. S., Utsumi, Y., Marthaler, M. & Schön, G. Fluctuation theorem for a double quantum dot coupled to a point-contact electrometer. Phys. Rev. B 84, 075323 (2011).

    Article  ADS  Google Scholar 

  22. Nakamura, S. et al. Nonequilibrium fluctuation relations in a quantum coherent conductor. Phys. Rev. Lett. 104, 080602 (2010).

    Article  ADS  Google Scholar 

  23. Jarzynski, C. Nonequilibrium equality for free energy differences. Phys. Rev. Lett. 78, 2690–2693 (1997).

    Article  ADS  Google Scholar 

  24. Crooks, G. E. Entropy production fluctuation theorem and the nonequilibrium work relation for free energy differences. Phys. Rev. E 60, 2721–2726 (1999).

    Article  ADS  Google Scholar 

  25. Büttiker, M. Zero-current persistent potential drop across small-capacitance Josephson junctions. Phys. Rev. B 36, 3548–3555 (1987).

    Article  ADS  Google Scholar 

  26. Averin, D. V. & Likharev, K. K. in Mesoscopic Phenomena in Solids (eds Altshuler, B. L., Lee, P. A. & Webb, R. A.) 173–271 (Elsevier, 1991).

    Book  Google Scholar 

  27. Lafarge, P. et al. Direct observation of macroscopic charge quantization. Z. Phys. B 85, 327–332 (1991).

    Article  ADS  Google Scholar 

  28. Averin, D. V. & Pekola, J. P. Statistics of the dissipated energy in driven single-electron transitions. Europhys. Lett. 96, 67004 (2011).

    Article  ADS  Google Scholar 

  29. Saira, O-P. et al. Test of Jarzynski and Crooks fluctuation relations in an electronic system. Phys. Rev. Lett. 109, 180601 (2012).

    Article  ADS  Google Scholar 

  30. Koski, J. V. et al. Distribution of entropy production in a single-electron box. Nature Phys. 9, 644–648 (2013).

    Article  ADS  Google Scholar 

  31. Seifert, U. Entropy production along a stochastic trajectory and an integral fluctuation theorem. Phys. Rev. Lett. 95, 040602 (2005).

    Article  ADS  Google Scholar 

  32. Toyabe, S., Sagawa, T., Ueda, M., Muneyuki, E. & Sano, M. Experimental demonstration of information-to-energy conversion and validation of the generalized Jarzynski equality. Nature Phys. 6, 988–992 (2010).

    Article  ADS  Google Scholar 

  33. Strasberg, P., Schaller, G., Brandes, T. & Esposito, M. Thermodynamics of a physical model implementing a Maxwell Demon. Phys. Rev. Lett. 110, 040601 (2013).

    Article  ADS  Google Scholar 

  34. Koski, J. V., Maisi, V. F., Pekola, J. P. & Averin, D. V. Experimental realization of a Szilard engine with a single electron. Proc. Natl Acad. Sci. USA 111, 13786–13789 (2014).

    Article  ADS  Google Scholar 

  35. Koski, J. V., Maisi, V. F., Sagawa, T. & Pekola, J. P. Experimental observation of the role of mutual information in the nonequilibrium dynamics of a Maxwell Demon. Phys. Rev. Lett. 113, 030601 (2014).

    Article  ADS  Google Scholar 

  36. Landauer, R. Irreversibility and heat generation in the computing process. IBM Res. J. Dev. 5, 183–191 (1961).

    Article  MathSciNet  Google Scholar 

  37. Bérut, A. et al. Experimental verification of Landauer’s principle linking information and thermodynamics. Nature 483, 187–189 (2012).

    Article  ADS  Google Scholar 

  38. Sagawa, T. & Ueda, M. Generalized Jarzynski equality under nonequilibrium feedback control. Phys. Rev. Lett. 104, 090602 (2010).

    Article  ADS  Google Scholar 

  39. Kurchan, J. A quantum fluctuation theorem. Preprint at http://arxiv.org/abs/cond-mat/0007360 (2000).

  40. Talkner, P., Lutz, E. & Hänggi, P. Fluctuation theorems: Work is not an observable. Phys. Rev. E 75, 050102(R) (2007).

    Article  ADS  Google Scholar 

  41. Esposito, M., Harbola, U. & Mukamel, S. Nonequilibrium fluctuations, fluctuation theorems, and counting statistics in quantum systems. Rev. Mod. Phys. 81, 1665–1702 (2009).

    Article  ADS  MathSciNet  Google Scholar 

  42. Campisi, M., Hänggi, P. & Talkner, P. Colloquium: Quantum fluctuation relations: Foundations and applications. Rev. Mod. Phys. 83, 771–791 (2011).

    Article  ADS  Google Scholar 

  43. Makhlin, Y., Schön, G. & Shnirman, A. Quantum-state engineering with Josephson-junction devices. Rev. Mod. Phys. 73, 357–400 (2001).

    Article  ADS  Google Scholar 

  44. Clarke, J. & Wilhelm, F. K. Superconducting quantum bits. Nature 453, 1031–1042 (2008).

    Article  ADS  Google Scholar 

  45. Pekola, J. P., Solinas, P., Shnirman, A. & Averin, D. V. Calorimetric measurement of work in a quantum system. New J. Phys. 15, 115006 (2013).

    Article  ADS  Google Scholar 

  46. Schmidt, D. R., Yung, C. S. & Cleland, A. N. Nanoscale radio-frequency thermometry. Appl. Phys. Lett. 83, 1002–1004 (2003).

    Article  ADS  Google Scholar 

  47. Govenius, J. et al. Microwave nanobolometer based on proximity Josephson junctions. Phys. Rev. B 90, 064505 (2014).

    Article  ADS  Google Scholar 

  48. Gasparinetti, S. et al. Fast electron thermometry towards ultra-sensitive calorimetric detection. Preprint at http://arxiv.org/abs/1405.7568 (2014).

  49. Dorner, R. et al. Extracting quantum work statistics and fluctuation theorems by single-qubit interferometry. Phys. Rev. Lett. 110, 230601 (2013).

    Article  ADS  Google Scholar 

  50. Mazzola, L., Chiara, G. D. & Paternostro, M. Measuring the characteristic function of the work distribution. Phys. Rev. Lett. 110, 230602 (2013).

    Article  ADS  Google Scholar 

  51. Campisi, M., Blattmann, R., Kohler, S., Zueco, D. & Hänggi, P. Employing circuit QED to measure nonequilibrium work fluctuations. New J. Phys. 15, 105028 (2013).

    Article  ADS  Google Scholar 

  52. Van den Broeck, C. & Kawai, R. Brownian refrigerator. Phys. Rev. Lett. 96, 210601 (2006).

    Article  ADS  MathSciNet  Google Scholar 

  53. Pekola, J. P. & Hekking, F. W. J. Normal-metal-superconductor tunnel junction as a brownian refrigerator. Phys. Rev. Lett. 98, 210604 (2007).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

I thank J. Koski and M. Campisi for comments on the manuscript, and A. Feshchenko for providing illustration material. This work has been supported in part by the European Union Seventh Framework Programme INFERNOS (FP7/2007-2013) under grant agreement no. 308850, and by Academy of Finland (projects 250280 and 272218).

Author information

Authors and Affiliations

  1. Department of Applied Physics, Low Temperature Laboratory, Aalto University School of Science, PO Box 13500, 00076 Aalto, Finland

    Jukka P. Pekola

Authors
  1. Jukka P. Pekola
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jukka P. Pekola.

Ethics declarations

Competing interests

The author declares no competing financial interests.

Rights and permissions

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Pekola, J. Towards quantum thermodynamics in electronic circuits. Nature Phys 11, 118–123 (2015). https://doi.org/10.1038/nphys3169

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nphys3169

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing