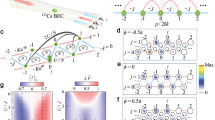
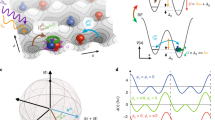
Abstract
Magnetism plays a key role in modern science and technology, but still many open questions arise from the interplay of magnetic many-body interactions. Deeper insight into complex magnetic behaviour and the nature of magnetic phase transitions can be obtained from, for example, model systems of coupled XY and Ising spins. Here, we report on the experimental realization of such a coupled system with ultracold atoms in triangular optical lattices. This is accomplished by imposing an artificial gauge field on the neutral atoms, which acts on them as a magnetic field does on charged particles. As a result, the atoms show persistent circular currents, the direction of which provides an Ising variable. On this, the tunable staggered gauge field, generated by a periodic driving of the lattice, acts as a longitudinal field. Further, the superfluid ground state presents strong analogies with the paradigm example of the fully frustrated XY model on a triangular lattice.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Villain, J. Spin glass with non-random interactions. J. Phys. C 10, 1717–1735 (1977).
Yosefin, M. & Domany, E. Phase transitions in fully frustrated spin systems. Phys. Rev. B 32, 1778–1795 (1985).
Choi, M. Y. & Doniach, S. Phase transitions in uniformly frustrated XY models. Phys. Rev. B 31, 4516–4526 (1985).
Sachdev, S. Quantum Phase Transitions 2nd edn (Cambridge Univ. Press, 2011).
Diep, H. T. (ed.) Frustrated Spin Systems (World Scientific, 2004).
Hasenbusch, M., Pelissetto, A. & Vicari, E. Multicritical behavior in the fully frustrated XY model and related systems. J. Stat. Mech. 2005, P12002 (December 005).
Ling, X. S. et al. Nature of phase transitions of superconducting wire networks in a magnetic field. Phys. Rev. Lett. 76, 2989–2992 (1996).
Martinoli, P. & Leemann, C. Two dimensional Josephson junction arrays. J. Low Temp. Phys. 118, 699–731 (2000).
Affolter, J., Tesei, M., Pastoriza, H., Leemann, C. & Martinoli, P. Observation of Ising-like critical fluctuations in frustrated Josephson junction arrays with modulated coupling energies. Physica C 369, 313–316 (2002).
Bloch, I., Dalibard, J. & Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 80, 885–964 (2008).
Lewenstein, M., Sanpera, A. & Ahufinger, V. Ultracold Atoms in Optical Lattices Simulating Quantum Many-body Systems (Oxford Univ. Press, 2012).
Schweikhard, V. et al. Vortex-lattice dynamics in rotating spinor Bose-Einstein condensates. Phys. Rev. Lett. 93, 210403 (2004).
Bretin, V., Stock, S., Seurin, Y. & Dalibard, J. Fast rotation of a Bose–Einstein condensate. Phys. Rev. Lett. 92, 050403 (2004).
Lin, Y-J., Compton, R. L., Jiménez-Garcìa, K., Porto, J. V. & Spielman, I. B. Synthetic magnetic fields for ultracold neutral atoms. Nature 462, 628–632 (2009).
Lin, Y-J. et al. A synthetic electric force acting on neutral atoms. Nature Phys. 7, 531–534 (2011).
Aidelsburger, M. et al. Experimental realization of strong effective magnetic fields in an optical lattice. Phys. Rev. Lett. 107, 255301 (2011).
Jiménez-García, K. et al. Peierls substitution in an engineered lattice potential. Phys. Rev. Lett. 108, 225303 (2012).
Struck, J. et al. Quantum simulation of frustrated classical magnetism in triangular optical lattices. Science 333, 996–999 (2011).
Struck, J. et al. Tunable gauge potential for neutral and spinless particles in driven optical lattices. Phys. Rev. Lett. 108, 225304 (2012).
Becker, C. et al. Ultracold quantum gases in triangular optical lattices. New J. Phys. 12, 065025 (2010).
Pitaevskii, Lev. P. & Stringari, S. Bose-Einstein Condensation (Oxford Univ. Press, 2003).
Arimondo, E., Ciampini, D., Eckardt, A., Holthaus, M. & Morsch, O. in Advances In Atomic, Molecular, and Optical Physics Vol. 61 (eds Paul Berman, E. A. & Lin, C.) Ch. 10 (Academic, 2012).
Huang, K., Yang, C. N. & Luttinger, J. M. Imperfect Bose gas with hard-sphere interaction. Phys. Rev. 105, 776–784 (1957).
Korshunov, S. E. Kink pairs unbinding on domain walls and the sequence of phase transitions in fully frustrated XY models. Phys. Rev. Lett. 88, 167007 (2002).
Lee, S. & Lee, K-C. Phase transitions in the fully frustrated triangular XY model. Phys. Rev. B 57, 8472–8477 (1998).
Capriotti, L., Vaia, R., Cuccoli, A. & Tognetti, V. Phase transitions induced by easy-plane anisotropy in the classical Heisenberg antiferromagnet on a triangular lattice: A Monte Carlo simulation. Phys. Rev. B 58, 273–281 (1998).
Okumura, S., Yoshino, H. & Kawamura, H. Spin-chirality decoupling and critical properties of a two-dimensional fully frustrated XY model. Phys. Rev. B 83, 094429 (2011).
Obuchi, T. & Kawamura, H. Spin and chiral orderings of the antiferromagnetic XY model on the triangular lattice and their critical properties. J. Phys. Soc. Jpn 81, 054003 (2012).
Hauke, P. et al. Non-abelian gauge fields and topological insulators in shaken optical lattices. Phys. Rev. Lett. 109, 145301 (2012).
Eckardt, A. et al. Frustrated quantum antiferromagnetism with ultracold bosons in a triangular lattice. Europhys. Lett. 89, 10010 (2010).
Simon, J. et al. Quantum simulation of antiferromagnetic spin chains in an optical lattice. Nature 472, 307–312 (2011).
Trotzky, S. et al. Time-resolved observation and control of superexchange interactions with ultracold atoms in optical lattices. Science 319, 295–299 (2008).
Greif, D., Uehlinger, T., Jotzu, G., Tarruell, L. & Esslinger, T. Short-range quantum magnetism of ultracold fermions in an optical lattice. Science 340, 1307–1310 (2013).
Parker, et al. In situ observation of strongly interacting ferromagnetic domains in a shaken optical lattice. Preprint at http://arxiv.org/abs/1305.5487 (2013).
Eckardt, A., Weiss, C. & Holthaus, M. Superfluid-insulator transition in a periodically driven optical lattice. Phys. Rev. Lett. 95, 260404 (2005).
Acknowledgements
We acknowledge support from the Deutsche Forschungsgemeinschaft (GRK1355, SFB925) and the Landesexzellenzinitiative Hamburg (supported by the Joachim Herz Stiftung), ERC AdG QUAGATUA, AAII-Hubbard, Spanish MICINN (FIS2008-00784), Catalunya-Caixa, EU Projects AQUTE and NAMEQUAM, the Spanish foundation Universidad.es, the Austrian Science Fund (SFB F40 FOQUS), the DARPA OLE program and the John von Neumann Institute for Computing (NIC) for providing us with computing time on the supercomputers of the Juelich Supercomputing Centre (JSC).
Author information
Authors and Affiliations
Contributions
J. Struck, M.W., C.Ö., P.W., J. Simonet and K.S. proposed the study and performed the experimental work. R.H., P.H., A.E, M.L. and L.M. performed the theoretical work.
All authors discussed the experimental as well as the theoretical results and contributed to writing the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 1362 kb)
Rights and permissions
About this article
Cite this article
Struck, J., Weinberg, M., Ölschläger, C. et al. Engineering Ising-XY spin-models in a triangular lattice using tunable artificial gauge fields. Nature Phys 9, 738–743 (2013). https://doi.org/10.1038/nphys2750
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys2750
This article is cited by
-
Observation of frustrated chiral dynamics in an interacting triangular flux ladder
Nature Communications (2023)
-
Realizing discontinuous quantum phase transitions in a strongly correlated driven optical lattice
Nature Physics (2022)
-
Strain and pseudo-magnetic fields in optical lattices from density-assisted tunneling
Communications Physics (2022)
-
Dispersive and dissipative coupling of photon Bose-Einstein condensates
Communications Physics (2022)
-
Multidimensional hyperspin machine
Nature Communications (2022)