Abstract
Quantum entanglement1,2 plays a vital role in many quantum-information and communication tasks3. Entangled states of higher-dimensional systems are of great interest owing to the extended possibilities they provide. For example, they enable the realization of new types of quantum information scheme that can offer higher-information-density coding and greater resilience to errors than can be achieved with entangled two-dimensional systems (see ref. 4 and references therein). Closing the detection loophole in Bell test experiments is also more experimentally feasible when higher-dimensional entangled systems are used5. We have measured previously untested correlations between two photons to experimentally demonstrate high-dimensional entangled states. We obtain violations of Bell-type inequalities generalized to d-dimensional systems6 up to d=12. Furthermore, the violations are strong enough to indicate genuine 11-dimensional entanglement. Our experiments use photons entangled in orbital angular momentum7, generated through spontaneous parametric down-conversion8,9, and manipulated using computer-controlled holograms.
Similar content being viewed by others
Main
Quantum-information tasks requiring high-dimensional bipartite entanglement include teleportation using qudits10,11, generalized dense coding (that is, with pairs of entangled d-level systems; ref. 12) and some quantum key distribution protocols13. More generally, schemes such as quantum secret sharing14 and measurement-based quantum computation15 apply multiparticle entanglement. These are promising applications, especially in view of recent progress in the development of quantum repeaters (see ref. 16 and references therein). However, practical applications of such protocols are only conceivable when it is possible to experimentally prepare, and moreover detect, high-dimensional entangled states. Therefore, the ability to verify high-dimensional entanglement between physical qudits is of crucial importance. Indeed, much progress has generally been made on the generation and detection of high-dimensional entangled states (please see ref. 17 and references within).
Here we report the experimental investigation of high-dimensional, two-photon entangled states. We focus on photon orbital angular momentum (OAM) entangled states generated by spontaneous parametric down-conversion (SPDC), and demonstrate genuine high-dimensional entanglement using violations of generalized Bell-type inequalities6. Previously, qutrit Bell-type tests have been carried out using photon OAM to verify three-dimensional entanglement (see ref. 18 and references within). In addition to testing whether correlations in nature can be explained by local realist theories19, the violation of Bell-type inequalities may be used to demonstrate the presence of entanglement. Bell-type experiments have been carried out using two-dimensional subspaces of the OAM state space of photons20,21 and experimentalists have demonstrated two-dimensional entanglement using up to 20 different two-dimensional subspaces22. Careful studies have also been carried out to describe how specific detector characteristics bound the dimensionality of the measured OAM states in photons generated by SPDC using Shannon dimensionality23.
Our experimental study of high-dimensional entanglement is based on the theoretical work of Collins et al. 6, which was applied in experiments for qutrits encoded in the OAM states of photons18,24. We encode qudits using the OAM states of photons, with eigenstates defined by the azimuthal index ℓ. These states arise from the solution of the paraxial wave equation in its cylindrical co-ordinate representation, and are the Laguerre–Gaussian modes L Gp,ℓ, so called because they are light beams with a Laguerre–Gaussian amplitude distribution.
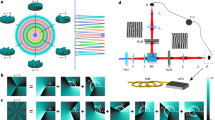
In our set-up (Fig. 1), OAM entangled photons are generated through a frequency-degenerate type-I SPDC process, and the OAM state is manipulated with computer-controlled spatial light modulators (SLMs) acting as reconfigurable holograms. Conservation of angular momentum ensures that, if the signal photon is in the mode specified by |ℓ〉, the corresponding idler photon can only be in the mode |−ℓ〉. Assuming that angular momentum is conserved9, a pure state of the two-photon field produced will have the form

where subscripts A and B label the signal and idler photons respectively, |cℓ|2 is the probability to create a photon pair with OAM ±ℓℏ and |ℓ〉 is the OAM eigenmode with mode number ℓ.
It has been shown6 that, for correlations that can be described by theories based on local realism1, a family of Bell-type parameters Sd satisfies the inequalities

Alternatively, if quantum mechanics is assumed to hold, then the violation of an inequality of type (1) indicates the presence of entanglement. Sd can be expressed as the expectation value of a quantum mechanical observable, which we denote as  . The expressions for Sd,
. The expressions for Sd,  and the operators
and the operators  and
and  are provided in Supplementary Section SI.
are provided in Supplementary Section SI.
The parameters Sd are calculated using coincidence probabilities for measurements made locally by two observers, Alice and Bob, on their respective subsystems, which in our case are the signal and idler photons from the SPDC source. Alice’s detector has two settings labelled by a∈{0,1} with d outcomes for each setting, and similarly for Bob’s detector with settings b∈{0,1}. The measurement bases corresponding to the detector settings of Alice and Bob are defined as


where v and w both run from 0 to d−1 and denote the outcomes of Alice’s and Bob’s measurements respectively, and the parameters α0=0, α1=1/2, β0=1/4 and β1=−1/4.
The measurement bases {|v〉aA} and {|w〉aA} have been shown5,25 to maximize the violations of inequality (1) for the maximally entangled state of two d-dimensional systems given by  It turns out that we are able to parameterize these d-dimensional measurement basis states with ‘mode analyser’ angles θA and θB, and write them in the form
It turns out that we are able to parameterize these d-dimensional measurement basis states with ‘mode analyser’ angles θA and θB, and write them in the form


where


The function g(ℓ) is defined as

where [x] is the integer part of x, and u(ℓ) is the discrete unit step function.
Figure 2 shows an example of the experimental data points for the self-normalized coincidence rates as function of the relative angle (θA−θB) using d=11 (see also Supplementary Fig. S1). For a maximally entangled state

where h(ℓ)=1 for all ℓ when d is odd, and h(ℓ≠0)=1, h(0)=1 when d is even, the coincidence rate of detecting one photon in state |θA〉 and the other in state |θB〉 is proportional to

The key result of our paper is shown in Fig. 3, which shows a plot of experimental values of parameter Sd as a function of the number of dimensions d. The plot compares theoretically predicted violations for a maximally entangled state, the experimental readings and the local hidden variable (LHV) limit. The maximum possible violations (shown in Supplementary Table S1) are slightly larger than the corresponding violations produced by a maximally entangled state. Violations persist up to as much as d=12 when entanglement concentration26 is applied. We find S11=2.39±0.07 and S12=2.24±0.08, which clearly violate Sd≤2 (see also Supplementary Table S4). In the corresponding experiment using L Gp,ℓ modes with only p=0, violations are obtained up to d=11. Without entanglement concentration, we observe violations only up to d=9 (please see Supplementary Fig. S2 in Section SIV). Above d∼11, the strength of the signal becomes so low that noise begins to overshadow the quantum correlations. In Fig. 2, the theoretical prediction in equation (5) for a state with maximal 11-dimensional entanglement is fitted to the experimental coincidence data obtained using the mode analyser settings defined in equation (4) for d=11, with only the vertical offset and amplitude left as free parameters. The observed fringes are seen to closely match those theoretically obtained for a state with maximal 11-dimensional entanglement.
The violation of a Bell inequality in d×d dimensions directly indicates that the measured state was entangled. It remains to determine how many dimensions were involved in the entanglement. Measuring the coincidence probabilities, that is, of there being the joint state  (Fig. 4), together with the parameters Sd for different d, can be seen as a partial tomography of the SPDC source state. Numerical investigations indicate that a state with the experimentally observed coincidence probabilities and parameters S2,S3,…,S11 must contain genuine 11-dimensional entanglement. In other words, it is not possible to obtain the observed levels of violation with a state that contains entanglement involving only 10 dimensions or less. Our analysis assumes a special form of the states, based on the coincidence measurement results shown in Fig. 4. Further details are given in Supplementary Section SII.
(Fig. 4), together with the parameters Sd for different d, can be seen as a partial tomography of the SPDC source state. Numerical investigations indicate that a state with the experimentally observed coincidence probabilities and parameters S2,S3,…,S11 must contain genuine 11-dimensional entanglement. In other words, it is not possible to obtain the observed levels of violation with a state that contains entanglement involving only 10 dimensions or less. Our analysis assumes a special form of the states, based on the coincidence measurement results shown in Fig. 4. Further details are given in Supplementary Section SII.
 with ℓs,ℓi=−5,…,+5.
with ℓs,ℓi=−5,…,+5.Our results hold much promise for applications requiring entangled qudits in general. As mentioned earlier, progress in the development of quantum repeaters (see ref. 16 and references therein) would make quantum key distribution using high-dimensional entangled states13 a possible application. Conventional quantum communication will fail for sufficiently large transmission distances because of loss, and quantum repeaters are one possible solution to this problem. Although experimental quantum key distribution has been demonstrated with OAM qutrits24, our findings provide experimental evidence that such protocols could be implemented using photons entangled in OAM in up to 11 dimensions, resulting in a considerable increase in information coding density.
A possible extension to our work would be to investigate the generation of multiphoton, high-dimensional OAM entanglement. We can conceive of achieving this using a cascade of down-conversion crystals for generating multipartite entangled photons, which has been done for polarization entangled photons17. It also seems to be within reach to combine the high-dimensional photon OAM entanglement with entanglement in the polarization and path degrees of freedom, creating even larger hyper-entangled states (see ref. 17 and references within).
On a more fundamental note, Bell test experiments carried out so far have one or both of two main loopholes, namely the locality and detection loopholes. However, a recent theoretical work reveals that even low-dimensional qudits can provide a significant advantage over qubits for closing the detection loophole5. In fact, it was found that as much as 38.2% loss can be tolerated using four-dimensional entanglement. Our results raise interesting possibilities regarding the role higher-dimensional entangled qudits could play in closing this loophole. We emphasize that neither the detection nor the locality loophole has been closed in our experiments, because the overall efficiency of our experimental set-up is 1–2%, and the switching time for our measurement devices (SLMs) is of the order of tens of milliseconds. However, closing these loopholes was not the immediate goal of our experiments. We are instead using the violation of Bell inequalities, up to fair sampling assumptions, as a means of verifying the presence of high-dimensional entanglement, within the framework of quantum mechanics.
In summary, we have been able to experimentally demonstrate violations of Bell-type inequalities generalized to d-dimensional systems6 with up to d=12, enough to indicate genuine 11-dimensional entanglement in the OAM of signal and idler photons in parametric down-conversion. It seems that this could be extended to even higher dimensions by using a brighter source of entangled photons.
Methods
In our experiments, we use computer-controlled SLMs (Hamamatsu) operating in reflection mode with a resolution of 600×600 pixels. In the detection, the SLMs are prepared in the states defined in equation (4) respectively. An SLM prepared in a given state transforms a photon in that state to the Gaussian |ℓ=0〉 mode. The reflected photon is then coupled into a single-mode fibre which feeds a single photon detector. As only the |ℓ=0〉 mode couples into the fibre, a count in the detector indicates a detection of the state in which the SLM was prepared. The hologram generation algorithm introduced in ref. 27 is applied to configure the SLMs.
Figure 1 shows a schematic diagram of the experimental set-up as well as examples of SLM settings used where d=1. For the SPDC, we use a pump beam, with ℓ=0, produced by a frequency-tripled, mode-locked Nd-YAG laser with an average output power of 150 mW at 355 nm. The collimated laser beam is normally incident on a 3-mm-long β-barium borate (BBO) crystal cut for type-I collinear phase matching. A 50:50 beam splitter (BS) then separates the co-propagating OAM entangled photons probabilistically into the signal and idler paths. Spectral filters with 10 nm bandwidth are used to reduce the detection of noise photons. The coincidence resolving time is 10 ns and an integration time of 20 s is used for the measurements.
For tests within a d-dimensional subspace and for odd d, we choose the modes ℓ=−(d−1)/2,…,0,…,(d−1)/2 as the computational basis states |j〉 in equations (2) and (3), where j=0,…,d−1. For even d, we use ℓ=−d/2,…,−1,1,…,d/2, omitting the ℓ=0 mode. A projection of the SPDC output state onto a d-dimensional subspace results in a non-maximally entangled state owing to the limited spiral bandwidth28. To enhance the entanglement, we use the so-called Procrustean method of entanglement concentration26. This is generally done by means of a filtering technique that equalizes the mode amplitudes, thereby probabilistically enhancing the entanglement of the two-photon state29. This can be achieved by applying local operations to one or both of the signal and idler photons. We choose local operations matched to the spiral bandwidth measurement for our SPDC source (please see Supplementary Section SIV), so as to obtain a close approximation to a maximally entangled state. The method applied in ref. 30 uses lenses for equalizing amplitudes in a superposition of three OAM modes. We however use alterations of the diffraction efficiencies of blazed phase gratings in the SLMs to achieve this goal for up to 14 modes. Figure 4 contrasts the results of coincidence measurements with and without Procrustean filtering, with the SLMs in the state  where ℓA,ℓB∈{−5,…,+5}. The disadvantage of the Procrustean method is the associated reduction in the number of detected photons (see Supplementary Section SIII for further details).
where ℓA,ℓB∈{−5,…,+5}. The disadvantage of the Procrustean method is the associated reduction in the number of detected photons (see Supplementary Section SIII for further details).
References
Einstein, A., Podolsky, B. & Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47, 777–780 (1935).
Schrödinger, E. Die gegenwärtige Situation in der Quantenmechanik. Naturwissenschaften 23 807–812; 823–828; 844–849 (1935).
Nielsen, M. A. & Chuang, I. L. Quantum Computation and Quantum Information (Cambridge Univ. Press, 2000).
Durt, T., Kaszlikowski, D., Chen, J-L. & Kwek, L. C. Security of quantum key distributions with entangled qudits. Phys. Rev. A 69, 032313 (2004).
Vértesi, T., Pironio, S. & Brunner, N. Closing the detection loophole in Bell experiments using qudits. Phys. Rev. Lett. 104, 060401 (2010).
Collins, D., Gisin, N-l., Linden, N., Massar, S. & Popescu, S. Bell inequalities for arbitrarily high dimensional systems. Phys. Rev. Lett. 88, 040404 (2002).
Allen, L., Beijersbergen, M. W., Spreeuw, R. J. C. & Woerdman, J. P. Orbital angular momentum of light and the transformation of Laguerre–Gaussian laser modes. Phys. Rev. A 45, 8185–8189 (1992).
Kwiat, P. G. et al. New high-intensity source of polarization-entangled photon pairs. Phys. Rev. Lett. 75, 4337–4341 (1995).
Walborn, S. P., de Oliveira, A. N., Thebaldi, R. S. & Monken, C. H. Entanglement and conservation of orbital angular momentum in spontaneous parametric down-conversion. Phys. Rev. A 69, 023811 (2004).
Bennett, C. H. et al. Teleporting an unknown quantum state via dual classical and Einstein–Podolsky–Rosen channels. Phys. Rev. Lett. 70, 1895–1899 (1993).
You-Bang, Z., Qun-Yong, Z., Yu-Wu, W. & Peng-Cheng, M. Schemes for teleportation of an unknown single-qubit quantum state by using an arbitrary high-dimensional entangled state. Chinese Phys. Lett. 27, 10307–10310 (2010).
Bennett, C. H. & Wiesner, S. J. Communication via one- and two-particle operators on Einstein–Podolsky–Rosen states. Phys. Rev. Lett. 69, 2881–2884 (1992).
Ekert, A. K. Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 67, 661–663 (1991).
Hillery, M., Bužek, V. & Berthiaume, A. Quantum secret sharing. Phys. Rev. A 59, 1829–1834 (1999).
Raussendorf, R., Browne, D. E. & Briegel, H. J. Measurement-based quantum computation on cluster states. Phys. Rev. A 68, 022312 (2003).
Yuan, Z-S. et al. Experimental demonstration of a BDCZ quantum repeater node. Nature 454, 1098–1101 (2008).
Gao, W-B. et al. Experimental demonstration of a hyper-entangled ten-qubit Schrödinger cat state. Nature Phys. 6, 331–335 (2010).
Vaziri, A., Weihs, G. & Zeilinger, A. Experimental two-photon, three-dimensional entanglement for quantum communication. Phys. Rev. Lett. 89, 240401 (2002).
Aspect, A., Grangier, P. & Roger, G. Experimental realization of Einstein–Podolsky–Rosen–Bohm gedankenexperiment: A new violation of Bell’s inequalities. Phys. Rev. Lett. 49, 91–94 (1982).
Leach, J. et al. Violation of a Bell inequality in two-dimensional orbital angular momentum state-spaces. Opt. Exp. 17, 8287–8293 (2009).
Jack, B. et al. Entanglement of arbitrary superpositions of modes within two-dimensional orbital angular momentum state spaces. Phys. Rev. A 81, 043844 (2010).
Jack, B. et al. Precise quantum tomography of photon pairs with entangled orbital angular momentum. New J. Phys. 11, 103024 (2009).
Pors, J. B. et al. Shannon dimensionality of quantum channels and its application to photon entanglement. Phys. Rev. Lett. 101, 120502 (2008).
Gröblacher, S., Jennewein, T., Vaziri, A., Weihs, G. & Zeilinger, A. Experimental quantum cryptography with qutrits. New J. Phys. 8, 75 (2006).
Durt, T., Kaszlikowski, D. & Żukowski, M. Violations of local realism with quantum systems described by N-dimensional Hilbert spaces up to N=16. Phys. Rev. A 64, 024101 (2001).
Bennett, C. H., Bernstein, H. J., Popescu, S. & Schumacher, B. Concentrating partial entanglement by local operations. Phys. Rev. A 53, 2046–2052 (1996).
Leach, J., Dennis, M. R., Courtial, J. & Padgett, M. J. Vortex knots in light. New J. Phys. 7, 55 (2005).
Torres, J. P., Alexandrescu, A. & Torner, L. Quantum spiral bandwidth of entangled two-photon states. Phys. Rev. A 68, 050301 (2003).
Law, C. K. & Eberly, J. H. Analysis and interpretation of high transverse entanglement in optical parametric down conversion. Phys. Rev. Lett. 92, 127903 (2004).
Vaziri, A., Pan, J-W., Jennewein, T., Weihs, G. & Zeilinger, A. Concentration of higher dimensional entanglement: Qutrits of photon orbital angular momentum. Phys. Rev. Lett. 91, 227902 (2003).
Acknowledgements
We acknowledge suggestions from S. M. Barnett. This work was funded by the Engineering and Physical Sciences Research Council (EPSRC). A.C.D. acknowledges funding support from the Scottish Universities Physics Alliance (SUPA).
Author information
Authors and Affiliations
Contributions
A.C.D. and E.A. devised the concept of the experiment. E.A. supervised the theoretical aspects of the project. G.S.B. and M.J.P. advised on aspects of experimental design. A.C.D. and J.L. carried out the experiment. A.C.D. and E.A. wrote the paper with contributions from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 773 kb)
Rights and permissions
About this article
Cite this article
Dada, A., Leach, J., Buller, G. et al. Experimental high-dimensional two-photon entanglement and violations of generalized Bell inequalities. Nature Phys 7, 677–680 (2011). https://doi.org/10.1038/nphys1996
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys1996
This article is cited by
-
Integrated optical vortex microcomb
Nature Photonics (2024)
-
Preparation of maximally-entangled states with multiple cat-state qutrits in circuit QED
Frontiers of Physics (2024)
-
Remote transport of high-dimensional orbital angular momentum states and ghost images via spatial-mode-engineered frequency conversion
Nature Communications (2023)
-
Interferometric imaging of amplitude and phase of spatial biphoton states
Nature Photonics (2023)
-
On-chip parallel processing of quantum frequency comb
npj Quantum Information (2023)