Abstract
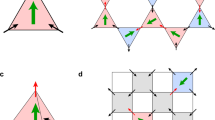
Magnetic monopoles have been predicted to occur as emergent fractional quasiparticles inside pyrochlore spin ice, a frustrated magnetic insulator. Experimental signatures of such emergent monopoles accompanied by Dirac strings have been detected by means of neutron scattering in reciprocal space in pyrochlore spin ice at sub-Kelvin temperatures, but their real-space observation has remained elusive. Here we report on direct, real-space observations of emergent monopoles and their associated Dirac strings in two-dimensional (2D) artificial kagome spin ice at room temperature using synchrotron X-ray photoemission electron microscopy. Magnetization reversal proceeds through the nucleation and avalanche-type dissociation of monopole–antimonopole pairs along 1D Dirac strings. This is in sharp contrast to conventional domain growth in 2D systems, providing a striking example of dimensional reduction due to frustration. The observed hysteresis, monopole densities and 1D Dirac-string avalanches are quantitatively explained by Monte Carlo simulations.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Dirac, P. A. M. Quantised singularities in the electromagnetic field. Proc. R. Soc. Lond. A 133, 60–72 (1931).
’t Hooft, G. Magnetic monopoles in unified gauge theories. Nucl. Phys. B 79, 276–284 (1974).
Polyakov, A. M. Particle spectrum in quantum field theory. JETP Lett. 20, 194–195 (1974).
Goldhaber, A. S. & Trower, W. P. Magnetic monopoles. Am. J. Phys. 58, 429–439 (1990).
Abbiendi, G. et al. Search for Dirac magnetic monopoles in e+e− collisions with the OPAL detector at LEP2. Phys. Lett. B 663, 37–42 (2008).
Castelnovo, C., Moessner, R. & Sondhi, S. L. Magnetic monopoles in spin ice. Nature 451, 42–45 (2008).
Harris, M. J., Bramwell, S. T., McMorrow, D. F., Zeiske, T. & Godfrey, K. W. Geometrical frustration in the ferromagnetic pyrochlore Ho2Ti2O7 . Phys. Rev. Lett. 79, 2554–2557 (1997).
Bramwell, S. T. & Gingras, M. J. P. Spin ice state in frustrated magnetic pyrochlore materials. Science 294, 1495–1501 (2001).
Ramirez, A. P., Hayashi, A., Cava, R. J., Siddharthan, R. & Shastry, B. S. Zero-point entropy in ‘spin ice’. Nature 399, 333–335 (1999).
Pauling, L. The structure and entropy of ice and of other crystals with some randomness of atomic arrangement. J. Am. Chem. Soc. 57, 2680–2684 (1935).
Tchernyshyov, O. Magnetism: Freedom for the poles. Nature 451, 22–23 (2008).
Balents, L. Spin liquids in frustrated magnets. Nature 464, 199–208 (2010).
Heeger, A. J., Kivelson, S., Schrieffer, J. R. & Su, W. P. Solitons in conducting polymers. Rev. Mod. Phys. 60, 781–850 (1988).
Faddeev, L. D. & Takhtajan, L. A. What is the spin of a spin-wave? Phys. Lett. A 85, 375–377 (1981).
Lake, B., Tennant, D. A., Frost, C. D. & Nagler, S. E. Quantum criticality and universal scaling of a quantum antiferromagnet. Nature Mat. 4, 329–334 (2005).
Braun, H. B. et al. Emergence of soliton chirality in a quantum antiferromagnet. Nature Phys. 1, 159–163 (2005).
dePicciotto, R. et al. Direct observation of a fractional charge. Nature 389, 162–164 (1997).
Dolev, M., Heiblum, M., Umansky, V., Stern, A. & Mahalu, D. Observation of a quarter of an electron charge at the ν=5/2 quantum Hall state. Nature 452, 829–835 (2008).
Jaubert, L. D. C. & Holdsworth, P. C. W. Signature of magnetic monopole and Dirac string dynamics in spin ice. Nature Phys. 5, 258–261 (2009).
Morris, D. J. P. et al. Dirac strings and magnetic monopoles in spin ice Dy2Ti2O7 . Science 326, 411–414 (2009).
Fennell, T. et al. Magnetic Coulomb phase in the spin ice Ho2Ti2O7 . Science 326, 415–417 (2009).
Bianchi, A. D. et al. Superconducting vortices in CeCoIn5: Toward the Pauli-limiting field. Science 319, 177–180 (2008).
Laver, M. & Forgan, E. M. Magnetic flux lines in type-II superconductors and the hairy ball theorem. Nature Comm. 1, 1–4 (2010).
Nelson, D. R. Defects and Geometry in Condensed Matter Physics 303 (Cambridge Univ. Press, 2002).
Kadowaki, H. et al. Observation of magnetic monopoles in spin ice. J. Phys. Soc. Jpn 78, 103706 (2009).
Ladak, S., Read, D. E., Perkins, G. K., Cohen, L. F. & Branford, W. R. Direct observation of magnetic monopole defects in an artificial spin-ice system. Nature Phys. 6, 359–363 (2010).
Wang, R. F. et al. Artificial ‘spin ice’ in a geometrically frustrated lattice of nanoscale ferromagnetic islands. Nature 439, 303–306 (2006).
Mengotti, E. et al. Building blocks of an artificial kagome spin ice: Photoemission electron microscopy of arrays of ferromagnetic islands. Phys. Rev. B 78, 144402 (2008).
Remhof, A. et al. Magnetostatic interactions on a square lattice. Phys. Rev. B 77, 134409 (2008).
Möller, G. & Moessner, R. Artificial square ice and related dipolar nanoarrays. Phys. Rev. Lett. 96, 237202 (2006).
Wills, A. S., Ballou, R. & Lacroix, C. Model of localized highly frustrated ferromagnetism: The kagome spin ice. Phys. Rev. B 66, 144407 (2002).
Tabata, Y. et al. Kagome ice state in the dipolar spin ice Dy2Ti2O7 . Phys. Rev. Lett. 97, 257205 (2006).
Fennell, T., Bramwell, S. T., McMorrow, D. F., Manuel, P. & Wildes, A. R. Pinch points and Kasteleyn transitions in kagome ice. Nature Phys. 3, 566–571 (2007).
Möller, G. & Moessner, R. Magnetic multipole analysis of kagome and artificial spin-ice dipolar arrays. Phys. Rev. B 80, 140409 (2009).
Chern, G. W., Mellado, P. & Tchernyshyov, O. Two-stage ordering of spins in dipolar spin ice on kagome. Preprint at http://arxiv.org/abs/0906.4781 (2009).
Im, M. Y., Fischer, P., Kim, D. H. & Shin, S. C. Direct observation of individual Barkhausen avalanches in nucleation-mediated magnetization reversal processes. Appl. Phys. Lett. 95, 182504 (2009).
Sethna, J. P. et al. Hysteresis and hierarchies: Dynamics of disorder-driven first-order phase transformations. Phys. Rev. Lett. 70, 3347 (1993).
Sethna, J. P., Dahmen, K. A. & Myers, C. R. Crackling noise. Nature 410, 242–250 (2001).
Sethna, J. P., Dahmen, K. A. & Perkovic, O. Random-field Ising models of hysteresis. Preprint at http://arxiv.org/abs/0406320 (2004).
Chubykalo, O. A., González, J. M. & González, J. Avalanches as propagating domain walls in a micromagnetic model. Physica D 113, 382–386 (1998).
Schumann, A., Sothmann, B., Szary, P. & Zabel, H. Charge ordering of magnetic dipoles in artificial honeycomb patterns. Appl. Phys. Lett. 97, 022509 (2010).
Stöhr, J. et al. Element-specific magnetic microscopy with circularly polarized X-rays. Science 259, 658–661 (1993).
Christensen, K. & Moloney, N. R. Complexity and Criticality (Imperial College Press, 2005).
Acknowledgements
The authors would like to thank: M. Horisberger, E. Deckardt, A. Weber, C. David, A. Steger, L. Le Guyader, A. Kleibert, J. Raabe, D. Eastwood and D. Atkinson for their help. We also acknowledge helpful discussions with V. Lobaskin, W. Nahm and M. Sigrist. This work was supported by the Swiss National Science Foundation and the Science Foundation of Ireland (08/RFP/PHY1532 and 05/IN1/I853), and part of this work was carried out at the Swiss Light Source, Paul Scherrer Institute, Villigen, Switzerland. We also acknowledge receipt of an HEA Ireland equipment fund.
Author information
Authors and Affiliations
Contributions
Sample preparation: E.M.; measurements: E.M., A.F.R.; analysis and interpretation: E.M., L.J.H., H.B.B.; theory and simulations: R.V.H., H.B.B.; preparation of the manuscript: E.M., L.J.H., H.B.B.; supervision of the project: L.J.H., H.B.B., F.N. All authors discussed the results and implications, and commented on the manuscript at all stages.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 1991 kb)
Rights and permissions
About this article
Cite this article
Mengotti, E., Heyderman, L., Rodríguez, A. et al. Real-space observation of emergent magnetic monopoles and associated Dirac strings in artificial kagome spin ice. Nature Phys 7, 68–74 (2011). https://doi.org/10.1038/nphys1794
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys1794
This article is cited by
-
Clocked dynamics in artificial spin ice
Nature Communications (2024)
-
Revealing emergent magnetic charge in an antiferromagnet with diamond quantum magnetometry
Nature Materials (2024)
-
Distinguishing artificial spin ice states using magnetoresistance effect for neuromorphic computing
Nature Communications (2023)
-
Geometrical control of topological charge transfer in Shakti-Cairo colloidal ice
Communications Physics (2023)
-
Electrically programmable magnetic coupling in an Ising network exploiting solid-state ionic gating
Nature Communications (2023)