Abstract
Preparing and manipulating quantum states of mechanical resonators is a highly interdisciplinary undertaking that now receives enormous interest for its far-reaching potential in fundamental and applied science1,2. Up to now, only nanoscale mechanical devices achieved operation close to the quantum regime3,4. We report a new micro-optomechanical resonator that is laser cooled to a level of 30 thermal quanta. This is equivalent to the best nanomechanical devices, however, with a mass more than four orders of magnitude larger (43 ng versus 1 pg) and at more than two orders of magnitude higher environment temperature (5 K versus 30 mK). Despite the large laser-added cooling factor of 4,000 and the cryogenic environment, our cooling performance is not limited by residual absorption effects. These results pave the way for the preparation of 100-μm scale objects in the quantum regime. Possible applications range from quantum-limited optomechanical sensing devices to macroscopic tests of quantum physics5,6.
Similar content being viewed by others
Main
Recently, the combination of high-finesse optical cavities with mechanical resonators has opened up new possibilities for preparing and detecting mechanical systems close to—and even in—the quantum regime by using well-established methods of quantum optics. Most prominently, the mechanism of efficient laser cooling has been demonstrated7,8,9,10,11,12,13 and has been shown to be capable, in principle, of reaching the quantum ground state14,15,16. A particularly intriguing feature of this approach is that it can be applied to mechanical objects of almost arbitrary size, from the nanoscale in microwave strip-line cavities13 up to the centimetre scale in gravitational-wave interferometers11. In addition, whereas quantum-limited readout is still a challenging development step for non-optical schemes3,17,18, optical readout techniques at the quantum limit are readily available19.
Approaching and eventually entering the quantum regime of mechanical resonators through optomechanical interactions essentially requires the following three conditions to be fulfilled: (1) sideband-resolved operation; that is, the cavity amplitude decay rate κ has to be small with respect to the mechanical frequency ωm; (2) both ultralow noise and low absorption of the optical cavity field (phase noise at the mechanical frequency can act as a finite-temperature thermal reservoir and absorption can increase the mode temperature and even diminish the cavity performance in the case of superconducting cavities); and (3) sufficiently small coupling of the mechanical resonator to the thermal environment; that is, low environment temperature T and large mechanical quality factor Q (the thermal coupling rate is given by kBT/ℏQ, where kB is the Boltzmann constant and ℏ is the reduced Planck constant). So far, no experiment has demonstrated all three requirements simultaneously. Criterion (1) has been achieved10,13,20; however, the performance was limited in one case by laser phase noise10 and in the other cases by absorption in the cavity13,20. Other, independent, experiments have implemented only criterion (2)11,12,19,21. Finally, criterion (3) has been realized in several cryogenic experiments4,13,21,22, however not in combination with both (1) and (2).
We have designed a novel micro-optomechanical device that enables us to meet all requirements at the same time. Specifically, we have fabricated a Si3N4 micromechanical resonator that carries a high-reflectivity, ultralow-loss Bragg mirror (Fig. 1a), which serves as the end mirror of a Fabry–Pérot cavity. We designed the system to exhibit a fundamental mechanical mode at relatively high frequency (of the order of 1 MHz; Fig. 1b) such that sideband-resolved operation (criterion (1)) can be achieved already with a medium-finesse cavity. Criterion (2) can first be fulfilled because our solid-state pump laser used for optical cooling exhibits low phase noise (laser linewidth below 1 kHz). Second, absorption in the Bragg mirror is sufficiently low to prevent residual heating in the mechanical structure. Absorption levels as low as 10−6 have been reported for similar Bragg mirrors23 and recent measurements suggest even lower values of 4×10−7 for the specific coatings used in this experiment (R. Lalezari, private communication). In addition, although absorption in Si3N4 is comparable to silicon, the transmission mismatch of the two cavity mirrors (∼10:1) and the resulting low transmission through the Bragg mirror prevents residual heating of the resonator as has been observed for cryogenically cooled silicon cantilevers24. Finally, criterion (3) requires low temperature and high mechanical quality. The mechanical properties of our design are dominated by the Si3N4, which is known to exhibit superior performance in particular at low temperatures, where Q-factors beyond 106 have been observed at millikelvin temperatures25.
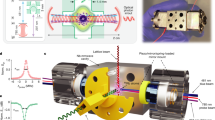
a, Scanning electron micrograph of the basic mechanical system, which is formed by a doubly clamped Si3N4 beam. A circular, high-reflectivity Bragg mirror is used as the end mirror of a Fabry–Pérot cavity. The Bragg mirror is made of low-absorption, alternating dielectric stacks of Ta2O5/SiO2. The magnified section in the inset shows the stacking sequence. b, Micromechanical displacement spectra shown as noise power spectra of the readout-beam phase quadrature for a locked and an unlocked cavity. The fundamental mode at ωm=2π×945 kHz and all higher mechanical modes are identified by finite element simulation. For the cases that involve large Bragg mirror displacements, we provide the simulated mode profile.
We operate our device, a 100 μm×50 μm×1 μm microresonator, in a cryogenic 4He environment at 10−7 mbar and in direct contact with the cryostat cold finger. To measure the mechanical displacement, the frequency of a 7 μW continuous-wave Nd:YAG laser is locked close to resonance of the cryogenic Fabry–Pérot cavity (length L≈25 mm), which consists of a fixed macroscopic mirror and the moving micromechanical mirror. The optical cavity of finesse F≈3,900 achieves moderate sideband resolution (κ≈0.8ωm), which in principle would allow cooling to a final occupation number 〈n〉min=(κ2/4ωm2)≈0.16, that is, well into the quantum ground state14,15. The experimentally achievable temperature is obtained as the equilibrium state of two competing processes, namely the laser cooling rate and the coupling rate to the thermal (cryogenic) environment. In essence, laser cooling is driven (in the ideal resolved-sideband limit and at detuning Δ=ωm) at a rate Γ≈G2/(2κ) (G is the effective optomechanical coupling rate, as defined in ref. 16), whereas mechanical relaxation to the thermal environment at temperature T takes place at a rate (kBT/ℏQ). The final achievable mechanical occupation number is therefore, to first order, given by nf≈(1/Γ)×(kBT/ℏQ). A more accurate derivation taking into account effects of non-ideal sideband resolution can be found, for example, in refs 14, 15, 16, 26. Our experimental parameters limit the minimum achievable mode temperature to approximately 1 mK (nf≈30). The fact that we can observe this value in the experiment (see below) shows that other residual heating effects are negligible. The micromechanical flexural motion modulates the cavity-field phase quadrature, which is measured by optical homodyning. For Q≫1 its noise power spectrum (NPS) is a direct measure of the mechanical position spectrum Sq(ω), as described in ref. 16. We observe a minimum noise floor of 2.6×10−17 m Hz−0.5, which is a factor of 4 above the achievable quantum (shot-noise) limit, when taking into account the finite cavity linewidth, the cavity losses and the non-perfect mode-matching, and due to the residual amplitude noise of the pump laser at the sideband frequency of our mechanical mode. We observe the fundamental mechanical mode at ωm=2π×945 kHz with an effective mass meff=43±2 ng and a quality factor Q≈30,000 at 5.3 K (Q≈5,000 at 300 K). These values are consistent with independent estimates based on finite-element method simulations yielding ωm=2π×945 kHz and meff=53±5 ng (see Supplementary Information).
Optomechanical laser cooling requires driving of the cavity with a red-detuned (that is, off-resonant), optical field6,7,8,9,10,11,12,13. We achieve this by coupling a second laser beam—detuned by Δ in frequency but orthogonal in polarization—into the same spatial cavity mode (Fig. 2a). Birefringence of the cavity material leads to both an optical path length difference for the two cavity modes (resulting in an 800 kHz frequency difference of the cavity peak positions) and a polarization rotation of the outgoing fields. We compensate both effects by an offset in Δ and by extra linear optical phase retarders, respectively. A change in detuning Δ modifies the mechanical rigidity and results in both an optical spring effect (ωeff(Δ)) and damping (γeff(Δ)), which is directly extracted by fitting the NPS using the expressions from ref. 16. Figure 2b shows the predicted behaviour for several powers of the red-detuned beam. The low-power curve at 140 μW is used to determine both the effective mass of the mechanical mode, meff, and the cavity finesse, F. For higher powers and detunings closer to cavity resonance, the onset of cavity instability prevents a stable lock (see, for example, ref. 16). All experimental data are in agreement with theory and hence in accordance with pure radiation-pressure effects15.
a, The laser is split at a polarizing beamsplitter (PBS) into a weak locking field (red) tuned near cavity resonance ωc and the cooling field (blue) tuned off-resonant with an acousto-optical modulator (AOM) to ωc+Δ≈ωc−ωm. An electro-optical modulator (EOM) in the weak field is used to generate a Pound–Drever–Hall error signal for cavity locking. The beams are recombined on a PBS into the same spatial mode at orthogonal polarization before they enter the cavity comprising an input mirror (IM) and the micro-mechanical mirror. The phase quadrature of the locking beam is measured in a homodyne detection scheme (BS: beamsplitter; LO: local oscillator; Φ: local oscillator phase; SA: spectrum analyser). Φ is stabilized in a separate proportional–integral–derivative controller (PID). A combination of a Faraday rotator (FR) and a half-wave plate (λ/2) separates the reflected from the original signal. b, The effective frequency ωeff and damping γeff of the micro-mechanical motion for different detuning and power settings. All power levels follow the theoretical predictions for pure radiation-pressure interaction. The symbols are experimental data, and the solid lines are simulations based on ref. 16. The inset shows the data set taken at 140 μW optical power.
The effective mode temperature is obtained through the equipartition theorem. For our experimental parameter regime, Q≫1 and 〈n〉≫0.5, the integrated NPS is also a direct measure of the mean mechanical mode energy and hence, through the equipartition theorem, of its effective temperature through  . Note that, for the case of strong optomechanical coupling, normal-mode splitting can occur and has to be taken into account when evaluating the mode temperature27. In our present case, this effect is negligible because of the large cavity decay rate κ. The amplitude of the NPS is calibrated by comparing the mechanical NPS with the NPS of a known frequency modulation applied to the laser (see, for example, ref. 28). For a cold-finger temperature of 5.3 K, we obtain a mode temperature T=2.3 K, which is consistent with an expected moderate cooling due to slightly off-resonant locking of the Fabry–Pérot cavity (by less than 3% of the cavity intensity linewidth). The locking point is deliberately chosen to be on the cooling side to avoid unwanted parametric mechanical instabilities. The mean thermal occupancy was calculated according to 〈n〉=kBTeff/ℏωeff. We note, however, that Bose–Einstein statistics will have a dominant role as one approaches the quantum ground state.
. Note that, for the case of strong optomechanical coupling, normal-mode splitting can occur and has to be taken into account when evaluating the mode temperature27. In our present case, this effect is negligible because of the large cavity decay rate κ. The amplitude of the NPS is calibrated by comparing the mechanical NPS with the NPS of a known frequency modulation applied to the laser (see, for example, ref. 28). For a cold-finger temperature of 5.3 K, we obtain a mode temperature T=2.3 K, which is consistent with an expected moderate cooling due to slightly off-resonant locking of the Fabry–Pérot cavity (by less than 3% of the cavity intensity linewidth). The locking point is deliberately chosen to be on the cooling side to avoid unwanted parametric mechanical instabilities. The mean thermal occupancy was calculated according to 〈n〉=kBTeff/ℏωeff. We note, however, that Bose–Einstein statistics will have a dominant role as one approaches the quantum ground state.
Figure 3a shows mechanical noise power spectra with the cooling beam switched off and with maximum cooling beam pump power at 7 mW. For a detuning Δ≈ωm, we demonstrate laser cooling to a mean thermal occupation of 32±4 quanta, which is more than 2 orders of magnitude lower than previously reported values for optomechanical devices10 and is comparable to the lowest reported temperature of 25 quanta for nano-electromechanical systems4 (NEMS). In contrast to previous experiments10,13, the achieved cooling performance is not limited by optical absorption or residual phase noise, but follows exactly the theoretically predicted behaviour (Fig. 3b). This agrees with the expected device performance: a fraction of approximately 10−6 of the intra-cavity power is absorbed by the Bragg mirror (∼13 μW at maximum cooling) and a maximum of 1% of the transmitted power is absorbed by the Si3N4 beam29 (∼14 μW at maximum cooling and taking into account the impedance mismatch of the cavity mirrors). The cryogenic cooling power of the cryostat used is orders of magnitude larger than the maximum heat load expected on the micromechanical structures. The absence of absorption can also be seen from the inferred mode temperature Teff, which decreases with the mechanical damping rate γeff in strict accordance with the power law Teff∝γeff−1. This relation follows immediately from the simple expression for the mechanical occupation nf given above (nf∝Γ−1) and from the fact that the laser cooling rate Γ is to first approximation equivalent to the effective mechanical damping γeff, at least for all data points of our experiment. Both heating and the onset of normal-mode splitting for strong coupling27 would result in a deviation of this behaviour.
a, Calibrated noise power spectra for the fundamental mechanical mode at 5.3 K environmental temperature with small cavity cooling (top) and at maximum cooling (bottom). The thermal energy is reduced from ≈53,000 quanta at 7 μW laser power to 32±4 quanta at 7 mW. The vertical axes in both plots are logarithmic. The change in the technical noise floor is due to different locking levels of the local oscillator phase Φ in the homodyne detection. b, Plot of the calibrated effective temperature Teff versus the observed damping γeff for various power and detuning values of the cooling beam. No deviations from the theoretically expected power-law dependence (red solid line) can be observed. The inset shows the mean thermal occupation 〈n〉 as a function of detuning for maximal laser power. Cavity instability prevents detunings arbitrarily close to resonance. The red solid curve is a simulation based on ref. 16 that uses only experimentally obtained parameters.
The remaining obstacle that prohibits us from reaching the quantum ground state is the intrinsic phonon coupling to the thermal environment at rate kBT/ℏQ≈1.4×107 Hz. By reducing the reservoir temperature to that of NEMS experiments (20 mK), this coupling will significantly reduce, not only owing to the lower bath temperature but also because Si3N4 resonators markedly improve in mechanical Q with decreasing temperature. For example, thermal heating rates as low as 3×103 Hz have been observed for Si3N4 at 300 mK (ref. 25), which would place our effective mode temperature already well into the quantum ground state using otherwise unchanged parameters.
In summary, we have demonstrated optical cooling of the fundamental mode of a 100 μm scale mechanical resonator in a cryogenic cavity to a thermal occupation of only 32±4 quanta. This is comparable to the performance of state-of-the-art NEMS devices. In contrast to previous approaches, the large laser cooling rates attained are no longer limited by residual absorption or phase-noise effects. This is achieved by a new micro-optomechanical resonator design with exceptionally low intrinsic optical absorption and both high optical and mechanical quality. This leaves the reduction of the thermal coupling, for example, by further decreasing the environment temperature to those available in conventional 3He cryostats, as the only remaining hurdle to prepare the mechanical quantum ground state. Our approach hence establishes a feasible route towards the quantum regime of massive micromechanical systems.
Methods
Micro-mirror fabrication.
Our micro-mechanical oscillator is made of 1-μm-thick low-stress Si3N4 deposited on a Si substrate and coated through ion beam sputtering with a high-reflectivity Bragg mirror. Standard photolithography and plasma etching is used for forming, in subsequent steps, the mirror pad and the micro-mechanical resonator, which is finally released from the Si substrate in a XeF2 atmosphere. The mirror stack, designed and deposited by ATFilms, comprises 36 alternating layers of Ta2O5 and SiO2 with an overall nominal reflectivity of 99.991% at 1,064 nm. The measured finesse of 3,900 is consistent with an input coupler reflectivity of 99.91% and with extra diffraction losses due to a finite size of the cavity beam waist.
References
Schwab, K. C. & Roukes, M. L. Putting mechanics into quantum mechanics. Phys. Today 58, 36–42 (2005).
Aspelmeyer, M. & Schwab, K. C. (eds) Mechanical systems at the quantum limit. New J. Phys. 10, 095001 (2008).
LaHaye, M., Buu, O., Camarota, B. & Schwab, K. C. Approaching the quantum limit of a nanomechanical resonator. Science 304, 74–77 (2004).
Naik, A. et al. Cooling a nanomechanical resonator with quantum backaction. Nature 443, 193–196 (2006).
Marshall, W., Simon, C., Penrose, R. & Bouwmeester, D. Towards quantum superpositions of a mirror. Phys. Rev. Lett. 91, 130401 (2003).
Vitali, D. et al. Optomechanical entanglement between a movable mirror and a cavity field. Phys. Rev. Lett. 98, 030405 (2007).
Metzger, C. H. & Karrai, K. Cavity cooling of a microlever. Nature 432, 1002–1005 (2004).
Gigan, S. et al. Self-cooling of a micromirror by radiation pressure. Nature 444, 67–71 (2006).
Arcizet, O., Cohadon, P.-F., Briant, T., Pinard, M. & Heidmann, A. Radiation-pressure cooling and micromechanical instability of a micromirror. Nature 444, 71–75 (2006).
Schliesser, A., Rivière, R., Anetsberger, G., Arcizet, O. & Kippenberg, T. J. Resolved-sideband cooling of a micromechanical oscillator. Nature Phys. 4, 415–419 (2007).
Corbitt, T. et al. An all-optical trap for a gram-scale mirror. Phys. Rev. Lett. 98, 150802 (2007).
Thompson, J. D. et al. Strong dispersive coupling of a high-finesse cavity to a micromechanical membrane. Nature 452, 72–75 (2008).
Teufel, J. D., Harlow, J. W., Regal, C. A. & Lehnert, K. W. Dynamical backaction of microwave fields on a nanomechanical oscillator. Phys. Rev. Lett. 101, 197203 (2008).
Wilson-Rae, I., Nooshi, N., Zwerger, W. & Kippenberg, T. J. Theory of ground state cooling of a mechanical oscillator using dynamical backaction. Phys. Rev. Lett. 99, 093901 (2007).
Marquardt, F., Chen, J. P., Clerk, A. A. & Girvin, S. M. Quantum theory of cavity-assisted sideband cooling of mechanical motion. Phys. Rev. Lett. 99, 093902 (2007).
Genes, C., Vitali, D., Tombesi, P., Gigan, S. & Aspelmeyer, M. Ground-state cooling of a micromechanical oscillator: Comparing cold damping and cavity-assisted cooling schemes. Phys. Rev. A 77, 033804 (2008).
Regal, C. A., Teufel, J. D. & Lehnert, K. W. Measuring nanomechanical motion with a microwave cavity interferometer. Nature Phys. 4, 555–560 (2008).
Poggio, M. et al. An off-board quantum point contact as a sensitive detector of cantilever motion. Nature Phys. 4, 635–638 (2008).
Arcizet, O. et al. High-sensitivity optical monitoring of a micromechanical resonator with a quantum-limited optomechanical sensor. Phys. Rev. Lett. 97, 133601 (2006).
Cole, G. D., Gröblacher, S., Gugler, K., Gigan, S. & Aspelmeyer, M. Monocrystalline AlGaAs heterostructures for high-reflectivity high-Q micromechanical resonators in the MHz regime. Appl. Phys. Lett. 92, 261108 (2008).
Gröblacher, S., Gigan, S., Böhm, H. R., Zeilinger, A. & Aspelmeyer, M. Radiation-pressure self-cooling of a micromirror in a cryogenic environment. Eur. Phys. Lett. 81, 54003 (2008).
Poggio, M., Degen, C. L., Mamin, H. J. & Rugar, D. Feedback cooling of a cantilever’s fundamental mode below 5 mK. Phys. Rev. Lett. 99, 017201 (2007).
Rempe, G., Thompson, R. J., Kimble, H. J. & Lalezari, R. Measurement of ultralow losses in an optical interferometer. Opt. Lett. 17, 363–365 (1996).
Bleszynski-Jayich, A. C., Shanks, W. E. & Harris, J. G. E. Noise thermometry and electron thermometry of a sample-on-cantilever system below 1 K. Appl. Phys. Lett. 92, 013123 (2008).
Zwickl, B. M. et al. High quality mechanical and optical properties of commercial silicon nitride membranes. Appl. Phys. Lett. 92, 103125 (2008).
Wilson Rae, I., Nooshi, N., Dobrindt, J., Kippenberg, T. J. & Zwerger, W. Cavity-assisted backaction cooling of mechanical resonators. New J. Phys. 10, 095007 (2008).
Dobrindt, J. M., Wilson-Rae, I. & Kippenberg, T. J. Optomechanical normal-mode splitting. Phys. Rev. Lett. 101, 263602 (2008).
Pinard, M., Hadjar, Y. & Heidmann, A. Effective mass in quantum effects of radiation pressure. Eur. Phys. J. D 7, 107–116 (1997).
Edward, D., Palik, E. D. & Ghosh, G. (eds) Handbook of Optical Constants of Solids (Academic, 1998).
Acknowledgements
We thank R. Lalezari (ATFilms) and M. Metzler, R. Ilic and M. Skvarla (CNF) and F. Blaser, T. Corbitt and W. Lang for discussion and support. We acknowledge support by the Austrian Science Fund FWF (Projects P19539, L426, START), by the European Commission (Projects MINOS, IQOS) and by the Foundational Questions Institute fqxi.org (Grants RFP2-08-03, RFP2-08-27). Part of this work was carried out at the Cornell NanoScale Facility, a member of the National Nanotechnology Infrastructure Network, which is supported by the National Science Foundation (Grant ECS-0335765). S.Gr. is a recipient of a DOC-fellowship of the Austrian Academy of Sciences and G.D.C. of a Marie Curie Fellowship of the European Commission. S.Gr. and M.R.V. are members of the FWF doctoral program Complex Quantum Systems (W1210).
Author information
Authors and Affiliations
Contributions
All authors have made a significant contribution to the concept, design, execution or interpretation of the presented work.
Corresponding author
Supplementary information
Supplementary Information
Supplementary Information (PDF 317 kb)
Rights and permissions
About this article
Cite this article
Gröblacher, S., Hertzberg, J., Vanner, M. et al. Demonstration of an ultracold micro-optomechanical oscillator in a cryogenic cavity. Nature Phys 5, 485–488 (2009). https://doi.org/10.1038/nphys1301
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys1301
This article is cited by
-
Study of optical bistability/multistability and transparency in cavity-assisted-hybrid optomechanical system embedded with quantum dot molecules
Optical and Quantum Electronics (2024)
-
Colossal Nernst power factor in topological semimetal NbSb2
Nature Communications (2022)
-
A lensed fiber Bragg grating-based membrane-in-the-middle optomechanical cavity
Scientific Reports (2022)
-
Optomechanical ratchet resonators
Science China Physics, Mechanics & Astronomy (2022)
-
Coherent control of quantum and entanglement dynamics via periodic modulations in optomechanical semiconductor resonator coupled to quantum-dot excitons
Quantum Information Processing (2021)