Abstract
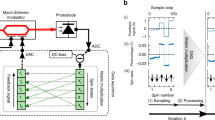
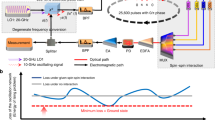
Solving combinatorial optimization problems is becoming increasingly important in modern society, where the analysis and optimization of unprecedentedly complex systems are required. Many such problems can be mapped onto the ground-state-search problem of the Ising Hamiltonian, and simulating the Ising spins with physical systems is now emerging as a promising approach for tackling such problems. Here, we report a large-scale network of artificial spins based on degenerate optical parametric oscillators (DOPOs), paving the way towards a photonic Ising machine capable of solving difficult combinatorial optimization problems. We generate >10,000 time-division-multiplexed DOPOs using dual-pump four-wave mixing in a highly nonlinear fibre placed in a cavity. Using those DOPOs, a one-dimensional Ising model is simulated by introducing nearest-neighbour optical coupling. We observe the formation of spin domains and find that the domain size diverges near the DOPO threshold, which suggests that the DOPO network can simulate the behaviour of low-temperature Ising spins.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Papadimitriou, C. H. & Steiglits, K. Combinatorial Optimization: Algorithms and Complexity (Dover Publications, 1998).
Barahona, F. On the computational complexity of Ising spin glass models. J. Phys. A 15, 3241–3253 (1982).
Johnson, M. W. et al. Quantum annealing with manufactured spins. Nature 473, 194–198 (2011).
Kim, K. et al. Quantum simulation of frustrated Ising spins with trapped ions. Nature 465, 590–593 (2010).
Yamaoka, M. et al. 20k-spin Ising chip for combinatorial optimization problem with CMOS annealing. Proc. Inter. Solid-State Circ. Conf. 24.3 (2015).
Mahboob, I. & Yamaguchi, H. An electromechanical Ising machine. Preprint at http://arxiv.org/abs/1505.02467 (2015).
Utsunomiya, S., Takata, K. & Yamamoto, Y. Mapping of Ising models onto injection-locked laser systems. Opt. Express 19, 18091–18108 (2011).
Takata, K., Utsunomiya, S. & Yamamoto, Y. Transient time of an Ising machine based on injection-locked laser network. New J. Phys. 14, 013052 (2012).
Wang, Z., Marandi, A., Wen, K., Byer, R. L. & Yamamoto, Y. Coherent Ising machine based on degenerated optical parametric oscillators. Phys. Rev. A 88, 063853 (2013).
Haribara, Y., Yamamoto, Y., Kawarabayashi, K. I. & Utsunomiya, S. A coherent Ising machine for MAX-CUT problems: performance evaluation against semidefinite programming relaxation and simulated annealing. Preprint at http://arxiv.org/abs/1501.07030 (2015).
Marandi, A., Wang, Z., Takata, K., Byer, R. L. & Yamamoto, Y. Network of time-multiplexed optical parametric oscillators as a coherent Ising machine. Nature Photon. 8, 937–942 (2014).
Nabors, C. D., Yang, S. T., Day, T. & Byer, R. L. Coherence properties of a doubly-resonant monolithic optical parametric oscillator. J. Opt. Soc. Am. B 7, 815–820 (1990).
Marandi, A., Leindecker, N. C., Pervak, V., Byer, R. L. & Vodopyanov, K. L. Coherence properties of a broadband femtosecond mid-IR optical parametric oscillator operating at degeneracy. Opt. Express 20, 7255–7262 (2012).
Serkland, D. K., Bartolini, G. D., Agarwal, A., Kumar, P. & Kath, W. L. Pulsed degenerate optical parametric oscillator based on a nonlinear-fiber Sagnac interferometer. Opt. Lett. 23, 795–797 (1998).
Okawachi, Y. et al. Dual-pumped degenerate Kerr oscillator in a silicon nitride microresonator. Opt. Lett. 40, 5267–5270 (2015).
McKinstrie, C. & Radic, S. Phase-sensitive amplification in fibre. Opt. Express 12, 4973–4979 (2004).
Fan, J. & Migdall, A. Phase-sensitive four-wave mixing and Raman suppression in a microstructure fibre with dual laser pumps. Opt. Lett. 31, 2771–2773 (2006).
Marhic, M. E., Hsia, C. H. & Jeong, J. M. Optical amplification in a nonlinear fibre interferometer. Electron. Lett. 27, 210–211 (1991).
Levenson, J. A., Abram, I., Rivera, Th. & Grangier, Ph. Reduction of quantum noise in optical parametric amplification. J. Opt. Soc. Am. B 10, 2233–2238 (1993).
Choi, S.-K., Vasilyev, M. & Kumar, P. Noiseless optical amplification of images. Phys. Rev. Lett. 83, 1938–1941 (1999).
Imajuku, W., Takada, A. & Yamabayashi, Y. Low-noise amplifcation under the 3 dB noise figure in high-gain phase-sensitive fibre amplifier. Electron. Lett. 35, 1954–1955 (1999).
Tong, Z. et al. Towards ultrasensitive optical links enabled by low-noise phase-sensitive amplifiers. Nature Photon. 5, 430–436 (2011).
Ising, E. Beitrag zur theorie des ferromagnetismus. Zeitschrift fur Physik A 31, 253–258 (1925).
Brown, W. F. Jr Micromagnetics (Wiley, 1963).
McCoy, B. M. & Wu, T. T. The Two-Dimensional Ising Model 2nd edn (Dover, 2014).
Agrawal, G. P. Nonlinear Fibre Optics (Academic, 1989).
Yamamoto, Y. & Inoue, K. Noise in amplifiers. J. Lightw. Technol. 21, 2895–2915 (2003).
Drummond, P. D. & Corney, J. F. Quantum noise in optical fibers. I. Stochastic equations. J. Opt. Soc. Am. B 18, 139–152 (2001).
Acknowledgements
The authors thank S. Utsunomiya, A. Marandi, P. McMahon, K. Igarashi, S. Tamate, K. Takata, Y. Haribara, K. Shimizu, I. Mahboob and W. J. Munro for discussions, and H. Tamura for various types of support. This research was funded by the Impulsing Paradigm Change through Disruptive Technologies (ImPACT) Program of the Council of Science, Technology and Innovation (Cabinet Office, Government of Japan).
Author information
Authors and Affiliations
Contributions
T.I. and H.T. constructed the DOPO set-up and performed the experiments. R.H. and K.Inaba developed the theoretical model. T.I., K.Inaba, R.H. and H.T. analysed the data. H.T., K.Inoue and Y.Y. conceived the concept for the experiment. All authors discussed the results and wrote the paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 295 kb)
Rights and permissions
About this article
Cite this article
Inagaki, T., Inaba, K., Hamerly, R. et al. Large-scale Ising spin network based on degenerate optical parametric oscillators. Nature Photon 10, 415–419 (2016). https://doi.org/10.1038/nphoton.2016.68
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2016.68
This article is cited by
-
Nonlinear topological symmetry protection in a dissipative system
Nature Communications (2024)
-
Parametrically driven pure-Kerr temporal solitons in a chip-integrated microcavity
Nature Photonics (2024)
-
Recent progress on coherent computation based on quantum squeezing
AAPPS Bulletin (2023)
-
Optical experimental solution for the multiway number partitioning problem and its application to computing power scheduling
Science China Physics, Mechanics & Astronomy (2023)
-
Very-large-scale integrated quantum graph photonics
Nature Photonics (2023)