Abstract
Two genetic findings from twin research have far-reaching implications for understanding individual differences in the development of brain function as indexed by general cognitive ability (g, aka intelligence): (1) The same genes affect g throughout development, even though (2) heritability increases. It is now possible to test these hypotheses using DNA alone. From 1.7 million DNA markers and g scores at ages 7 and 12 on 2875 children, the DNA genetic correlation from age 7 to 12 was 0.73, highly similar to the genetic correlation of 0.75 estimated from 6702 pairs of twins from the same sample. DNA-estimated heritabilities increased from 0.26 at age 7 to 0.45 at age 12; twin-estimated heritabilities also increased from 0.35 to 0.48. These DNA results confirm the results of twin studies indicating strong genetic stability but increasing heritability for g, despite mean changes in brain structure and function from childhood to adolescence.
Similar content being viewed by others
Introduction
Although developmental research from childhood to adolescence reveals species-general changes in brain structure and function,1, 2 much less is known about the development of individual differences within our species, which has been called ‘one of the preeminent challenges of neuroimaging’.3 It is important to understand the developmental etiology of individual differences, because societal problems often involve individual differences—for example, why some children are slow to speak, to learn or to read. The description and causes of species’ means are not necessarily related to the description and causes of variances within a species.4 Two well-replicated genetic findings from twin studies comparing monozygotic and dizygotic (DZ) twins suggest hypotheses at the level of individual differences in cognitive ability that may be relevant to neuroscience, to the extent that brain structure and function underlie cognitive outcomes. These twin-study findings involve general cognitive ability, which was labeled g by Spearman more than a century ago,5 but is commonly known as intelligence.6 g is the most researched cognitive trait in genetics7 and has important links with neuroscience.8, 9
First, the heritability of g increases during development, even from childhood to adolescence.10 This finding is counterintuitive to the extent that genetic effects are thought to be static, and environmental effects are expected to accumulate during development. The increasing heritability of g also seems at odds with the second genetic finding: The same genes largely affect g throughout development.11 For example, in a longitudinal twin analysis from childhood to adolescence, the genetic correlation was estimated as 0.96, although the 95% confidence interval for this estimate was 0.74–1.0.12 The genetic correlation is literally the correlation between genetic effects on g at the two ages independent of heritability.11 The high genetic correlation implies that if a gene is found to be associated with g in childhood, the gene is also highly likely to be associated with g in adolescence. Later, we offer a hypothesis as to how heritability can increase when genetic effects are stable from age to age.
These two genetic findings have not found much traction in the neurodevelopmental literature. This neglect might be due in part to a lack of attention to individual differences, but it might also be due to skepticism about the twin method, which relies on some major assumptions, most notably, equal environmental treatment of monozygotic and DZ twins.11 Quantitative genetic designs such as the twin method would no longer be needed if it were possible to identify all of the genes responsible for heritability.13 However, it has proven more difficult than expected to identify genes for complex traits,14 including g,15 which has led to the refrain of ‘missing heritability’.16, 17 Nonetheless, it is now possible to use DNA itself to estimate genetic variance and covariance in any sample of unrelated individuals, not just samples consisting of special family members such as twins or adoptees. The method, called genome-wide complex trait analysis (GCTA)18 correlates genomic similarity across hundreds of thousands of single nucleotide polymorphisms (SNPs) with phenotypic similarity in a large sample of unrelated individuals.19 This population-based DNA approach does not rely on the strong assumptions made in classical twin studies. GCTA compares similarity across hundreds of thousands of SNPs with phenotypic similarity pair by pair in a large sample of unrelated individuals. Although conventionally unrelated individuals only vary in their genetic similarity by a small amount, GCTA accumulates all the genotype−phenotype association signals using the massive information available in a matrix of thousands of individuals, each compared pair by pair with every other individual in the sample. GCTA has been used to estimate genetic influence for height,19 weight,20 psychiatric and medical disorders,21, 22, 23 personality24 and even economic and political preferences.25 GCTA has also been applied to g in adults26 and children.27 These GCTA estimates of genetic influence, although substantial, have been lower than heritability typically found in twin studies of these traits. Using the 12-year data from the sample in the present report, GCTA and twin estimates of heritability were compared explicitly for several cognitive measures; the GCTA estimate of g was 35% and the twin estimate was 46%.28 Precision in comparing GCTA and twin estimates is important because, as explained later, this comparison reveals important information about a trait’s genetic architecture.
This previous GCTA research involves univariate analysis in that it decomposes the phenotypic variance of a single trait into genetic and non-genetic components of variance. Recently, GCTA has been extended to bivariate analysis, which decomposes the phenotypic covariance between traits into components of covariance. The first preliminary attempt to extend GCTA to bivariate analysis reported a genetic correlation of 0.62 for g in childhood (age 11) and old age.27 Here, we use a new bivariate GCTA method18, 29 to test the hypotheses of strong stability and increasing heritability for g from age 7 to 12. We also compare GCTA estimates with those from a twin analysis based on the same sample at the same ages using the same measures.
Materials and methods
Sample
The sample was drawn from the Twins Early Development Study (TEDS), which is a multivariate longitudinal twin-study that recruited over 11 000 twin pairs born in England and Wales in 1994, 1995 and 1996.30, 31 TEDS is representative of the UK population.32 The project received approval from the Institute of Psychiatry ethics committee (05/Q0706/228), and parental consent was obtained before data collection. Individuals were included if their first language was English and they had no major medical or psychiatric problems. GCTA was conducted on g at ages 7 and 12 for 2875 unrelated individuals in TEDS (only one member of a twin pair), of which 1334 had g data at both ages. Twin model-fitting analyses of g at ages 7 and 12 were conducted for 6702 TEDS twin pairs, of which 2269 pairs had g data at both ages. As expected for representative twin studies, the twins included similar numbers of monozygotic twins, same-sex DZ twins and opposite-sex DZ twins.
Genotyping
Although DNA is available for more than 12 000 TEDS participants, funds were available to genotype 3665 individuals (one member only per twin pair) on Affymetrix GeneChip 6.0 (Affymetrix Inc., Santa Clara, CA, USA) SNP genotyping arrays using standard experimental protocols as part of the WTCCC2 project. In addition to nearly 700 000 genotyped SNPs, more than one million other SNPs were imputed using IMPUTE v.2 software (https://mathgen.stats.ox.ac.uk/impute/impute.html).33 DNA for 3152 individuals (1446 males and 1706 females) survived quality control criteria. Of these 3152 individuals, 2875 had g scores at least at one age and 1344 had g scores at both ages. To control for ancestral stratification, we performed principal component analyses on a subset of 100 000 quality-controlled SNPs after removing SNPs in linkage disequilibrium (r2>0.2).34 Using the Tracy−Widom test,35 we identified 8 axes with P<0.05, which were used as covariates in GCTA analyses.
Measures
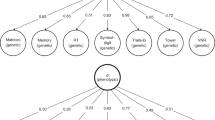
The measures and testing procedures have been described in detail for age 736 and 12.37 At each age, a composite index of g was derived from two verbal tests and two non-verbal tests. At age 7, the two verbal tests consisted of the Similarities subtest and the Vocabulary subtest from the WISC-III-UK, and the two non-verbal tests were the picture completion subtest from the WISC-III-UK and the Conceptual Grouping subtest from the McCarthy Scales of Children’s Abilities. At age 12, the verbal tests included the Information and Vocabulary subtests from the WISC-III-PI Multiple Choice test, and the two non-verbal reasoning tests were WISC-III-UK Picture Completion and Raven’s Standard and Advanced Progressive Matrices. At age 7, testing was conducted by telephone as described elsewhere;36 at age 12, testing was conducted online.37 For each cognitive measure at each age, scores were regressed on sex and age and standardized residuals were derived, ranked with random values given to tied data, and quantile normalized.38, 39 Finally, total composites for g were created as unit-weighted means requiring complete data for at least three of the four tests. All the procedures were executed using R (www.r-project.org).40
Statistical analyses
Genome-wide complex trait analysis
The first step in GCTA is to calculate pairwise genomic similarity between all pairs of individuals in the sample using all genetic markers genotyped on the SNP array. Because GCTA is designed to estimate genetic variance due to linkage disequilibrium between unknown causal variants and genotyped SNPs from a sample of unrelated individuals in the population, any close genetic relatedness is eliminated; for this reason any individual whose genetic similarity is equal to or greater than a fourth cousin is removed (estimate of pairwise relatedness >0.025). The essence of GCTA is to compare a matrix of pairwise genomic similarity to a matrix of pairwise phenotypic similarity using a random-effects mixed linear model.18 In univariate analysis, the variance of a trait can be partitioned using residual maximum likelihood into genetic and residual components. Detailed description of this method can be found in Yang, Lee et al.18 and Yang, Benyamin et al.19 The bivariate method extends the univariate model by relating the pairwise genetic similarity matrix to a phenotypic covariance matrix between traits 1 and 2, allowing for correlated residuals.29 The eight principal components described earlier were used as covariates in our GCTA analyses; as mentioned, all phenotypes were age- and sex-regressed before analysis.
Twin modeling
The classical twin design and model-fitting is discussed elsewhere.11 We fit a bivariate twin model using OpenMx,41 which provided a direct comparison with the bivariate GCTA. The correlated factor solution is the least restricted model allowing variables to correlate with one another via genetic, shared environment and non-shared environment. Because previous analyses of these data indicated nonsignificant differences in model-fitting results between males and females,32, 42 we combined same-sex and opposite DZ twin pairs in order to increase the power of the analyses. Twin analyses limited to same-sex twins yielded highly similar results (available from the first author).
Results and Discussion
Genetic stability
As shown in Table 1, the GCTA genetic correlation between g at ages 7 and 12 was 0.73 (0.29 standard error, s.e.). Table 2 shows that the twin-study yielded a highly similar genetic correlation of 0.75 (0.08 s.e.). The genetic correlation indexes the correlation between genetic effects on g at the two ages independent of heritability. That is, the genetic correlation can be high even if heritability is low. It is also possible to weight the genetic correlation by heritability in order to estimate the genetic contribution to the phenotypic correlation. The phenotypic correlation for g between ages 7 and 12 was 0.46 (0.02) for 2408 children (one member randomly chosen from each twin pair) with g data at both ages. For GCTA, the genetic contribution to the phenotypic correlation was 0.25 (0.11), which is the GCTA genetic correlation weighted by heritability (that is, the product of the square roots of the GCTA heritabilities of g at the two ages). Another way of expressing this is as bivariate heritability, which is the proportion of the phenotypic correlation that can be attributed to genetic covariance. GCTA bivariate heritability was 0.60 (that is, 0.25÷0.42), indicating that 60% of the phenotypic correlation could be accounted for by genetic factors. The comparable twin-study estimate of the genetic contribution to the phenotypic correlation was 0.31 (0.03), yielding a bivariate heritability of 0.68.
Increasing heritability
Despite the substantial genetic correlation of 0.73 from age 7–12, GCTA estimates of genetic influence on g increased from 0.26 (0.17 s.e.) at age 7 to 0.45 (0.14 s.e.) at age 12, although the large standard errors indicate that the increase did not reach statistical significance. Heritability increased significantly in the twin model-fitting analyses, from 0.36 (0.03) at age 7 to 0.49 (0.03) at age 12. Thus, GCTA estimates account for 74% of the twin-study heritability estimate of g at age 7 and 94% at age 12.
Why genetic stability but increasing heritability?
In summary, GCTA confirms the twin-study hypotheses of strong genetic stability and increasing heritability. In other words, the same genes are largely (about 75%) responsible for genetic influence on g at age 7 and age 12, yet the effect of these genes (heritability) increases substantially from age 7 to 12. How is this possible? We hypothesize that the same genes affect g from age to age but heritability increases as children select their own environments that are correlated with their g-related genetic propensities,10 a process called genotype−environment correlation.11 This hypothesis makes three predictions. The first prediction is that g-related experiences will themselves show genetic influence, for which there is considerable evidence from twin studies.43, 44 Second, the links between these experiences and g are expected to be mediated genetically, evidence which is beginning to emerge from twin studies.45 The third prediction is that genetic links between g and experience should strengthen during development, but this has not yet been investigated. These genetic links are expected especially for experiences in which children are able to select or modify their environments in line with their genetic propensities, in contrast to environments that are passively imposed on children. Supportive evidence to date for this genotype−environment hypothesis relies on twin data, but GCTA can also be used to address these issues with DNA alone.
Genetic architecture
Our GCTA results clarify the genetic architecture of g in ways that are relevant to solving the ‘missing heritability’ puzzle that has emerged from the limited success of genome-wide association studies to identify the genes responsible for heritability.46 Two of the major hypotheses to account for missing heritability are epistatic (nonadditive) genetic effects and rare variants, because genome-wide association research is limited to detecting additive genetic effects and genetic effects that can be tagged by the common SNPs used to date on commercially available DNA arrays.19 Because GCTA is also limited in these same two ways, finding significant GCTA estimates of genetic influence provides strong evidence that current genome-wide association research strategies can detect the majority of the missing heritability if samples are sufficiently large to provide power to detect associations of small effect size. As noted above, our GCTA estimates of genetic influence account for 74–94% of our twin-study heritability estimates, which implies that most of the missing heritability can be found with additive effects of common SNPs. The heritability that remains missing might be due to epistatic effects and rare variants.
In our longitudinal genetic analyses from age 7 to 12, the GCTA estimate of genetic covariance is also somewhat lower than the twin-study estimate. As shown in Table 1, the genetic covariance for g between ages 7 and 12—that is, the genetic contribution to the phenotypic covariance—is 20% lower for GCTA than for twins (that is, 0.25 for GCTA and 0.31 for twins). However, the GCTA genetic correlation of 0.73 is highly similar to the twin-study genetic correlation of 0.76. The likely reason is that GCTA genetic variance and covariance estimates are attenuated by imperfect linkage disequilibrium between causal variants and genotyped SNPs, but the GCTA estimate of the genetic correlation is unbiased, because the genetic correlation is derived from the ratio between genetic covariance and genetic variance. Because GCTA genetic variance and covariance estimates are biased to the same extent due to imperfect linkage disequilibrium, they cancel each other out in the calculation of the genetic correlation, leaving an unbiased estimate of the genetic correlation.
Implications for brain structure and function
To the extent that g indexes general brain function, the present results suggest hypotheses for the etiology of individual differences in brain development. The same genes can be expected to be responsible for individual differences throughout brain development despite the major mean changes that occur during development. The hypothesis of increasing heritability for individual differences in brain development points to genotype−environment correlation as the process by which genotypes become phenotypes. Importantly, the correspondence between GCTA and twin results indicates that special samples such as twins are no longer needed to test such genetic hypotheses in neurodevelopment—GCTA makes it possible to test them in any large sample of unrelated individuals.
References
Blakemore SJ . Imaging brain development: the adolescent brain. Neuroimage 2012; 61: 397–406.
Power JD, Fair DA, Schlaggar BL, Petersen SE . The development of human functional brain networks. Neuron 2010; 67: 735–748.
Giedd JN, Rapoport JL . Structural MRI of pediatric brain development: what have we learned and where are we going? Neuron 2010; 67: 728–734.
Plomin R, Kosslyn S . Genes brain and cognition. Nat Neurosci 2001; 4: 1153–1155.
Spearman C . ‘General intelligence’, objectively determined and measured. Am J Psychol 1904; 15: 201–292.
Deary IJ . Intelligence. Ann Rev Psychol 2012; 63: 453–482.
Deary IJ, Johnson W, Houlihan LM . Genetic foundations of human intelligence. Hum Genet 2009; 126: 215–232.
Deary IJ, Penke L, Johnson W . The neuroscience of human intelligence differences. Nat Rev Neurosci 2010; 11: 201–211.
Karama S, Colom R, Johnson W, Deary IJ, Haier R, Waber DP et al. Cortical thickness correlates of specific cognitive performance accounted for by the general factor of intelligence in healthy children aged 6–18. Neuroimage 2011; 55: 1443–1453.
Haworth CMA, Wright MJ, Luciano M, Martin NG, de Geus EJC, van Beijsterveldt CEM et al. The heritability of general cognitive ability increases linearly from childhood to young adulthood. Mol Psychiatry 2010; 15: 1112–1120.
Plomin R, DeFries JC, Knopik VS, Neiderhiser JM . Behavioral Genetics 6th edn Worth: New York, 2013.
van Soelen ILC, Brouwer RM, van Leeuwen M, Kahn RS, Pol HEH, Boomsma DI . Heritability of verbal and performance intelligence in a pediatric longitudinal sample. Twin Res Hum Genet 2011; 14: 119–128.
Haworth CMA, Plomin R . Quantitative genetics in the era of molecular genetics: learning abilities and disabilities as an example. J Am Acad Child Adolesc Psychiatry 2010; 49: 783–793.
McCarthy MI, Abecasis GR, Cardon LR, Goldstein DB, Little J, Ioannidis JPA et al. Genome-wide association studies for complex traits: consensus, uncertainty and challenges. Nat Rev Genet 2008; 9: 356–369.
Chabris CF, Hebert BM, Benjamin DJ, Beauchamp JP, Cesarini D, van der Loos M et al. Most reported genetic associations with general intelligence are probably false positives. Psychol Sci 2012; 23: 1314–1323.
Maher B . Personal genomes: the case of the missing heritability. Nature 2008; 456: 18–21.
Plomin R . Child development and molecular genetics: 14 years later. Child Dev 2012; 30: 1467–8624.
Yang JA, Lee SH, Goddard ME, Visscher PM . GCTA: a tool for genome-wide complex trait analysis. Am J Hum Genet 2011; 88: 76–82.
Yang J, Benyamin B, McEvoy BP, Gordon S, Henders AK, Nyholt DR et al. Common SNPs explain a large proportion of the heritability for human height. Nat Genet 2010; 42: 565–569.
Yang J, Manolio TA, Pasquale LR, Boerwinkle E, Caporaso N, Cunningham JM et al. Genome partitioning of genetic variation for complex traits using common SNPs. Nat Genet 2011; 43: 519–525.
Lee SH, Wray NR, Goddard ME, Visscher PM . Estimating missing heritability for disease from genome-wide association studies. Am J Hum Genet 2011; 88: 294–305.
Lee SH, DeCandia TR, Ripke S, Yang J, Sullivan PF, Goddard ME et al. Estimating the proportion of variation in susceptibility to schizophrenia captured by common SNPs. Nat Genet 2012; 44: 247–250.
Lubke GH, Hottenga JJ, Walters R, Laurin C, de Geus EJ, Willemsen G et al. Estimating the genetic variance of major depressive disorder due to all single nucleotide polymorphisms. Biol Psychiatry 2012; 72: 707–709.
Vinkhuyzen AAE, Pedersen NL, Yang J, Lee SH, Magnusson PKE, Iacono WG et al. Common SNPs explain some of the variation in the personality dimensions of neuroticism and extraversion. Transl Psychiatry 2012; 2: e102.
Benjamin DJ, Cesarini D, van der Loos MJHM, Dawes CT, Koellinger PD, Magnusson PKE et al. The genetic architecture of economic and political preferences. Proc Natl Acad Sci USA 2012; 109: 8026–8031.
Davies G, Tenesa A, Payton A, Yang J, Harris SE, Liewald D et al. Genome-wide association studies establish that human intelligence is highly heritable and polygenic. Mol Psychiatry 2011; 16: 996–1005.
Deary IJ, Yang J, Davies G, Harris SE, Tenesa A, Liewald D et al. Genetic contributions to stability and change in intelligence from childhood to old age. Nature 2012; 482: 212–215.
Plomin R, Haworth CMA, Meaburn EL, Price T . Wellcome Trust Case Control Consortium 2, Davis OSP. Common DNA markers can account for more than half of the genetic influence on cognitive abilities. Psychol Sci 2001; 178: 41–48.
Lee SH, Yang J, Goddard ME, Visscher PM, Wray NR . Estimation of pleiotropy between complex diseases using SNP-derived genomic relationships and restricted maximum likelihood. Bioinformatics 2012; 28: 2540–2542.
Haworth CMA, Davis OSP, Plomin R . Twins Early Development Study (TEDS): a genetically sensitive investigation of cognitive and behavioural development from childhood to young adulthood. Twin Res Hum Genet 2012; FirstView: 1–9.
Oliver BR, Plomin R . Twins Early Development Study (TEDS): a multivariate, longitudinal genetic investigation of language, cognition and behaviour problems from childhood through adolescence. Twin Res Hum Genet 2007; 10: 96–105.
Kovas Y, Haworth CMA, Dale PS, Plomin R . The genetic and environmental origins of learning abilities and disabilities in the early school years. Monogr Soc Res Child Dev 2007; 72: 1–144.
Howie BN, Donnelly P, Marchini J . A flexible and accurate genotype imputation method for the next generation of genome-wide association studies. PLoS Genet 2009; 5: e1000529.
Fellay J, Shianna KV, Ge D, Colombo S, Ledergerber B, Weale M et al. A whole-genome association study of major determinants for host control of HIV-1. Science 2007; 317: 944–947.
Patterson N, Price AL, Reich D . Population structure and eigenanalysis. PLoS Genet 2006; 2: 2074–2093.
Petrill SA, Rempell J, Oliver B, Plomin R . Testing cognitive abilities by telephone in a sample of 6-to 8-year olds. Intelligence 2002; 30: 353–360.
Haworth CMA, Harlaar N, Kovas Y, Davis OS, Oliver BR, Hayiou-Thomas ME et al. Internet cognitive testing of large samples needed in genetic research. Twin Res Hum Genet 2007; 10: 554–563.
van der Waerden BL . On the sources of my book "Moderne Algebra". Hist Math 1975; 2: 31–40.
Lehmann EL . Nonparametrics: Statistical Methods Based on Ranks. Holden-Day: San Francisco, 1975.
Team RDC. R: A language and environment for statistical computing (Version 2.13.1). 2011 Available from http://www.R-project.org/.
Boker S, Neale M, Maes H, Wilde M, Spiegel M, Brick T et al. OpenMx: an open source extended structural equation modeling framework. Psychometrika 2011; 76: 306–317.
Davis O, Arden R, Plomin R . g in middle childhood: moderate genetic and shared environmental influence using diverse measures of general cognitive ability at 7, 9 and 10 years in a large population sample of twins. Intelligence 2008; 36: 68–80.
Hanscombe KB, Haworth CMA, Davis OSP, Jaffee SR, Plomin R . The nature (and nurture) of children’s perceptions of family chaos. Learn Individ Differ 2010; 20: 549–553.
Kendler KS, Baker JH . Genetic influences on measures of the environment: a systematic review. Psychol Med 2007; 37: 615–626.
Hanscombe KB, Haworth CMA, Davis OSP, Jaffee SR, Plomin R . Chaotic homes and school achievement: a twin study. J Child Psychol Psychiatry 2011; 52: 1212–1220.
Visscher PM, Brown MA, McCarthy MI, Yang J . Five years of GWAS discovery. Am J Hum Genet 2012; 90: 7–24.
Acknowledgements
TEDS is supported by a program grant to RP from the UK Medical Research Council (G0901245, and previously G0500079), with additional support from the US National Institutes of Health (HD044454; HD046167). Genome-wide genotyping was made possible by a grant from the Wellcome Trust to the Wellcome Trust Case Control Consortium 2 project (085475/B/08/Z; 085475/Z/08/Z). RP is supported by a Medical Research Council Research Professorship award (G19/2) and a European Advanced Investigator award (295366); MT is supported by a Medical Research Council studentship.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare no conflict of interest.
Rights and permissions
This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License. To view a copy of this license, visit http://creativecommons.org/licenses/by-nc-nd/3.0/
About this article
Cite this article
Trzaskowski, M., Yang, J., Visscher, P. et al. DNA evidence for strong genetic stability and increasing heritability of intelligence from age 7 to 12. Mol Psychiatry 19, 380–384 (2014). https://doi.org/10.1038/mp.2012.191
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/mp.2012.191
Keywords
This article is cited by
-
The P-factor and its genomic and neural equivalents: an integrated perspective
Molecular Psychiatry (2022)
-
A cognitive neurogenetic approach to uncovering the structure of executive functions
Nature Communications (2022)
-
Canonical transformation for multivariate mixed model association analyses
Theoretical and Applied Genetics (2022)
-
Genetic influence on cognitive development between childhood and adulthood
Molecular Psychiatry (2021)
-
A statistical approach to fine-mapping for the identification of potential causal variants related to human intelligence
Journal of Human Genetics (2019)