Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Protective role of the glans penis during coitus

International Journal of Impotence Research volume 15, pages 337–342 (2003)Cite this article

Abstract

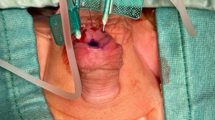
To examine the hypothesis that the glans penis acts protectively, absorbing forces, during coitus. Five potent patients (mean age 46.8±9.7 y), who had indication for surgical excision of the glans for penile carcinoma were included in the present study. Intraoperatively, intracavernosal pressure (ICP) was adjusted by saline infusion and maintained by a pressure feedback infusion pump to a pressure value of 70 mmHg. Using a dynamometer, an external compressive force of 0.5 kg was applied at the glans penis and the changes in ICP were monitored. Measurements were repeated after surgical excision of the glans. Significant ICP changes were noticed in all patients after excision of the glans. Mean preoperative ICP was 161±11.5 mmHg, while after glansectomy it reached 206.6±13 mmHg. ΔICP was 45.8±10.57 mmHg. Two of the patients' partners reported pain during intercourse postoperatively, possibly due to the impact of the force applied by the rigid corpora cavernosa on the anterior vaginal wall without any absorption by the glans. The glans penis restricts the increase in ICP during sexual intercourse, playing a protective role for both the corpora cavernosa and the female genitalia.

This is a preview of subscription content, access via your institution

Access options

Rent or buy this article

Prices vary by article type

from$1.95

to$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Figure 1

References

  1. Lue TF, Tanagho EA . Physiology of erection and pharmacological management of impotence. J Urol 1987; 137: 829–836.

    Article  CAS  Google Scholar 

  2. Lue TF . Physiology of penile erection and pathophysiology of erectile dysfunction and priapism. In: Walsh PC, Retik AB, Vaughan ED, Wein AJ (eds). Campell's Urology. Saunders: Philadelphia, 1998, pp 1157–1179.

    Google Scholar 

  3. Lue TF, Tanagho EA . Hemodynamics of erection. In: Tanagho EA, Lue TF, McClure RD (eds). Contemporary Management of Impotence and Infertility. Williams & Wilkins: Baltimore, 1988, pp 28–38.

    Google Scholar 

  4. Hatzichristou DG et al. Glansectomy: an alternative surgical treatment for Buschke–Lowenstein tumors of the penis. Urology 2001; 57: 966–969.

    Article  CAS  Google Scholar 

  5. Pescatori ES, Hatzichristou DG, Namburi S, Goldstein I . A positive intracavernous injection test implies normal veno-occlusive but not necessarily normal arterial function: a hemodynamic study. J Urol 1994; 151: 1209–1216.

    Article  CAS  Google Scholar 

  6. Goldstein AM, Padma-Nathan H . The microarchitecture of the intracavernosal smooth muscle and the cavernosal fibrous skeleton. J Urol 1990; 144: 1144–1146.

    Article  CAS  Google Scholar 

  7. Hatzichristou DG et al. In vivo assessment of trabecular smooth muscle tone, its application in pharmaco-cavernosometry and analysis of intracavernous pressure determinants. J Urol 1995; 153: 1126–1135.

    Article  CAS  Google Scholar 

  8. Udelson D et al. Engineering analysis of penile hemodynamic and structural–dynamic relationships: Part I—clinical implications of penile tissue mechanical properties. Int J Impot Res 1998; 10: 15–24.

    Article  CAS  Google Scholar 

  9. Nitahara KS, Lue TF . Microscopic anatomy of the penis. In: Carson C, Kirby R, Goldstein I (eds). Textbook of Erectile Dysfunction. Isis Medical Media: Oxford, 1999, pp 31–41.

    Google Scholar 

  10. Udelson D et al. Engineering analysis of penile hemodynamic and structural–dynamic relationships: Part III—clinical considerations of penile hemodynamic and rigidity erectile responses. Int J Impot Res 1998; 10: 89–99.

    Article  CAS  Google Scholar 

  11. Berman JR, Goldstein I . Female sexual dysfunction. Urol Clin North Am 2001; 28: 405–416.

    Article  CAS  Google Scholar 

  12. Levin RJ . Sex and the human female reproductive tract—what really happens during and after coitus. Int J Impot Res 1998; 10 (Suppl 1): S14–S21.

    PubMed  Google Scholar 

  13. Karacan I, Moore C, Sahmay S . Measurement of pressure necessary for vaginal penetration. Sleep Res 1985; 14: 269A.

    Google Scholar 

  14. Hardy SB, Whitten PL . Patterning of sexual activity. In: Smus BB, Cheney DL, Seyfarth RM, Wrangham RW, Struhsaker TT (eds). Primate Societies. Chicago University Press: Chicago, 1987, pp 370–384.

    Google Scholar 

  15. Penson DF et al. The hemodynamic pathophysiology of impotence following blunt trauma to the erect penis. J Urol 1992; 148: 1171–1180.

    Article  CAS  Google Scholar 

  16. Bitsch M, Kromann-Andersen B, Schou J, Sjontoft E . The elasticity and the tensile strength of tunica albuginea of the corpora cavernosa. J Urol 1990; 143: 642–645.

    Article  CAS  Google Scholar 

  17. Hoch Z . Vaginal erotic sensitivity by sexological examination. Acta Obstet Gynecol Scand 1986; 65: 767–773.

    Article  CAS  Google Scholar 

  18. Fox CA . Some aspects and implications of coital physiology. J Sex Marital Ther 1976; 2: 205–213.

    Article  CAS  Google Scholar 

  19. Kermanidis T . Strength of Materials, Vol. II. Singular Publications: Patras, 1995.

    Google Scholar 

  20. Shigley JE, Mischke CR . Mechanical Engineering Design. McGraw-Hill: New York, 1989.

    Google Scholar 

  21. Abramowitz M, Stegun IA . Handbook of Mathematical Functions with Formulas, Graphs and Mathematical Tables. Dover Publications: New York, 1996.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Urology and Center for Sexual and Reproductive Health, Aristotle University of Thessaloniki, Greece

    D G Hatzichristou, V Tzortzis, K Hatzimouratidis, A Apostolidis & K Moysidis

  2. Department of Mechanical Engineering and Aeronautics, University of Patras, Greece

    S Panteliou

Authors
  1. D G Hatzichristou
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. V Tzortzis
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. K Hatzimouratidis
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. A Apostolidis
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. K Moysidis
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. S Panteliou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to D G Hatzichristou.

Appendix

Appendix

The mechanical behaviour of the examined structure in this paper (penis with and without the glans) can be initially modelled as shown in Figure 2.

Figure 2
figure 2

Models of the mechanical behaviour of the examined structure in this paper (penis with and without the glans).

Full size image

Both cases (I, II) can be treated as columns subjected to an axial compressive static load, which in the specific application had a value of 0.5 kp.

The observation was made such that the pressures needed to render the two structures (I, II) capable to withstand the load P without buckling are: σI=206±13 mmHg and σII=161±11.5 mmHg, respectively.

This means that stresses σcrI=−σI and σcrII=−σII are developed in each section of the structures, respectively.

Considering buckling of a column, having Young's modulus E, length L, moment of inertia I and section A, and loaded with a compressive axial load, the critical load of components I, II can be expressed according to the following equations:19,20

where V(x) is the deflection function.

Since

Hence,

From equations (1) and (3) we get

Splitting the integral on the left side of the inequality leads to

Considering that A2=A1, I2=I1, E2=E1, inequality (5) can be reduced to

The function V(x) is of the type: V(x)=C, sin (πx/L), where C=constant.

Then

According to Abramowitz-Stegun21 (relationship 4.3.139, p. 78), for α=0, n=2, b=π/L1, and z=x:

From equations (6) and (7) we can write

Similarly, we can write

Applying relationship (4.3.138) from Abramowitz and Stegun:21

Hence,

From equations (8) and (9) we get

For a circular section

From equations (10) and (11)

Inequality (12) is valid because d1, d3>0, d1<d3 → d12<d32 and E1<E3, given that the construction with glans is the initial penis construction, reinforced with the glans, thus leading to a higher value of Young's modulus.

Rights and permissions

Reprints and Permissions

About this article

Cite this article

Hatzichristou, D., Tzortzis, V., Hatzimouratidis, K. et al. Protective role of the glans penis during coitus. Int J Impot Res 15, 337–342 (2003). https://doi.org/10.1038/sj.ijir.3901039

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/sj.ijir.3901039

Keywords

Search

Advanced search

Quick links