Introduction

Thermoelectricity is a phenomenon wherein a temperature difference in a material induces energy flow via electrical charge diffusion from hot to cold side thereby providing a simple and environmentally-friendly way of converting heat into electricity in single step without any need of moving mechanical components equipped in many of today’s thermal power generation systems1. Wide applications of this ideal convertor require compounds with high thermoelectric figure of merits2,3,4,5. Recently, there has been growing interest in exploring quantum confinement effects in low-dimensional structures for better thermoelectrics6,7,8,9,10,11. Alike the nanomaterials investigated to date, such as superlattices12, nanotube/nanowires13, and quantum dots14, a single-molecule interconnected to metallic electrodes is a quantum system having discrete electronic states that promise giant thermopower by chemically-engineering the electronic structures through optimizing the molecular architectures15,16,17,18. Much progress has been accomplished in understanding and controlling the single-molecule thermoelectric transport properties, such as length dependence19,20,21, intermolecular interactions22, and gate control23, owing to the advance in experimental techniques to address the electron and heat transport in molecular bridges17. In sharp contrast, the roles of electrode-molecule contacts on the thermoelectric properties have remained almost unexplored24,25 albeit the theoretically predicted impact on the electronic structures26,27,28,29,30, due mostly to the technical difficulty to evaluate the geometrical dependence of thermoelectricity in molecular junctions that requires a reliable method to form and hold a molecular bridge for long-enough time to measure their thermoelectric properties and meanwhile controllably deform the configurations at an atomistic level. In the present study, therefore, we report molecular junction diagnosis by thermoelectric analysis for characterizing the anchor dependence to geometrical sensitivity of single-molecule thermopower.

Results

Conductance and thermovoltage traces

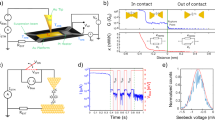
We studied benzene molecules possessing amine and thiol groups at the para positions: 1,4-benzenedithiol (BDT), 1,4-benzenediamine (BDA), and 4-aminobenzenethiol (ABT) (Fig. 1a). Microheater-embedded lithographically-defined mechanically-controllable break junctions (MCBJs)25 were employed to carry out simultaneous measurements of the conductance G and the thermovoltage ΔVj of metal-molecule-metal junctions under stretching at a rate of 0.5 pm/s through a piezo-voltage (Vpiezo) control with a temperature gradient imposed through Joule heat generated at the Pt coil with the applied voltage Vh at room temperature in vacuum (Figs S1 and S2; see also Fig. S9 for calibration measurements to convert Vpiezo into the junction displacement Δd). The breaking process was implemented for 200 times per Vh condition by reconnecting the Au contact after breakdown of molecular junctions. In each trial, a Au contact was broken at first to create a pair of nanoelectrodes, wherein G decreased to below 1 G0 in a discrete manner where G0 = 2e2/h is the conductance quantum with e and h denoting the electron charge and Planck constant, respectively (Fig. 1b). Thereafter, conductance plateaus were often found at 10−2 G0 to 10−3 G0 suggesting formations of molecular bridges31. Meanwhile, the concurrently recorded thermovoltage behaved somewhat synchronously to the change in the conductance (Fig. 1c). Specifically, positive ΔVj was obtained at G > 1 Gα reflecting the negative thermopower SAu = −ΔVj/(ΔT) of Au nanocontacts32, where ΔT is the temperature difference created therein (see Fig. S8 for estimations of ΔT), owing to the sign of the energy derivative of the density of electronic states at the Fermi level33. In contrast, the thermoelectric voltage turns out to be negative at the low conductance regime. The corresponding positive Seebeck coefficient implies charge transport through the highest-occupied molecular orbital (HOMO) levels for the molecules tested34,35.

Figure 1: Thermoelectric measurements of molecular junctions.
figure 1

(a) Schematic model of single-molecule junctions comprised of a X-benzene-Y molecule bonded to two Au electrodes. Thermopower was measured by imposing a temperature differential through electrical heating of a microheater and probing the thermovoltage. X and Y tested were SH and/or NH2. (b) Conductance (G) and thermovoltage (ΔVj) traces recorded simultaneously during tensile breakdown of a Au-1,4-benzenediamine-Au bridge under the heater voltage Vh = 3.5 V displaying positive and negative thermovoltage of Au nanocontacts and the molecular junction, respectively. (c) G versus ΔVj two-dimensional histogram. White and black plots are the average ΔVj in cases with and without molecules being added to the junctions, respectively. (d,e) ΔVj distributions constructed with the trace data showing G in a range from 6 × 10−2 G0 to 0.3 × 10−3 G0 for benzenediamine (BDA) (d) and aminobenzenethiol (ABT) (e). VP1, VP2, and VP3 denote the peak positions as defined by Gaussian fit to the histograms represented by solid curves.

Full size image

Conductance versus thermovoltage characteristics

In order to shed further light on molecular nature in the obtained thermoelectric properties, two-dimensional histograms of G and ΔVj were constructed (Fig. 1c; see also Fig. S3). The plots conspicuously depict the presence of Au nanocontacts as a cluster of data accumulated at above 1 G0 with positive ΔVj. In contrast, the low conductance region below 1 × 10−3 G0 reveals a regime with negligibly small yet finite thermovoltage on average, which is attributable to tunnelling thermopower in a vacuum gap36,37 as corroborated by a control experiment wherein no molecules were added (black plots). On the other hand, there lies another distinct feature at the intermediate range of conductance around 10−2 G0 wherein the negative ΔVj can be ascribed to the aforementioned HOMO-derived carrier transport in the molecular junctions with relatively large scattering presumably reflecting the geometrical sensitivity of the electronic structure28,38.

Statistical variations of junction thermopower

Having confirmed the identity of molecular bridges, statistical variations of the thermoelectric voltage was analysed by building ΔVj histograms with data extracted using a conductance window from 3 × 10−3 G0 to 6 × 10−2 G0 whereat the characteristic negative thermoelectric voltage was detected (we note that the single-molecule conductance falls in this range of conductance39,40,41). Interestingly, we found bimodal and trimodal distributions for the symmetric (BDA and BDT) and asymmetric (ABT) molecules, respectively (Fig. 1d; see also Figs S4 and S5). Gaussian fit extracted peaks positioned at VP1 through VP3. Plots of VP, along with the average thermovoltage of Au nanocontacts deduced from ΔVj at G > 1 G0, with respect to the heater voltage illuminated linear increase in the absolute values of the characteristic thermoelectric voltage with Vh2 (Fig. 2a–c). This is interpreted as due to the fact that the temperature difference at the junction is produced through the power Vh2/Rh (Rh is the resistance of the heater) dissipated locally at the Pt micro-coil that heats up one side of the Au leads via thermal conduction through the Al2O3 layer25.

Figure 2: Molecular junction diagnosis.
figure 2

(a–c) Characteristic VP states plotted as a function of Vh2 and ΔT for BDA (a), BDT (b), and ABT (c). Solid curves are linear fitting to the plots. (d–f) Change in G (d) and ΔVj (e) under mechanical elongation Δd of Au-BDA-Au junctions showing ΔVave higher than −0.5 mV at ΔT = 59 K. The exponential decay in G indicates absence of molecules in the electrode gap in case for the thermopower states of VP1 (f). (g–i) High-thermovoltage traces with ΔVave ≤ −0.5 mV (h) reveal a plateau at close to the single-molecule conductance (g) whereby suggesting electron tunnelling through Au-BDA-Au bridges for VP2. Broken lines in (d) and (g) show a linear fit to the semi-logarithmic plots and the single-molecule conductance state, respectively.

Full size image

Thermovoltage signatures of molecular junctions

It is of importance to elucidate the origin of the multiple thermovoltage states revealed by the ΔVj histograms. For this, we inspected the conductance traces of junctions having average thermovoltage ΔVave =  in the low conductance regimes suggestive of molecular bridge formations, where n is the number of data points in each ΔVj − t curves (Fig. 2d–g). Interestingly, we found exponential decay in the conductance with respect to the distance dgap between the Au electrodes in case of junctions with ΔVave ≥ −0.5 mV at Vh = 3.5 V (ΔT = 59 K), i.e. VP1 state, for BDAs (Fig. 2d–e), which is naturally ascribed to electron tunnelling through vacuum gaps instead of charge transport through molecular bridges (Fig. 2f)42. In sharp contrast, a plateau was observed at around 8 × 10−3 G0 for the plots with ΔVave < −0.5 mV (VP2 state) suggesting formations of Au-BDA-Au links (Fig. 2g–i)40. Similar trends were seen for the other molecules as well (see Fig. S6). These results imply that whereas VP2 is unequivocally attributed to the intrinsic thermoelectric transport characteristics of molecular junctions, VP1 is most likely the tunnelling thermopower of a vacuum gap, whose values are in fact close to the low thermovoltage detected for open contacts in the control experiments whereat no molecules are expected between the electrodes. Rough estimation of the barrier height energy Φ from the slope of the conductance decay by assuming G ∝ exp(−2κdgap) with the coefficient κ =  yields Φ = 6.8 eV with the electron mass me = 9.11 × 10−31 kg (Fig. 2d), which is close to the work function of Au43 thereby corroborating the vacuum tunnelling scenario for VP1.

Stretching-induced thermopower enhancement

The high thermovoltage states of VP3 observed for ABT (Fig. 1e) is a superior property from viewpoints of its thermoelectric applications compared to the VP2 states. We found that this distinct characteristics arises upon excessive elongation of the molecular junctions (Fig. 3a–d; see also Fig. S7). The stretching effects were also present in BDTs25,26 but were not evident in the thermovoltage histograms, and thus not considered here in detail due to the relatively short-lived nature of the high-ΔVj states that emerges on the verge of mechanical breakdown, in comparison to the long lifetime of the Au-thiol linkages. On the other hand, the mechanically-induced thermopower enhancement was confirmed to be completely absent in BDAs indicating important roles of the thiol linkers (Fig. S3d).

Figure 3: Stretching-induced thermopower enhancement.
figure 3

(a,b) G and ΔVj curves demonstrating increased thermovoltage in a highly-elongated ABT junction. The histograms of the traces reveal the conductance and thermovoltage representative of the junction conditions at each stage of the tensile breakdown. The stretching caused P3 states with enhanced thermovoltage. (c,d) Two-dimensional ΔVj histograms of ABT (c) and BDA junctions (d). Note that P3 states are present only in ABT.

Full size image

Discussion

Direct tunnelling contributions

From a naïve point of view, thermoelectric transport in molecular junctions should accompany an effect of direct tunnelling through vacuum44. In order to quantify its possible influence on the measured junction properties, we analysed the conductance versus thermovoltage characteristics in detail. First we noticed that although the positive ΔVj at G > 1 G0 is suggestive of quantum thermopower of Au nanocontacts (Fig. 1c), a care should be taken on the quantitative interpretation as it is well-established that molecules are often bridging aside the Au atom-sized contacts before the opening of electrode nanogaps45,46. This can be seen as multiple batteries, each contributing the single-molecule thermovoltage, with internal resistance Ri connected in parallel when considering no notable quantum interference47,48 in the thermoelectric transport (Fig. 4a). Then, what we measured was actually the combined voltage described as ΔVj = (VAumRmol + VmolRAu)(RmolRAu)/(RmolRs + RAuRs + RmolRAu)(Rmol + RAu), where Rmol = RSMJ/m is the net resistance of m molecules bridging in parallel with the single molecule resistance RSMJ, RAu is the resistance of the Au contacts, and Rs is the serial resistance. This equation tells us that the junction thermovoltage is prone to be determined solely by the source, either molecules or tunnelling gaps, having lower resistance. As RSMJ in the present study is in a range of MΩ39,40,41, Vmol virtually vanishes and ΔVj is dominated by that engendered at the Au contacts whereby yielding the positive thermovoltage of the atomic chains at the conductance above 1 G0. In the meantime, it rapidly decreases to a small negative value as G declines to below 1 G0, which indicates opening of an electrode gap wherein a few molecules can be connected to the electrodes. Whether molecular feature becomes observable at this stage is thus determined by the relative significance of the thermopower contributions of molecular bridges and vacuum gaps47. On the other hand, we find nice fit on the ΔVj − G characteristics with the parallel circuit model by assuming four molecules bridging the electrodes (m = 4) with RSMJ = 2.2 MΩ and VP2 = Vmol = 0.47 mV and variable tunnelling gap size having conductance from 0.1 × 10−3 G0 to 0.2 G0 with Vgap = VP1 = 0.054 mV at Vh = 3.5 V (ΔT = 59 K) for BDA junctions (Fig. 4b), thus elucidating the non-negligible influence of vacuum tunnelling on the molecular junction thermoelectric transport (Fig. 4c). This in turn suggests the possible use of thermopower for molecular junction diagnosis to assess the vacuum gap contribution as well as to count the number of energy-carrying molecules, which, in the present work, manifested the importance to widen inter-electrode distance to mitigate the detrimental influence of direct tunnelling on the thermoelectric performance of single-molecule junctions.

Figure 4: Anchor-dependent contact mechanics.
figure 4

(a) Equivalent circuit of molecular junctions consisting of m molecules with resistance RSMJ and thermovoltage V2 bridging a Au electrode gap. Contribution of direct tunnelling is included as the resistance Rgap and the tunnelling thermopower component Vgap connected in parallel. Rs is the thermovoltage sensing resistor connected in series to the junctions. (b) Direct tunnelling contribution. The thermovoltage versus conductance characteristics complies with the circuit model assuming quadruple-molecule junctions with variable size of vacuum gap with 1/Rgap from 1 × 10−4 G0 to 1 × 10−1 G0. (c) Energy-optimized structure of BDA quadruple-molecule junctions. (d) Seebeck coefficients S estimated by taking the thermopower of Au nanocontacts as reference. (e,f) Contact mechanics of BDA (e) and ABT (f). Junction images show energy-optimized structures. Arrows point toward stretching of junctions defined as the distance Lc between the molecule-linked Au atoms. Au-molecule bond lengths and angles at the both sides are plotted with respect to Lc.

Full size image

Anchor dependence of molecular thermopower

Quantitative evaluations of molecular junction thermopower involves intriguing argument about the temperature differential in the atomic scale systems49. Here, we instead examined a comparative analysis of the anchor dependence of the molecular junction thermopower by exploiting the Au junctions as an atomic thermometer to characterize the temperature differential. The slope α of the linear VAu − Vh2 dependence constitutes a well-defined Seebeck coefficient of the metal nanocontacts SAu = −αAu/β probed in the present measurements, where β is a coefficient that converts the heater voltage to the temperature difference as ΔT = βVh2. Here, SAu contains a bulk contribution of Sbulk = 1.96 μV/K50 in addition to the quantum thermopower Sq at the atom-sized contacts as they were connected to millimeter-long leads. We calculated β by comparing SAu = Sq − Sbulk to the experimental −αAu with Sq = −0.75 μV/K for ballistic Au atom-sized contacts33 whose radius rc is much smaller than the electron mean free path l in the conductance range of 1 G0 < G < 5 G0. This yielded SBDT = 9.5 μV/K for VP2 of Au-BDT-Au bridges, which fairly agrees with the previous literatures33. Comparatively, BDA and ABT exhibited higher thermoelectric power of SABT = 10.6 μV/K and SBDA = 10.3 μV/K, respectively (Fig. 4d).

Geometrical dependence of molecular junction thermopower

We have observed a concomitant enhancement of the conductance and thermopower in highly-stretched thiol-anchored molecular junctions. We explored the underlying contact mechanics by first-principles calculations of junction motifs under various inter-electrode distance conditions Lc, which is defined as the displacement of the molecule-linked Au atoms from the optimized configurations, to unveil the cause of stretching-induced thermopower enhancement observed for the thiolate molecules. We found that while BDAs cannot withstand the tensile straining above 1.6 Å (Fig. 4e), due to the restricted Au-NH2 bond configurations that hinders the conformational degrees of freedom of molecules in the electrode gap51, Au-S bond angles and lengths are more amenable to the mechanical stretching reflecting the diverse forms of covalent interactions between the sulphur and Au surface atoms (Fig. 4f)52, which allowed ABT to take upright conformations. Here, it is worth noting that the Au-S bond in ABT is capable of being elongated by up to 1 Å whereas the NH2-Au links can be pulled apart by less than 0.3 Å. Under this level of bond elongation, it is anticipated theoretically that G increases by junction stretching due to the entailed shift in the conducting molecular orbital level toward the electrode Fermi level EF53,54,55. In a framework of single-particle model, the mechanical modulation of molecular states also anticipates thermopower enhancement since the slope of transmission curve at EF tends to be steeper as the transport becomes closer to a resonance, the overall trend of which explains why the mutual enhancement of G and ΔVj was observed only in the thiol-anchored molecular junction, thereby suggesting in turn that the electromechanical characteristics would rather be a universal feature in stretched molecular junctions having at least one Au-S link56, the verification of which calls for future efforts to explore the mechanical effects on various organic molecules other than the mono-benzene derivatives studied in the present work. These findings provide a guide for designing optimal anchoring groups for achieving high thermoelectric power with single-molecule junctions.

Methods

Fabrication of heater-embedded MCBJs

Microelectrodes were formed on a polyimide coated phosphor bronze substrate by photolithography and radio-frequency magnetron sputtering of a Cr/Au (2 nm/50 nm thickness) layer followed by lift-off through sonication in N,N-dimethylformamide. Subsequently, Al2O3 thermal bath was created by electron beam lithography, inductively-coupled plasma sputter deposition of Al2O3 (40 nm thick), and the lift-off process. On the heat reservoir, Pt coils and a Au nanojunction were further prepared by two additional electron beam lithography processes. Finally, the polyimide layer was deep-etched by 2 μm in depth through reactive ion etching using O2 etchant gas so as to free the Au junction from the substrate. The free-standing length of the junction was approximately 2 μm, which provided the attenuation factor r of 1.3 × 10−4 as calibrated by measuring electrode gap size dependence of the tunnelling current (Fig. S9).

Formations of molecular junctions

A heater-embedded MCBJ was mounted on a sample stage where there were two counter supports. On the other side, there was a piezo-driven pushing rod that expands or shrink by changing the voltage Vp by ΔVp with ratio −1 μm/Vp. In experiments, a droplet of dilute toluene solution of molecules (either BDA, BDT, or ABT) at concentration 5 μM was added on a Au junction. The MCBJ beam was then bent mechanically by moving the pushing rod to break the Au junction, which led to the molecules to adhere chemically on the fracture surface. A chamber was then evacuated to a pressure around 10−6 Torr so as to remove the solvent. Thereafter, stretching of the junction was implemented by a feedback control of the piezo-actuator expansion. Specifically, the strain rate was set to 1 nm/s during elongation of Au nanocontacts until G decreased to below 6 G0. After that, the junction elongation speed was set to be 0.5 pm/s by applying a Vp ramp of 4 mV/s. This slow straining yielded enough amount of time for precise measurements of the junction thermopower and conductance. When G eventually dropped to lower than 10−5 G0, Au electrodes were reconnected. The series of breaking/connecting processes were repeated for 200 times at each Vh condition from 2.0 V to 5.0 V.

Thermoelectric voltage and electrical conductance measurements

The electrical conductance and thermovoltage of junctions were measured simultaneously during the tensile stretching. For this, a constant dc bias voltage Vh was applied to a Pt microheater throughout the experiments to create a temperature differential at the junctions necessary to induce detectable amount off thermovoltage. Furthermore, a picoammeter/source unit (Keithley 6487) was used to apply the dc voltage Vb = 0.1 V and acquire the current flowed through the junctions, from which the conductance G = I/Vb was obtained (the deduction included subtraction of a serial resistance). After the acquisition of G, Vb was set to zero and the potential drop Vm at the thermovoltage-sensing resistor having variable resistance Rs from 10 kΩ to 1 MΩ was obtained using a nanovoltmeter (Keithley 2182). Rs was changed electrically during the junction breaking in response to G by using a home-built relay device. The junction thermoelectric voltage ΔVj was computed from Vm considering the division at Rs and 1/G. Subsequently, Vb was switched back to 0.1 V for the next conductance measurement. The sampling rate of G and Vm was about 3 Hz.

First-principle calculations

Gaussian 09 package was employed to characterize mechanical stretching effects on molecular junction configurations. The atomic motifs of a single-molecule junction were modelled by respectively placing BDA, or ABT between two Au14 clusters. Density functional theory (DFT) level of calculations were performed with B3LYP hybrid functional for optimizing the junction structures. The basis sets of 6–31 G + (d, p) and LANL2DZ were employed for (C, H, S, N) and (Au), respectively. Completing the optimization processes, the distance between the two molecule-linking Au atoms was widened gradually by 0.2 Å steps. At every each step, the structural optimization was performed and the bond length as well as the angle at the metal-molecule links were measured from the coordination of the atoms. The series of calculations were continued until the molecules bridged the Au clusters.

Theoretical derivation of quadruple molecular junction strucutre

To deduce the parallel bridging structure of multiple molecules in an electrode gap, we started modelling from an optimized structure of a single-molecule junction wherein ABT is placed between two Au14 clusters. In the optimized geometry, N-C and S-C bonds of the ABT molecule leaned to 97.4° and 99.7° from [111] direction of each Au14 cluster. By expanding a Au14-ABT-Au14 motif to Au22-ABT-Au22 structure, we constructed the slab model of 3 × 3 supercell and then conducted geometry and cell optimization calculations by using a density functional based tight binding (DFTB) method with the AuOrg Slater-Koster parameters under a periodic boundary condition. All calculations were performed by using Material Studio package. The optimized lattice parameters were a = b = 8.02 Å and c = 42.9 Å, α = 118°, β = 117° and γ = 61.0°, and the atomic distance of Au for molecular transport and direct tunnelling was evaluated as 9.52 Å and 6.29 Å, respectively55,56.

Additional Information

How to cite this article: Tsutsui, M. et al. Roles of vacuum tunnelling and contact mechanics in single-molecule thermopower. Sci. Rep. 7, 44276; doi: 10.1038/srep44276 (2017).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.