Abstract
Due to its time-reversal nature, optical phase conjugation generates a monochromatic light wave which retraces its propagation paths. Here, we demonstrate the regeneration of a subwavelength optical focus by phase conjugation. Monochromatic light from a subwavelength source is scattered by random nanoparticles, and the scattered light is phase conjugated at the far-field region by coupling its wavefront into a single-mode optical reflector using a spatial light modulator. Then the conjugated beam retraces its propagation paths and forms a refocus on the source at the subwavelength scale. This is the first direct experimental realisation of subwavelength focusing beyond the diffraction limit with far-field time reversal in the optical domain.
Similar content being viewed by others
Introduction
A time reversal mirror (TRM) reflects a wave which is a time-reversed solution of the wave equation of the original impinging wavefront on the system. Regardless of the complexity of the system, TRM generates a wave that exactly retraces its original paths, as long as the system satisfies the time-reversal symmetry. This intriguing property of TRMs suggests promising applications, especially for wave transport in inhomogeneous systems where conventional mirrors are unusable. It permits a wave to be delivered through a disordered system without full knowledge of the system. With the aid of developments in electromagnetic devices, TRMs have been demonstrated in acoustic1 and microwave regimes2 by directly recording a waveform of interest and generating a time-reversed replication of it.
However, in the optical domain, true means of achieving TRM have not been realised, mainly due to the limited bandwidths of electronic devices, where the oscillation frequencies of optical waves are still several orders faster than the current state-of-the-art technology. Alternatively, a phase conjugation mirror (PCM) can be used to demonstrate the time-reversal of monochromatic light, since the complex conjugation of a monochromatic wave is the time-reversed solution of the original wave equation, where the medium is lossless and satisfies the reciprocity3. Historically, PCM was first demonstrated in the 1960s using the holographic principle to record a permanent wavefront on a nonlinear optical material and then replay it with a phase-conjugated reference beam4. Later, PCMs were demonstrated with degenerated four-wave mixing methods5, stimulated Brillouin6 and Raman7 scattering methods. Recently, using electronic devices, digital versions of PCMs have been demonstrated in various applications8,9,10,11,12,13,14.
An important aspect of the TRM is its ability to focus beyond the diffraction limit by reversing waves originating from a subwavelength-sized source containing evanescent components15. This suggests promising applications in various fields such as telecommunications16, imaging17,18, and lithography19. In practice, to realise a time-reversal system of waves from a subwavelength source, one also needs to generate a time-reversed replication of the source, such as a subwavelength sink20, which limits the application potential.
However, recently it was shown that this practical barrier can be overcome by utilising an inhomogeneous environment16. The theoretical analysis states that the time-reversed electromagnetic field from a subwavelength dipole source in an inhomogeneous medium is proportional to the imaginary part of the dyadic Green’s function of the system21 which reflects the spatial distribution of the electromagnetic properties of the surrounding medium22,23. After first being realised in the microwave domain16, there have been attempts to demonstrate this concept in the optical domain. However, although a theoretical study showed promising results24, it has still not been realised due to the challenging experimental scheme that is required.
Here, we experimentally demonstrate the time-reversal of a subwavelength optical focus containing near-field information, with far-field optical phase conjugation. A monochromatic optical field from a subwavelength light source was scattered by random nanoparticles, which effectively converted the evanescent near-field components of the incident wave to propagating far-field components of the scattered wave. By modulating the wavefront of the scattered field using a spatial light modulator (SLM), the scattered far-field was coupled into a single-mode optical reflector, which generates a phase-conjugated beam by utilising its ‘single-mode nature’12. Due to the reci time-reversal propertie of the multiple light scattering, the phase conjugated beam is refocused into the source position with a subwavelength scale. To the best of our knowledge, this is the first direct experimental realisation of generating a subwavelength focus by exploiting the time-reversal nature of waves in the optical domain. This time-reversal approach of delivering subwavelength optical information will open new avenues in various optical fields such as telecommunication, imaging and lithography.
Results and Discussion
The idea is schematically illustrated in Fig. 1. When a monochromatic light from a subwavelength source is directed onto a conventional mirror, it generates a diverging wavefront following the laws of reflection [Fig. 1(a)]. When the light from the source is reflected by a PCM, the light retraces its propagation path and focuses on the source position. However, the size of this phase conjugated focus is larger than the original subwavelength focus since the spatial frequency components of the wave beyond the Abbe’s diffraction limit decay exponentially upon propagation [Fig. 1(b)]. By placing an inhomogeneous medium, where the spatial variation of the dielectric property of the medium is smaller than the size of the diffraction limit, at a proximity of the light source, PCM can reverse the subwavelength focus [Fig. 1(c)] by exploiting multiple light scatterings; high spatial frequency components of the wave is scattered by the inhomogeneous medium and converted into the propagating far-field components of the wave. Thus, when the scattered wave is phase conjugated at the far-field region, the light retraces its scattering process and regenerates the subwavelength focus.
To time-reverse the monochromatic optical wave, we employed a digital optical phase conjugation system, which couples the optical field into a single-mode optical reflector. The wavefront of a scattered field from a disordered medium consisting of random nanoparticles is modulated by a phase-only SLM to couple the optical field into the single-mode reflector consisting of a sub-diffraction limit sized pinhole. We utilise the fact that two counter-propagating monochromatic waves in a single-mode system are in phase-conjugated states where the shapes of the wavefronts into and from the single-mode pinhole are identical. Thus, a time-reversed wave is realised by coupling an optical field into a single-mode pinhole and simply reversing the propagation direction of the optical field at the pinhole12.
The use of the single-mode optical reflector avoids the issue arises from the requirement for precise optical alignment11 in conventional digital optical phase conjugation systems, which is extremely challenging, and typically limits the experimental realisation of reversing subwavelength focus in the optical domain. Our system does not require precise optical alignment or wavefront correction systems25 to realise PCM, but as an expense of an iterative optimisation process of the SLM.
Figure 2 shows the detailed experimental scheme. A modified commercial near-field scanning optical microscopy (NSOM, WiTec Alpha SNOM) system is used to generate a time-reversed monochromatic subwavelength optical focus together with PCM. A beam from a narrow band diode-pumped solid-state laser (λ = 532 nm, 100 mW, Shanghai Dream Laser) is split into a signal arm and a conjugation arm. The signal beam is coupled to an NSOM tip aperture which is in contact with a turbid sample and acts as a subwavelength point source. The light from the subwavelength source is scattered by the turbid medium made up of zirconium dioxide random nanoparticles. A scattered far-field at the back side of the turbid medium is collected by an objective lens (NA = 0.8, Nikon) and relayed into a PCM by 4-f telescopic imaging systems.
The PCM consists of a SLM (x10468-01, Hamamatsu) and a single mode pinhole. The scattered field from the turbid medium is directed to the SLM and focused into the single mode pinhole by a lens. The SLM was placed at the conjugated plane of the back surface of the sample with a lateral magnification of x600. Thus, the granular size of the far-field scattered speckle pattern imaged on the SLM is about 12 times larger than the pixel size of the SLM. The single-mode pinhole is placed at a Fourier plane of the SLM where a size of the pinhole was set to be 20 μm, which is about 1.5 times smaller than a size of diffraction limited spot of the optical system. The intensity signal from the scattered wave which propagates through the pinhole was collected by a single photon counting module (SPCM, ID100-20, ID Quantique) for optimisation of the wavefront, in order to couple the scattered field into the single-mode pinhole. After optimisation, a conjugation beam illuminates the pinhole from the opposite direction.
We adopted the spatial frequency domain for an optimisation process; wave vectors were used as an orthogonal basis for optical modes. Optimal phase value for each optical mode was found by measuring intensity signal of the transmitted beam through the turbid medium and pinhole while imposing a total of eight different phase values on the wave vector components of the incident beam using the SLM. During the optimisation process, an area of the wavefront of the incident beam was divided into two and optimal phase values for each wavefront segment were found individually; an imposing phase value on one area remains zero and serves as a reference beam during the optimisation of the other area. On average, about 240 optical modes were used, and the detected signal at the SPCM was enhanced about 120 times.
After the optimisation, the propagating direction of the beam at the pinhole was reversed and the scattered field redirected to the position of the subwavelength light source. This time-reversed near-field signal was acquired by collecting the coupled electromagnetic field from the NSOM tip aperture using a photomultiplier tube. Raster scanning was performed to get a 2D image of the near-field signal at the proximity of the sample.
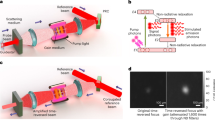
The experimental result is shown in Fig. 3. A subwavelength point source is generated by the NSOM probe tip [Fig. 3(a)]. The size of the aperture of the tip was measured to be ~55 nm, which defines the size of the subwavelength light source.
(a) Subwavelength focus is made by illuminating NSOM probe tip. Inset, bottom view of the NSOM probe aperture. (b) Far-field time-reversal of the subwavelength focus in a homogeneous environment. (c) Using zirconium dioxide random nanoparticles, a near-field focus is regenerated by far-field time-reversal of multiply scattered light. (d) Intensity distributions of the foci. Red dash-dotted line, an ensemble average of far-field foci. Green solid line, near-field foci (n = 18). Black thick solid line, an ensemble average of the near-field foci.
At first, the focus was time-reversed in a homogeneous medium; that is, the focus was reversed through the air. A bare glass was placed at the image plane of the SLM. The NSOM probe tip was positioned 10 μm above the bare glass. The light from the NSOM tip was directed to the SLM and optimised. The time-reversal of the optimised beam generated a focus at the position of the NSOM probe tip. The size of the focus was measured by raster scanning of the NSOM probe tip. As shown in Fig. 3(b), the FWHM of the resultant focus was measured to be 780 nm, which is decided by the optical transfer function which the effective numerical aperture of the optical system can support due to diffraction.
Next, we inserted random zirconium dioxide nanoparticles in the proximity of the NSOM tip probe to couple the evanescent near-field component of the light to the propagating far-field. The transmitted far-field from the turbid medium was directed to the SLM and collected by the SPCM. After optimisation, the beam was time-reversed by the PCM. The time-reversal of the scattered far-field cause it to retrace its propagation path, and it is focused on the position of the subwavelength source. The image of the resultant focus was acquired by raster scanning of the near-field signal using the NSOM. As expected, the size of the acquired focus was beyond the diffraction limit.
We quantitatively measured the FWHM of the focus [Fig. 3(c)]. The average size of the focus was measured to be 151 ± 17 nm (λ/3.5, n = 18) which is 5 fold smaller than using PCM in the homogeneous environment in our system, and 2 fold smaller than the perfect imaging system of a numerical aperture of 0.8. However, the size of the focus is still larger than the subwavelength source since the spatial variation of electromagnetic properties of the zirconium dioxide random nanoparticles cannot support spatial frequency components larger than ~2π/150 nm (see Supplementary Fig. S1). This spatial frequency limit can be enhanced by using smaller random nanoparticles as a near-field to far-field coupling medium.
It is worth noting that regardless of the incompleteness of the optical phase conjugation system, the near-field focus was effectively regenerated. In our experiment, a significant fraction of incident light was backscattered by the thick layer of zirconium dioxide nanoparticles (~10 μm) and an only small fraction of transmitted light was phase conjugated at the opposite side. In addition, only phase profile of the scattered field was conjugated by the SLM where a spatial intensity profile of the scattered field from the disordered medium is also non-uniform when it is incident at the SLM plane. However, still, the subwavelength focus was clearly reconstructed in our experimental demonstration. In practice, the most important factor affects the quality of reconstruction when using the digital optical phase conjugation system is the number of controlled optical modes. The use of a limited number of optical modes results in undesirable background speckle noise where a signal to background noise ratio is directly proportional to the number of controlled optical modes12,26. In other words, the fidelity of the reconstruction can be enhanced by utilising a large number of optical modes.
We should also note that the principle of the present approach is significantly different from the previous reports17,18. For example, ref. 17 is limited to imaging of optical near-field information via the computation of the time-reversal of a transmission matrix of a random nanoparticles sample. In addition, although the generation of sub-wavelength focus has been reported previously18, the principle of the previous work is a wavefront shaping approach27 whereas present work demonstrates the direct time-reversal of a subwavelength source that the propagating direction of the field was physically reversed and retraces its original paths. More importantly, the time-reversal approach can regenerate arbitrary shapes of optical fields in principle. This three different approaches, which are the transmission matrix, the wavefront shaping, and the optical phase conjugation, for addressing light transport in inhomogeneous media will be merged to open a new avenue for controlling near-field signal using multiple light scattering17,18,28,29.
Conclusion
In summary, we demonstrated the regeneration of a subwavelength optical focus using random nanoparticles and PCM, which is in principle the time-reversal of a monochromatic light wave. The time-reversal of the scattered far-field rewinds the scrambled paths of the propagating optical waves in a disordered medium, and it couples to evanescent high spatial frequency components to reconstruct a subwavelength optical focus. To the best of our knowledge, this is the first direct demonstration of generating a subwavelength focus with far-field-time-reversal in the optical domain.
Although we have demonstrated the regeneration of a subwavelength focus, the present approach can also be readily expanded to the multi-colour and the polarisation dependent subwavelength focusing because it has been shown that multiple light scattering in turbid media is strongly dependent on the wavelength and the polarisation states of light fields30,31. Furthermore, when combined with a plasmonic metalens and polychromatic light32, it will also enable deep sub-wavelength focusing.
Additional Information
How to cite this article: Park, J. et al. Time-reversing a monochromatic subwavelength optical focus by optical phase conjugation of multiply-scattered light. Sci. Rep. 7, 41384; doi: 10.1038/srep41384 (2017).
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
Fink, M. et al. Time-reversed acoustics. Rep. Prog. Phys. 63, 1933 (2000).
Lerosey, G. et al. Time reversal of electromagnetic waves. Phys. Rev. Lett. 92, 193904 (2004).
Potton, R. J. Reciprocity in optics. Rep. Prog. Phys. 67, 717 (2004).
Kogelnik, H. Holographic image projection through inhomogeneous media. Bell. Syst. Tech. J. 44, 2451–2455 (1965).
Yariv, A. & Pepper, D. M. Amplified reflection, phase conjugation, and oscillation in degenerate four-wave mixing. Opt. Lett. 1, 16–18 (1977).
Zel’dovich, B. Y., Popovichev, V., Ragul’Skii, V. & Faizullov, F. Connection between the wavefronts of the reflected and exciting light in stimulated Mandel’shtam Brillouin scattering. Sov. Phys. J. Exp. Theor. Phys. 15, 109–112 (1972).
Zel’dovich, B. Y. & Shkunov, V. Wavefront reproduction in stimulated Raman scattering. Quant. Electron. 7, 610–615 (1977).
Cui, M. & Yang, C. Implementation of a digital optical phase conjugation system and its application to study the robustness of turbidity suppression by phase conjugation. Opt. Express 18, 3444–3455 (2010).
Wang, Y. M., Judkewitz, B., DiMarzio, C. A. & Yang, C. Deep-tissue focal fluorescence imaging with digitally time-reversed ultrasound-encoded light. Nat. communications 3, 928 (2012).
Xu, X., Liu, H. & Wang, L. V. Time-reversed ultrasonically encoded optical focusing into scattering media. Nat. Photon. 5, 154–157 (2011).
Hillman, T. R. et al. Digital optical phase conjugation for delivering two-dimensional images through turbid media. Sci. Rep. 3 (2013).
Lee, K., Lee, J., Park, J.-H., Park, J.-H. & Park, Y. One-wave optical phase conjugation mirror by actively coupling arbitrary light fields into a single-mode reflector. Phys. Rev. Lett. 115, 153902 (2015).
Lai, P., Wang, L., Tay, J. W. & Wang, L. V. Photoacoustically guided wavefront shaping for enhanced optical focusing in scattering media. Nat. Photon. 9, 126–132 (2015).
Horstmeyer, R., Ruan, H. & Yang, C. Guidestar-assisted wavefront-shaping methods for focusing light into biological tissue. Nat. Photon. 9, 563–571 (2015).
Carminati, R., Saenz, J., Greffet, J.-J. & Nieto-Vesperinas, M. Reciprocity, unitarity, and time-reversal symmetry of the S matrix of fields containing evanescent components. Phys. Rev. A 62, 012712 (2000).
Lerosey, G., De Rosny, J., Tourin, A. & Fink, M. Focusing beyond the diffraction limit with far-field time reversal. Science 315, 1120–1122 (2007).
Park, C. et al. Full-field subwavelength imaging using a scattering superlens. Phys. Rev. Lett. 113, 113901 (2014).
Park, J.-H. et al. Subwavelength light focusing using random nanoparticles. Nat. Photon. 7, 454–458 (2013).
Alkaisi, M., Blaikie, R., McNab, S., Cheung, R. & Cumming, D. Sub-diffraction-limited patterning using evanescent near-field optical lithography. Appl. Phys. Lett. 75, 3560–3562 (1999).
de Rosny, J. & Fink, M. Overcoming the diffraction limit in wave physics using a time-reversal mirror and a novel acoustic sink. Phys. Rev. Lett. 89, 124301 (2002).
Carminati, R., Pierrat, R., De Rosny, J. & Fink, M. Theory of the time reversal cavity for electromagnetic fields. Opt. Lett. 32, 3107–3109 (2007).
Birowosuto, M., Skipetrov, S., Vos, W. & Mosk, A. Observation of spatial fluctuations of the local density of states in random photonic media. Phys. Rev. Lett. 105, 013904 (2010).
Cazé, A., Pierrat, R. & Carminati, R. Spatial coherence in complex photonic and plasmonic systems. Phys. Rev. Lett. 110, 063903 (2013).
Pierrat, R., Vandenbem, C., Fink, M. & Carminati, R. Subwavelength focusing inside an open disordered medium by time reversal at a single point antenna. Phys. Rev. A 87, 041801 (2013).
Jang, M., Ruan, H., Zhou, H., Judkewitz, B. & Yang, C. Method for auto-alignment of digital optical phase conjugation systems based on digital propagation. Opt. Express 22, 14054–14071 (2014).
Vellekoop, I. M. & Mosk, A. Focusing coherent light through opaque strongly scattering media. Opt. Lett. 32, 2309–2311 (2007).
Mosk, A. P., Lagendijk, A., Lerosey, G. & Fink, M. Controlling waves in space and time for imaging and focusing in complex media. Nat. Photon. 6, 283–292 (2012).
Yu, H. et al. Recent advances in wavefront shaping techniques for biomedical applications. Curr. Appl. Phys. 15, 632–641 (2015).
Park, J. et al. Scattering Optical Elements: Stand-Alone Optical Elements Exploiting Multiple Light Scattering. ACS Nano, 10, 6871–6876 (2016).
Park, J.-H., Park, C., Yu, H., Cho, Y.-H. & Park, Y. Dynamic active wave plate using random nanoparticles. Opt. Exp. 20, 17010–17016 (2012).
Park, J. H., Park, C. H., Yu, H., Cho, Y. H. & Park, Y. K. Active spectral filtering through turbid media. Opt. Lett. 37, 3261–3263 (2012).
Lemoult, F., Fink, M. & Lerosey, G. A polychromatic approach to far-field superlensing at visible wavelengths. Nat. Commun. 3, 889 (2012).
Acknowledgements
This work was supported by KAIST, and the National Research Foundation of Korea (2015R1A3A2066550, 2014K1A3A1A09063027, 2012-M3C1A1-048860, 2014M3C1A3052537, 2016R1A2A1A05005320).
Author information
Authors and Affiliations
Contributions
J.P. performed the experiments and analysed the data. C.P., K.L., Y.-H.C. contributed experimental and analytical tools. Y.P. conceived and supervised the project. J.P. and Y.P. wrote the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Park, J., Park, C., Lee, K. et al. Time-reversing a monochromatic subwavelength optical focus by optical phase conjugation of multiply-scattered light. Sci Rep 7, 41384 (2017). https://doi.org/10.1038/srep41384
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep41384
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.