Introduction

Surface plasmons (SPs), or surface plasmon polaritons (SPPs), are propagating excitations of charge-density waves associated with the electromagnetic fields along the interface between a metal and a dielectric medium1,2,3,4,5,6. Surface plasmons in metallic nanostructures possess many advantages, such as enhanced transmission through subwavelength apertures7,8, amplification by stimulated emission of radiation9,10, enhanced photoluminescence from quantum wells11, enhanced fluorescence12,13,14,15, and surface-enhanced Raman scattering12,16. Moreover, there are many potential applications such as subwavelength imaging1,17,18, waveguiding devices below the diffraction limit19,20, biosensing21, and biological detection22. Therefore, designing and fabricating subwavelength optical devices using SPs23 open up new horizons of the research in this field.

With the tunable luminescence properties, such as localized surface plasmon resonances (LSPRs)24, plasmon-induced fluorescence enhancement15, broad excitation spectra, narrow emission spectra, and size-dependent emission25, quantum dot (QD) has recently attracted much attention for its ability to act as a photon detector25 or being an excellent single photon source26,27,28. On the other hand, metallic nanowire (MNW) is also an important class of plasmonic nanostructure for the SPs29,30,31,32,33,34, resonators30, sub-diffraction limit plasmon wave31, and plasmon lasers10.

Owing to the numerous advantages of both QD and MNW, QD that couples to MNW has emerged as an appealing system for coherent single-photon transport35 and long-range energy transfer with a high efficiency36. By the virtue of coherent transport, there are many extended applications, such as transistors37, plasmonic nanolaser38, quantum switch39,40, single-photon source41, biological sensing42,43, and nanoantennas44,45. Furthermore, the hybrid systems with exciton-plasmon interaction can reveal the features of cavity quantum electrodynamics46,47,48,49,50,51 and have applications in quantum information processing5,52,53,54,55.

A variety of experimental33,36,41,56,57,58,59,60,61,62 and theoretical works9,35,39,40,42,47,48,49,50,51,63,64,65,66,67,68,69,70,71,72,73,74,75,76,77 have been focused on the photon transport properties in the NW-QD systems. Recently, an experiment has reported that two QDs located nearby the NW are separated not only with a distance d, but also with an angle ϕ along the azimuthal direction60. Therefore, the difference in the angles between the QDs should be taken into account when investigating the scattering properities33,34,48,78.

In this work, we study the scattering spectra of the nanowire SPs coupled to double QDs with an azimuthal angle difference. We also consider the system comprising N QDs. Taking into account the angle difference between the dots, we study the scattrering properties of the SPs by using the transfer matrices. Compared to the double-dot case, we find the transmission/reflection profile reveals the periodic behavior for the three-dot case58 when rotating each QD along direction. We further study the scattering spectra of the Hybrid Quantum System (HQS) consisting of QDs and a metal nanoparticle79,80,81. It can be viewed as a cavity82,83,84,85 coupled to the NW-QD system. We find that the spectra reveal sharp and asymmetric response line shapes in the hybrid configuration. We analyze the results and provide explanations for the appearance of the Fano resonance.

Results

The Model

Lets us consider two identical QDs near a cylindrical metal nanowire. Assuming that they have the same separation from the metal wire, both with energy spacing ħω0, separated not only with a distance d, but also with an angle ϕ as shown in Fig. 1. Since the propagating modes are along the and directions, the phase differences acquired by the second dot are ikx and inϕ, where k and n are the wave number and quantum number governing the x and φ components, respectively. Under the rotating wave approximation, the interactions between the propagating photons and quantum dots can be described by the Hamiltonian,

Figure 1
figure 1

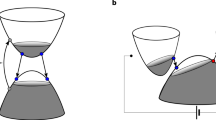
Schematic diagram of the two-QD model.

Schematic diagram of the model: a metal nanowire coupled to two semiconductor QDs. A single surface plasmon injected from the left can be scattered by the two QDs which are placed on top of the nanowire with a inter-dot distance d along the direction and an azimuthal angle ϕ along the direction.

Full size image

where represents the diagonal element of the jth QD operator with a atomic resonance frequency ω0 and represents the rasing operator. Here, (ak,n) is the creation (annihilation) operator of the SP. We assume a SP is incident from the left with energy Ek = vgk for the nth mode. Here, vg and k are the group velocity and wave number of the incident SP, respectively. Since the SPs are confined on the surface of the cylindrical nanowire, the summation of n in Eq. (1) stands for the contributions from all the possible n modes, and g is the coupling constant between the SP and QD exciton. Note that Γ′ ≡ γ0 + Γ0 is the total dissipation including the decay rate into free space γ0 and other dissipative channels Γ0. By using the Fourier transform, each term in Eq. (1) can be easily represented in real space

where is a bosonic operator creating a right-going (left-going) SP at x and φ. The stationary state of the above QDs-NW coupled system with the energy matching condition Ek = vgk can be written as

where |g1, g2〉 |0〉sp denotes that both the QDs are in their ground states with zero SP state, and is the probability amplitude that the jth QD jumps to its excited state. Suppose that a SP is incident from the left, the scattering amplitudes and take the forms

where t and r are the transmission and reflection amplitude, respectively. Here, a and b represent the probability amplitudes of the SP between x = 0 and d, φ = 0 and ϕ, respectively. Besides, θ(x) is the unit step function. From the eigenvalue equation, H|Ek〉 = Ek|Ek〉, one can obtain the following relations for the coefficients:

where is the detuning between the incident SP energy with Ek and the QD exciton energy ω0. By solving Eq. (5), the exact forms of the transmission and reflection amplitudes, t and r, are given by

Here, we have defined the function , where is the decay rate into the SP modes. The transmission and reflection probabilities of the SP are defined as T = |t|2 and R = |r|2, respectively, as shown in Fig. 2.

Figure 2
figure 2

Effect from azimuthal angle difference in transmission spectra.

The transmission probabilities |t|2 (dashed line) and reflection probabilities |r|2 (solid line) of the surface plasmon scattered by two QDs with azimuthal angle diference as a function of detuning . When d = 0 (here, it means the inter-dot distance is very small compared with the wavelength of incident fields), the two QDs are assumed to be at the same position.

Full size image

Plasmon Scattered By N Quantum Dots

We now consider further a general model consisting of N identical QDs coupled to the SP. Under the rotating wave approximation, the interaction Hamiltonian becomes

where d(j) is the distance between the first dot and jth dot, and ϕj is the angle of jth QD with respect to the first QD along the direction when setting d1 and ϕ1 being zero. On the other hand, the scattering property of a nanowire coupled to N identical QDs can also be studied by applying the transfer-matrix method. Let us briefly review the transmission amplitude t and the reflection amplitude r for the case of a single-dot coupled to the nanowire:

where F has been defined in Sec. II. By making use of the transmission and reflection coefficients in Eq. (8), the transfer matrix Tq of the NW coupled to a single-QD can be written as

Thus, the transfer matrix τ for the entire system is determined by

where

represents the transfer matrix of free propagation with . Consequently, the total reflect and transmit amplitudes with N QDs can be obtained:

In order to make a comparison to the double-dot case, we specifically consider the three-dot case58 as shown in Fig. 3. By solving the eigenvalue equation with N = 3 in Eq. (7) or Eq. (12), the transmission and reflection amplitudes can be obtained. For simplicity, we only show the transmission amplitudes

Figure 3
figure 3

Schematic diagram of the three-QD model.

Schematic diagram of the three QDs coupled to a metal nanowire with azimuthal angle difference along the direction.

Full size image

where we have defined the phase terms ζ ≡ 2nϕ2, α ≡ 2[kd3 + n(ϕ2 + ϕ3)], β ≡ 2(kd2 + 2nϕ2) and γ ≡ 2[k(d3 − d2) + nϕ3], respectively. Here, we are interested in the scattering spectra resulting from the varying angles of QD-2 and QD-3. Figure 4 shows the scattering spectra as functions of the angles ϕ2 and ϕ3. We find that the transmission (reflection) coefficient reveals the periodic maximum (minimum) value 1 (0), when keeping one QD fixed at the certain angle along the direction.

Figure 4
figure 4

The interference of the azimuthal differences for the three-QD case.

Analysis of the scattering probabilities of three quantum dots with the azimuthal angle differences between the second QD (ϕ2) and third QD (ϕ3) for the mode n = 1, k = 1, d2 = π, d3 = 2π and δ/Γpl = 1. Here, the red regions indicate the high transmission probability around unity.

Full size image

QDs-NW System Coupled To Cavity

Recently, hybrid quantum system (HQS) has attracted renewed attention for its prospect of applications in future quantum devices. Here, we consider the HQS of the QDs (with nanowire) coupled to a metal-nanoparticle (MNP) as shown in Fig. 5. it was reported82,83,84,85,86,87 that, for the very small separation between a quantum emitter and a metal nanoparticle, the spectral density of the surface electromagnetic fields of the nanoparticle becomes Lorentzian. This indicates that the emitter-nanoparticle system can form an effective cavity quantum electrodynamics (QED) system. We therefore study the scattering spectra of two kinds of HQS comprising the cavity coupled to two QDs. For the first case, we assume both QD-1 and QD-2 are coupled to the same cavity as shown in Fig. 6. In real space, the Hamiltonian of the cavity photon with a loss rate κ can be written as

Figure 5
figure 5

Schematic diagram of the QD-wire-nanoparticle hybrid system.

Schematic diagram of the hybrid quantum system comprising QDs-wire and metal nanoparticle. The coupling strengths between the metal nanoparticle and QD-1, QD-2 are J1 and J2, respectively. The metal nanoparticle can be viewed as a special “cavity” in the strong coupling regime.

Full size image
Figure 6
figure 6

Schematic sketch of the effective hybrid model: common cavity.

Schematic sketch of the effective hybrid model. Both QD-1 and QD-2 are coupled to a common cavity with a loss rate κ of the cavity photons. The transmission spectrum shows the Fano lineshape due to the interference between different channels. Here, the coupling strengths between cavity and QD-1, QD-2 are J1 and J2, respectively.

Full size image

where is the diagonal element of the cavity operator, and is the bosonic creation(annihilation) operator of the cavity mode. Here, Jj represents the coupling strength between the cavity and jth QD. The transmission and reflection coefficients can be written as

Here, we have defined the function , , and the phase term . The detuning between the incident SP energy (with Ek) and the cavity resonant frequency (ωc) is labeled by the symbol ε. For the second case, we study the configuration that each QD is individually coupled to its own cavity as shown in Fig. 7. Here, we have assumed the two cavities are identical for simplicity. The Hamiltonian of the composite system can be rewritten as

Figure 7
figure 7

Schematic sketch of the effective hybrid model: individual cavity.

Schematic draw of the model for each QD is individually coupled to different cavities with the same loss rate κ of the cavity photons.

Full size image

where represents the diagonal element of the ith cavity operator, and is the bosonic creation(annihilation) operator of the jth cavity mode. Also, the scattering coefficients can be obtained by solving eigenvalue equation:

We plot in Figs 8, 9 and 10 the transmission probabilities T = |t|2 (dashed lines) and reflection probabilities R = |r|2 (solid lines) as a function of the detuning for the both cases. In plotting Fig. 8, we find that when kd1 + nϕ2 = πm with m being an integer and being close to with appropriate value of ϵ/Γpl, the transmission and reflection spectra have a more distinct Fano-type line shapes. In Fig. 9, when increasing the detuning ϵ/Γpl, the position of the Fano-type line shapes would be shifted from the right to the left along the δ/Γpl axis. For the second case, however, we can only observe two peaks with the absence of asymmetric Fano-type line shape as shown in Fig. 10. When increasing the detuning ϵ, the inter-peak separation is reduced rapidly.

Figure 8
figure 8

Scattering spectra for the two QDs coupled to common nanoparticle.

(a) The transmission (dashed) and reflection (solid) probabilities as a function of detuning , and when kd1 + nϕ2 = mπ with m being an odd (red) or even (black) integer. (b) When m is an odd integer and is close to with appropriate value of ϵ/Γpl, the typical transmission spectra present more distinct Fano resonance for the first case. For Γ′ = 0 and κ = 0, we plot the transmission probabilities T1(red-dashed), T2(green-solid), T3(blue-dot-dashed), T4(black-dotted) with the detuning ϵ/Γpl = 0.05, 0.6, 1.2, 3 when , , respectively. (c) The transmission probabilities T as a function of ϵ/Γpl = 1, for Γ′ = 0 and κ = 0 when , respectively.

Full size image
Figure 9
figure 9

Analysis of Fano resonance.

The transmission (dashed) and reflection (solid) probabilities as a function of , for Γ′ = 0 and κ = 0 when (a) ϵ/Γpl = 0.2, 0.3, 0.5, (b) ϵ/Γpl = 1.2, 1.5, 2, respectively. The position of the Fano lineshape can be shifted from the right to the left along the δ/Γpl axis when increasing the detuning ϵ/Γpl.

Full size image
Figure 10
figure 10

Scattering spectra for the two QDs coupled to individual nanoparticles.

For the second case, we set , Γ′ = κ = 0, and kd1 + nϕ2 = mπ with m being an integer. (a) The transmission (dashed) and reflection (solid) probabilities as a function of and ϵ/Γpl = 0.07. This panel shows a standard Breit-Wigner lineshape without the Fano resonance for two QDs. (b) The transmission probabilities as a function for (red-dashed), 0.7 (green-solid), 0.9 (blue-dot-dashed), 1.1 (black-dotted), respectively with ϵ/Γpl = 0.1. By adjusting coupling strength between each QD to the cavity, the position of each peak along the δ/Γpl axis can be controlled. (c) When , the overlap of two peaks makes the two QDs act like a single QD in transmission(dashed) and reflection(solid) probabilities with ϵ/Γpl = 0.07.

Full size image

Discussion

Since the Fano resonance only occurs in the first case, it is interesting to ask: What makes the two cases different? To answer this, let us note that, in Fig. 8(b), the stronger coupling strength of the two QDs to the cavity, the larger detuning ϵ is required to form the Fano-type line shapes for the first case. Contrarily, when J1 coincides with J2, the Fano resonance vanishes rapidly. In this regard, the Fano resonance arises from the constructive and destructive interference between the localized and delocalized channels by the virtue of the coupling of the two QDs to the same cavity. Here, the localized channel represents the single QD mode, and the delocalized channel denotes the hybridization mode of the cavity photon and the two dots88. The surface plasmons passing through the two channels carry different phases and result in the interference. On the other hand, we can easily control the position of each peak along the δ/Γpl axis by adjusting the coupling strength between each QD to the cavity in the second case as shown in Fig. 10. When J1 = J2, the overlapping of two peaks makes the two QDs collectively act like a single QD. The notable feature of these results indicates that the Fano-type line shape can’t be created due to the individual coupling to each own cavity. In other words, the difference between J1 and J2 is the primary cause of the Fano resonance.

In conclusion, the real-space Hamiltonians and transfer-matrix method are used to obtain the transport properties of SPs propagating on the surface of a silver NW coupled to QDs. The transmission and reflection spectra of the SPs depend not only on the position, but also on the azimuthal angle of the QDs. For the double-dot case, even the two QDs are placed at the same position in the -axis, changing the angle of a QD along direction also affects the reflection (transmission) spectra. For the triple-dot case, the transmission (reflection) coefficient reveals the periodic maximum (minimum) value when keeping one QD fixed at the certain angle along the direction. Moreover, when there is an additional cavity coupled to QDs, the Fano-type line shape can be created if both the QDs are coupled to the same cavity. The appearance of Fano resonances is attributed to the interference between the localized and delocalized modes.

Additional Information

How to cite this article: Kuo, P.-C. et al. Scattering of nanowire surface plasmons coupled to quantum dots with azimuthal angle difference. Sci. Rep. 6, 37766; doi: 10.1038/srep37766 (2016).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.