Abstract
In optical experiments one-sided reflectionless (ORL) and coherent perfect absorption (CPA) are unusual scattering properties yet fascinating for their fundamental aspects and for their practical interest. Although these two concepts have so far remained separated from each other, we prove that the two phenomena are indeed strictly connected. We show that a CPA–ORL connection exists between pairs of points lying along lines close to each other in the 3D space-parameters of a realistic lossy atomic photonic crystal. The connection is expected to be a generic feature of wave scattering in non-Hermitian optical media encompassing, as a particular case, wave scattering in parity-time (PT) symmetric media.
Similar content being viewed by others
Introduction
Scattering from complex potentials and the associated non-Hermitian Hamiltonians1 are usually introduced to describe dissipation or decay processes in open systems. Likewise light wave propagation phenomena through media with complex susceptibilities are genuine realizations of scattering from localized non-Hermitian potentials and provide a clear illustration of how Hermitian and non-Hermitian processes differ from one another. The optical scattering matrix S fully governs the propagation of light and, in particular, one-sided reflectionless (ORL) scattering of light waves impinging from “one” direction2,3,4,5,6 can be associated with a non-Hermitian degeneracy7 of the scattering matrix (also known as an exceptional point8). More intriguing phenomena appear, however, when coherent waves impinge on “both” sides of a complex potential9. Among them, coherent perfect absorption (CPA)10, which refers to complete absorption of both incident waves, is being extensively investigated11,12,13,14,15,16,17,18. The interest in CPA stems not only for fundamental reasons10,11,13, since it can be interpreted as the time-reversed counterpart of lasing and related to parity-time (PT) symmetry19, but also in view of its potential applications. Such efforts have spurred investigations and experiments in various areas that span, among others, absorption enhancement20, perfect energy feeding into nanoscale systems21, intersubband polaritons22, slow light waveguides23, graphene-based perfect absorbers24,25,26,27, and Fano resonant plasmonic metasurfaces28.
The concepts of one-sided reflectionless and coherent perfect absorption have remained so far separated from each other, probably because of the lack of suitable physical systems in which both features would be accessible. Here, we show how a lossy medium that exhibits ORL can in general also exhibit CPA. The connection is general, not restricted to PT symmetric media and could be easily observed in a realistic 1D lossy medium through smooth deformations of the system’s externally tunable parameters. We further argue how this connection, intrinsic to the structure of non-Hermitian degeneracies of scattering matrix S, can actually be extended to all points of a CPA-line. Such a line is a novel topological structure of non-Hermitian optical media predicted to occur next to a ORL-line. Although there has been a number of recent advances in each of these areas of research, particularly restricted to the case of PT symmetric media requiring a balance of loss and gain29,30,31,32,33,34, one-sided reflectionless and coherent perfect absorption – taken together – may lead to a more complete understanding of non-Hermitian optics in a large class of materials where absorption plays a key role for applications. Photodetectors, photovoltaics and non-reciprocal optical devices just to mention a few instances. The connection we present here is fairly general, hinges on non-Hermitian scattering degeneracies with common notions from quantum mechanics and, though clearly relevant to optics in view of one-way mirrors, cloaks of invisibility and coherent laser absorbers, may well be relevant to unusual phenomena recently observed for acoustic waves35,36,37,38,39,40,41.
ORL and CPA
The scattering properties of a 1D-medium are fully determined by the complex amplitudes t = tL = tR, rL and rR respectively for (reciprocal) transmission and reflection upon incidence from the left (L) or from the right (R). ORL means that rL = 0 with rR ≠ 0 (or vice versa). The CPA condition corresponds, instead, to a specific configuration of input beams, incident at the same time one from the left and one from the right with a definite phase relationship, which are completely absorbed by the sample. Thus, for this configuration of input beams, the output beams to the right and to the left are both vanishing. This means that the CPA input beams represent an eigenvector of the scattering matrix S with eigenvalue zero. As discussed below, the CPA condition can finally be stated as t2 = rRrL, i.e. det S = 010 (see Eq. (3)).
Thus, the main focus of the work is how to connect in general the two conditions rL = 0 (ORL) and t2 = rRrL (CPA) upon smooth deformations of medium’s external driving parameters. More specifically, for a lossy 1D-photonic crystal, the scattering properties near Bragg reflection can be described5,4 by the following model susceptibility

with χ0,  , and w being non negative real parameters, a the crystal period and the phases {α, β} defined within the interval [0, π]. The real part of the spatially independent background susceptibility is ignored for simplicity as it plays no significant role, while its imaginary part χ0 should be large enough with respect to
, and w being non negative real parameters, a the crystal period and the phases {α, β} defined within the interval [0, π]. The real part of the spatially independent background susceptibility is ignored for simplicity as it plays no significant role, while its imaginary part χ0 should be large enough with respect to  to have everywhere a lossy medium, i.e.,
to have everywhere a lossy medium, i.e.,  . In this rather generic model, the ORL condition (rL = 0) is simply attained when w = 05,4, in which case the real and imaginary parts of the susceptibility modulation χ(z) − iχ0 are spatially shifted by π/2 and satisfy the spatial Kramers-Kronig relations6. The reflection and transmission of a light beam with a wave-vector
. In this rather generic model, the ORL condition (rL = 0) is simply attained when w = 05,4, in which case the real and imaginary parts of the susceptibility modulation χ(z) − iχ0 are spatially shifted by π/2 and satisfy the spatial Kramers-Kronig relations6. The reflection and transmission of a light beam with a wave-vector  can be described on the basis of a minimal coupled-mode model accounting for Bragg scattering in a sample of length L ≫ a, as usual. Then, the CPA condition is attained when
can be described on the basis of a minimal coupled-mode model accounting for Bragg scattering in a sample of length L ≫ a, as usual. Then, the CPA condition is attained when

where  (with Re[η] > 0 due to losses). The last term in Eq. (2) holds when |eηL| ≫ 1 (|t| ≪ 1) and w ≪ 1, and this is precisely the regime we are interested in as it can occur near a ORL point in a lossy medium. It thus appears that, while the parameter α is immaterial, the CPA condition can in general be satisfied only if β can be tuned at will within the whole interval [0, π], regardless of the value of w. In fact, although
(with Re[η] > 0 due to losses). The last term in Eq. (2) holds when |eηL| ≫ 1 (|t| ≪ 1) and w ≪ 1, and this is precisely the regime we are interested in as it can occur near a ORL point in a lossy medium. It thus appears that, while the parameter α is immaterial, the CPA condition can in general be satisfied only if β can be tuned at will within the whole interval [0, π], regardless of the value of w. In fact, although  ,
,  need not be small at the CPA point as kL ≫ 1.
need not be small at the CPA point as kL ≫ 1.
Though solid-state photonic structures may be considered4, coherently-prepared multi-level atoms5,42 are attractive for exploring non-Hermitian optics, because of the easy reconfiguration of the scattering process through well established control techniques enabled by electromagnetically induced transparency (EIT)43. In fact, the realization of atomic platforms to investigate non-Hermitian models is currently a very active experimental endeavor44,45. We consider the realistic atomic system of Fig. 1, which provides an implementation of the model of Eq.(1). The photonic crystal consists of cold atoms coherently driven by a near-resonant probe beam (Ωp, Δp ≈ 0), a resonant coupling beam (Ωc, Δc = 0) and an far-detuned dressing field (Ωd,|Δd| ≫ 0). The latter has both a traveling-wave (TW) and a standing-wave (SW) components with opposite detunings and induces on level |2〉 a dynamic shift  , where
, where  and the phase shift 2ϕd is relative to the optical lattice modulating the atomic density. As a matter of fact, by adjusting only three of the above independent control parameters, namely {Δp, δd0, ϕd}, it is possible to identify scattering processes for which the existence of the CPA–ORL connection can be proven. More specifically, this is done by solving the density matrix equations for the atomic level configuration of Fig. 1 whose matrix elements will depend, among other parameters kept fixed here as in Fig. 6 of ref. 45, on the three parameters (Δp, δd0, ϕd) (See sect. II of ref. 45). For each choice of these three experimentally tunable parameters, we numerically compute the full susceptibility χ(z), which can be cast in the form of Eq. (1) when its higher order Fourier components are disregarded. From χ(z) we then directly obtain through transfer matrix calculations46 the scattering amplitudes t, rL and rR that identify a specific scattering process.
and the phase shift 2ϕd is relative to the optical lattice modulating the atomic density. As a matter of fact, by adjusting only three of the above independent control parameters, namely {Δp, δd0, ϕd}, it is possible to identify scattering processes for which the existence of the CPA–ORL connection can be proven. More specifically, this is done by solving the density matrix equations for the atomic level configuration of Fig. 1 whose matrix elements will depend, among other parameters kept fixed here as in Fig. 6 of ref. 45, on the three parameters (Δp, δd0, ϕd) (See sect. II of ref. 45). For each choice of these three experimentally tunable parameters, we numerically compute the full susceptibility χ(z), which can be cast in the form of Eq. (1) when its higher order Fourier components are disregarded. From χ(z) we then directly obtain through transfer matrix calculations46 the scattering amplitudes t, rL and rR that identify a specific scattering process.
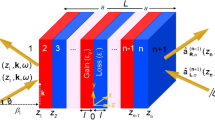
The CPA – ORL connection scattering scheme.
(a) Cold 87Rb atoms are loaded in a 1D optical lattice (black-solid) of period a. These atoms suffer a dynamic level shift (red-dashed) with the same periodicity, but phase shifted with respect to the optical lattice. The incident probe electric field amplitudes ( ) are scattered by the atomic lattice into the outgoing electric field amplitudes (
) are scattered by the atomic lattice into the outgoing electric field amplitudes ( ). For fields (
). For fields ( ) incident from the right, e.g., outgoing amplitudes consist of waves (
) incident from the right, e.g., outgoing amplitudes consist of waves ( ) transmitted with amplitude tR in the −z direction as well as waves (
) transmitted with amplitude tR in the −z direction as well as waves ( ) reflected with amplitude rR in the +z direction; likewise for fields (
) reflected with amplitude rR in the +z direction; likewise for fields ( ) incident from the left and reflected (transmitted) with amplitude rL (tL); while in general rL ≠ rR, tL = tR = t. (b) A four-level N-configuration through which 87Rb atoms are driven by a weak near-resonant probe field (green) on the
) incident from the left and reflected (transmitted) with amplitude rL (tL); while in general rL ≠ rR, tL = tR = t. (b) A four-level N-configuration through which 87Rb atoms are driven by a weak near-resonant probe field (green) on the  transition, a moderate resonant coupling field (blue) on the
transition, a moderate resonant coupling field (blue) on the  transition and a strong far-detuned dressing field (red) on the
transition and a strong far-detuned dressing field (red) on the  transition. (c) The probe, with Rabi frequency Ωp and detuning Δp, and the resonant coupling (Δc = 0), with Rabi frequency Ωc, propagate in the z direction. The dressing field has instead a TW component propagating in the x direction, with Rabi frequency Ωd and detuning −Δd, and a SW component modulated in the z direction, with detuning +Δd.
transition. (c) The probe, with Rabi frequency Ωp and detuning Δp, and the resonant coupling (Δc = 0), with Rabi frequency Ωc, propagate in the z direction. The dressing field has instead a TW component propagating in the x direction, with Rabi frequency Ωd and detuning −Δd, and a SW component modulated in the z direction, with detuning +Δd.
Plots of ρ (a) and θ (b) along the directions marked by the two color-dashed arrows in Fig. 3(a) for {ϕd = 0.15 × π, Δp = 0.576δd0 − 0.220 MHz} (blue-dashed line) and for {ϕd = 0.25 × π, Δp = 0.0 MHz} (red-solid line). The two CPA-points ( ) and (
) and ( ) (circles) placed at δd0 = 3.025 MHz (ϕd = 0.15 × π) and at δd0 = 2.896 MHz (ϕd = 0.25 × π) correspond to those shown respectively in Fig. 5(a,b) (non-Hermitian) and Fig. 5(c,d) (pseudo-Hermitian). The two ORL-points (
) (circles) placed at δd0 = 3.025 MHz (ϕd = 0.15 × π) and at δd0 = 2.896 MHz (ϕd = 0.25 × π) correspond to those shown respectively in Fig. 5(a,b) (non-Hermitian) and Fig. 5(c,d) (pseudo-Hermitian). The two ORL-points ( ) and (
) and ( ) (squares) placed at δd0 = 2.748 MHz (ϕd = 0.15 × π) and at δd0 = 2.615 MHz (ϕd = 0.25 × π) correspond to those shown respectively in Fig. 5(a,b) (non-Hermitian) and Fig. 5(c,d) (pseudo-Hermitian). At the ORL-points the phase θ is not defined and changes by π, as shown by vertical dotted lines in panel (b). At the CPA-point (
) (squares) placed at δd0 = 2.748 MHz (ϕd = 0.15 × π) and at δd0 = 2.615 MHz (ϕd = 0.25 × π) correspond to those shown respectively in Fig. 5(a,b) (non-Hermitian) and Fig. 5(c,d) (pseudo-Hermitian). At the ORL-points the phase θ is not defined and changes by π, as shown by vertical dotted lines in panel (b). At the CPA-point ( ) the ratio of right to left incoming intensities is about 0.082 while at the CPA-point (
) the ratio of right to left incoming intensities is about 0.082 while at the CPA-point ( ) the ratio is 0.053.
) the ratio is 0.053.
A relevant sets of ORL points (rL = 0) and the associated CPA-points (t2 = rRrL) are reported in the 3D parameter space {Δp, δd0, ϕd} of Fig. 2. A CPA-line lying roughly parallel to an ORL-line is shown there. Hence, we can access a CPA-point starting from a ORL-point essentially by adjusting the parameter δd0. The reason is simply that (i) the transmission amplitude t is always small in our lossy atomic medium and (ii) the reflection amplitudes rL and rR are more sensitive to δd0 than Δp at a fixed value of ϕd. A range of ϕd values centered at ϕd = π/4 is shown, being our system periodic in ϕd with period π, while varying ϕd from ϕd = π/4 to 3π/4 (or to −π/4) simply changes the reflectionless behavior from the “left” into reflectionless from the “right”. Notice also that the CPA-lines and ORL-lines are symmetric under the simultaneous changes ϕd → π/2−ϕd and Δp → −Δp. We can always find an isolated CPA-point associated to a nearby isolated ORL-point through cuts along {Δp, δd0}-planes as shown in Fig. 3. Figure 4 illustrates further examples of how ORL-points and the associated CPA-points are computed. ORL-points are characterized by rL = 0 and are here obtained by solving the two real equations Re[rL] = 0 and Im[rL] = 0. In the neighborhood of a solution both Re[rL] and Im[rL] change sign and their product changes sign in four alternating sections (i.e., deformed quadrants) of the {Δp, δd0}-plane as shown in Fig. 4(a–c,e–g). This corresponds to the fact that the phase of rL varies by 2π when a ORL-point is encircled in the {Δp, δd0}-plane, which embodies the freedom of choice of β in Eq. (2), and is a key point as discussed below. CPA-points, characterized by t2 = rLrR, are illustrated instead in Fig. 4(b–d,f–h) as minima of the function |t2 − rLrR|.
A CPA-line and the nearby ORL-line for a typical photonic crystal structure are shown in the parameter space {Δp, δd0, ϕd}.
The two lines which are nearly “parallel” are also shown projected onto the {δd0, ϕd} plane (blue lines), the {Δp, ϕd} plane (green lines) and the {Δp, δd0} plane (red lines). The points labeled ( ), (
), ( ), (
), ( ) and (
) and ( ) correspond to those marked in Fig. 3.
) correspond to those marked in Fig. 3.
Pairs of ORL (+) and CPA (*) points in the {Δ p, δd0} plane (yellow plane in Fig. 2) corresponding to values of ϕd ranging from 0.15 × π to 0.30 × π (top to bottom).
a–c,e–g (left column)] Contour plots of Re[rL] × Im[rL]: ORL-points (white-dots) occur when both Re[rL] and Im[rL] change sign in the {Δp, δd0}-plane. [b–d,f–h (right column)] Corresponding CPA-points (white-dots) occur when |t2 − rLrR| vanishes. Each pair of ORL-CPA points is found for a given value of ϕd (from top to bottom: ϕd/π = 0.20, 0.25, 0.30, 0.35).
Discussion
The CPA – ORL connection can also be assessed in more general terms starting from the two-ports scattering process,

where the S matrix relates the outgoing (electric) field amplitudes  and
and  to the incoming (electric) field amplitudes
to the incoming (electric) field amplitudes  and
and  (see Fig. 1a). The eigenvalues and eigenvectors of S are obtained through the last term in Eq. (3). It is here worth noting that we have chosen one of the most common representations of the S matrix, the other one having instead rL and rR on the diagonal. While the scattering is solely determined by the measurable complex amplitudes t, rL and rR and all physical results are independent of which S matrix representation is used, the specific choice of S in Eq. (3) is appropriate to prove the CPA – ORL connection, where the ORL condition is in this case directly related to a non-Hermitian degeneracy (or exceptional point) of S, as we illustrate in the following.
(see Fig. 1a). The eigenvalues and eigenvectors of S are obtained through the last term in Eq. (3). It is here worth noting that we have chosen one of the most common representations of the S matrix, the other one having instead rL and rR on the diagonal. While the scattering is solely determined by the measurable complex amplitudes t, rL and rR and all physical results are independent of which S matrix representation is used, the specific choice of S in Eq. (3) is appropriate to prove the CPA – ORL connection, where the ORL condition is in this case directly related to a non-Hermitian degeneracy (or exceptional point) of S, as we illustrate in the following.
In general, S is non-Hermitian, its eigenvalues

are complex and the (unnormalized) eigenvectors  are not orthogonal. Non-Hermitian degeneracies of S occur when the eigenvalues merge into one another [Fig. 5(a–d)] and the eigenvectors coalesce into a single state7, being the S matrix no longer diagonalizable. The two coalescing eigenvalues are analytically connected by a square-root branch-point, with associated Riemann sheets, and are physically associated with unidirectional reflectionless scattering states occurring when rL = 0 (or rR = 0)4,5. For a non-Hermitian matrix, degeneracies are of codimension two, that is points in a two-parameter space (NHD-point) and curves in a three-parameter space (NHD-line). Meanwhile, CPA occurs when either one of the two eigenvalues
are not orthogonal. Non-Hermitian degeneracies of S occur when the eigenvalues merge into one another [Fig. 5(a–d)] and the eigenvectors coalesce into a single state7, being the S matrix no longer diagonalizable. The two coalescing eigenvalues are analytically connected by a square-root branch-point, with associated Riemann sheets, and are physically associated with unidirectional reflectionless scattering states occurring when rL = 0 (or rR = 0)4,5. For a non-Hermitian matrix, degeneracies are of codimension two, that is points in a two-parameter space (NHD-point) and curves in a three-parameter space (NHD-line). Meanwhile, CPA occurs when either one of the two eigenvalues  or
or  vanishes [Fig. 5(a–d)] along with the determinant of S (this condition is independent of the specific choice of S matrix representation). The corresponding eigenvector describes a perfect absorption state10 with amplitudes and phases of the incoming fields from the left and from the right precisely chosen so that no outgoing light intensity can be observed13,18.
vanishes [Fig. 5(a–d)] along with the determinant of S (this condition is independent of the specific choice of S matrix representation). The corresponding eigenvector describes a perfect absorption state10 with amplitudes and phases of the incoming fields from the left and from the right precisely chosen so that no outgoing light intensity can be observed13,18.
Coherent Perfect Absorption (CPA) and Non-Hermitian Degeneracies (NHD).
Typical topology of the S-matrix eigenvalues (4) around a NHD (circle) for non-Hermitian (a,b) and pseudo-Hermitian (c,d) scattering processes in the photonic crystal structure of Fig. 1. Vertical green line indicate CPA-points next to a NHD-point respectively at (a,b) δd0 = 3.02 MHz (with Δp = 1.52 MHz, point ( ) in Fig. 3) and δd0 = 2.75 MHz (with Δp = 1.36 MHz, point (
) in Fig. 3) and δd0 = 2.75 MHz (with Δp = 1.36 MHz, point ( ) in Fig. 3) and at (c,d) δd0 = 2.89 MHz (with Δp = 0, point (
) in Fig. 3) and at (c,d) δd0 = 2.89 MHz (with Δp = 0, point ( ) in Fig. 3) and δd0 = 2.61 MHz (with Δp = 0, point (
) in Fig. 3) and δd0 = 2.61 MHz (with Δp = 0, point ( ) in Fig. 3). Polar representation of the two eigenvalues before (e) and at (f ) the NHD-point, and at (g) and after (h) the CPA-point for the case (c,d) (with Δp = 0). Light green and violet arrows mark respectively the two eigenvalues half-sum (t) and half-difference (
) in Fig. 3). Polar representation of the two eigenvalues before (e) and at (f ) the NHD-point, and at (g) and after (h) the CPA-point for the case (c,d) (with Δp = 0). Light green and violet arrows mark respectively the two eigenvalues half-sum (t) and half-difference ( ).
).
We start providing an intuitive illustration of how CPA and ORL are connected with one another in the particular, but important, case for which (i) the reflection phases are such that ϕL + ϕR = {0, π} and (ii) the transmission amplitude t is real. The corresponding eigenvalues are either real or complex conjugate in pairs depending on whether the two phases add up to 0 or to π [Fig. 5(c,d)]. Thus (half) sum of the two eigenvalues represents t and can be depicted, as we move in the parameter space toward degeneracy, by a vector whose magnitude decreases along the real axis of Fig. 5(e) for decreasing transmission. So does (half) difference of the two eigenvalues representing the geometric mean of rL and rR, which can be depicted by a vector parallel to the imaginary axis. As we move through degeneracy, the eigenvalues sum will keep decreasing but their difference will increase after moving away from zero (degeneracy) [Fig. 5(f)] owing to the intrinsic bifurcation (topological) structure of the branch-point. Hence there will always be a point where sum and difference will be equal (to each other), i.e.,  [Fig. 5(g)]. It is worth noting that under the conditions (i.) and (ii.) an Hermitian invertible transformation η exists indeed for which the adjoint of the (non-Hermitian) scattering matrix S satisfies S† = ηSη−1, i.e., S is pseudo-Hermitian48. The reverse is also true and hence the pseudo-Hermiticity of S is the basic mathematical structure responsible for the direct connection between the ORL and the CPA point, at least for the specific spectrum of S shown in Fig. 5(c,d). Note that this particular case – realized in the all-optically tunable atomic system of Fig. 1 simply setting Δp = 0 – is essentially analogous to a PT symmetric one, even though our system is always lossy, both before and after the NHD point.
[Fig. 5(g)]. It is worth noting that under the conditions (i.) and (ii.) an Hermitian invertible transformation η exists indeed for which the adjoint of the (non-Hermitian) scattering matrix S satisfies S† = ηSη−1, i.e., S is pseudo-Hermitian48. The reverse is also true and hence the pseudo-Hermiticity of S is the basic mathematical structure responsible for the direct connection between the ORL and the CPA point, at least for the specific spectrum of S shown in Fig. 5(c,d). Note that this particular case – realized in the all-optically tunable atomic system of Fig. 1 simply setting Δp = 0 – is essentially analogous to a PT symmetric one, even though our system is always lossy, both before and after the NHD point.
Yet, a CPA-point can be typically found in the vicinity of a ORL-point under more general conditions and, in particular, without restricting ourselves to pseudo-Hermiticity. For definiteness we take the NHD-point at  assuming, without loss of generality, that around this point |rR| and |t| are nonvanishing. For lossy media we may further take |t| ≪ 1, with |rR| being in general on the order of unity5. The perfect absorption condition
assuming, without loss of generality, that around this point |rR| and |t| are nonvanishing. For lossy media we may further take |t| ≪ 1, with |rR| being in general on the order of unity5. The perfect absorption condition  is satisfied when rLrR = t2, i.e. when
is satisfied when rLrR = t2, i.e. when

are both satisfied, implying that |rL| and arg(rL) should be independently adjusted (just as the phase β in Eq. (2) should be tuned at will, regardless of the value of w). Note that the CPA conditions in Eq. (5) generalize those given above for the pseudo-Hermitian case, and are only restricted by the requirement that |rL| be small at the CPA-point, which occurs when this point is associated to a nearby ORL-point. In general, we do expect t2/rR to be smoothly varying in the vicinity of this point while arg(rL) can be varied at will when the parameters defining the system are smoothly changed so to encircle the ORL-point, i.e. the NHD of S5. A simple geometric illustration of this property similar to that provided in Fig. 5(e–h) is not so viable in the general, non pseudo-Hermitian case (such as that of Fig. 5(a,b)); yet, a direct analytical argument shows that |rL| and arg(rL) can be independently adjusted when encircling the ORL-point.
In a typical scattering process, rL depends smoothly on several experimental parameters. We consider here how the real (u) and the imaginary (v) parts of rL vary near the ORL-point as a function of only two of these parameters, keeping all other ones fixed. In terms of these two parameters, say x and y, one has

where the partial derivatives ux = ∂u/∂x, uy = ∂u/∂y, vx = ∂v/∂x, and vy = ∂v/∂y are evaluated at the ORL-point taken at (x, y) = (0, 0). Note that it is not needed to combine x and y into a single complex parameter x + iy as rL is not assumed to be holomorphic here. When uxvy − vxuy ≠ 0, it is always possible to select x and y to obtain any required values of arg(rL) and of |rL|, provided the latter is small enough that higher order terms in Eq. (6) are indeed negligible. Thus, under typical circumstances we expect a CPA and a ORL points to be close to each other in a scattering process from lossy media with |t| small. For example, in Fig. 3 the case ϕd = 0.25 × π (pink-arrow) represents changes in the scattering matrix as one moves from its NHD-point ( ) to its CPA-companion (
) to its CPA-companion ( ), namely for a pseudo-Hermitian matrix (Δp = 0). Similarly, the case ϕd = 0.15 × π (blue-arrow) represents changes as one moves from the NHD-point (
), namely for a pseudo-Hermitian matrix (Δp = 0). Similarly, the case ϕd = 0.15 × π (blue-arrow) represents changes as one moves from the NHD-point ( ) to its CPA-companion (
) to its CPA-companion ( ), namely for the general non-Hermitian case. Actually, the case in which uxvy − vxuy = 0 cannot be excluded. Assuming that (uy, vy) ≠ (0, 0) and writing (ux, vx) = μ(uy, vy) with μ real, one then has
), namely for the general non-Hermitian case. Actually, the case in which uxvy − vxuy = 0 cannot be excluded. Assuming that (uy, vy) ≠ (0, 0) and writing (ux, vx) = μ(uy, vy) with μ real, one then has

which implies that, while |rL| = |ΔrL| can be varied, arg(rL) = arg(ΔrL) is fixed because  . In this case, we expect to find no CPA-point in the vicinity of a ORL-point when all other parameters are kept constant. Clearly, also when higher order terms in the above expansion of rL become important as for instance in the peculiar case where all partial derivatives in Eq. (6) are vanishingly small, the occurrence of the CPA point is not granted.
. In this case, we expect to find no CPA-point in the vicinity of a ORL-point when all other parameters are kept constant. Clearly, also when higher order terms in the above expansion of rL become important as for instance in the peculiar case where all partial derivatives in Eq. (6) are vanishingly small, the occurrence of the CPA point is not granted.
Defining ρeiθ ≡ −rL/t, the scattering matrix eigenvector at the CPA-point, where the corresponding eigenvalue  vanishes, can be eventually written as,
vanishes, can be eventually written as,

The complex quantity ρeiθ is examined in Fig. 6 both for the pseudo-Hermitian and non-Hermitian cases. At the CPA-point, the eigenvector’s components scale as  , with ρ ≪ 1 according to Eq. (5). Both modulus (ρ) and phase (θ) of the (small) incoming field from the right, with respect to the incoming field from the left (i.e, the nearly reflectionless side), should be properly chosen to observe the typical perfect absorption behavior. Since the CPA-point considered here is associated to a ORL point, in general, perfect absorption requires very unbalanced incoming fields. As a matter of fact, the characteristic destructive interference conditions leading to perfect absorption for light scattering in both directions occur here for very unbalanced right and left reflectivities |rR| ≫ |rL|. In turn, a tiny input field from the right is sufficient to ensure that the outgoing field to the left vanishes, while a large input field from the left is necessary to destructively interfere with the reflected field from the right side. This CPA configuration provides, in particular, a high-contrast reflectivity control of a test beam incident from the right via a pump beam incident from the left.
, with ρ ≪ 1 according to Eq. (5). Both modulus (ρ) and phase (θ) of the (small) incoming field from the right, with respect to the incoming field from the left (i.e, the nearly reflectionless side), should be properly chosen to observe the typical perfect absorption behavior. Since the CPA-point considered here is associated to a ORL point, in general, perfect absorption requires very unbalanced incoming fields. As a matter of fact, the characteristic destructive interference conditions leading to perfect absorption for light scattering in both directions occur here for very unbalanced right and left reflectivities |rR| ≫ |rL|. In turn, a tiny input field from the right is sufficient to ensure that the outgoing field to the left vanishes, while a large input field from the left is necessary to destructively interfere with the reflected field from the right side. This CPA configuration provides, in particular, a high-contrast reflectivity control of a test beam incident from the right via a pump beam incident from the left.
Conclusions
A new insight into the non-Hermitian optics of a familiar class of lossy photonic crystals is here discussed. Through continuous deformations of the scattering matrix S around a one-sided reflectionless (ORL) point, a CPA point can be typically attained. Nearby pairs of ORL and CPA “points” or even “lines” appear, respectively, through controlling the crystal 2D or 3D parameter space. In such cases, the CPA scattering states associated to ORL points turn out to be significantly unbalanced, indicating a dynamically reversible high-contrast reflectivity control of the input beams. Finally, while the results here presented refer to realistic atomic structures44,45, our general discussion can be easily adapted to atomic-like multilevel centers49 in solids, such as NV diamond or rare-earth-doped crystals, also allowing for EIT control of light scattering50,51. Hence the optics of photonic crystals is poised to have a privileged place in assessing that not only standard Hermitian models but also a broad set of non-Hermitian ones are bound to have physical interpretations.
Additional Information
How to cite this article: Wu, J.-H. et al. Coherent perfect absorption in one-sided reflectionless media. Sci. Rep. 6, 35356; doi: 10.1038/srep35356 (2016).
References
Bender, C. M. Making sense of non-Hermitian Hamiltonians. Rep. Prog. Phys. 70, 947–1018 (2007).
Lin, Z. et al. Unidirectional invisibility induced by PT-symmetric periodic structures. Phys. Rev. Lett. 106, 213901 (2011).
Regensburger, A. et al. Parity-time synthetic photonic lattices. Nature 488, 167–171 (2012).
Feng, L. et al. Experimental demonstration of a unidirectional reflectionless parity-time metamaterial at optical frequencies. Nat. Mater. 12, 108–113 (2012).
Wu, J.-H., Artoni, M. & La Rocca, G. C. Non-Hermitian degeneracies and unidirectional reflectionless atomic lattices. Phys. Rev. Lett. 113, 123004 (2014).
Horsley, S. A. R., Artoni, M. & La Rocca, G. C. Spatial Kramers-Kronig relations and the reflection of waves. Nat. Photon. 9, 436–439 (2015).
Berry, M. V. Physics of non-Hermitian degeneracies. Czech. J. Phys. 54, 1039–1047 (2004).
Heiss, W. D. The physics of exceptional points. J. Phys. A: Math. Theor. 45, 444016 (2012).
Fang, X., MacDonald, K. F. & Zheludev, N. I. Controlling light with light using coherent metadevices: all-optical transistor, summator and invertor. Light: Sci. Appl. 4, e292 (2015).
Chong, Y.-D., Ge, L., Cao, H. & Stone, A. D. Coherent perfect absorbers: time-reversed laser. Phys. Rev. Lett. 105, 053901 (2010).
Longhi, S. PT-symmetric laser absorber. Phys. Rev. A 82, 031801(R) (2010).
Chong, Y.-D., Ge, L. & Stone, A. D. PT-Symmetry breaking and laser-absorber modes in optical scattering systems. Phys. Rev. Lett. 106, 093902 (2011).
Wan, W. et al. Time-reversed lasing and interferometric control of absorption. Science 331, 889–892 (2011).
Dutta-Gupta, S., Martin, O. J. F., Dutta Gupta, S. & Agarwal, G. S. Controllable coherent perfect absorption in a composite film. Opt. Express 20, 1330–1336 (2012).
Zhang, J., MacDonald, K. F. & Zheludev, N. I. Controlling light-with-light without nonlinearity. Light: Sci. Appl. 1, e18 (2012).
Fang, X. et al. Ultrafast all-optical switching via coherent modulation of metamaterial absorption. Appl. Phys. Lett. 104, 141102 (2014).
Yan, X.-B. et al. Coherent perfect absorption, transmission, and synthesis in a double-cavity optomechanical system. Opt. Express 22, 4886–4895 (2014).
Zanotto, S. & Tredicucci, A. Universal lineshapes at the crossover between weak and strong critical coupling in Fano-resonant coupled oscillators. Sci. Rep. 6, 24592 (2016).
Bender, C. M. & Boettcher, S. Real spectra in non-Hermitian Hamiltonians having PT symmetry. Phys. Rev. Lett. 80, 5243–5246 (1998).
Chong, Y.-D. & Stone, A. D. Hidden black: Coherent enhancement of absorption in strongly scattering media. Phys. Rev. Lett. 107, 163901 (2011).
Noh, H., Chong, Y.-D., Stone, A. D. & Cao, H. Perfect coupling of light to surface plasmons by coherent absorption. Phys. Rev. Lett. 108, 186805 (2012).
Zanotto, S. et al. Perfect energy-feeding into strongly coupled systems and interferometric control of polariton absorption. Nat. Phys. 10, 830–834 (2014).
Gutman, N., Sukhorukov, A. A., Chong, Y.-D. & Martijn de Sterke, C. Coherent perfect absorption and reflection in slow-light waveguides. Opt. Lett. 38, 4970–4973 (2013).
Grande, M. et al. Graphene-based absorber exploiting guided mode resonances in one-dimensional gratings. Opt. Express 22, 31511–31519 (2014).
Fagotti, M., Bonati, C., Logoteta, D., Marconcini, P. & Macucci, M. Armchair graphene nanoribbons: PT-symmetry breaking and exceptional points without dissipation. Phys. Rev. B 83, 241406(R) (2011).
Fan, Y.-C. et al. Tunable mid-infrared coherent perfect absorption in a graphene meta-surface. Sci. Rep. 5, 13956 (2015).
Zhang, J.-F. et al. Coherent perfect absorption and transparency in a nanostructured graphene film. Opt. Express 22, 12524–12532 (2014).
Kang, M., Chong, Y.-D., Wang, H.-T., Zhu, W.-R. & Premaratne, M. Critical route for coherent perfect absorption in a Fano resonance plasmonic system. Appl. Phys. Lett. 105, 131103 (2014).
Ge, L., Chong, Y.-D. & Stone, A. D. Conservation relations and anisotropic transmission resonances in one-dimensional PT-symmetric photonic heterostructures. Phys. Rev. A 85, 023802 (2012).
Ambichl, P. et al. Breaking of PT symmetry in bounded and unbounded scattering systems. Phys. Rev. X 3, 041030 (2013).
Sun, Y., Tan, W., Li, H.-Q., Li, J.-S. & Chen, H. Experimental demonstration of a coherent perfect absorber with PT phase transition. Phys. Rev. Lett. 112, 143903 (2014).
Ahmed, Z. Coherent perfect absorption with and without lasing in complex potentials. J. Phys. A: Math. Theor 47, 385303 (2014).
Garmon, S., Gianfreda, M. & Hatano, N. Bound states, scattering states, and resonant states in PT-symmetric open quantum systems. Phys. Rev. A 92, 022125 (2015).
Zhu, B.-G., Lu, R. & Chen, S. PT-symmetry breaking for the scattering problem in a one-dimensional non-Hermitian lattice model. Phys. Rev. A 93, 032129 (2016).
Fleury, R., Sounas, D. L., Sieck, C. F., Haberman, M. R. & Alu, A. Sound isolation and giant linear nonreciprocity in a compact acoustic circulator. Science 343, 516–519 (2014).
Fleury, R., Sounas, D. & Alu, A. An invisible acoustic sensor based on parity-time symmetry. Nat. Commun. 6, 5905 (2014).
Zhu, X.-F., Ramezani, H., Shi, C.-Z., Zhu, J. & Zhang, X. PT-symmetric acoustics. Phys. Rev. X 4, 031042 (2014).
Duan, Y.-T. et al. Theoretical requirements for broadband perfect absorption of acoustic waves by ultra-thin elastic meta-films. Sci. Rep. 5, 12139 (2015).
Shi, C. et al. Accessing the exceptional points of parity-time symmetric acoustics. Nat. Commun. 7, 11110 (2016).
Cummer, S. A., Christensen, J. & Alu, A. Controlling sound with acoustic metamaterials. Nat. Rev. Mater. 1, 16001 (2016).
Christensen, J., Willatzen, M., Velasco, V. R. & Lu, M.-H. Parity-time synthetic phononic media. Phys. Rev. Lett. 116, 207601 (2016).
Horsley, S. A. R., Wu, J.-H., Artoni, M. & La Rocca, G. C. Optical nonreciprocity of cold atom bragg mirrors in motion. Phys. Rev. Lett. 110, 223602 (2013).
Fleischhauer, M., Imamoglu, A. & Marangos, J. P. Electromagnetically induced transparency: Optics in coherent media. Rev. Mod. Phys. 77, 633–673 (2005).
Peng, P. et al. Anti-parity-time symmetric optics via flying atoms, doi: 10.1038/NPHYS3842.
Zhang, Z.-Y. et al. Observation of parity-time symmetry in optically induced atomic lattices. Phys. Rev. Lett. 117, 123601 (2016).
Wu, J.-H., Artoni, M. & La Rocca, G. C. Parity-time-antisymmetric atomic lattices without gain. Phys. Rev. A 91, 033811 (2015).
Artoni, M., La Rocca, G. C. & Bassani, F. Resonantly absorbing one-dimensional photonic crystals. Phys. Rev. E 72, 046604 (2005).
Mostafazadeh, A. Pseudo-Hermitian representation of quantum mechanics. Int. J. Geom. Methods Mod. Phys. 07, 1191–1306 (2010).
Childress, L., Walsworth, R. & Lukin, M. D. Atom-like crystal defects: From quantum computers to biological sensors. Phys. Today 67(10), 38 (2014).
He, Q.-Y. et al. Coherently induced stop-bands in resonantly absorbing and homogeneously broadened doped crystals. Phys. Rev. B 73, 195124 (2006).
Wu, J.-H., La Rocca, G. C. & Artoni, M. Controlled light-pulse propagation in driven color centers in diamond. Phys. Rev. B 77, 113106 (2008).
Acknowledgements
Insightful discussions with S. Horsley and M. Berry at the early stage of the work are kindly acknowledged. J.H.W is grateful for the hospitality at Scuola Normale Superiore in Pisa and the support from National Natural Science Foundation of China (No. 61378094, 11534002, and 11674049).
Author information
Authors and Affiliations
Contributions
J.-H.W. developed theoretical frameworks and implemented numerical calculations. M.A. and G.C.L.R. conceived the mechanism, analyzed the results, and wrote the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Wu, JH., Artoni, M. & La Rocca, G. Coherent perfect absorption in one-sided reflectionless media. Sci Rep 6, 35356 (2016). https://doi.org/10.1038/srep35356
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep35356
This article is cited by
-
Coherent perfect absorbers: linear control of light with light
Nature Reviews Materials (2017)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.