Abstract
The fundamental parameters of the superconducting state such as coherence length and pairing strength are essential for understanding the nature of superconductivity. These parameters can be estimated by measuring critical parameters such as upper critical field, Hc2. In this work, Hc2 of a superconducting (110) LaAlO3/SrTiO3 interface is determined through magnetoresistive measurements as a function of the gate voltage, VG. When VG increases, the critical temperature has a dome-like shape, while Hc2 monotonically decreases. This relationship of independence between the variation of Tc and of Hc2 suggests that the Cooper pairing potential is stronger in the underdoped region and the coherence length increases with the increase of VG. The result is as for high temperature superconducting cuprates and it is different than for conventional low temperature superconductors.
Similar content being viewed by others
Introduction
Oxide heterostructures have attracted much attention in recent years due to a plethora of novel or enhanced physical phenomena observed at interfaces1,2. For example, the two dimensional electron gas (2DEG) formed at the interface between two perovskite insulators, LaAlO3 (LAO) and SrTiO3 (STO), becomes superconducting3 at around 200 mK. This discovery triggered intense investigations of the superconductivity mechanism4,5,6,7,8 at interfaces. A number of intriguing properties emerge at the superconducting interface. Literature indicate on a pseudogap-like behavior9, on the electron pre-formed pairing10 and on the coexistence of superconductivity and ferromagnetism11,12,13. The phase diagram for an interface is similar to that of high temperature superconducting (HTS) cuprates7,9. The interface exhibits superconductivity for an extremely low carrier density14,15. Presented aspects may indicate on unconventional pairing mechanism. Nevertheless, the conventional electron-phonon coupling has been also considered5,16. Therefore, the microscopic mechanism responsible for interface superconductivity is still unclear and more research is of high interest.
Recently, it was reported that an energy gap persists above the superconducting dome defined as the variation of the critical temperature, Tc, vs. carrier doping. Furthermore, the energy gap increases monotonically as the gate voltage VG decreases. This correlation indicates on a pseudogap-like behavior of (001) LAO/STO interfaces9 and it is opposite to the VG dependence of the superfluid density14. For additional information about electron pairing in interfaces, it is useful to determine fundamental superconducting parameters such as the coherence length, ξ0 and the strength of pairing potential. These parameters can be extracted from upper critical field, Hc2; a higher Hc2 means a smaller coherence length and a stronger pairing potential17. Previous investigations of Hc2 have shown that superconductivity observed for (001) or (110) LAO/STO interfaces is of two dimensional nature3,18,19,20, but the VG dependence of Hc2 that is associated to pairing strength while being distinct from the superfluid density, has not been reported. Noteworthy is also that most studies are focused on (001) LAO/STO, while the (110) system is less explored although its study can bring an important contribution to understanding of superconductivity at interfaces20,21,22.
In this work we report the dependence of Hc2 on VG in a superconducting 2DEG (110) LAO/STO interface. We found that Hc2 decreases when VG increases. At the same time, the Tc(VG) curve shows a dome-like shape (with a maximum). Our results indicate that the Cooper pairing potential becomes stronger in the underdoped regime.
Results and Discussions
Critical temperature, critical current and normal state resistance as a function of gate voltage
In Fig. 1 are presented as examples, curves of (interface or sheet) resistance, Rs, versus temperature for three different gate voltages (−5 V, 0 V and 25 V) under zero magnetic field. Temperature for which Rs drops below the detection limit of the measuring equipment defines the critical temperature,  (Fig. 1). The curve of
(Fig. 1). The curve of  as a function of VG (in zero magnetic field) has a dome-like shape with a maximum at 25 V (Fig. 1 inset). The dome-like shape of the
as a function of VG (in zero magnetic field) has a dome-like shape with a maximum at 25 V (Fig. 1 inset). The dome-like shape of the  (VG) curves was previously observed in LAO/STO and (001) LaTiO3/SrTiO3 (LTO/STO) interfaces7,20,23. A dome-like shape with a maximum at VG = 25 V is also obtained for the variation of the critical current Ic(VG). The critical current, Ic, was determined at 50 mK from I-V curves measurements (see Supplementary Information, Fig. S1). The
(VG) curves was previously observed in LAO/STO and (001) LaTiO3/SrTiO3 (LTO/STO) interfaces7,20,23. A dome-like shape with a maximum at VG = 25 V is also obtained for the variation of the critical current Ic(VG). The critical current, Ic, was determined at 50 mK from I-V curves measurements (see Supplementary Information, Fig. S1). The  (VG) and Ic(VG) similar dome-like dependencies indicate a strong influence of the carrier density on
(VG) and Ic(VG) similar dome-like dependencies indicate a strong influence of the carrier density on  and Ic.
and Ic.
The resistance Rs of the (110) LAO/STO interface as a function of temperature at three representative gate voltages and for B = 0 T.
The inset shows the gate voltage VG dependence of the critical temperature  and of the normal state resistance Rn. The maximum of the
and of the normal state resistance Rn. The maximum of the  (VG) curve is at 138 mK and at 25 V. Lines are guide to the eyes.
(VG) curve is at 138 mK and at 25 V. Lines are guide to the eyes.
From the measurements of Rs with magnetic field (up to 1.6 T) applied perpendicular to the surface of the interface at a fixed temperature and gate voltage one can determine the normal state resistance, Rn. An example of Rs(B) curves at 50 mK and for VG from −25 to +200 V is presented in Fig. 2a. Inset to Fig. 2a shows for selected (T, VG) values how Rn is determined. Namely, Rn is the resistance of the cross point between the fitting lines of the steepest part of the Rs(B) experimental curve and of the region where Rs almost saturates. For a fixed temperature (50 mK), one Rn(VG) curve is plotted in Fig. 1 inset. Enhancement of VG decreases Rn. Curve is non-linear and the decrease rate is smaller for higher VG.
(a) Curves of Rs(B) for −5 ≤ VG ≤ 200 V at 50 mK. The inset in the right corner shows determination of Rn,  and
and  . The inset in the left corner shows
. The inset in the left corner shows  (VG) and
(VG) and  (VG) curves at 50 mK; (b) Curves of dRs/dB vs. B at 50 mK. Arrows indicate points of maximum of these curves and dashed line is guide for eyes. Magnetic field of a maximum point is defined as
(VG) curves at 50 mK; (b) Curves of dRs/dB vs. B at 50 mK. Arrows indicate points of maximum of these curves and dashed line is guide for eyes. Magnetic field of a maximum point is defined as  . The inset shows variation of
. The inset shows variation of  as a function of VG.
as a function of VG.
It is remarkable that VG can tune superconducting and normal state characteristics such as  and Rn, respectively. Results suggest that VG influences 2DEG superconductivity features and the carrier density. Our results are consistent with literature20.
and Rn, respectively. Results suggest that VG influences 2DEG superconductivity features and the carrier density. Our results are consistent with literature20.
Upper critical field as a function of gate voltage
For a fixed temperature and VG, the upper critical field Hc2 is determined as the field where resistance is 10%, 50% of Rn in the Rs(B) curves (Fig. 2a inset, right corner). This methodology was used to determine Hc2 for different superconducting systems24,25,26. We note that magnetic field B is applied in this work only perpendicular to the interface surface. Reported articles19,20 indicate that Hc2 shows large anisotropy when B is applied in-plane and out-of-plane. At 50 mK, the  (VG) or
(VG) or  (VG) (Fig. 2 inset, left corner) curves are unexpectedly without a dome-like shape that is specific for the
(VG) (Fig. 2 inset, left corner) curves are unexpectedly without a dome-like shape that is specific for the  (VG) and Ic(VG) (Figs 1 and S1) curves. Namely, for a lower VG, Hc2 monotonically increases (Fig. 2 inset, left corner). At the same time, a decrease of VG below VG, max = 25 V counter-intuitively produces the decrease of both
(VG) and Ic(VG) (Figs 1 and S1) curves. Namely, for a lower VG, Hc2 monotonically increases (Fig. 2 inset, left corner). At the same time, a decrease of VG below VG, max = 25 V counter-intuitively produces the decrease of both  and Ic (Figs 1 and S1).
and Ic (Figs 1 and S1).
The differential curves dRs/dB as a function of B, obtained from Rs(B) data (Fig. 2a) for different VG and at 50 mK, are presented in Fig. 2b. Each curve displays a peak (marked with an arrow in Fig. 2b). The peak shifts to higher B and its intensity increases when VG decreases. The magnetic field of the peak, denoted  , as a function of VG (at 50 mK) is shown in Fig. 2b, inset. A dome-like shape is not obtained and, as expected, this curve has a similar behavior as
, as a function of VG (at 50 mK) is shown in Fig. 2b, inset. A dome-like shape is not obtained and, as expected, this curve has a similar behavior as  (VG) or
(VG) or  . (VG) curves. Furthermore, the values of
. (VG) curves. Furthermore, the values of  and of
and of  are close to each other, but we shall keep both parameters in the discussion because of their different background: Hc250% is arbitrary taken, while Hc2peak defines the inflexion point of the Rs(B) cur. The inflexion point may have a physical meaning and, hence, Hc2peak can be more sensitive to external factors than
are close to each other, but we shall keep both parameters in the discussion because of their different background: Hc250% is arbitrary taken, while Hc2peak defines the inflexion point of the Rs(B) cur. The inflexion point may have a physical meaning and, hence, Hc2peak can be more sensitive to external factors than  .
.
Figure 3 shows curves of  (T) for different VG from −5 V up to 200 V. For all gate voltages,
(T) for different VG from −5 V up to 200 V. For all gate voltages,  increases with temperature decrease. Curves of
increases with temperature decrease. Curves of  . (T) shift into the region of higher values of
. (T) shift into the region of higher values of  -T for decreasing VG. This enhancement occurs even for negative VG, where a lower VG induces a lower
-T for decreasing VG. This enhancement occurs even for negative VG, where a lower VG induces a lower  . Moreover, the slope (d
. Moreover, the slope (d /dT|Tc) of the
/dT|Tc) of the  (T) curve approaching
(T) curve approaching  (Tc) = 0 systematically increases when VG decreases. The overall observed tendency does not change, if we use in the analysis
(Tc) = 0 systematically increases when VG decreases. The overall observed tendency does not change, if we use in the analysis  or
or  instead of
instead of  (Fig. S2 a,b). According to Ginzburg-Landau (GL) theory, near Tc the upper critical field is a linear function of (Tc − T) and smoothly saturates when lowering the temperature. Our results suggest an anomalous unconventional behavior of the upper critical field vs. temperature and it is no longer appropriate to describe this dependence using the GL theory. The
(Fig. S2 a,b). According to Ginzburg-Landau (GL) theory, near Tc the upper critical field is a linear function of (Tc − T) and smoothly saturates when lowering the temperature. Our results suggest an anomalous unconventional behavior of the upper critical field vs. temperature and it is no longer appropriate to describe this dependence using the GL theory. The  (T = 0 K) values obtained by linear extrapolation of e data at low temperature are increasing with decreasing VG. The determination of Hc2 at T = 0 K lacks precision. However, if we estimate the relative Hc2-increase (δHc2) at a finite temperature (e.g. 60 mK) between the curves for VG = −5 V and VG = 0 V, a criterion closer to Rs = 0 shows a lower value (i.e. δ
(T = 0 K) values obtained by linear extrapolation of e data at low temperature are increasing with decreasing VG. The determination of Hc2 at T = 0 K lacks precision. However, if we estimate the relative Hc2-increase (δHc2) at a finite temperature (e.g. 60 mK) between the curves for VG = −5 V and VG = 0 V, a criterion closer to Rs = 0 shows a lower value (i.e. δ = 1.3%, while δ
= 1.3%, while δ = 5.5%). This finds its understanding in the following: The emergence of Rs = 0 is due to the global superconductivity. With increasing magnetic field, a finite resistance occurs, and, hence, global superconductivity disappears. However, local superconductivity still exists before the system recovers to normal state. Thus, it is reasonable that we observe a larger Hc2 for a more negative VG. This indicates that Cooper pair can persist up to a higher magnetic field than the upper critical field corresponding to global superconductivity.
= 5.5%). This finds its understanding in the following: The emergence of Rs = 0 is due to the global superconductivity. With increasing magnetic field, a finite resistance occurs, and, hence, global superconductivity disappears. However, local superconductivity still exists before the system recovers to normal state. Thus, it is reasonable that we observe a larger Hc2 for a more negative VG. This indicates that Cooper pair can persist up to a higher magnetic field than the upper critical field corresponding to global superconductivity.
Superconductor-insulator transition
The magnetic-field-induced superconductor-insulator transition(SIT)27 shows a characteristic fan-shaped pattern of Rs(B) isotherms crossing at one point. For example, in Fig. 4a the SIT cross point for VG = −5 V is at 810 mT. The Rs(T) curves extracted from Rs(B) show a plateau for 810 mT (Fig. 4b). The plateau separates two regimes. Therefore, the magnetic field drives a continuous quantum phase transition from a superconducting 2DEG to a weakly insulating state. Further finite-size scaling analysis shows that the data can be collapsed onto a bi-value curve (Fig. 4a, inset). It results that the crossing point is a quantum critical point (QCP), at which the phase transition occurs. Literature often show magnetic-field-induced SIT for different 2D superconductors27,28, including for interfaces such as LAO/STO and (001) LTO/STO29,30.
(a) SIM pattern: curves of Rs(B) at different temperatures for V= −5 V. Note the crossing point at 810 mT that defines the quantum critical point (QCP) with (Bc = 810 mT, Rc = 1297.54 Ω). The inset shows the bi-value curve obtained by collapse of the date by finite-size scaling analysis. (b) Curves of Rs(T) at different magnetic fields B and for VG = −5 V. Dashed line shows a plateau corresponding to QCP. Continuous lines are guide for the eyes.
As already noted, Hc2(T) for a fixed VG depends on the criterion adopted for the Hc2 determination in the case of a broad transition26,31 and the Hc2(T = 0 K) cannot be determined in a reliable manner. This situation questions the intrinsic nature of the Hc2 – enhancement (for a lower VG) in the underdoped region. Due to this, here we use another method to directly determine the zero - temperature upper critical field,  . This method is independent of the criterion applied to Rs(B) curves. At the QCP, taken as a transition point between superconducting and normal states of the 2DEG, the corresponding magnetic field is defined as
. This method is independent of the criterion applied to Rs(B) curves. At the QCP, taken as a transition point between superconducting and normal states of the 2DEG, the corresponding magnetic field is defined as  . The
. The  curve as a function of VG is plotted in Fig. 5. Although it is considered that this method does not give accurate values of
curve as a function of VG is plotted in Fig. 5. Although it is considered that this method does not give accurate values of  30, this barely affects our main results, the dependence on VG of
30, this barely affects our main results, the dependence on VG of  being similar to that of
being similar to that of  (T = 0 K) (Fig. 5). One reason for a non-precise determination of
(T = 0 K) (Fig. 5). One reason for a non-precise determination of  vs. VG is: when VG ≠ −5 V, e.g., a VG = 75 V is used for the construction of the magnetic-field-induced SIT pattern, the crossing point of the Rs(B) isotherms transforms into a field domain centered at 0.55 T and extending over ±0.05 T (Fig. S3a). In this case, the conventional power-law scaling behavior fails to describe the quantum criticality (Fig. S3b). Multiple quantum criticality was also found and reported for the (001) LTO/STO interface29. The phenomenon of multiple critical exponents suggests an unconventional critical behavior of SIT in the (110) LAO/STO interface and it will be discussed elsewhere.
vs. VG is: when VG ≠ −5 V, e.g., a VG = 75 V is used for the construction of the magnetic-field-induced SIT pattern, the crossing point of the Rs(B) isotherms transforms into a field domain centered at 0.55 T and extending over ±0.05 T (Fig. S3a). In this case, the conventional power-law scaling behavior fails to describe the quantum criticality (Fig. S3b). Multiple quantum criticality was also found and reported for the (001) LTO/STO interface29. The phenomenon of multiple critical exponents suggests an unconventional critical behavior of SIT in the (110) LAO/STO interface and it will be discussed elsewhere.
Phase diagram
The superconducting phase diagram of the (110) LAO/STO interface is presented in Fig. 5. As already addressed in the previous sections, the upper critical field Hc2 independently of the criterion for its determination monotonically increases when VG decreases, while  displays a dome-like curve with a maximum. In the inset to Fig. 5 is shown the GL coherence length ξ0 determined from Hc2 as a function of VG. The most striking anomalous result is enhancement of Hc2 accompanied by the decrease of ξ0 for decreasing VG, i.e. for the carrier depletion reflected by the dome-like curve in the underdoped region. Results indicate that the Cooper pairing potential is stronger in the underdoped region.
displays a dome-like curve with a maximum. In the inset to Fig. 5 is shown the GL coherence length ξ0 determined from Hc2 as a function of VG. The most striking anomalous result is enhancement of Hc2 accompanied by the decrease of ξ0 for decreasing VG, i.e. for the carrier depletion reflected by the dome-like curve in the underdoped region. Results indicate that the Cooper pairing potential is stronger in the underdoped region.
A systematic increase in Hc2 with decreasing doping has been reported both in high-Tc cuprates and iron-based superconductors31,32. This is usually considered as an evidence for the existence of a so-called ‘pseudogap’ state, in which the bosonic pairs form above Tc but cannot condensate into superconducting state due to dilution of pairs33. Recently, the planar tunneling spectroscopy study in 2DEG at a (001) LAO/STO interface has shown that the energy gap ∆ increases with charge carrier depletion in both underdoped and overdoped regions9. And, the coherence-peak-broadening parameter Γ derived from the Dynes fit, that is related to the strength of the superconducting pairing interaction, increases steeply with decreasing VG. GuangLei Cheng et al.10 recently reported that the Cooper pairs form at temperatures well above the superconducting transition temperature of the (001)LAO/STO superconducting system. In the experiments they used a superconducting single-electron transistor and they observed that pairs condensate at low magnetic fields and temperatures. The physical understanding of the processes at (110) LAO/STO interface is analogous to that of hole-doped cuprates31, namely, the pairing potential is stronger and the Ginzburg-Landau coherence length ξ0decreases in the underdoped region as VG decreases. The trend differs from that of superfluid density and superconducting transition temperature7,14. Namely, the superfluid density decreases with decreasing VG providing that the phase fluctuation is important14,34,35. The observation of ∆/Γ scaled with Tc in ref. 9 implies that the limited quasiparticle lifetime controls Tc effectively9 and the reduction in Tc versus ∆ was attributed to a competing order parameter or to a weak phase coherence. In addition, in LAO/STO system the spin-orbit coupling is non negligible and strongly depends on VG36,37. Both conventional and unconventional pairing mechanisms have been considered to describe superconductivity in interfaces5,6,16,38. For example, the spin-orbit coupling36,37 and the coexistence of superconductivity and ferromagnetism11,12,13 may indicate formation of possible exotic superconducting states such as finite momentum Cooper pairing39,40. One has also to consider a different orbital reconstruction between (001) and (110) systems20,41. Superconducting properties and Rashba spin-orbit coupling can be largely tuned by controlling selective orbital occupancy in different crystal orientations20. For the underdoped region, it has been reported that a Lishiftz transition is observed at (001) interface42,43. The (110) system is expected to be characterized only by a 3dxz/dyz filled electronic state; thus the superconducting properties of the (110) interface system can be substantially different from that of the (001) system.
Conclusions
We systematically investigated the upper critical field as a function of gate voltage by ultralow temperature magnetoresistance measurements in superconducting 2DEG of a (110) LAO/STO interface. We found that upper critical field increases as the gate voltage decreases. Two independent methods to determine the upper critical field give a similar trend. This implies that the pairing potential is stronger in the underdoped region. This observation is similar to recent reports that consider a pseudogap-like behavior at the (001) LAO/STO interface. Our results for an interface with a different orientation contribute to understanding of the pairing mechanism of superconductivity at LAO/STO interface.
Method
A five-unit-cell LaAlO3 thin film was grown on the (110) SrTiO3 substrate (500 μm thickness) by pulsed laser deposition. Details were described in ref. 19. A metallic back gate was evaporated and attached to the rear of the substrate. Leakage current was low (below the maximum value of 5 nA at VG = 200 V). Standard four-terminal resistance measurements were made using wedge-bonding contacts. The sample was cooled in a dilution refrigerator with a base temperature of 10 mK. The measurement current is sufficiently low (~50 nA) to avoid sample heating at ultralow temperatures. To ensure the reversible behavior of the superconductivity, the gate voltage was ramped up to 200 V after cooling down. Perpendicular magnetic field B was applied to the sample (interface) surface and the field direction is the same for all measurements.
Additional Information
How to cite this article: Shen, S. C. et al. Gate dependence of upper critical field in superconducting (110) LaAlO3/SrTiO3 interface. Sci. Rep. 6, 28379; doi: 10.1038/srep28379 (2016).
References
Hwang, H. Y. Atomic control of the electronic structure at complex oxide heterointerfaces. MRS bulletin 31, 28–35 (2006).
Hwang, H. Y. et al. Emergent phenomena at oxide interfaces. Nat. Mater. 11, 103–113 (2012).
Reyren, N. et al. Superconducting interfaces between insulating oxides. Science 317, 1196–1199 (2007).
Michaeli, K., Potter, A. C. & Lee, P. A. Superconducting and ferromagnetic phases in SrTiO3/LaAlO3 oxide interface structures: possibility of finite momentum pairing. Phys. Rev. Lett. 108, 117003 (2012).
Boschker, H., Richter, C., Fillis-Tsirakis, E., Schneider, C. W. & Mannhart, J. Electron-phonon Coupling and the Superconducting Phase Diagram of the LaAlO3/SrTiO3 Interface. Sci. Rep. 5, 12309 (2015).
Scheurer, M. S. & Schmalian, J. Topological superconductivity and unconventional pairing in oxide interfaces. Nat. Commun. 6, 6005 (2015).
Caviglia, A. D. et al. Electric field control of the LaAlO3/SrTiO3 interface ground state. Nature 456, 624–627 (2008).
Gariglio, S., Gabay, M., Mannhart, J. & Triscone, J.-M. Interface superconductivity. Physica C: Superconductivity and its Applications 514, 189–198 (2015).
Richter, C. et al. Interface superconductor with gap behaviour like a high-temperature superconductor. Nature 502, 528–531 (2013).
Cheng, G. L. et al. Electron pairing without superconductivity. Nature 521, 196–199 (2015).
Dikin, D. et al. Coexistence of superconductivity and ferromagnetism in two dimensions. Phys. Rev. Lett. 107, 056802 (2011).
Bert, J. A. et al. Direct imaging of the coexistence of ferromagnetism and superconductivity at the LaAlO3/SrTiO3 interface. Nat. Phys. 7, 767–771 (2011).
Li, L., Richter, C., Mannhart, J. & Ashoori, R. C. Coexistence of magnetic order and two-dimensional superconductivity at LaAlO3/SrTiO3 interfaces. Nat. Phys. 7, 762–766 (2011).
Bert, J. A. et al. Gate-tuned superfluid density at the superconducting LaAlO3/SrTiO3 interface. Phys. Rev. B 86, 060503 (2012).
Lin, X., Zhu, Z., Fauqué, B. & Behnia, K. Fermi Surface of the Most Dilute Superconductor. Phys. Rev. X 3, 021002 (2013).
Klimin, S., Tempere, J., Devreese, J. & Van Der Marel, D. Interface superconductivity in LaAlO3/SrTiO3 heterostructures. Phys. Rev. B 89, 184514 (2014).
Tinkham, M. Introduction to superconductivity. (Courier Corporation, 2012).
Reyren, N. et al. Anisotropy of the superconducting transport properties of the LaAlO3/SrTiO3 interface. Appl. Phys. Lett. 94, 112506 (2009).
Han, Y. L. et al. Two-dimensional superconductivity at (110) LaAlO3/SrTiO3 interfaces. Appl. Phys. Lett. 105, 192603 (2014).
Herranz, G. et al. Engineering two-dimensional superconductivity and Rashba spin-orbit coupling in LaAlO3/SrTiO3 quantum wells by selective orbital occupancy. Nat. Commun. 6, 6028 (2015).
Herranz, G., Sanchez, F., Dix, N., Scigaj, M. & Fontcuberta, J. High mobility conduction at (110) and (111) LaAlO3/SrTiO3 interfaces. Sci. Rep. 2, 758 (2012).
Annadi, A. et al. Anisotropic two-dimensional electron gas at the LaAlO3/SrTiO3 (110) interface. Nat. Commun. 4, 1838 (2013).
Biscaras, J. et al. Two-dimensional superconducting phase in LaTiO3/SrTiO3 heterostructures induced by high-mobility carrier doping. Phys. Rev. Lett. 108, 247004 (2012).
Fournier, P. & Greene, R. Doping dependence of the upper critical field of electron-doped Pr2−xCexCuO4 thin films. Phys. Rev. B 68, 094507 (2003).
Fuchs, G. et al. High-Field Pauli-Limiting Behavior and Strongly Enhanced Upper Critical Magnetic Fields near the Transition Temperature of an Arsenic-Deficient LaO0.9F0.1FeAs1−δ Superconductor. Phys. Rev. Lett. 101, 237003 (2008).
Vedeneev, S., Piot, B., Maude, D. & Sadakov, A. Temperature dependence of the upper critical field of FeSe single crystals. Phys. Rev. B 87, 134512 (2013).
Goldman, A. Superconductor-insulator transitions. Int. J. Mod. Phys. B 24, 4081–4101 (2010).
Gantmakher, V. F. & Dolgopolov, V. T. Superconductor-insulator quantum phase transition. Physics-Uspekhi 53, 1–49 (2010).
Biscaras, J. et al. Multiple quantum criticality in a two-dimensional superconductor. Nat. Mater. 12, 542–548 (2013).
Mehta, M. et al. Magnetic field tuned superconductor-to-insulator transition at the LaAlO3/SrTiO3 interface. Phys. Rev. B 90, 100506 (2014).
Wang, Y. et al. Dependence of upper critical field and pairing strength on doping in cuprates. Science 299, 86–89 (2003).
Kohama, Y. et al. Doping dependence of the upper critical field and Hall resistivity of LaFeAsO1−xFx(x = 0, 0.025, 0.05, 0.07, 0.11 and 0.14). Phys. Rev. B 79, 144527 (2009).
Timusk, T. & Statt, B. The pseudogap in high-temperature superconductors: an experimental survey. Rep. Prog. Phys. 62, 61 (1999).
Uemura, Y. et al. Universal Correlations between Tc and ns/m*(Carrier Density over Effective Mass) in High-T c Cuprate Superconductors. Phys. Rev. Lett. 62, 2317 (1989).
Emery, V. & Kivelson, S. Importance of phase fluctuations in superconductors with small superfluid density. Nature 374, 434–437 (1995).
Shalom, M. B., Sachs, M., Rakhmilevitch, D., Palevski, A. & Dagan, Y. Tuning spin-orbit coupling and superconductivity at the SrTiO3/LaAlO3 interface: a magnetotransport study. Phys. Rev. Lett. 104, 126802 (2010).
Caviglia, A. et al. Tunable Rashba spin-orbit interaction at oxide interfaces. Phys. Rev. Lett. 104, 126803 (2010).
Stephanos, C., Kopp, T., Mannhart, J. & Hirschfeld, P. Interface-induced d-wave pairing. Phys. Rev. B 84, 100510 (2011).
Michaeli, K., Potter, A. C. & Lee, P. A. Superconducting and ferromagnetic phases in SrTiO3/LaAlO3 oxide interface structures: possibility of finite momentum pairing. Phys. Rev. Lett. 108, 117003 (2012).
Loder, F., Kampf, A. P. & Kopp, T. Superconductivity with Rashba spin–orbit coupling and magnetic field. Journal of Physics: Condensed Matter 25, 362201 (2013).
Pesquera, D. et al. Two-Dimensional electron gases at LaAlO3/SrTiO3 interfaces: orbital symmetry and hierarchy engineered by crystal orientation. Phys. Rev. Lett. 113, 156802 (2014).
Joshua, A., Pecker, S., Ruhman, J., Altman, E. & Ilani, S. A universal critical density underlying the physics of electrons at the LaAlO3/SrTiO3 interface. Nat. Commun. 3, 1129 (2012).
Stornaiuolo, D. et al. Tunable spin polarization and superconductivity in engineered oxide interfaces. Nat. Mater. 15, 278–283 (2016).
Acknowledgements
Authors gratefully acknowledge the technical assistance of Haiou Li with transport measurements. We also would like to thank Badica Petre for useful discussions. This work was supported by the Ministry of Science and Technology of China (Grants Nos 2013CB921701, 2013CBA01603 and 2014CB920903) and the National Natural Science Foundation of China (Grants Nos 11474022, 51172029, 11374035, and11422430).
Author information
Authors and Affiliations
Contributions
J.C.N., G.P.G. and S.C.S. proposed and designed experiments. S.C.S., C.J.L. and Y.P.H. prepared the samples. S.C.S. performed the measurements with the assistance from B.B.C. and G.C. Results were analyzed by J.C.N., S.C.S., H.X.X. and X.X.W. The manuscript was written by S.C.S. and J.C.N. and discussed with C.M.X., R.F.D. and L.H. All authors discussed and contributed with comments regarding results and the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Electronic supplementary material
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Shen, S., Chen, B., Xue, H. et al. Gate dependence of upper critical field in superconducting (110) LaAlO3/SrTiO3 interface. Sci Rep 6, 28379 (2016). https://doi.org/10.1038/srep28379
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep28379
This article is cited by
-
A possible superconductor-like state at elevated temperatures near metal electrodes in an LaAlO3/SrTiO3 interface
Scientific Reports (2018)
-
Formation of Two-dimensional Electron Gas at Amorphous/Crystalline Oxide Interfaces
Scientific Reports (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.

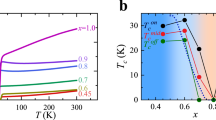




 for different VG.
for different VG.

 ,
,  (T = 0 K) (green diamonds) and
(T = 0 K) (green diamonds) and  .
. where
where  is the quantum flux. Continuous lines are guide for the eyes.
is the quantum flux. Continuous lines are guide for the eyes.