Abstract
Quantum entanglement of identical particles is essential in quantum information theory. Yet, its correct determination remains an open issue hindering the general understanding and exploitation of many-particle systems. Operator-based methods have been developed that attempt to overcome the issue. Here we introduce a state-based method which, as second quantization, does not label identical particles and presents conceptual and technical advances compared to the previous ones. It establishes the quantitative role played by arbitrary wave function overlaps, local measurements and particle nature (bosons or fermions) in assessing entanglement by notions commonly used in quantum information theory for distinguishable particles, like partial trace. Our approach furthermore shows that bringing identical particles into the same spatial location functions as an entangling gate, providing fundamental theoretical support to recent experimental observations with ultracold atoms. These results pave the way to set and interpret experiments for utilizing quantum correlations in realistic scenarios where overlap of particles can count, as in Bose-Einstein condensates, quantum dots and biological molecular aggregates.
Similar content being viewed by others
Introduction
Entanglement of identical particles is fundamental in understanding and exploiting composite quantum systems1,2, being a resource for scalable quantum information tasks2,3,4,5,6,7, for instance in Bose-Einstein condensates (ultracold gases in an optical lattice)8,9,10 or in quantum dots11,12,13,14. Therefore, its correct determination becomes a central requirement in quantum information theory. However, in systems of identical particles a new aspect emerges compared to systems of distinguishable particles, namely the role played by quantum particle indistinguishability in entanglement determination, which remains debated15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32. For instance, there is to date no general agreement either on the very simple case if two identical particles in the same site are entangled or not17,20,21,22,23,33,34, although there are recent experiments where this situation is analyzed13,35.
The main issue is that usual entanglement measures of quantum information theory, such as the von Neumann entropy of the reduced state, fail to be directly applied to identical particle states because they witness entanglement even for independent separated particles which are clearly uncorrelated, also showing contradictory results for bosons and fermions20,21. We remark that this issue exists not only in the particle-based (first quantization) description20,21 but also in the mode-based (second quantization) one15,16, where name labels do not explicitly appear but are however implicitly assumed. This problem has induced to develop methods to identify identical particle entanglement that are at variance with respect to the usual ones adopted for nonidentical particles, by redefining the notion of entanglement17,18,19,20,21,22,23,24,25,26,27 or searching for tensor product structures supported by the observables28,29,30,31,32,36 and whose aim is to discriminate the physical part of entanglement from the unphysical one. This necessity of new notions to discuss quantum correlations for identical and nonidentical particles looks surprising. Moreover, these approaches remain somewhat technically awkward and not well suited to quantify entanglement under general conditions of scalability and realistic scenarios where the constituting identical particles are close together to spatially overlap8,9,10,13,14,37. These drawbacks jeopardize analysis of entanglement and interpretation of experiments both under complete overlap and more strongly in the case of partial overlap. Thus, the relationship between entanglement and identity of particles is still an open issue from both conceptual and practical viewpoint hindering the general understanding and exploitation of composite quantum systems made of identical particles.
In quantum mechanics, name-labels are assigned to identical particles making them distinguishable. In order that this new fictitious system behaves as the real bosonic or fermionic one only the symmetrized or antisymmetrized states with respect to labels are permitted38,39. While this procedure works well in the usual practice, when it comes to entanglement which crucially depends on the form of the state vector, confusion arises linked to appearance of simultaneous real and fictitious (label born) contributions to it.
In this work we aim at providing an advancement towards the straightforward description of quantum correlations in identical particle systems grounded on simple physical arguments which can unambiguously answer the general question: when and at which extent quantum particle indistinguishability assumes physical relevance in determining the entanglement among the particles? We present here a treatment of identical particles which, like the second quantization, does not resort to name labels yet adopting a particle-based (first quantization) formalism in terms of states. This approach assumes that a many-particle state is a whole single object, characterized by a complete set of commuting observables and quantifies the physical entanglement of bosons and fermions on the same footing by the same notions used for distinguishable particles such as the von Neumann entropy of partial trace. It allows the study of identical particle entanglement under arbitrary conditions of wave function overlap at the same complexity level required for nonidentical particles and, albeit presented here for two particles, it is straightforwardly generalizable to many-particle systems for scalability. The known results for distinguishable particles can be also retrieved by imposing the condition of spatially separated (i.e., non overlapping) particles. Our approach quantitatively establishes the role of local measurements, particle nature and spatial overlap in assessing identical particle entanglement, supplying theoretical support to very recent experimental observations of entangling operations for identical atoms35.
Results
Description of the new approach
Indistinguishability requires that the identical particles cannot be individually addressed and, in accordance to quantum mechanics, introduction of unphysical quantities to treat them is thus unneeded. In fact, the system can be completely described in terms of observables determining the one-particle states. The global state, taken as the set of one-particle states, must be considered as a “holistic” indivisible entity. We illustrate this point by taking for simplicity a system of two identical particles whose state vector describes one particle in the state ϕ and one in ψ: it is thus completely characterized by enumerating the states and represented as  . The physical predictions on the system follow from the two-particle probability amplitudes
. The physical predictions on the system follow from the two-particle probability amplitudes  , where φ, ζ are one-particle states of another global two-particle state vector. We assume that it can be expressed by means of the one-particle amplitudes
, where φ, ζ are one-particle states of another global two-particle state vector. We assume that it can be expressed by means of the one-particle amplitudes  (l = φ, ζ; r = ϕ, ψ) and linearly depends on one-particle states. Due to the indistinguishability, the probability amplitude of finding one particle in φ (ζ) comes from having one particle in ϕ or ψ, as illustrated in Fig. 1. By simply applying the quantum mechanical superposition principle of alternative paths39, we define the two-particle probability amplitude
(l = φ, ζ; r = ϕ, ψ) and linearly depends on one-particle states. Due to the indistinguishability, the probability amplitude of finding one particle in φ (ζ) comes from having one particle in ϕ or ψ, as illustrated in Fig. 1. By simply applying the quantum mechanical superposition principle of alternative paths39, we define the two-particle probability amplitude  as a symmetrized inner product of two state vectors in terms of a linear combination with same weight of products of one-particle amplitudes
as a symmetrized inner product of two state vectors in terms of a linear combination with same weight of products of one-particle amplitudes
The probability amplitude  of Eq. (1) originates quantum mechanically from the lack of which-way information: the transition of one particle to φ (ζ) can equally come from ϕ and ψ.
of Eq. (1) originates quantum mechanically from the lack of which-way information: the transition of one particle to φ (ζ) can equally come from ϕ and ψ.

where η2 = 1. This equation constitutes the core of our approach and directly encompasses the required particle spin-statistics symmetry, or symmetrization postulate38,40 (see Methods about particle exchange in our approach). The right-hand side of Eq. (1) induces a symmetry with respect to the swapping of one-particle state position within the two-particle state vector:  or
or  . The probability amplitude of finding the two particles in the same state φ is
. The probability amplitude of finding the two particles in the same state φ is  . As usual, according to the Pauli exclusion principle this must take the minimum value (zero) for fermions which gives η = −1, while the maximum value for bosons implying η = +1. Linearity of the two-particle state vector with respect to one-particle states immediately follows from the linearity of the one-particle amplitudes in Eq. (1). The two-particle state vectors thus span a no-label symmetric state space
. As usual, according to the Pauli exclusion principle this must take the minimum value (zero) for fermions which gives η = −1, while the maximum value for bosons implying η = +1. Linearity of the two-particle state vector with respect to one-particle states immediately follows from the linearity of the one-particle amplitudes in Eq. (1). The two-particle state vectors thus span a no-label symmetric state space  . In general, as in second quantization, a two-particle state
. In general, as in second quantization, a two-particle state  is not normalized. The corresponding normalized state
is not normalized. The corresponding normalized state  , such that
, such that  , is
, is  , where
, where  is obtained by Eq. (1). For orthogonal one-particle states,
is obtained by Eq. (1). For orthogonal one-particle states,  , one has
, one has  and
and  .
.
A one-particle operator A(1), according to the standard definition38, acts on a two-particle state as  . Its expectation value on a normalized state is
. Its expectation value on a normalized state is  . In a one-particle state space with basis
. In a one-particle state space with basis  , in general
, in general  . Using symmetry and linearity from Eq. (1), it is straightforward to show that
. Using symmetry and linearity from Eq. (1), it is straightforward to show that  . We now define a non-separable symmetric external product of one-particle states
. We now define a non-separable symmetric external product of one-particle states  , from which
, from which  and
and  (for fermions it recalls Penrose’s wedge product defined in terms of labelled states41). This permits us to write
(for fermions it recalls Penrose’s wedge product defined in terms of labelled states41). This permits us to write  which defines a symmetric inner product between state spaces of different dimensionality
which defines a symmetric inner product between state spaces of different dimensionality

This equation provides the unnormalized reduced one-particle pure state obtained after projecting a two-particle state on  (one-particle projective measurement). Consider now the one-particle projection operator
(one-particle projective measurement). Consider now the one-particle projection operator  and then define the one-particle identity operator
and then define the one-particle identity operator  , such that
, such that  . It is immediate to see that
. It is immediate to see that  and thus
and thus  . Therefore, the normalized reduced one-particle pure state
. Therefore, the normalized reduced one-particle pure state  and the probability pk to observe it after the projective measurement
and the probability pk to observe it after the projective measurement  are, respectively,
are, respectively,

where  is obtained by Eq. (2) and
is obtained by Eq. (2) and  . If the two one-particle states are orthonormal
. If the two one-particle states are orthonormal  then
then  , which corresponds to the sum of probabilities of two incompatible outcomes, as expected. The partial trace of a system is physically interpreted as the statistical ensemble of all the normalized reduced states obtained after projective measurement on the basis states, that operationally corresponds to measure a subsystem particle without registering the outcomes1,2. Hence, from Eq. (3) we immediately determine the one-particle reduced density matrix as
, which corresponds to the sum of probabilities of two incompatible outcomes, as expected. The partial trace of a system is physically interpreted as the statistical ensemble of all the normalized reduced states obtained after projective measurement on the basis states, that operationally corresponds to measure a subsystem particle without registering the outcomes1,2. Hence, from Eq. (3) we immediately determine the one-particle reduced density matrix as

where  and
and  . For calculation convenience, we emphasize that
. For calculation convenience, we emphasize that  is obtained starting from unnormalized two-particle states and finally introducing a normalization constant
is obtained starting from unnormalized two-particle states and finally introducing a normalization constant  such that
such that  . We stress that the definition of partial trace given above is a physical operation on the system state, based on effective projective measurements, which never suffers the controversies exhibited by the unphysical partial trace operation performed in the description with name labels (see Methods for comparison and discussions)20,21.
. We stress that the definition of partial trace given above is a physical operation on the system state, based on effective projective measurements, which never suffers the controversies exhibited by the unphysical partial trace operation performed in the description with name labels (see Methods for comparison and discussions)20,21.
As a consequence of remaining within  , we can exploit the ordinary notion that the degree of mixing of the reduced density matrix is directly related to the amount of entanglement of the global pure state1. Entanglement is a nonlocal quantum feature and its presence in composite systems of nonidentical particles is individuated by local measurements made on the individual particles1,2,21. To quantify entanglement of identical particles, which are individually unaddressable, a suitable definition of one-particle measurement must be given which requires the condition of locality. Here we give the following:
, we can exploit the ordinary notion that the degree of mixing of the reduced density matrix is directly related to the amount of entanglement of the global pure state1. Entanglement is a nonlocal quantum feature and its presence in composite systems of nonidentical particles is individuated by local measurements made on the individual particles1,2,21. To quantify entanglement of identical particles, which are individually unaddressable, a suitable definition of one-particle measurement must be given which requires the condition of locality. Here we give the following:
Definition. A local one-particle measurement for systems of identical particles is the measurement of a property of one particle performed on a localized region of space M (site or spatial mode) where the particle has nonzero probability of being found.
We stress that this definition is in perfect analogy with the meaning of local measurement in ordinary Bell nonlocality tests1,2,20 and, in the case of spatially separated particles, reduces to the usual measurement on a given (addressable) particle. In fact, identical particles are recognized behaving as nonidentical when they live in spatially separated modes42, that is a natural request in recovering the distinguishability of bosons and fermions in experiments43. It is known that nonlocal measurements on uncorrelated spatially separated identical particles produce the so-called “measurement-induced entanglement”21,44 (see Methods). Along this work, we are only interested to the entanglement determined by local measurements according to the above definition. Peculiar particle identity effects on entanglement are expected to manifest when particles are close enough to have overlapping spatial modes.
Due to these fundamental preliminary results of our new approach, the entanglement  of a pure state of two identical particles can be then quantified via the Von Neumann entropy of the one-particle reduced density matrix derived by the localized partial trace, which is obtained by Eq. (4) with the sum over the index k limited to the subset kM corresponding to the subspace
of a pure state of two identical particles can be then quantified via the Von Neumann entropy of the one-particle reduced density matrix derived by the localized partial trace, which is obtained by Eq. (4) with the sum over the index k limited to the subset kM corresponding to the subspace  of one-particle basis states localized in
of one-particle basis states localized in  , where
, where  is a normalization constant such that
is a normalization constant such that  . The latter trace (Tr(1)) is meant within the complete one-particle basis
. The latter trace (Tr(1)) is meant within the complete one-particle basis  where
where  is in general defined. Thus, we have
is in general defined. Thus, we have

where  is the von Neumann entropy and λi are the eigenvalues of
is the von Neumann entropy and λi are the eigenvalues of  . Due to particle indistinguishability the amount of EM is in general expected to depend on M, that is on the localized region M where the measurement is performed21,23. When the particles are either spatially separated or in the same mode there cannot be any dependence on the localized mode where the measurement is done and we deem the entanglement obtained in this case as the “intrinsic”, absolute entanglement of the system. We also notice that a wider scenario surfaces here regarding the entanglement determination for identical particles. In fact, after obtaining
. Due to particle indistinguishability the amount of EM is in general expected to depend on M, that is on the localized region M where the measurement is performed21,23. When the particles are either spatially separated or in the same mode there cannot be any dependence on the localized mode where the measurement is done and we deem the entanglement obtained in this case as the “intrinsic”, absolute entanglement of the system. We also notice that a wider scenario surfaces here regarding the entanglement determination for identical particles. In fact, after obtaining  by locally tracing out one particle, the remained particle can be measured either again in the same localized mode M or in a separated localized mode M′.
by locally tracing out one particle, the remained particle can be measured either again in the same localized mode M or in a separated localized mode M′.
As an advancement with respect to the state-of-art, the described approach allows the quantification in complete generality of the physical entanglement of the two-particle system by directly applying the standard notion of partial trace to the assigned system state. This remarkable aspect makes our treatment very convenient and naturally generalizable to systems of many particles (to be addressed elsewhere), thus overcoming the drawbacks present in other specific name-labelled methods where redefinition of entanglement measures and witnesses are required20,21,23,25 (see Methods for some details on this point).
Application
We now apply our method to the simplest case where entanglement may befall, that is a two-qubit system. We take each single-qubit state  as
as  , where A indicates the spatial mode and
, where A indicates the spatial mode and  two pseudospin internal states (e.g., components ±1/2 of a spin-1/2 fermion, two energy levels of a boson, horizontal H and vertical V photon polarizations). We are interested in systems where spatial modes can overlap for an arbitrary extent. A simple system where this situation can happen is depicted in the asymmetric double-well configuration of Fig. 2, where one particle is in the mode
two pseudospin internal states (e.g., components ±1/2 of a spin-1/2 fermion, two energy levels of a boson, horizontal H and vertical V photon polarizations). We are interested in systems where spatial modes can overlap for an arbitrary extent. A simple system where this situation can happen is depicted in the asymmetric double-well configuration of Fig. 2, where one particle is in the mode  and one particle is in the mode
and one particle is in the mode  . This represents, for instance, a physical situation when at a given time the particle initially localized in
. This represents, for instance, a physical situation when at a given time the particle initially localized in  may slowly tunnel into
may slowly tunnel into  , which may occur in Bose-Einstein condensates and quantum dots8,11,12,13. It is then convenient to study states whose structure make them entangled in the spatial separation scenario, as the Bell-like states. We thus choose two identical qubits prepared in the linear combination, valid for bosons, fermions and nonidentical particles,
, which may occur in Bose-Einstein condensates and quantum dots8,11,12,13. It is then convenient to study states whose structure make them entangled in the spatial separation scenario, as the Bell-like states. We thus choose two identical qubits prepared in the linear combination, valid for bosons, fermions and nonidentical particles,

where a is positive real,  and
and  .
.
Using Eq. (4), suitably normalized, with projective measurements onto the localized one-particle subspace  , it is straightforward to find the amount of entanglement
, it is straightforward to find the amount of entanglement  , which is given by Eq. (5) with only two nonzero eigenvalues (see Supplemental Material)
, which is given by Eq. (5) with only two nonzero eigenvalues (see Supplemental Material)

where  is the overlap parameter, which here coincides with the spatial mode fidelity. This result shows the quantitative manifestation of wave function overlap and particle statistics directly on the quantum entanglement of the system state, differently from other methods which require state tomography reconstruction at the level of separated detectors23. In fact, our formalism permits the spatial overlap to explicitly prove responsible of a statistics-sensitive quantum interference phenomenon due to the phase in
is the overlap parameter, which here coincides with the spatial mode fidelity. This result shows the quantitative manifestation of wave function overlap and particle statistics directly on the quantum entanglement of the system state, differently from other methods which require state tomography reconstruction at the level of separated detectors23. In fact, our formalism permits the spatial overlap to explicitly prove responsible of a statistics-sensitive quantum interference phenomenon due to the phase in  (see Methods). Entanglement
(see Methods). Entanglement  is plotted in Fig. 3 to evidence its dependence on the nature of the particles. Relative phase θ and particle statistics η have an effect for any 0 < χ < 1 (provided that
is plotted in Fig. 3 to evidence its dependence on the nature of the particles. Relative phase θ and particle statistics η have an effect for any 0 < χ < 1 (provided that  ). For states of the same form, entanglement is different for fermions and bosons: fermionic entanglement (η = −1) and bosonic one (η = +1) can be obtained one from the other by shifting θ of π. When χ = 0 (B = R, orthogonal or spatially separated modes), one as expected gets
). For states of the same form, entanglement is different for fermions and bosons: fermionic entanglement (η = −1) and bosonic one (η = +1) can be obtained one from the other by shifting θ of π. When χ = 0 (B = R, orthogonal or spatially separated modes), one as expected gets  where
where  is the entanglement entropy of nonidentical particles1,2. This fact confirms that, under the condition of spatial separation, identical particles have the same entanglement of nonidentical particles42. Moreover, for χ = 0 and a = 1,
is the entanglement entropy of nonidentical particles1,2. This fact confirms that, under the condition of spatial separation, identical particles have the same entanglement of nonidentical particles42. Moreover, for χ = 0 and a = 1,  becomes manifestly unentangled (E = 0) as should be, a result to be contrasted with the entanglement entropy S = log2 2 = 1 obtained so far for such a state via partial trace within both first and second quantization approaches15,16,20,21,28. This fact made it necessary a rule to bypass this problem that however required a priori knowledge of the true quantum correlations in the state20,21.
becomes manifestly unentangled (E = 0) as should be, a result to be contrasted with the entanglement entropy S = log2 2 = 1 obtained so far for such a state via partial trace within both first and second quantization approaches15,16,20,21,28. This fact made it necessary a rule to bypass this problem that however required a priori knowledge of the true quantum correlations in the state20,21.
(A) Entanglement  as a function of a2 for θ = 0 and χ = 0.3 for bosons (blue dotted line) and fermions (orange dashed line), compared to the corresponding entanglement of nonidentical particles
as a function of a2 for θ = 0 and χ = 0.3 for bosons (blue dotted line) and fermions (orange dashed line), compared to the corresponding entanglement of nonidentical particles  (red solid line).
(red solid line).  is always over the “nonidentical particle fence” delimited by
is always over the “nonidentical particle fence” delimited by  , collapsing to it when χ = 0. (B) Density plot of bosonic entanglement
, collapsing to it when χ = 0. (B) Density plot of bosonic entanglement  , for a = 0.5, as a function of both relative phase θ and overlap parameter χ. The corresponding nonidentical particle entanglement, retrieved when χ = 0, is constantly equal to
, for a = 0.5, as a function of both relative phase θ and overlap parameter χ. The corresponding nonidentical particle entanglement, retrieved when χ = 0, is constantly equal to  . (C) Density plot of the difference between bosonic and fermionic entanglement, for a = 0.5.
. (C) Density plot of the difference between bosonic and fermionic entanglement, for a = 0.5.
When a = 1, 0, the state  reduces to the elementary states
reduces to the elementary states  ,
,  , respectively. In this case
, respectively. In this case  for both bosons and fermions which ranges from 0 (χ = 0) to 1 (χ = 1), while for nonidentical particles one has Eni = 0 since these states represent separable states and the one-particle reduced state is pure.
for both bosons and fermions which ranges from 0 (χ = 0) to 1 (χ = 1), while for nonidentical particles one has Eni = 0 since these states represent separable states and the one-particle reduced state is pure.
We now aim at answering unambiguously to the question whether two particles in the same site are entangled. We shall show that the answer depends on the configuration of the internal degrees of freedom (pseudospins). In particular, the state  (χ = 1) exhibits E = 1: two identical particles in the same site with opposite pseudospins are maximally entangled. We adopt two argumentations, a conceptual and a definitional one, to further explain this result. On the one hand, it is physically intuitive to recognize that, if two identical particles in the same site with opposite spins are measured in their spins, it is impossible to distinguish which particle has a given spin because of the impossibility to individually address them. Indeed, the local spin measurement under this configuration is unavoidably “nonlocal” with respect to the particles, so implying the presence of entanglement in analogy to the entanglement induced by nonlocal measurements on uncorrelated spatially separated particles21. However, the two-particle entanglement is here not induced but intrinsic of the system due to its own spatial configuration. On the other hand, a two-particle state is maximally entangled if the two particles are perfectly correlated with respect to their spins when projecting (measuring) one particle along an arbitrary spin direction u, as happens for Bell states of distinguishable particles1,2. Our approach shows that this is the case for the two-particle elementary state
(χ = 1) exhibits E = 1: two identical particles in the same site with opposite pseudospins are maximally entangled. We adopt two argumentations, a conceptual and a definitional one, to further explain this result. On the one hand, it is physically intuitive to recognize that, if two identical particles in the same site with opposite spins are measured in their spins, it is impossible to distinguish which particle has a given spin because of the impossibility to individually address them. Indeed, the local spin measurement under this configuration is unavoidably “nonlocal” with respect to the particles, so implying the presence of entanglement in analogy to the entanglement induced by nonlocal measurements on uncorrelated spatially separated particles21. However, the two-particle entanglement is here not induced but intrinsic of the system due to its own spatial configuration. On the other hand, a two-particle state is maximally entangled if the two particles are perfectly correlated with respect to their spins when projecting (measuring) one particle along an arbitrary spin direction u, as happens for Bell states of distinguishable particles1,2. Our approach shows that this is the case for the two-particle elementary state  above (we omit the mode state L for simplicity). By using Eq. (2), it is straightforward to see that
above (we omit the mode state L for simplicity). By using Eq. (2), it is straightforward to see that

where the versor u′ is the reflection of u with respect to the x-y plane (see Methods for calculation details). Notice that the spin correlations found in Eq. (8) for η = ±1 (bosons and fermions) are exactly those occurring, respectively, for the Bell states of distinguishable particles  by measuring a given (addressable) particle. The latter property provides theoretical support and prediction of the very recent experimental observations of spontaneous entanglement creation where such a state is generated by simply transporting two ultracold atoms of rubidium with opposite spins into the same optical tweezer35. Contrarily, we observe that the entanglement of the state
by measuring a given (addressable) particle. The latter property provides theoretical support and prediction of the very recent experimental observations of spontaneous entanglement creation where such a state is generated by simply transporting two ultracold atoms of rubidium with opposite spins into the same optical tweezer35. Contrarily, we observe that the entanglement of the state  (valid for bosons) is zero, as also verifiable by the fact that
(valid for bosons) is zero, as also verifiable by the fact that  independently of the first projective measurement: the state is uncorrelated (see Methods). These results clarify the crucial role of both spatial mode and internal state configuration in assessing the entanglement of identical particles.
independently of the first projective measurement: the state is uncorrelated (see Methods). These results clarify the crucial role of both spatial mode and internal state configuration in assessing the entanglement of identical particles.
It is now worth to observe that the entanglement of  of Eq. (6) when determined by tracing out the particle spin in the localized mode
of Eq. (6) when determined by tracing out the particle spin in the localized mode  results
results  (see Methods for details). Putting these findings together shows how the entanglement fundamentally depends on the local measurement, a peculiar trait of identical particles which does not exist for distinguishable (or spatially separated) particles. In particular, the dependence of entanglement on the particle overlap appears only if both particles have nonzero probability of staying in the same localized mode where the measurement is done. The amount of entanglement naturally ceases to be dependent on the measurement localization only in the extremal situations when the particles are spatially separated or in the same mode, that is when we can speak about an intrinsic, absolute entanglement of the system. Identical particles prove more “flexible” than nonidentical ones in getting entangled: identical particle entanglement is never exceeded by the nonidentical particle counterpart.
(see Methods for details). Putting these findings together shows how the entanglement fundamentally depends on the local measurement, a peculiar trait of identical particles which does not exist for distinguishable (or spatially separated) particles. In particular, the dependence of entanglement on the particle overlap appears only if both particles have nonzero probability of staying in the same localized mode where the measurement is done. The amount of entanglement naturally ceases to be dependent on the measurement localization only in the extremal situations when the particles are spatially separated or in the same mode, that is when we can speak about an intrinsic, absolute entanglement of the system. Identical particles prove more “flexible” than nonidentical ones in getting entangled: identical particle entanglement is never exceeded by the nonidentical particle counterpart.
We remark that a way to broadcast and exploit identical particle entanglement in the presence of spatial overlap is by extraction procedures, for instance, from Bose-Einstein condensates via tunneling8 or from fermions (electrons) in quantum dots via photon emission12. The extraction of entanglement of overlapping identical particles has been so far investigated solely for the case of complete overlap based on particle labels33,34. This extraction can be also easily performed within our approach (see Methods). Our results directly indicate that the fundamental reason why entanglement can be extracted in this case is due to its intrinsic presence in the state.
Discussion
We have introduced a description of identical particles which, without resorting to particle labels, enables the treatment of their entanglement by means of the same notions usually adopted in entanglement theory for distinguishable particles, such as the von Neumann entropy of the reduced state after partial trace. Our approach treats bosons and fermions on the same footing whose technical complexity is comparable to that for nonidentical particles. This aspect significantly facilitates both the quantitative study of entanglement under arbitrary conditions of particle wave function overlap and its generalization to many-particle systems for scalability.
We have found that, contrarily to what happens for distinguishable particles, the amount of entanglement of identical particles exhibits a dependence on local measurements. In the specific case when two particles with opposite internal degrees of freedom (pseudospins) partially overlap in the same site where the projective measurements are done, we have shown that entanglement is a function of their spatial overlap and an “ordering” emerges for different particle types. It is also of interest that the degree of entanglement associated to identical particles is never surpassed by the counterpart of nonidentical ones. This result indicates that identical particles may be more efficient than distinguishable ones for entanglement-based quantum information tasks. Summarizing, we have the following properties: (i) it is not possible, by a set of local measurements, to assess an absolute measurement-independent amount of entanglement for systems of identical particles unless the particles are spatially separated or in the same spatial mode; (ii) bringing identical particles into overlapping modes acts like an “entangling gate” whose effectiveness depends on the amount of overlap. Therefore, our approach clearly demonstrates that a natural creation of maximally entangled states is possible just by moving two identical particles with opposite pseudospin into the same site, as recently confirmed in a recent experiment with ultracold atoms35.
This study can in perspective lead to determine not only entanglement but also other kinds of quantum correlations45,46 for systems of many identical particles, even in mixed states. Due to the importance of entanglement as a resource, its dynamical preservation is a crucial requirement47,48: our analysis provides the groundwork for further investigating this aspect. Our results supply theoretical grounds in setting and interpreting experiments to use quantum correlations even in realistic scenarios where overlap of particles is important, as in condensed matter (Bose-Einstein condensates, spin chains, anyonic models)8, quantum dots11,13, superconducting circuits49, trapped ions50, photons in waveguides51 and biological molecular aggregates37,52. Our approach finally enables the extension of extraction procedures33,34 for utilizing identical particle entanglement within the most general geometries of particle wave functions.
Methods
Particle exchange
One of the points that fundamentally differentiates the standard label-based approach from the present one is the role played by the exchange of particles. In fact, in the former the particle exchange operation is fundamental to construct a name label symmetrized state vector38, here it is not. Nevertheless, physical exchange of two identical particles continues being a well-defined operation. After the exchange, the physical two-particle state is indistinguishable from before and the state vector acted by the exchange operator  remains the same up to a global phase factor:
remains the same up to a global phase factor:  , the sign + being associated to bosons and − to fermions38. Using then Eq. (1), it follows
, the sign + being associated to bosons and − to fermions38. Using then Eq. (1), it follows  . Thus, the action of
. Thus, the action of  on the two-particle state simply swaps the position of the one-particle states as they appear in the state. The definition of the two-particle probability amplitude of Eq. (1) therefore directly encompasses the required particle spin-statistics symmetry, or symmetrization postulate38.
on the two-particle state simply swaps the position of the one-particle states as they appear in the state. The definition of the two-particle probability amplitude of Eq. (1) therefore directly encompasses the required particle spin-statistics symmetry, or symmetrization postulate38.
Measurement-induced entanglement
Consider two identical qubits in the state  , where L, R are two spatially separated orthogonal modes. This state is obtainable from the state
, where L, R are two spatially separated orthogonal modes. This state is obtainable from the state  considered in the main text for a = 1 and B = R (orthogonal modes). As discussed in the main text after Eq. (7), when the partial trace is performed by local projective measurement and the modes are spatially separated (orthogonal), the identical particles behave like nonidentical ones regarding their entanglement, that results to be
considered in the main text for a = 1 and B = R (orthogonal modes). As discussed in the main text after Eq. (7), when the partial trace is performed by local projective measurement and the modes are spatially separated (orthogonal), the identical particles behave like nonidentical ones regarding their entanglement, that results to be  , as expected. Differently, we now perform the partial trace of Eq. (4) onto the nonlocal one-particle basis
, as expected. Differently, we now perform the partial trace of Eq. (4) onto the nonlocal one-particle basis  , where
, where  are orthogonal nonlocal superpositions of the localized spatial modes
are orthogonal nonlocal superpositions of the localized spatial modes  ,
,  . Using Eqs (2) and (4), one easily obtains the “measurement-induced” reduced density matrix
. Using Eqs (2) and (4), one easily obtains the “measurement-induced” reduced density matrix  , from which
, from which  : the two qubits are maximally (measurement-induced) entangled. This fact confirms the peculiar property of entanglement of spatially separated identical particles to be sensitive to the nonlocal character of the measurement21.
: the two qubits are maximally (measurement-induced) entangled. This fact confirms the peculiar property of entanglement of spatially separated identical particles to be sensitive to the nonlocal character of the measurement21.
Derivation of the identical particle entanglement
In this section we explicitly derive the identical particle entanglement given by Eqs (5) and (7) of the two-qubit state  , where a is positive real,
, where a is positive real,  and
and  . Mode
. Mode  is a general linear combination of L and R as:
is a general linear combination of L and R as:  . Notice that when
. Notice that when  ,
,  . The (mode-spin) one-particle basis is then
. The (mode-spin) one-particle basis is then  . Using this basis and the linearity property, the state
. Using this basis and the linearity property, the state  can be written as
can be written as

In the state above, for partial overlap  , it is seen how both relative phase θ and particle statistics η = ±1 are expected to play a role in the entanglement property. In fact by using Eq. (2), suitably normalized, with projective measurements onto the localized one-particle subspace
, it is seen how both relative phase θ and particle statistics η = ±1 are expected to play a role in the entanglement property. In fact by using Eq. (2), suitably normalized, with projective measurements onto the localized one-particle subspace  , we obtain the following one-qubit reduced density matrix expressed in the above basis
, we obtain the following one-qubit reduced density matrix expressed in the above basis 

where

and  . Diagonalizing this reduced density matrix we find only two nonzero eigenvalues λ1, λ2 as reported in Eq. (7) of the main text, so that the identical particle entanglement is obtained by equation (5) of the manuscript as
. Diagonalizing this reduced density matrix we find only two nonzero eigenvalues λ1, λ2 as reported in Eq. (7) of the main text, so that the identical particle entanglement is obtained by equation (5) of the manuscript as  .
.
Let us now determine the entanglement of the state  of equation (8) by tracing out the particle in the other localized mode
of equation (8) by tracing out the particle in the other localized mode  . Since there is no overlap between
. Since there is no overlap between  and
and  , one expects that the amount of overlap does not play any role in this case. In fact, it is easy to see that, using Eq. (2) suitably normalized with projective measurements onto the localized one-particle subspace
, one expects that the amount of overlap does not play any role in this case. In fact, it is easy to see that, using Eq. (2) suitably normalized with projective measurements onto the localized one-particle subspace  , the one-particle reduced density matrix is localized in L and reads
, the one-particle reduced density matrix is localized in L and reads

which implies an entanglement entropy  . A projection of the reduced density matrix
. A projection of the reduced density matrix  on L obviously does not change its form, being already localized in L, that is:
on L obviously does not change its form, being already localized in L, that is:  , where
, where  .
.
We also notice that a wider scenario surfaces here regarding the entanglement determination for identical particles. In fact, after obtaining  by localized partial trace, the remained particle can be measured either again in the same localized mode M or in a separated localized mode M′. We can also choose to study the entanglement when the first partial trace is made on L and the second trace is made on R. By projecting
by localized partial trace, the remained particle can be measured either again in the same localized mode M or in a separated localized mode M′. We can also choose to study the entanglement when the first partial trace is made on L and the second trace is made on R. By projecting  of Eq. (9) on
of Eq. (9) on  by means of the projection operator
by means of the projection operator  and renormalizing we get
and renormalizing we get

which coincides with  and gives an entanglement entropy
and gives an entanglement entropy  .
.
We finally calculate the entanglement when the first partial trace is made on L and the second trace is made again on L. By projecting  on
on  by means of the projection operator
by means of the projection operator  and renormalizing we get
and renormalizing we get

which provides an entanglement entropy  .
.
These results show how identical particle entanglement quantitatively depends on the way the local measurement is performed. We notice that such a behavior already surfaced in the entanglement measured by the so-called “detection-level concurrence” Cd 23, which also includes the effects of spatial overlap and particle statistics. However, this new notion of entanglement measure Cd, introduced specifically for identical particles, presents drawbacks regarding the case of particles in the same site and generalizations to many particles (see discussion in Methods section: “Comparing our particle-based approach with the usual name-labelled one” below).
Entanglement extraction
We show here that identical particle entanglement extraction can be obtained within our approach, without resorting to particle name labels. For simplicity, we consider two identical qubits in the state  . This state is obtainable from
. This state is obtainable from  considered in the main text for
considered in the main text for  (B = L). We already know from the manuscript that this state is maximally entangled. This identical particle entanglement is unexploitable by local operations and classical communication (LOCC) because the single particles (subsystems) cannot be individually addressed. We then consider a unitary spin-insensitive splitting transformation (tunneling)
(B = L). We already know from the manuscript that this state is maximally entangled. This identical particle entanglement is unexploitable by local operations and classical communication (LOCC) because the single particles (subsystems) cannot be individually addressed. We then consider a unitary spin-insensitive splitting transformation (tunneling)  , where
, where  and
and  , that transfers a particle from the localized mode L to the two distinct modes C, D with amplitudes r, t, respectively. Such a transformation represents a beam splitter transformation from optics that, for instance, is equivalent to a tunneling operation for Bose-Einstein condensates where particles can leak from mode A into neighboring modes C, D34. Applying the splitting transformation to the input state
, that transfers a particle from the localized mode L to the two distinct modes C, D with amplitudes r, t, respectively. Such a transformation represents a beam splitter transformation from optics that, for instance, is equivalent to a tunneling operation for Bose-Einstein condensates where particles can leak from mode A into neighboring modes C, D34. Applying the splitting transformation to the input state  we immediately obtain the normalized output state
we immediately obtain the normalized output state

where  ,
,  and
and  . States
. States  and
and  are exactly of the same type of the input state, with all the particles being in a single mode: these two states thus have the same entanglement of the input state but this entanglement remains unexploitable by LOCC. The state
are exactly of the same type of the input state, with all the particles being in a single mode: these two states thus have the same entanglement of the input state but this entanglement remains unexploitable by LOCC. The state  instead is a maximal linear combination of two identical particles in two orthogonal distinct modes (like a Bell state). We know from the main text that this state is maximally entangled. This entanglement is now an exploitable resource within the LOCC framework because it is (probabilistically) established between two particles in accessible distinguishable (spatially separated) modes.
instead is a maximal linear combination of two identical particles in two orthogonal distinct modes (like a Bell state). We know from the main text that this state is maximally entangled. This entanglement is now an exploitable resource within the LOCC framework because it is (probabilistically) established between two particles in accessible distinguishable (spatially separated) modes.
We remark that the entanglement of three identical bosons in the same mode  , which is exactly the same state considered in ref. 34, can be straightforwardly extracted within our approach by following analogous calculations. In ref. 34 the extraction procedure required particle name labels, but we demonstrate that particle names, although useful in this specific case, are unneeded to perform this operation.
, which is exactly the same state considered in ref. 34, can be straightforwardly extracted within our approach by following analogous calculations. In ref. 34 the extraction procedure required particle name labels, but we demonstrate that particle names, although useful in this specific case, are unneeded to perform this operation.
Comparing our particle-based approach with the usual name-labelled one
In our particle-based approach with no labels, the elementary state of two independent identical particles having orthonormal states φ1 and φ2 is simply  . In the standard first quantization approach, where name labels are assigned to the particles, the same state is given by
. In the standard first quantization approach, where name labels are assigned to the particles, the same state is given by  , where + is for bosons (symmetrized state) and − for fermions (antisymmetrized state), respectively38,39. We stress that our state
, where + is for bosons (symmetrized state) and − for fermions (antisymmetrized state), respectively38,39. We stress that our state  is meant as a whole object and does not insinuate at all the presence of name labels for the particles. Differently, the state
is meant as a whole object and does not insinuate at all the presence of name labels for the particles. Differently, the state  , which has the structure of an entangled state with respect to the labels (1, 2), it is meant as a product state
, which has the structure of an entangled state with respect to the labels (1, 2), it is meant as a product state  of the two particles since it is obtainable by symmetrizing (antisymmetrizing) just this state20. To stress this difference, we notice that in our framework an entangled state of spatially separated identical particles has always the form
of the two particles since it is obtainable by symmetrizing (antisymmetrizing) just this state20. To stress this difference, we notice that in our framework an entangled state of spatially separated identical particles has always the form  , exactly as happens for distinguishable particles. Such a state in the standard description with labels has, instead, four ket states.
, exactly as happens for distinguishable particles. Such a state in the standard description with labels has, instead, four ket states.
We then remark that the one-particle projective measurement of Eq. (2) and the partial trace operation of Eq. (4) defined in our approach are physical operations performed on the two-particle system, giving the physical reduced state of the system, where it is not contemplated the addressing of an individual particle. At variance, a one-particle partial trace performed on a particle with a given name does not correspond to a physical trace. This is what happens in the name-labelled approaches for one-particle partial trace operations which are mere mathematical tools20,21. The latter fact gives rise to problems and contradictions between fermions and bosons in taking the von Neumann entropy of the reduced state as a faithful entanglement measure (see, for instance, discussions at pages 5–7 of ref. 20). Our (physical) partial trace operation conceptually differs from this one, never suffers these issues and always provides the physical entanglement of the system.
We now consider another important point. A standard criterion employed by the name-labelled approaches to witness entanglement is that, if a two-particle state is obtainable after symmetrization (antisymmetrization) with respect to the labels of a product state of orthonormal states, then it must be considered unentangled20,21. This assumption immediately excludes the possibility that an elementary state of two identical particles in orthogonal states can be entangled. In fact, in this case one retrieves the state  which is unentangled according to this criterion. The consequence of this fact is that two identical particles located in the same spatial mode A (complete overlap) with orthogonal internal states (e.g., opposite spins) are unentangled. We notice that this assumption and the same conclusion are also contained in the so-called “detection-level concurrence”23, which quantifies the entanglement of the two-particle state (expressed in terms of name labels) constructed by state tomography at spatially separated detectors where the particles are measured. But these results contrast with the findings obtained by entanglement extraction procedures33,34 and recent experimental observations14,35 which give a strong evidence of entanglement for identical particles with opposite spins in the same site. Our approach, where elementary identical particle states are assumed as a whole entity as should physically be, provides theoretical support to these observations. In general: an elementary two-particle state (e.g.,
which is unentangled according to this criterion. The consequence of this fact is that two identical particles located in the same spatial mode A (complete overlap) with orthogonal internal states (e.g., opposite spins) are unentangled. We notice that this assumption and the same conclusion are also contained in the so-called “detection-level concurrence”23, which quantifies the entanglement of the two-particle state (expressed in terms of name labels) constructed by state tomography at spatially separated detectors where the particles are measured. But these results contrast with the findings obtained by entanglement extraction procedures33,34 and recent experimental observations14,35 which give a strong evidence of entanglement for identical particles with opposite spins in the same site. Our approach, where elementary identical particle states are assumed as a whole entity as should physically be, provides theoretical support to these observations. In general: an elementary two-particle state (e.g.,  ) can be entangled even if the one-particle states are orthogonal
) can be entangled even if the one-particle states are orthogonal  provided that the spatial modes overlap
provided that the spatial modes overlap  .
.
It is also worth noticing that the mentioned detection-level concurrence23, besides missing the intrinsic entanglement present between two identical particles in the same site, is not suitable for treating entanglement in many-particle systems since it would require the construction by quantum state tomography of the overall state at the level of spatially separated detectors. The power of our approach becomes evident also in this respect: being based on the standard notion of (physical) partial trace on particles of the system, it indeed enables a natural extension to many-particle systems in analogy to the case of distinguishable particles.
Spin correlations for two identical particles in the same site
Let us take two identical particles with opposite spins in the same spatial mode, described by the state  and consider an arbitrary direction for the spin individuated by the versor
and consider an arbitrary direction for the spin individuated by the versor  , where θ and φ are the polar and azimuthal angle, respectively. We want to find what happens to the remaining particle of the state above after projecting one particle along the spins
, where θ and φ are the polar and azimuthal angle, respectively. We want to find what happens to the remaining particle of the state above after projecting one particle along the spins  and
and  38. By using Eq. (2) we easily find
38. By using Eq. (2) we easily find

It is immediately seen that, after each of the one-particle measurements above, the other particle is left in, respectively: (i) for fermions, η = −1,  ,
,  ; (ii) for bosons, η = +1,
; (ii) for bosons, η = +1,  ,
,  , where u′ is the versor defined by the angles
, where u′ is the versor defined by the angles  and φ′ = φ. Notice that the same results are obtained for distinguishable particles in the Bell states
and φ′ = φ. Notice that the same results are obtained for distinguishable particles in the Bell states  by projecting the first particle, for instance, along the spin directions above. The above results testify the perfect spin correlations between the two identical particles in the same site, which behave exactly like being maximally entangled. No particle has a definite state but the two particles are a whole object in a perfectly correlated (non-separable) state.
by projecting the first particle, for instance, along the spin directions above. The above results testify the perfect spin correlations between the two identical particles in the same site, which behave exactly like being maximally entangled. No particle has a definite state but the two particles are a whole object in a perfectly correlated (non-separable) state.
On the contrary, let us see what happens for a spin projective measurement on the (unnormalized) state  . We immediately see that
. We immediately see that

which, apart a normalization constant, gives the one-particle state  independently of the first measurement. The state
independently of the first measurement. The state  is thus unentangled (separable).
is thus unentangled (separable).
Additional Information
How to cite this article: Lo Franco, R. and Compagno, G. Quantum entanglement of identical particles by standard information-theoretic notions. Sci. Rep. 6, 20603; doi: 10.1038/srep20603 (2016).
References
Horodecki, R., Horodecki, P., Horodecki, M. & Horodecki, K. Quantum entanglement. Rev. Mod. Phys. 81, 865–942 (2009).
Amico, L., Fazio, R., Osterloh, A. & Vedral, V. Entanglement in many-body systems. Rev. Mod. Phys. 80, 517–576 (2008).
Giovannetti, V., Lloyd, S. & Maccone, L. Quantum-enhanced measurements: Beating the standard quantum limit. Science 306, 1330–1336 (2004).
Riedel, M. F. et al. Atom-chip-based generation of entanglement for quantum metrology. Nature 464, 1170–1173 (2010).
Benatti, F., Alipour, S. & Rezakhani, A. T. Dissipative quantum metrology in manybody systems of identical particles. New J. Phys. 16, 015023 (2014).
Cramer, M. et al. Spatial entanglement of bosons in optical lattices. Nature Commun. 4, 2161 (2013).
Marzolino, U. & Buchleitner, A. Quantum teleportation with identical particles. Phys. Rev. A 91, 032316 (2015).
Bloch, I., Dalibard, J. & Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 80, 885–964 (2008).
Hayes, D., Julienne, P. & Deutsch, I. Quantum logic via the exchange blockade in ultracold collisions. Phys. Rev. Lett. 98, 070501 (2007).
Anderlini, M. et al. Controlled exchange interaction between pairs of neutral atoms in an optical lattice. Nature 448, 452 (2007).
Petta, J. R. et al. Coherent manipulation of coupled electron spins in semiconductor quantum dots. Science 309, 2180 (2005).
Lundskog, A. et al. Direct generation of linearly polarized photon emission with designated orientations from site-controlled InGaN quantum dots. Light Sci. Appl. 3, e139 (2014).
Tan, Z. B. et al. Cooper pair splitting by means of graphene quantum dots. Phys. Rev. Lett. 114, 096602 (2015).
Veldhorst, M. et al. A two-qubit logic gate in silicon. Nature 526, 410 (2015).
Li, Y.-S., Zeng, B., Liu, X.-S. & Long, G.-L. Entanglement in a two-identical-particle system. Phys. Rev. A 64, 054302 (2001).
Paskauskas, R. & You, L. Quantum correlations in two-boson wave functions. Phys. Rev. A 64, 042310 (2001).
Schliemann, J., Cirac, J. I., Kus, M., Lewenstein, M. & Loss, D. Quantum correlations in two-fermion systems. Phys. Rev. A 64, 022303 (2001).
Eckert, K., Schliemann, J., Bruss, D. & Lewenstein, M. Quantum correlations in systems of indistinguishable particles. Ann. Phys. 299, 88–127 (2002).
Plastino, A., Manzano, D. & Dehesa, J. Separability criteria and entanglement measures for pure states of n identical fermions. Europhys. Lett. 86, 20005 (2009).
Ghirardi, G. & Marinatto, L. General criterion for the entanglement of two indistinguishable particles. Phys. Rev. A 70, 012109 (2004).
Tichy, M. C., Mintert, F. & Buchleitner, A. Essential entanglement for atomic and molecular physics. J. Phys. B: At. Mol. Opt. Phys. 44, 192001 (2011).
Ghirardi, G. C., Reusch, L. & Weber, T. Entanglement and properties of composite quantum systems: a conceptual and mathematical analysis. J. Stat. Phys. 108, 49 (2002).
Tichy, M. C., de Melo, F., Kus, M., Mintert, F. & Buchleitner, A. Entanglement of identical particles and the detection process. Fortschr. Phys. 61, 225 (2013).
Buscemi, F., Bordone, P. & Bertoni, A. Linear entropy as an entanglement measure in two-fermion systems. Phys. Rev. A 75, 032301 (2007).
Reusch, A., Sperling, J. & Vogel, W. Entanglement witnesses for indistinguishable particles. Phys. Rev. A 91, 042324 (2015).
Shi, Y. Quantum entanglement of identical particles. Phys. Rev. A 67, 024301 (2003).
Benenti, G., Siccardi, S. & Strini, G. Entanglement in helium. Eur. Phys. J. D 67, 83 (2013).
Balachandran, A. P., Govindarajan, T. R., de Queiroz, A. R. & Reyes-Lega, A. F. Entanglement and particle identity: a unifying approach. Phys. Rev. Lett. 110, 080503 (2013).
Benatti, F., Floreanini, R. & Marzolino, U. Bipartite entanglement in systems of identical particles: the partial transposition criterion. Ann. Phys. 327, 1304–1319 (2012).
Sasaki, T., Ichikawa, T. & Tsutsui, I. Entanglement of indistinguishable particles. Phys. Rev. A 83, 012113 (2011).
Benatti, F., Floreanini, R. & Marzolino, U. Entanglement robustness and geometry in systems of identical particles. Phys. Rev. A 85, 042329 (2012).
Benatti, F., Floreanini, R. & Titimbo, K. Entanglement of identical particles. Open Syst. Inf. Dyn. 21, 1440003 (2014).
Cavalcanti, D., Malard, L. M., Matinaga, F. M., Cunha, M. O. T. & Santos, M. F. Useful entanglement from the Pauli principle. Phys. Rev. B 76, 113304 (2007).
Killoran, N., Cramer, M. & Plenio, M. B. Extracting entanglement from identical particles. Phys. Rev. Lett. 112, 150501 (2014).
Kaufman, A. M. et al. Entangling two transportable neutral atoms via local spin exchange. Nature 527, 208 (2015).
Friis, N., Lee, A. R. & Bruschi, D. E. Fermionic-mode entanglement in quantum information. Phys. Rev. A 87, 022338 (2013).
Jesenko, S. & Znidaric, M. Optimal number of pigments in photosynthetic complexes. New J. Phys. 14, 093017 (2012).
Cohen-Tannoudji, C., Diu, B. & Laloe, F. Quantum Mechanics (Wiley-VCH, Paris, France, 1992).
Feynman, R. P., Hibbs, A. R. & Styer, D. F. Quantum mechanics and path integrals (Dover, New York, 2010).
Goyal, P. Informational approach to the symmetrization postulate. New J. Phys. 17, 013043 (2015).
Penrose, R. The Road to Reality (Knopf Doubleday Publishing Group, New York, 2007).
Cunden, F. D., Di Martino, S., Facchi, P. & Florio, G. Spatial separation and entanglement of identical particles. Int. J. Quant. Inf. 12, 1461001 (2014).
Herbut, F. How to distinguish identical particles. Am. J. Phys. 69, 207–217 (2001).
Roch, N. et al. Observation of measurement-induced entanglement and quantum trajectories of remote superconducting qubits. Phys. Rev. Lett. 112, 170501 (2014).
Modi, K., Brodutch, A., Cable, H., Paterek, T. & Vedral, V. The classical-quantum boundary for correlations: Discord and related measures. Rev. Mod. Phys. 84, 1655–1707 (2012).
Iemini, F., Debarba, T. & Vianna, R. O. Quantumness of correlations in indistinguishable particles. Phys. Rev. A 89, 032324 (2014).
Xu, J.-S. et al. Experimental recovery of quantum correlations in absence of system-environment back-action. Nature Commun. 4, 2851 (2013).
Orieux, A. et al. Experimental on-demand recovery of quantum entanglement by local operations within non-Markovian dynamics. Sci. Rep. 5, 8575 (2015).
Barends, R. et al. Digital quantum simulation of fermionic models with a superconducting circuit. Preprint at arXiv:1501.07703 (2015).
Lamata, L., Mezzacapo, A., Casanova, J. & Solano, E. Efficient quantum simulation of fermionic and bosonic models in trapped ions. EPJ Quantum Technology 1, 9 (2014).
Crespi, A. et al. Particle statistics affects quantum decay and Fano interference. Phys. Rev. Lett. 114, 090201 (2015).
Sarovar, M., Ishizaki, A., Fleming, G. R. & Whaley, K. B. Quantum entanglement in photosynthetic light harvesting complexes. Nature Phys. 6, 462 (2010).
Acknowledgements
R.L.F. and G.C. acknowledge Università degli Studi di Palermo for financial support of this publication (voce di bilancio CA.C.B. 02.03.03. del 30/06/2015).
Author information
Authors and Affiliations
Contributions
G.C. devised the idea of a state-based identical particle description without name labels. R.L.F. devised the application to quantum entanglement. R.L.F. and G.C. developed the theory and wrote the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Lo Franco, R., Compagno, G. Quantum entanglement of identical particles by standard information-theoretic notions. Sci Rep 6, 20603 (2016). https://doi.org/10.1038/srep20603
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep20603
This article is cited by
-
Entanglement monogamy in indistinguishable particle systems
Scientific Reports (2023)
-
Method of constructing the entangled state of the particle system
Pramana (2023)
-
Dynamics of quantum correlations under correlated noisy channels
Optical and Quantum Electronics (2023)
-
Arbitrary entanglement of three qubits via linear optics
Scientific Reports (2022)
-
Directly proving the bosonic nature of photons
Nature Photonics (2021)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.

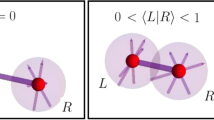



 equal to the localized ground state
equal to the localized ground state  of left well and one particle is in the (blue) mode
of left well and one particle is in the (blue) mode  which is a combination of
which is a combination of  and of the localized mode
and of the localized mode  of the right well, with
of the right well, with  .
.