Abstract
We introduce and analyze a one-dimensional quantum walk with two time-independent rotations on the coin. We study the influence on the property of quantum walk due to the second rotation on the coin. Based on the asymptotic solution in the long time limit, a ballistic behaviour of this walk is observed. This quantum walk retains the quadratic growth of the variance if the combined operator of the coin rotations is unitary. That confirms no localization exhibits in this walk. This result can be extended to the walk with multiple time-independent rotations on the coin.
Similar content being viewed by others
Introduction
Quantum walks (QWs) are valuable in diverse areas of science, such as quantum algorithms1,2,3,4,5,6, quantum computing7,8,9, transport in biological systems10,11 and quantum simulations of physical system and important phenomena such as Anderson localization12,13,14,15,16,17,18,19, Bloch oscillation20,21,22,23 and non-trivial topological structure24,25,26.
We study one possible route to the localization effect for the QW on the line: the use of multiple-rotation on the coin in order to change interference pattern between paths27. We find exact analytical expressions for the time-dependence of the first two moments  and
and  , show the behaviour of QWs with two time-independent rotations on the coin and present that a ballistic behaviour instead of localization is observed. This result can be extended to the walk with multiple time-independent rotations on the coin.
, show the behaviour of QWs with two time-independent rotations on the coin and present that a ballistic behaviour instead of localization is observed. This result can be extended to the walk with multiple time-independent rotations on the coin.
Results
The unitary operator for single-step of this QW with two time-independent rotations on the coin is

The two rotations on the coin shown in Fig. 1a

where  is the vector of Pauli matrices. The rotations are followed by a conditional position shift operator
is the vector of Pauli matrices. The rotations are followed by a conditional position shift operator

where  and
and  are two orthogonal projectors on the Hilbert space of the coin spanned by
are two orthogonal projectors on the Hilbert space of the coin spanned by  ,
,  and
and  are applied on the walker’s position. One can identify the eigenvectors
are applied on the walker’s position. One can identify the eigenvectors  of S and
of S and  ,
,

with eigenvalues

Here a discrete-time QW is considered as a stroboscopic realization of static effective Hamiltonian, defined via the single-step evolution operator

where  is the time it takes to carry out one step and we set
is the time it takes to carry out one step and we set  in the followings. The evolution operator for N steps is given by
in the followings. The evolution operator for N steps is given by  . For the general rotations in Eq. (2), the effective Hamiltonian can be written as
. For the general rotations in Eq. (2), the effective Hamiltonian can be written as

where the quasi-energy (Fig. 1b)

and the unit vector 

The inverse Fourier transformation is  . The initial state of the walker + coin system can be written as
. The initial state of the walker + coin system can be written as  , where the original position state of the walker is
, where the original position state of the walker is  . In the k basis, the evolution operator
. In the k basis, the evolution operator  becomes
becomes

where

is a  unitary matrix with the matrix elements
unitary matrix with the matrix elements

At time t (the time t is proportional to the step number N), the walker + coin state evolves to

The probability for the walker to reach a position x at time t is

where  , the group velocity of the walker
, the group velocity of the walker  . To determine if there is localization effect, we care more about the position variance and the dependence of the variance on time. Thus we restrict our interest to the moments of the distribution
. To determine if there is localization effect, we care more about the position variance and the dependence of the variance on time. Thus we restrict our interest to the moments of the distribution

With the formula of the delta function  , the expression of the mth moment is rewritten as
, the expression of the mth moment is rewritten as

Similar to a Hadamard coined walk28, one can find the eigenvectors  of
of  and corresponding eigenvalues
and corresponding eigenvalues  . We can expand the initial coin state
. We can expand the initial coin state  . With
. With  29, we only keep the diagonal non-oscillatory terms and obtain
29, we only keep the diagonal non-oscillatory terms and obtain

For non-degenerate unitary matrix  , except for the diagonal non-oscillatory terms, most of the terms are oscillatory, which average to zero in the long-time limit29.
, except for the diagonal non-oscillatory terms, most of the terms are oscillatory, which average to zero in the long-time limit29.
Similarly, the second moment is obtained

From Eqs. (8) and (11), we can see the spectrum  of
of  is non-degenerate. Even for degenerate
is non-degenerate. Even for degenerate  one can modify Eqs. (17) and (18) to include appropriate cross terms, which does not change the dependence of the position variance on time.
one can modify Eqs. (17) and (18) to include appropriate cross terms, which does not change the dependence of the position variance on time.
Generically, in the long-time limit, for a unitary coin the first moment of the QW undergoes a linear drift and the variance grows quadratically with time. There is a special case—the  coined QW, i.e.,
coined QW, i.e.,  , in which the eigenstates of
, in which the eigenstates of  are
are  and
and  , resulting in
, resulting in  (for
(for  . Thus the variance of the
. Thus the variance of the  coined QW does not depend on time.
coined QW does not depend on time.
In the two rotations case, the combination operation of two rotations on the coin  shown in Eq. (11) is unitary. Thus for arbitrary choices of parameters θ and
shown in Eq. (11) is unitary. Thus for arbitrary choices of parameters θ and  the position variance of the QW with two time-independent rotations on the coin grows quadratically and the behaviour of the QW is ballistic. Therefore, a second coin rotation does not change the behaviour of QW from a ballistic spread to localization.
the position variance of the QW with two time-independent rotations on the coin grows quadratically and the behaviour of the QW is ballistic. Therefore, a second coin rotation does not change the behaviour of QW from a ballistic spread to localization.
The asymptotic analysis of the behaviour of this QW with two time-independent coin rotations can be extended to more general QW with more time-independent rotations on the coin. Once the combined operator of the multiple-rotation on the coin is unitary, the position variance grows quadratically with time and this QW shows ballistic behaviour. No localization effect occurs.
This walk is homogeneous in either spatial or temporal space. The coin rotations do not cause inhomogeneity in this walk which usually leads to interesting localization effect.
Discussion
In summary, we study the QW with two time-independent rotations on the coin through the analytical solutions for the time dependence of the position variance. The asymptotic result can be extended to the walk with multiple time-independent rotations on the coin. As long as the combination of the multi-rotations is unitary, the variance grows quadratically with time and the QW shows ballistic behaviour. No localization effect is observed in this QW. Although the fact that two topics—QWs and localization effect meet, is fascinating and opens the door to rich theoretical and experimental investigation of quantum phenomena. Thus not only the investigation on simulating localization with QWs but also the study on the limitations on localization in quantum walk are important and worthy of attention. Our research exactly gives insight into limitations on localization.
Additional Information
How to cite this article: Xue, P. et al. A one-dimensional quantum walk with multiple-rotation on the coin. Sci. Rep. 6, 20095; doi: 10.1038/srep20095 (2016).
References
Kempe, J. Quantum random walks: An introductory overview. Cont. Phys. 44, 307–327 (2003).
Ambainis, A. Quantum walks and their algorithmic applications. Int. J. Quant. Inf. 1, 507–518 (2003).
Childs, A. M. et al. Exponential algorithmic speedup by quantum walk. Proc. ACM Symp. on Theory of Computing (STOC 2003) pp 59-68 (2003).
Shenvi, N., Kempe, J. & Whaley, K. B. Quantum random-walk search algorithm. Phys. Rev. A 67, 052307 (2003).
Berry, S. D. & Wang, J. B. Quantum-walk-based search and centrality. Phys. Rev. A 82, 042333 (2010).
Franco, C. & Di, McGettrick, M. & Busch, Th. Mimicking the probability distribution of a two-dimensional Grover walk with a single-qubit coin. Phys. Rev. Lett. 106, 080502 (2011).
Childs, A. M. Universal computation by quantum walk. Phys. Rev. Lett. 102, 180501 (2009).
Childs, A. M., Gosset, D. & Webb, Z. Universal computation by multiparticle quantum walk. Science 339, 791–794 (2013).
Lovett, N. B., Cooper, S., Everitt, M., Trevers, M. & Kendon, V. Universal quantum computation using the discrete-time quantum walk. Phys. Rev. A 81, 042330 (2010).
Lloyd, S. Quantum coherence in biological systems. J. Phys.: Conf. Series302, 012037 (2011).
Hoyer, S., Sarovar, M. & Whaley, K. B. Limits of quantum speedup in photosynthetic light harvesting. New J. Phys. 12, 065041 (2010).
Anderson, P. W. Absence of diffusion in certain random lattices. Phys. Rev. 109, 1492 (1958).
Wójcik, A. et al. Trapping a particle of a quantum walk on the line. Phys. Rev. A 85, 012329 (2012).
Konno, N. Localization of an inhomogeneous discrete-time quantum walk on the line. Quant. Inf. Proc. 9, 405–418 (2010).
Shikano, Y. & Katsura, H. Localization and fractality in inhomogeneous quantum walks with self-duality. Phys. Rev. E 82, 031122 (2010).
Zhang, R., Xue, P. & Twamley, J. One-dimensional quantum walks with single-point phase defects. Phys. Rev. A 89, 042317 (2014).
Schreiber, A. et al. Decoherence and disorder in quantum walks: from ballistic spread to localization. Phys. Rev. Lett. 106, 180403 (2011).
Crespi, A. et al. Localization properties of two-photon wave packets. Nat. Photonics 7, 322–328 (2013).
Xue, P., Qin, H. & Tang, B. Trapping photons on the line: controllable dynamics of a quantum walk. Sci. Rep. 4, 4825 (2014).
Wójcik, A., Łuczak, T., Kurzyński, P., Grudka, A. & Bednarska, M. Quasiperiodic dynamics of a quantum walk on the line. Phys. Rev. Lett. 93, 180601 (2004).
Bañuls, M. C., Navarrete, C., Pérez, A., Roldán, E. & Soriano, J. C. Quantum walk with a time-dependent coin. Phys. Rev. A 73, 062304 (2006).
Genske, M. et al. Electric quantum walks with individual atoms. Phys. Rev. Lett. 110, 190601 (2013).
Xue, P., Qin, H., Tang, B. & Sanders, B. C. Observation of quasiperiodic dynamics in a onedimensional quantum walk of single photons in space. New J. Phys. 16, 053009 (2014).
Kitagawa, T., Rudner, M. S., Berg, E. & Demler, E. Exploring topological phases with quantum walks. Phys. Rev. A 82, 033429 (2010).
Asbóth, J. K. Symmetries, topological phases and bound states in the one-dimensional quantum walk. Phys. Rev. B 86, 195414 (2012).
Kitagawa, T. et al. Observation of topologically protected bound states in photonic quantum walks. Nat. Commun. 3, 882 (2012).
Xue, P. et al. Experimental quantum-walk revival with a time-dependent coin. Phys. Rev. Lett. 112, 120502 (2014).
Nayak, A. & Vishwanath, A. Quantum walk on the line. arXiv:quant-ph/0010117.
Brun, T. A., Carteret, H. A. & Ambainis, A. Quantum to classical transition for random walks. Phys. Rev. Lett. 91, 130602 (2003).
Acknowledgements
This work has been supported by National Natural Science Foundation of China, Grant Nos. 11174052 and 11474049 and the CAST Innovation fund.
Author information
Authors and Affiliations
Contributions
P.X. developed the theory, supervised R.Z., H.Q., X.Z., Z.B. and J.L. and wrote most of the paper. R.Z. and H.Q. wrote the code. X.Z. and Z.B. checked the numerical simulations. All authors reviewed the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Xue, P., Zhang, R., Qin, H. et al. A one-dimensional quantum walk with multiple-rotation on the coin. Sci Rep 6, 20095 (2016). https://doi.org/10.1038/srep20095
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep20095
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.


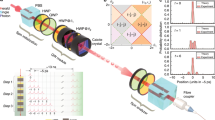


 and
and  in red lines or
in red lines or  in blue lines. The second rotation along x axis allows to close the quasi-energy gap for
in blue lines. The second rotation along x axis allows to close the quasi-energy gap for  .
.