Abstract
The concept of ‘fragility’ constitutes a central point of the glass transition science serving as the ‘universal’ metric linking previtreous dynamics of qualitatively distinct systems. Finding the fundamental meaning of fragility is the ‘condicio sine qua’ for reaching the long expected conceptual breakthrough in this domain. This report shows that fragility is determined by the ratio between two fundamental process energies, viz. the activation enthalpy and activation energy. The reasoning, avoiding any underlying physical model, is supported by the experimental evidence ranging from low molecular weight liquids and polymers to plastic crystals and liquid crystals. All these lead to the new general scaling plot for dynamics of arbitrary glass former. The limited adequacy of broadly used so far semi-empirical relationships between fragility and the activation energy is shown. Results presented remain valid for an arbitrary complex system and collective phenomena if their dynamics is described by the general super-Arrhenius relation.
Similar content being viewed by others
Introduction
Glass transition constitutes one of grand challenges of condensed and soft matter physics as well as modern materials science1,2,3,4, where a long-expected fundamental breakthrough could lead to innovative implementations ranging from silicate glasses5 and plastics6 to pharmaceuticals7 and foods8. The ultimate progress in this area is also important for biotechnological9, geophysical10, metallurgical11 and electronic devices12 implementations. Notable is the fact that glass transition physics is considered as one of key references for collective phenomena science, aimed to discover properties emerging from complex correlations13.
Of particular interest of the glass transition research is the identification of universal features in the previtreous dynamic regime shared amongst a surprising variety of systems including low molecular weight liquids, polymers, liquids crystals, plastic crystals, colloids, metallic alloys, silicates, spin glasses, etc2,3,4,5. A key metric linking so distinct glass formers was introduced by Austen Angell14, basing on a master plot of log10η(T) and/or log10τ(T) versus Tg/T where η(T) stands for viscosity, τ(T) for structural (primary) relaxation time and Tg is the glass temperature14,15. This was possible due to the empirical normalization assumption for the glass transition temperature η(Tg) = 1013 Poise and τ(Tg) = 100 s. Subsequently, a metric describing the slope for T → Tg, called ‘fragility’, was proposed14,15:

The fragility index m describes the degree of shifting from the basic Arrhenius dynamics to the super-Arrhenius (SA) one, described by the general form2:

where T > Tg, R denotes the gas constant and ΔEa(T) the apparent activation energy. The basic Arrhenius dependence is restored for ΔEa(T) = Ea = const.
There are two general types of glass formation defined by the fragility metric: (i) ‘fragile’ systems with highly SA dynamics (m > 50) and (ii) ‘strong’ ones, with close-to-Arrhenius behavior (m < 30)2,14,15. The basic Arrhenius behavior τ(T) = τ0 exp(Ea/RT) is associated with the minimal value of the fragility index and most often related to m = log10(τ(T = Tg)) −log10 τ0 = 2 + 14 = 16, i.e. assuming for the prefactor τ0 = 10−14 s in the SA eq. (2)2,15. Notwithstanding, for silicate liquids extremely strong SA behavior with a minimal m = 14.93 was found16. Experimental estimations of the prefactor in the SA equation ranges from ~10−11 s to even ~10−18 s2,17, what indicates on the system-dependent minimal fragility.
Qualitative mapping of the previtrous increase of relaxation times or viscosity onto a single chart has led to the concept of fragility, becoming a focal point for research in glass transition physics2,3. The most important appeared as the link between two basic properties, viz. fragility and the activation energy2,3,18,19,20. One may claim that the ultimate explanation of this problem is the “condicio sine qua” for reaching the conceptual breakthrough in glass transition physics2,3. Surprisingly, despite decades of studies the situation is puzzling.
The first and broadly implemented up to now dependence20,21,22,23,24,25,26 was proposed by Boehmer et al.15 in 1993:

In 2004, Novikov and Sokolov27 proposed yet another relation, supported by experimental evidence for a set of glass forming liquids27,28:

This report presents the critical discussion of eqs. (3) and (4) and shows that their validity is casual. Subsequently, it presents the lacking so far fundamental link between fragility and fundamental process energies, viz. the activation energy and the activation enthalpy. The new, ‘ultimate’, scaling relation linking fragility and the activation energy has been also derived. Analytic results are supported by the clear experimental evidence for a broad range of glass formers.
Results
The new insight into fragility of glass formers
In Refs. 29, 30 the new approach for the insight into dynamics of the previtreous domain, based solely on the SA eq. (2) and the metric describing relative changes of the apparent activation energy was introduced:

The analysis in Refs. 29, 30 was possible due to the innovative way of determining ΔEa(T), which avoids the biasing impact of generally unknown prefactor τ0 in the SA eq. (2). This model-free approach lead to a set of notable findings including the limited fundamental adequacy of the Vogel-Fulcher-Tamman (VFT)31,32,33 equation, identifying the role of local symmetry in glass formation and showing the ultimate way of “dynamic” estimation of the ideal glass transition temperature29,30.
Linking the SA eq. (2) and eq. (5) for the apparent activation energy temperature index one obtains:

where the identity d(1/T) = (−(1/T)dT)(1/T) = −(d ln T)(1/T) was used, ΔHa(T) denotes the apparent activations enthalpy (see also Ref. 17 and Suppl. Info of Refs. 29, 30 where clear derivations of the relationship between ΔEa(T), ΔHa(T) and d ln τ(T)/d(1/T) are given),  .
.
The above dependence directly yields:

Figure 1 shows that eq. (7) can serve as the base for the new “universal scaling plot” for the previtreous dynamics of arbitrary glass former. It includes representatives from LMW, P, ODIC and LC categories29,30,34,35,36,37,38,39. Notable is the correlation with the classical ‘Angell’ plot2,3,14,15, presented in the inset for the same systems. For both plots the increase of curvature indicates the rise of fragility. However, in the main plot fragility it is directly determined by the ratio of fundamental process energies, namely:

which lead to:

The onset of the Arrhenius behavior is associated with the negligible curvature and almost horizontal behavior for ΔHa(T)/ΔEa(T) ≪ 1 in Fig. 1.
The evolution of the ratio between the apparent activation enthalpy and energy for selected glass forming systems.
The inset shows the classic “Angell plot”2,14,15 for τ(T) experimental data, constituting the base for determining non-biased ratio of process energies in the main plot. For basic data see Table 1.
The classical fragility index m (eq. (1)) is still explained as the “formal” parameter, namely the slope at the ‘Angell’ plot2,14,15. The new fragility parameter M = IDO(Tg) gives directly value between two basic process energies, which are then key fundamental features determining the value of fragility. Using eqs. (1), (2) and (9) one obtains the link between the ‘classical’ (m) and new (M) fragility metrics:

where the constant C = 2 − log10(τ0) = 13–18.
The relationship between m and IDO(Tg) was indicated earlier by Hecksher et al.40, but without an explanation of the physical meaning of IDO(Tg).
The experimental confirmation of the behavior predicted by eq. (10) is given in Figure 2. It is notable that eq. (10), showing also the link of m to basic process energies, indicates also the uncertainty introduced by the prefactor τ0 or η0 in SA eq. (2). The summary of characteristics for aforementioned experimental systems is given in Table 1.
The experimental tests of the relationship between the ‘classical’ fragility metric m and the new metric M = ΔHa(Tg)/ΔEa(Tg) − 1.
Results are for selected glass forming LMW, P, ODC and LC systems (see Table 1).
However, the most fundamental eqs. (1) and (2) directly indicate that the increasing SA behavior is associated with the rising nonlinearity at the “Arrhenius-type” plot ln(ΔEa(T)) vs. 1/T. Fig. 3 presents such plot, revealing the lack of a correlation between the increasing curvature of the apparent activation energy, coupled to rising fragility m and the value of ΔE(Tg). This is in clear disagreement with mentioned above basic prediction (eq. (3)). Moreover, the simply linearization based on eqs. (1) and (2) yields  , i.e. the linear function with the intercept at cte = ln[R ln 10] > 0 and the directional factor b = 1. Such prediction is anti-correlated with experimental data, as shown in the inset in Fig. 3 via the dashed line. Consequently, the used so far basic link between the activation energy and fragility ΔEa(Tg) = RTgmln10, i.e. (eq. (3))2,14,16,20,21,22,23,24,25,26, is inherently invalid.
, i.e. the linear function with the intercept at cte = ln[R ln 10] > 0 and the directional factor b = 1. Such prediction is anti-correlated with experimental data, as shown in the inset in Fig. 3 via the dashed line. Consequently, the used so far basic link between the activation energy and fragility ΔEa(Tg) = RTgmln10, i.e. (eq. (3))2,14,16,20,21,22,23,24,25,26, is inherently invalid.
Degree of nonlinearity at “Arrhenius-type” plotted as ln(ΔEa(T)) vs. Tg/T for representative glass formers.
The figure indicates the lack of correlation between increasing curvature, coupled to fragility and the value of ΔEa(Tg). The clear disagreement with eq. (3) is stressed by the inset: the blue, dashed line is related to eq. (3) and the solid, black line is based on the MFR.
However, the simple analysis based solely on general eqs. (1) and (2) and eq. (9), derived above yields (see also Methods section):

Figure 4 shows that the implementation of eq. (11) orders “chaotically scattered” curves in the main part of Fig. 3. It also leads to the superior agreement with experimental data given the inset in Fig. 3 (the solid line). Consequently, eq. (11) can be considered as the new ultimate link between the activation energy and fragility, valid for an arbitrary glass former.
The “universal” scaling plot for activation energies in representative glass formers.
The apparent activation energy is obtained by MFR29,30 procedure (see Methods) which is rescaled at Tg using eq. (11). The figure shows the correlation between increasing curvature, indicating the rise of fragility and ΔEa(Tg). For basic data see also Table 1.
Novikov and Sokolov27,28 proposed yet another relation linking fragility (determined for Tg/T → 1) with the activation energy but this time taken for Tg/T → 0, viz. eq. (4). For explaining its meaning, let's recall that in the low temperature domain (Tg/T → 1) the increase of curvature and the slope in the ‘Angell’ plot14,15 is linked to increasing fragility m. For the same plot, log10 η(T) or log10 τ(T) vs. Tg/T, in the high temperature domain the decreasing slope is related to increasing value of m. This behavior is illustrated in Figure 5, showing that Novikov and Sokolov27,28 relation ΔEa(Tg/T → 0) ~ 1/m (eq. (4)) results from the construction of the ‘Angell’ plot. The underlying assumption of eq. (4) is also the “universal” value of the viscosity (log10 η0 = −4) or for relaxation time log10 τ0 = −14 for Tg/T → 0. This border values are considered as hypothetical universal values of the prefactor in the SA eqs. (1).
The schematic plot explaining the source of the hypothetical correlation between fragility m and the activation energy for the high temperature border case Tg/T → 0.
Fragility is related to the slope for Tg/T → 1, in the ultraviscous, “low temperature”, dynamic domains (indicated by colored symbols). Lines are for the high temperature domain terminating at Tg/T → 0. The plot recalls the basis of Novikov and Sokolov27,28 relationship linking fragility and activation energy in the high temperature domain (eq. (4)). The presumable “universal” high temperature (Tg/T → 0) values of prefectors in SA eq. (2) are η0 = 10−4 Poise or τ0 = 10−14 s2.
However, the existence of such universal values of prefactors can be questioned, particularly when taking into account different categories of glass formers, as discussed above. All these indicate on the necessity of a supplementary analysis, related to: (i) the activation energy determined without the biasing impact of the prefactor and (ii) the impact of the qualitative differences between dynamic the high- and low- temperatures domains. The latter is particularly important, because eq. (4) suggests direct causal link between different dynamic domains.
Following the above discussion, as well as the evidence from Refs. 2, 29, 30, one can indicate following basic features of the ultraslowing/ultraviscous domain:
-
There are no glass forming systems in the ultraviscous/ultrasowing domain where ΔEa(T) decreases on cooling, i.e.
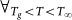 ∂ΔEa(T)/∂(1/T) ≥ 0 and IDO(T) ≥ 0.
∂ΔEa(T)/∂(1/T) ≥ 0 and IDO(T) ≥ 0. -
For extremely strong glass formers ΔEa(T) ~ ΔHa(T) for the whole low temperature dynamic domain. In the case of the Arrhenius behavior ΔEa(T) = ΔHa(T) = const.
-
In the ultraviscous/ultraslowing domain always ΔHa(T) > ΔEa(T) and in the vicinity of Tg even ΔHa(T) ≫ ΔEa(T). This is particularly evident for fragile ultraslowing and/or ultraviscous systems.
Discussion
The fragility and the activation energy are the most fundamental characteristics of glass transition. One can expect that a conceptual progress in this challenging area of condensed matter physics needs the unequivocal relationship between these quantities. However, this basic problem appeared to be surprisingly difficult, viz. the title of the recent Ref. 42: “The fragility and other properties of glass-forming liquids: Two decades of puzzling correlations”.
The current report presents the first ever evidence of the unequivocal link between fragility and ratio of two basic process energies: the activation energy and the activation enthalpy in the low temperature ultraviscous/ultraslowing dynamic domain. It is worth recalling that the activation energy ΔEa(T) is associated with the energy barrier necessary to boost a process, i.e. a transition state's free energy (the energy barrier) minus the energy of substrate's. This report shows the direct link of fragility to the ratio of these energies: m = (2 − log10 τ0)(ΔHa(Tg)/ΔEa(Tg)), but affected by the uncertainty associated with the SA prefactor τ0 (or η0). This biasing impact can be avoided for the new fragility metric M = IDO(Tg) = ΔHa(Tg)/ΔEa(Tg) − 1, ranging from M = 0 (the basic Arrhenius case) to M > 10 for strongly SA dynamics. The activation enthalpy can be easily calculated via  or
or  and the activation energy via the recently proposed model free route procedure (see Methods and Refs. 29, 30). This report shows that in the ultraviscous/ultraslowing domain always ΔHa(T) > ΔEa(T) and in the immediate vicinity of Tg even ΔHa(T) ≫ ΔEa(T). We emphasize this issue, since in a number of research reports the erroneous assumption that
and the activation energy via the recently proposed model free route procedure (see Methods and Refs. 29, 30). This report shows that in the ultraviscous/ultraslowing domain always ΔHa(T) > ΔEa(T) and in the immediate vicinity of Tg even ΔHa(T) ≫ ΔEa(T). We emphasize this issue, since in a number of research reports the erroneous assumption that  near the glass transition has been used20,21,22,23,43,44,45,46,47,48,49.
near the glass transition has been used20,21,22,23,43,44,45,46,47,48,49.
One of the most attracting questions regarding fragility is its maximal value. In Ref. 50 value m ≈ 175 was indicated as the maximal possible fragility, what is related to M ≈ 10. However, earlier m ≈ 214 was noted as the indicator of the most SA dynamics51. Basing on this report, recalling the basic Adam-Gibbs (AG) theory2,52 and Refs. 29, 30 the following general dependence for the apparent activation energy temperature index can be obtained (see Methods section):

The latter dependence and eq. (9) yields:

For example, for glass formers with rod-like molecules and the clear uniaxial, orientational symmetry n ≈ 1.6 and Tg − TN ≈ 10 with TN ≈ 300 K (see Suppl. Info to Ref. 30) one obtains m ≈ 280!
Following the given report and Refs. 29, 30 one can postulate that the transformation of τ(T) or η(T) experimental data to IDO(T) representation can yield all basic characteristics of previtreous dynamics, basing solely on inherently unambiguous linear regression fit, namely: (i) the local symmetry related parameter  , (ii) the extrapolated singular temperature for which
, (ii) the extrapolated singular temperature for which  and (iii) the fragility metric M = IDO(Tg), linked to basic process energies. Knowing (TN,M,n) (see Fig. 6 in Methods section) and the fragility m (from the “Angell” plot) the unambiguous estimation of τ0 or η0 prefactors is also possible.
and (iii) the fragility metric M = IDO(Tg), linked to basic process energies. Knowing (TN,M,n) (see Fig. 6 in Methods section) and the fragility m (from the “Angell” plot) the unambiguous estimation of τ0 or η0 prefactors is also possible.
The temperature scaling behavior of the reciprocal of the apparent activation temperature index in glass forming liquid crystalline n-octylocycyanobiphenyl (8*OCB).
The ultraviscous domain extends between Tg < T(≈ 100 K) < TB, where the latter denotes the dynamic crossover temperature2. Locations of the singular temperature TN and the extrapolation down to T = 0 as well as the method of calculating the experimental dependence  are shown29,30.
are shown29,30.
One of still mysterious features of the glass transition are different distances between the glass temperature (Tg) and the extrapolated Kauzmann temperature (TK) in various glass formers2,53. Following the finding the TN = TK (see Ref. 30 and Methods section) and eq. (13) one obtains M/n = TN/(Tg − TN) = M/n, i.e. the relative distance between TK( = TN) and Tg depends solely on the fragility (M) and symmetry related (n). It is notable that eq. (13) makes it possible also unequivocal calculation the τ0 for the SA eq. (2), avoiding any “ersatz equations”, like the VFT2 one used so far.
Worth noting is the relationship of the new fragility parameter M to the one introduced by Doremus54, who applied the empirical finding that at high temperature (HT) the activation energy low ( , originally denoted as QL is low) whereas at low temperatures prior to the vitrification its value is high (QH,
, originally denoted as QL is low) whereas at low temperatures prior to the vitrification its value is high (QH,  ). This lead to the Doremus fragility metric
). This lead to the Doremus fragility metric  with RD < 2 for “strong” glass formers and RD > 2 for “fragile” ones2,54. In the Doremus model QH = Hd + Hm, where Hd is associated with enthalpy of formation of broken bonds and Hm is responsible for motions. At high temperature Hm dominates and QL = Hm. Such picture results from the fact that Doremus introduced the model for ultraviscous silica, but its extension to other glass formers can be explained by the formations of bond-ordering local structures or heterogeneities. This led Doremus to the double-exponential function for description of the viscous flow, at least in silicates54. This report correlates with some fundamental of Doremus model54, although the analysis avoids any model assumptions, basing solely on the general Super-Arrhenius equation (eq. (2)).
with RD < 2 for “strong” glass formers and RD > 2 for “fragile” ones2,54. In the Doremus model QH = Hd + Hm, where Hd is associated with enthalpy of formation of broken bonds and Hm is responsible for motions. At high temperature Hm dominates and QL = Hm. Such picture results from the fact that Doremus introduced the model for ultraviscous silica, but its extension to other glass formers can be explained by the formations of bond-ordering local structures or heterogeneities. This led Doremus to the double-exponential function for description of the viscous flow, at least in silicates54. This report correlates with some fundamental of Doremus model54, although the analysis avoids any model assumptions, basing solely on the general Super-Arrhenius equation (eq. (2)).
It is also notable that results of the given report and empirical findings in Refs. 29, 30 lead to surprisingly simple form of relative changes of process energies in the ultraviscous/ultraslowing domain ΔEa(T)/[ΔHa(T) − ΔEa(T)] = a + bT with a ≠ 0 and b ≠ 0.
Concluding, this reports presents the link between fragility and basic process energies, in ultraviscous/ultraslowing glass forming materials lacking so far. However, results presented above are also significant for complex systems and collective phenomena if their dynamics is described by the general super-Arrhenius relation.
Methods
The analysis of experimental data and the model-free route (MFR) method
The “model-free” route procedure introduced in Refs. 29, 30 is a novel approach for getting insight into previtreous dynamics based on the transformation of basic structural relaxation time (τ(T)) or viscosity (η(T)) experimental data to apparent activation energy temperature index form, the magnitude first proposed for glass formers by Dyre and Olsen (DO)40 via:

The apparent activation energy  is determined from τ(T) and η(T) experimental data via the solution of the differential equation resulting from the general SA eq. (2)29,30:
is determined from τ(T) and η(T) experimental data via the solution of the differential equation resulting from the general SA eq. (2)29,30:

where the apparent activation enthalpy is given by  29.
29.
This way of determining  was only recently introduced in Refs. 29, 30. Previously, the apparent activation energy was calculated from the SA eq.(2) as ΔEa(T) = RT ln (τ(T)/τ0)2,40,41. and then it was inherently biased by the generally unknown estimation of τ0 prefactor2. In practice, a “universal” value of τ0 = 10−14 s was most commonly assumed2,40,41. An inherent advantage of the MFR approach for determining ΔEa(T) and IDO(T) includes also the application of a numerical filtering procedure based on Savitzky-Golay principle29,30.
was only recently introduced in Refs. 29, 30. Previously, the apparent activation energy was calculated from the SA eq.(2) as ΔEa(T) = RT ln (τ(T)/τ0)2,40,41. and then it was inherently biased by the generally unknown estimation of τ0 prefactor2. In practice, a “universal” value of τ0 = 10−14 s was most commonly assumed2,40,41. An inherent advantage of the MFR approach for determining ΔEa(T) and IDO(T) includes also the application of a numerical filtering procedure based on Savitzky-Golay principle29,30.
In Refs. 29, 30 the MFR have been implemented for a set of 55 glass forming systems, ranging from low molecular weight liquids (LMW) and polymers (P) to liquid crystal (LC), plastic crystal (ODIC) and spin glasses (SGL), in the previtreous domain. The analysis revealed a surprisingly simple pattern for the previtreous dynamics: 1/IDO(T) = aT + b, with a ≠ 0 and b ≠ 0 for all mentioned data sets. This led to the derivation of the new generalized configurational entropy equation Sc(T) = S0(1 − (TN/T))n, where the power exponent is determined as  and TN is the singular temperature estimated via IDO(T = TN)−1 = 029,30. Recently, basing on the MFR, the clear coincidence between the ideal glass (Kauzmann) temperature TK and TN, i.e. TK = TN, was found30. The analysis carried out in Refs. 29, 30 revealed that the parameter ranges between 0.18 < n < 1.53, where the lower limit is for systems with the clear positional symmetry (PS) and the higher one for systems with clear orientational, uniaxial symmetry (OS). The dynamics of PS and OS glass formers is relatively well portrayed by the critical-like equation29,38. The third characteristic case is for systems where n = 1 (no-symmetry). Only in this case the application of the popular Vogel-Fulcher-Tammann (VFT)2 equation is suitable29,30. Consequently, the fundamental justification of the VFT relation is limited to a small group of glass formers and otherwise (i.e. for n ≠ 1) it can be considered solely as an effective fitting tool.
and TN is the singular temperature estimated via IDO(T = TN)−1 = 029,30. Recently, basing on the MFR, the clear coincidence between the ideal glass (Kauzmann) temperature TK and TN, i.e. TK = TN, was found30. The analysis carried out in Refs. 29, 30 revealed that the parameter ranges between 0.18 < n < 1.53, where the lower limit is for systems with the clear positional symmetry (PS) and the higher one for systems with clear orientational, uniaxial symmetry (OS). The dynamics of PS and OS glass formers is relatively well portrayed by the critical-like equation29,38. The third characteristic case is for systems where n = 1 (no-symmetry). Only in this case the application of the popular Vogel-Fulcher-Tammann (VFT)2 equation is suitable29,30. Consequently, the fundamental justification of the VFT relation is limited to a small group of glass formers and otherwise (i.e. for n ≠ 1) it can be considered solely as an effective fitting tool.
The example of analysis employing the MFR analysis, based on transformed τ(T) experimental data in supercooled liquid crystalline n-octyloxycyanobiphenyl (8*OCB), is shown in Fig. 6. The way of determining the basic parameters is indicated.
Values of primary relaxation times τ(T) were determined as the reciprocal of the peak frequency of ε″(f) loss curve, obtained from broad band dielectric spectroscopy measurement (see Refs. 29, 30).
Derivation of the general form for the activation energy temperature index (eq. (12)
Recalling the Adam-Gibbs theory for glass transtion2,52, the apparent activation index can be written as29,30:

Substituting the new generalized configurational entropy (Ref. 29) Sc(T) = S0(1 − (TN/T))n, one obtains:

The above dependence make it possible to identify the impact of the entropic contribution in the anomalous behavior of the activation entropy temperature index.
Alternatively eq. (12) can be derived recalling the experimental finding that 1/IDO(T) = aT + b, where b = (−1/n) ≠ 0 and a = (1/nTN) ≠ 0 (the coefficient  and the singular temperature IDO(T = TN)−1 = 0)29,30, one obtains ΔHa(T)/ΔEa(T) = [TN(n − 1) + T]/(T − TN) and then IDO(T) = nTN/(T − TN).
and the singular temperature IDO(T = TN)−1 = 0)29,30, one obtains ΔHa(T)/ΔEa(T) = [TN(n − 1) + T]/(T − TN) and then IDO(T) = nTN/(T − TN).
References
Kennedy, D. 125 outstanding problems in all of science: What is the nature of the glassy state. Science 309, 83 (2005).
Ngai, K. L. Relaxation and Diffusion in Complex Systems (Springer, Berlin, 2011).
Stillinger, F. H. & Debenedetti, P. G. Glass transitions: thermodynamics and kinetics. Annu. Rev. Cond. Matt. Phys. 4, 262–270 (2013).
Jones, R. A. L. Soft condensed matter (Oxford Univ. Press., Oxford, 2002).
Mauro, J. C. Glass: The nanotechnology connection. Int. J. Appl. Glass Sci. 4, 64–75 (2013).
Thomas, S. A. & Zachariah, K. Polymer Processing and Characterization (Apple Academic Press, Boca Raton (FL, USA) 2012).
Jadhav, N. R., Gaikwad, V. L., Nair, K. J. & Kadam, H. M. Glass transition temperature: Basics and application in pharmaceutical sector. Asian J. Pharm. 3, 82–89 (2009).
Roos, Y. H. Glass transition temperature and its relevance in food processing. Ann. Rev. Food Sci.& Technol. 1, 469–496 (2010).
Starzonek, S., Rutkowska, M., Rzoska, S. J., Drozd-Rzoska, A. & Fonberg-Broczek et al. Dielectric spectroscopy of pressurized Saccharomyces cerevisiae, Food Biophysics 362, 1–8 (2014).
Poirier, J. P. Introduction to physics of the Earth interior (Cambridge Univ. Press, Cambridge, 2000).
Schroers, J. Condensed-matter physics: Glasses made from pure metals, Nature. 512, 142 (2014)
Singh, J. & Shimagawa, K. Advances in Amorphous semiconductors (CRC Press, London, 2003).
Mallamace, F. & Stanley, H. E. The physics of complex systems (New advances and perspectives) (IOS Press, Amsterdam, 2004).
Angell, C. A. Strong and fragile liquids, in: Relaxations in Complex Systems, eds. Ngai, K. L. & Wright, G. B. (National Technical Information Service, U.S. Dept. of Commerce, Springfield, 1985).
Boehmer, R., Ngai, K. L., Angell, C. A. & Plazek, J. D. Nonexponential relaxations in strong and fragile glass formers. J. Chem. Phys. 99, 4201–4209 (1993).
Zheng, Q., Mauro, J. C., Ellison, A. J., Potuzak, M. & Yue, Y. Universality of the high-temperature viscosity limit of silicate liquids, Phys. Rev. B 83, 212202 (2011).
Drozd-Rzoska, A. & Rzoska, S. J. On the derivative-based analysis for temperature and pressure evolution of dielectric relaxation times in vitrifying liquids. Phys. Rev. E 73, 041502 (2006).
Greer, A. L., Kelton, K. F. & Sastry, S. (eds.). Fragility of glassforming liquids (Hindustan Book Agency, Bengaluru, 2014).
Kivelson, S. A. & Tarjus, G. In search of a theory of supercooled liquids. Nature Mater. 7, 831–833 (2008).
Qin, Q., McKenna, G. B. Correlation between dynamic fragility and glass transition temperature for different classes of glass forming liquids, J. Non-Cryst. Solids 352, 2977–2985 (2006).
Moura Ramos, J. J., Correia, N. T., Taveira-Marques, R. & Collins, G. The activation energy at Tg and the fragility index of indomethacin, predicted from the influence of the heating rate on the temperature position and on the intensity of thermally stimulated depolarization current peak, Pharm. Res. 19, 1879–1884 (2002).
Zhao, J. & McKenna, G. B. The apparent activation energy and dynamic fragility of ancient ambers, Polymer 55, 2246–2253 (2014).
Soltan, A. S., Abu-Sehly, A. A., Joraid, A. A. & Alamri, S. N. The activation energy and fragility index of the glass transition in Se76Te21Sb3 chalcogenide glass, Thermochimica Acta 574, 73–78 (2013).
Vyazovkin, S., Sbirrazzuoli, N. & Dranca, I. Variation in activation energy of the glass transition for polymers of different dynamic fragility, Macromol. Chem. Phys. 207, 1126–1130 (2006).
Saffarini, G., Saiter, Ã. A., Garda, M. R. & Saite, J. M. Mean-coordination number dependence of the fragility in Ge–Se–In glass-forming liquids, Physica B 389, 275–280 (2007).
Aniya, M. Understanding the composition dependence of the fragility of AgI-Ag2O-MxOy glassy systems, IOP Conf. Series: Materials Science and Engineering 18, 112003 (2011).
Novikov, V. N. & Sokolov, A. P. Poisson's ratio and the fragility of glass-forming liquids, Nature 431, 961–963 (2004).
Novikov, V. N., Ding, Y., & Sokolov, A. P. Correlation of fragility of supercooled liquids with elastic properties of glasses, Phys. Rev. E 71, 061501 (2005).
Martinez-Garcia, J. C., Rzoska, S. J., Drozd-Rzoska, A. & Martinez-Garcia, J. A. universal description of ultraslow glass dynamics. Nat. Commun. 4, 1823–1830 (2013).
Martinez-Garcia, J. C., Rzoska, S. J., Drzozd-Rzoska, A., Martinez-Garcia, J. & Mauro, J. C. Divergent dynamics and the Kauzmann temperature in glass forming systems, Sci. Rep. 4, 5160 (2014).
Vogel, H. Temperaturabhangigkeitsgesetz der viskositaet von fluessigkeiten. Phys. Zeit. 22, 645–646 (1921).
Fulcher, G. S. Analysis of recent measurements of the viscosity of glasses. J. Am. Ceram. Soc. 8, 339–355 (1925).
Tammann, G. Glasses as supercooled liquids. J. Soc. Glass Technol. 9, 166–185 (1925).
Martinez-Garcia, J. C., Tamarit, J. Ll., Capaccioli, S., Barrio, M. & Veglio, N. et al. α-relaxation dynamics of orientanionally disordered mixed crystals composed of Cl-adamantane and CN-adamantane. J. Chem. Phys. 132, 164516–164526 (2010).
Martinez-Garcia, J. C., Tamarit, J. Ll., Pardo, L. C., Barrio, M. & Rzoska, S. J. et al. A. Disentangling the secondary relaxations in the orientationally disordered mixed crystals: cycloheptanol + cyclooctanol two-component system, J. Phys. Chem. B 114, 6099–6106 (2010).
Martinez-Garcia, J. C., Tamarit, J. Ll., Rzoska, S. J., Drozd-Rzoska, A. & Pardo, L. C. et al. Universal critical-like scaling of dynamics plastic crystals. J. Non-Cryst. Solids 357, 329–333 (2011).
Brand, R., Lunkenheimer, P., Schneider, U. & Loidl, A. Excess wing in the dielectric loss of glass-forming ethanol: A relaxation process. Phys. Rev. B 62, 8878–8883 (2000).
Drozd-Rzoska, A., Rzoska, S. J. & Paluch, M. Universal critical-like scaling of dynamic properties in symmetry-selected glass formers. J. Chem. Phys. 129, 184509–184515 (2008).
Stickel, F. “Untersuchungen der dynamik in niedermolekularen flüssigkeiten mit dielektrischer spektroskopie”, PhD. dissertation (Mainz University, 1995).
Dyre, J. C. & Olsen, N. B. Landscape equivalent of the shoving model. Phys. Rev. E 69, 042501 (2004).
Hecksher, T., Nielsen, A. I., Olsen, N. B. & Dyre, J. C. Little evidence for dynamic divergences in ultraviscous molecular liquids. Nature Phys. 4, 737–741 (2008).
Agapov, A. L., Novikov, V. N. & Sokolov, A. P. Fragility and other properties of glass-forming liquids: Two decades of puzzling correlations, in Greer, L. A., Kelton, K., Sastry, S. (eds.), Fragility of glass forming liquids (Hindustan Book Agency, 2013).
Debenedetti, P. G. & Stillinger, F. H. Supercooled liquids and the glass transition, Nature 410, 259–267 (2001).
Capaccioli, S., Lucchesi, M., Rolla, P. A. & Ruggeri, G. Dielectric response analysis of a conducting polymer dominated by the hopping charge transport, J. Phys.: Condens. Matter 10, 5595–5617 (1998).
Macovez, R., Zachariah, M., Romanini, M., Zygouri, P. & Gournis, D. et al. Hopping conductivity and polarization effects in a fullerene derivative salt, J. Phys. Chem. C 118, 12170–12175 (2014).
Monkos, K. Analysis of the viscosity-temperature-concentration dependence for dimeric bovine β-lactoglobulin aqueous solutions on the basis of the Vogel-Tammann-Fulcher's equation, Current Topics in Biophys. 31, 16–24 (2008).
Viciosa, M. T. & Dionısio, M. Molecular mobility and fragility in n-ethylene glycol dimethacrylate monomers, J. Non-Cryst. Solids 341, 60–67 (2004).
Jadżyn, J., Czechowski, G., Legrand, C. & Douali, R. Static and dynamic dielectric properties of strongly polar liquids in the vicinity of first order and weakly first order phase transitions. Phys. Rev. E 67, 041705 (2003).
Paluch, M., Roland, C. M., Casalini, R., Meier, G. & Patkowski, A. The relative contributions of temperature and volume to structural relaxation of van der Waals molecular liquids. J. Chem. Phys. 118, 4578–4582 (2003).
Wang, L.-M. & Mauro, J. C. An upper limit to kinetic fragility in glass-forming liquids. J. Chem. Phys. 134, 044522 (2011).
Simon, S. L., Plazek, D. J., Sobieski, J. W. & McGregor, E. T. Physical aging of a polyetherimide: Volume recovery and its comparison to creep and enthalpy measurements. J. Polym. Sci. Part B. Polym. Phys. 35, 929–936 (1997).
Adam, G. & Gibbs, J. H. On the temperature dependence of cooperative relaxation properties in glass-forming liquids. J. Chem. Phys. 43, 139–146 (1965).
Tanaka, H. Relation between thermodynamics and kinetics of glass-forming liquids. Phys. Rev. Lett. 90, 05570 (2003).
Doremus, R. H. J. Viscosity of silica, J. Appl. Phys. 92, 7619–7630 (2002).
Acknowledgements
This research was carried out due to the support of the National Centre for Science (Poland), for SJR via grant UMO-2011/03/B/ST3/02352 and for ADR via grant UMO-2011/01/B/NZ9/02537.
Author information
Authors and Affiliations
Contributions
J.C.M.G., S.J.R. and A.D.R. wrote the main manuscript, J.C.M.G. prepared figures, S.z.S. worked on data analysis, J.C.M. finally shaped the form of the manuscript. Authors' related experimental results are associated with measurements carried out J.C.M.G., S.J.R. and A.D.R. New conceptions proposed in the paper are proposed mainly by J.C.M.G. and S.J.R. but finally influenced by A.D.R. and J.C.M.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article's Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder in order to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Martinez-Garcia, J., Rzoska, S., Drozd-Rzoska, A. et al. Fragility and basic process energies in vitrifying systems. Sci Rep 5, 8314 (2015). https://doi.org/10.1038/srep08314
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep08314
This article is cited by
-
New paradigm for configurational entropy in glass-forming systems
Scientific Reports (2022)
-
Universal behavior of the apparent fragility in ultraslow glass forming systems
Scientific Reports (2019)
-
Activation volume in superpressed glass-formers
Scientific Reports (2019)
-
Structure and properties of epoxy polymer nanocomposites reinforced with carbon nanotubes
Journal of Polymer Research (2019)
-
The impact of the stretching exponent on fragility of glass-forming liquids
Journal of Thermal Analysis and Calorimetry (2017)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.








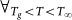 ∂ΔEa(T)/∂(1/T) ≥ 0 and IDO(T) ≥ 0.
∂ΔEa(T)/∂(1/T) ≥ 0 and IDO(T) ≥ 0.