Introduction

Soil is the most biologically active compartment of the biosphere, hosting unparalleled biodiversity at all scales1,2,3,4,5,6,7. Soil aqueous and biogeochemical environments are inherently heterogeneous and patchy2,8 and thus delineate ecological spheres of influence that may separate microbial communities with respect to location, physiology, or genetics1,3,9,10,11. Complex pore spaces and fragmented aqueous habitats impose constraints on nutrient transport and on microbial motion in unsaturated soils, whereby diffusion is the primary mechanism for nutrient supply relative to convection by rare infiltration episodes8,12,13. Additionally, pore space architecture and hydration conditions determine aqueous-phase configuration thereof, play a key role in shaping microbial community dynamics and composition in soils9,10,11. Cell motion is usually limited and is critical for survival and functioning in such patchy and heterogeneous environments1,5,8,14,15,16. Hydration constraints to motility and nutrient diffusion are expected to shape the dynamics and composition of the early phases of establishment of microbial communities on unsaturated rough surfaces inoculated by various processes (e.g. large convective flows)1,5,8,12,13. Recent studies have established relationships between hydration status that determine aqueous film properties and microbial flagellar motility12,13. We report a novel biophysical index for predicting hydration conditions that promote (or suppress) microbial coexistence on rough surfaces. We propose a framework for integrating quantifiable biophysical variables, such as aquatic habitat size and connectivity, nutrient diffusion rate affecting microbial growth rates and aqueous film thickness influencing microbial motility and dispersal distances, into a simple predictive index (see Fig. 1).

Figure 1
figure 1

Aqueous phase configuration on a schematic rough surface delineating connected clusters of sizes RC(ψ) and associated microbial mean generation length, RG(ψ) under (a) wet and (b) dry conditions.

Gray dash lines illustrate the additional distance required for a full generation length (additional time is required for cell division after reaching the aqueous cluster boundary). Red rods represented superior microbial species and blue ones inferior species, both are flagellated (dashed lines mark hypothetical cell trajectories).

Full size image

Results

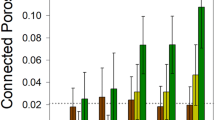
Figure 2 summarizes the variables used in deriving the proposed coexistence index. We first consider aqueous-phase fragmentation expressed as aqueous cluster size (Fig. 2a, with configurations shown also in Figs. 2d and 2e) as a function of water potential value and surface geometry properties. The aqueous clusters are connected through aqueous films too thin to support microbial flagellated motion, while support nutrient diffusion. Percolation theory17 predicts that the size of continuous aqueous clusters is expected to decrease with decreasing water potential (as a surface dries) with a distinct and abrupt drop occurring at a critical water potential value close to −4.0 kPa, which is in excellent agreement with numerical simulations of many different surface roughness networks. Invoking percolation theory, one may extend the predictions to volumes of connected aqueous clusters in 3D soil pore spaces (Fig. 2a and Supplementary Fig. S1). Accompanying the fragmentation of the aqueous phase, a significant drop in effective nutrient diffusion coefficient18 with decreasing water potential is predicted for various roughness networks, in good agreement with detailed simulation results (Fig. 2b). To complete the picture of hydration effects on microbial functions on unsaturated surfaces, we quantify effects of the macroscopic soil water potential on microbial flagellated motility (equation (3)). Comparisons of analytically-derived predictions with direct observations12 and detailed numerical simulations of cell motility considering many cells and different roughness networks show excellent agreement (Fig. 2c). The good agreement between simple analytical representations of key hydration-controlled processes motivates their joint use to predict hydration-mediated microbial coexistence on rough surfaces.

Figure 2
figure 2

(a) Predicted and simulated radii (mean±s.d., n = 5) of aqueous clusters (normalized by maximum cluster size under saturation condition) as a function of matric potential, (b) predicted and simulated (mean±s.d., n = 5) effective nutrient diffusion coefficients (normalized by diffusion coefficient of glucose in bulk water), UD and LR represent simulated diffusion coefficients with flux from top to bottom and from left to right boundaries of a domain, respectively, (c) analytical prediction for mean cell velocity and comparisons with numerical simulations and experimental measurements (mean±s.e.m., n = 34600 for simulation and n>248 for experiments) and aqueous cluster distributions on (d) wet and (e) dry surfaces, colors mark different clusters.

Full size image

The proposed CI postulates that existence of competing species within an aquatic island (cluster) critically depends on their presence at the boundaries of such a cluster, where they can intercept nutrients diffusing via aqueous films too thin to support flagellated motion. Figure 3 depicts analytical predictions of the proposed CI for multiple microbial populations on unsaturated rough surfaces. The CI is based solely on surface roughness properties and microbial physiological traits (growth rates, motility, etc.) as mediated by hydration status (expressed as a macroscopic quantity – matric potential). An important advantage of the proposed CI is that the analytical prediction does not require details regarding the structure of diffusion fields nor specifics concerning population interactions and growth dynamics. CI values of less than unity for high matric potential values (wet conditions) indicate that distances (mean generation length) traversed by motile cells within one generation (until binary fission or a doubling time) are shorter than aqueous cluster size (which, for wet conditions, could span a large fraction of the simulation domain). Low CI values also imply rapid increase in population size before reaching microhabitat boundaries. Consequently, the highly competitive species (superior species) may quickly dominate the boundaries, or even enclose slower growing species (inferior species) prior to reaching the boundaries and gradually intercept larger fractions of incoming nutrients thereby tipping competition balance, resulting in competitive exclusion of less effective competitors5. Figure 3b depicts the evolution of microbial relative abundance whereby the most competitive species dominate at CI values bellow unity (associated with wet surfaces). Under drier conditions (low matric potential values), predicted CI values gradually increase until a critical transition occurs at CI = 1 marking conditions for the onset of coexistence. These conditions are also marked by an abrupt transition in population evenness value expressed by the widely used Simpson index19. The transition occurs across a surprisingly narrow range of matric potentials within a few kPa (Fig. 3a). Additionally, the theoretically derived relative fitness (RF) indicates a transition to coexistence mode among microbial species above the critical threshold of CI = 1 (at around −4 kPa) (Fig. 3b).

Figure 3
figure 3

(a) Analytical microbial CI predictions (mean±s.d., n = 6, gray area marks 1 s.d.) and corresponding common Simpson species evenness (initial inoculation size of 64 cells of each species) and comparisons with simulated CI values (mean±s.d., n = 384) and Simpson evenness (mean±s.d., n = 16 mixed population inoculated colonies) and (b) analytical and simulated (mean±s.d., n = 16 mixed population inoculated colonies) relative abundance as a function of CI.

Note the trend towards higher evenness under drier conditions. The simulated abundance distributions were extracted from the same set of numerical simulations used for evenness indices presented in (a).

Full size image

The performance of the CI was evaluated primarily based on Monte Carlo simulations using a mechanistic discrete individual-based model12,13 simulating growth and life histories of large and multispecies microbial populations with typical results shown in Fig. 3 and Supplementary Fig. S2. Remarkably, the simulation results reflecting behaviors of many individual cells responding to their local microenvironments were in reasonable agreement with the simplified analytical predictions (Fig. 3) and thus lend credence to the underlying assumptions of the proposed CI. The predictive index was also evaluated for limited experimental data20 for two competing microbial species grown in soil (3D pore systems) under different hydration conditions. The comparison depicted in Fig. 4 shows that with decreasing matric potential, a marked increase of the relative fitness of inferior species occurs at the expected critical CI = 1 coinciding with hydration conditions for coexistence, which is consistent with experimental observations20.

Figure 4
figure 4

Analytical CI predictions and calculated relative fitness (RF) (initial inoculation size of 100 cells of each species) for 3D porous media and comparisons of RF with experimental data20 (“triangle” and “square” symbols mark experimental data extracted from Fig. 3 and Fig. 4 in Reference 20, respectively).

Full size image

The analytical estimates of aqueous habitat fragmentation based on percolation theory (equation (1)) were also used to estimate the numbers of aqueous clusters in soils yielding close agreement with numerical simulations and are well constrained by total numbers of soil grains21 (Supplementary Fig. S3). The aquatic and granular fragmentations provide estimates of distinct niches for accommodating the extremely diverse microbial populations consistent with the theories of spatial heterogeneity promoting microbial diversity in soils8,11,22,23. Additionally, the predicted sizes of aquatic habitats hosting competing microbial populations provide the basis for some of the coexistence calculations within the confines of aqueous clusters.

Discussion

Although the notion that dry conditions induce spatial segregation is well established8, the narrow range of hydration conditions (a few kPa) at which the aqueous-phase becomes fragmented is surprising and the generality of this strong fragmentation at a relatively wet state (in most soils and geographical regions) is important and not widely recognized. The CI prediction that lower water matric potential values increase microbial diversity is consistent with recently reported experimental observations22,23,24. For instance, the experimental results of Zhou et al.24 reveal orders of magnitude higher microbial diversity in unsaturated surface soils relative to saturated deeper soils. Despite limited experimental information, the general agreement inspires confidence in the potential usefulness of this new CI for prediction of conditions promoting or limiting soil microbial coexistence and biodiversity based on simple traits and ambient conditions. In contrast with standard diversity metrics such as relative fitness25, Shannon and Simpson indices19 that are all based on analyzing experimental or simulation results, the proposed CI is a predictive metric entirely based on simple and measurable biophysical parameters. It is anticipated that the mechanisms underlying the proposed CI are particularly important during early stages of microbial colonization by large convective events following extended dry periods (rewetting of surfaces)5,8,26.

The practical implementation of the proposed CI in a predictive mode would require information regarding ranges of specific growth rates and motilities of microbial populations inhabiting soil surfaces. However considering the strong constraints imposed by aquatic habitat fragmentation and formation of thin water films, differences in motility among species are likely to be suppressed, thereby reducing parameter requirements for CI application to estimates of specific growth rate range. Nominally, the mean growth rate for a population would suffice to identify hydration conditions for onset of coexistence; however, estimates of relative abundance would require information on the range of specific growth rate values of a population and the picture is likely to become more complex with consideration of hydration dynamics27.

The narrow range of hydration conditions (a few kPa) for aqueous phase fragmentation and limited nutrient diffusion is relatively general and leads to an almost universal transition to sessile microbial life due to cell pinning behind thin liquid films regardless of competitive advantages of a species. Additionally, conditions conducive to significant dispersal and population mixing are expected to be limited and rare in most soils (only a few hours several times per year even in temperate regions3), highlighting the inherent segregation in soils under natural climatic conditions and across all soil types. Despite numerous simplifications, the analytical CI represents a step towards linking the complex soil physical environment with microbial biodiversity in a predictive and ecologically consistent fashion and offers a potential for addressing core issues in contemporary soil microbial ecology concerning soil and water resource quality, the fate of environmental contaminants and global biogeochemical cycles28,29.

Methods

For the numerical simulations used to test the proposed analytical CI, we considered a model system of a rough soil surface represented by an equivalent network (with physical size of 34.4 × 34.4 mm with 200 × 173 sites on a hexagonal lattice) of simple roughness elements whose aqueous phase content and connectivity are functions of the matric potential and geometrical characteristics of the network12,13. An important ingredient in the foregoing analysis is the size of aqueous clusters defined as groups of interconnected pores or capillary channels that retain sufficiently thick aqueous films to support flagellar motility12 and border “empty” channels with aqueous films too thin to support cell motion but available for nutrient diffusion8,17. The physical picture of the aqueous phase changes dramatically as rough surfaces dry. As the ambient matric potential becomes lower (dryer), air-water interfaces recede deeper into crevices resulting in fragmentation of the previously connected aqueous network (Fig. 1a) into clusters of aqueous islands8,12 (Fig. 1b). Consequently, the interplay of capillary forces and surface geometries shape details of the aqueous-phase network. However, the effective size of the largest aqueous cluster RC(ψ) is predictable from universality of percolation theory17 and can be expressed as a function of the aqueous-phase content (controlled by the ambient matric potential, ψ),

where R0 is the radius of system size (for a finite domain), NC is the number of pores/channels of the largest cluster, N0 is the number of total pores/channels of a system and χ is a universal exponent dependent on the dimensionality of the network (see Supplementary information ). The aqueous cluster radius not only defines the size of an isolated microhabitat where competing microbial species may inhabit and interact, but it also determines the boundaries through which diffusive nutrient fluxes arrive and support life within the cluster.

Another important consequence of aqueous-phase fragmentation and film thinning is the reduction in effective nutrient diffusion (expressed as effective nutrient diffusion coefficient, Deff). The relationship between mean water content on the surface (〈θ(ψ)〉, a function of matric potential) and effective nutrient diffusion coefficient is expressed as18 (see Supplementary information ):

where D0 is nutrient diffusion coefficient in bulk water and φ is the effective “porosity” generated by surface roughness (relative to a smooth surface, see Supplementary information ). The averaged nutrient diffusive flux (represented by Deff) is a measure of nutrient limitation to microbial growth rate that, in turn, determines microbial life history (see Supplementary information ).

Despite severe limitations to microbial flagellar motion within thin films and fragmented aqueous networks, even minute changes in position within the network may play a critical role in the highly heterogeneous diffusion fields1,5,14, where conditions leading to population growth or decay may be a few channels or pores apart12,29. Recent studies12,13 have shown that microbial cell motion (expressed as mean flagellated cell velocity, 〈V(ψ)〉) was significantly restricted relative to flagellar motion in bulk water owning to the thinning of aqueous film that gives rise to additional viscous drag and capillary pinning forces according to:

where V0 is mean cell velocity in bulk water, FM, FC and Fλ are the viscous drag force opposing motion in bulk water, the viscous force associated with cell-surface hydrodynamic interactions and the capillary pinning force, respectively, f*(α,H) is bivariate probability density function of roughness elements spanning angle (α) and height (H) within the range of values Ωα and ΩH (see Supplementary information ).

We may now combine these hydration based factors (cluster size, nutrient diffusion and cell motility) to estimate a characteristic distance traversed by a microbial cell during a single generation. We term this integrative variable ‘mean generation length’ (RG), which explicitly incorporates intrinsic microbial growth characteristics with motility30 and hydration status as (see Supplementary information ):

where µeff is effective microbial specific growth rate and τ is the mean interval of microbial motile duration (see Supplementary information ). Considering limitations imposed by aqueous films for cell dispersal outside clusters, the boundaries of aqueous clusters are entry regions for nutrient fluxes supporting microbial life within the clusters. Consequently, species capable of establishing presence along these boundaries have a competitive advantage over species in the cluster interior31. Based on this line of reasoning, we propose a novel coexistence index defined as the ratio of microbial generation length 〈RG(ψ)〉 to the effective radius of aqueous cluster RC(ψ), with both characteristic lengths dependent on hydration condition – matric potential value (see Supplementary information ),

The proposed CI compares mean distance traversed by a cell during one generation with the effective size of aqueous clusters (islands) that may host multiple species. Note that 〈RG(ψ)〉 reflects not only net motion but also potential for nutrient interception required for cell growth and division. The criticality of nutrient entry zone in a diffusion controlled environment makes presence of multiple species in this zone a defining factor for microbial coexistence. Under wet and favorable environmental conditions, physiologically superior species with fast growth rate may rapidly form a large population dominating presence along these boundaries at greater proportions than relatively slower growing species and intercept a large fraction of nutrient fluxes from the boundaries. As a consequence, the resulting nutrient depletion at the interior of the microhabitat (aqueous cluster or island) would invariably lead to competitive exclusion of inferior species (Fig. 1a). In contrast, under drier conditions with fragmented aqueous-phase and reduced nutrient supply, microbial growth is limited below physiological capacity, lengthening microbial generation characteristic time (and length) relative to the size of the microhabitat thereof, enhancing chances of arrival of a diverse composition of species to the boundaries, giving rise to prolonged coexistence and more even species abundance (Fig. 1b). Overall, the model provides qualitative and quantitative estimates for the onset or loss of microbial coexistence, including relative abundance calculations in unsaturated soils (see Supplementary information ).