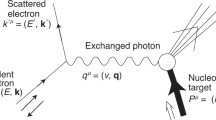
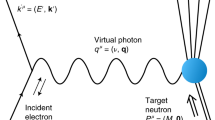
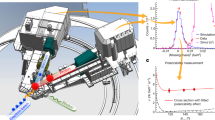
Abstract
More than three decades ago, the European Muon Collaboration published a surprising result on the spin structure of the proton: the spins of its three quark components account for only a small part of the spin of the proton. Ever since, theoretical and experimental progress has been made in understanding the origins of the proton spin. In this Review, we discuss what has been learned so far, what is still missing and what could be learned from the upcoming experiments, including the Jefferson Lab 12 GeV upgrade and the proposed Electron-Ion Collider. In particular, we focus on first-principles calculations and experimental measurements of the total gluon helicity ΔG, and the quark and gluon orbital angular momenta.
Key points
-
There are two established approaches to look at the composition of the proton spin: the frame-independent spin structure (or the Ji sum rule) and the infinite-momentum-frame or parton spin structure (or the Jaffe–Manohar sum rule).
-
In the frame-independent approach, the quark orbital and gluon angular momentum contributions can be extracted from the moments of the generalized parton distributions. Results from the Jefferson Lab 6 GeV and HERMES experiments suggest that there is a substantial quark orbital contribution.
-
In terms of partons, the quark and gluon helicity contributions have a simple physical interpretation, and the result from Relativistic Heavy Ion Collider spin experiments has provided a first important constraint on the total gluon helicity.
-
The development of a large-momentum effective theory along with lattice quantum chromodynamics simulations provide first-principles calculations of the spin structure. The results on the quark and gluon helicity contributions, and the quark orbital and gluon angular momentum contributions have provided the first complete theoretical picture.
-
The Jefferson Lab 12 GeV programme will provide better information on the quark orbital angular momentum and gluon angular momentum. The future Electron-Ion Collider will provide high-precision measurements on the gluon helicity and gluon angular momentum.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 digital issues and online access to articles
$99.00 per year
only $8.25 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Change history
21 December 2020
A Correction to this paper has been published: https://doi.org/10.1038/s42254-020-00274-2.
References
Rutherford, E. Collision of α particles with light atoms. IV. An anomalous effect in nitrogen. Phil. Mag. Ser. 6 37, 581–587 (1919).
Estermann, I., Frisch, R. & Stern, O. Magnetic moment of the proton. Nature 132, 169–170 (1933).
Dirac, P. A. M. The quantum theory of the electron. Proc. R. Soc. Lond. A117, 610–624 (1928).
Skyrme, T. H. R. A unified field theory of mesons and baryons. Nucl. Phys. 31, 556–569 (1962).
Gell-Mann, M. A schematic model of baryons and mesons. Phys. Lett. 8, 214–215 (1964).
Zweig, G. An SU(3) Model for Strong Interaction Symmetry and its Breaking Version 1 Report CERN-TH-401 (CERN, 1964).
Bhaduri, R. K. Models of the Nucleon: from Quarks to Solition (Lecture Notes and Supplements in Physics Vol. 22, Addison-Wesley, 1988).
Thomas, A. W. & Weise, W. The Structure of the Nucleon (Wiley, 2001).
Fritzsch, H., Gell-Mann, M. & Leutwyler, H. Advantages of the color octet gluon picture. Phys. Lett. 47B, 365–368 (1973).
Greenberg, O. W. Spin and unitary spin independence in a paraquark model of baryons and mesons. Phys. Rev. Lett. 13, 598–602 (1964).
Isgur, N. & Karl, G. Positive parity excited baryons in a quark model with hyperfine interactions. Phys. Rev. D 19, 2653–2677 (1979).
Close, F. E. An Introduction to Quarks and Partons (Academic Press, 1979).
Hughes, V. W. & Kuti, J. Internal spin structure of the nucleon. Ann. Rev. Nucl. Part. Sci. 33, 611–644 (1983).
Ashman, J. et al. A measurement of the spin asymmetry and determination of the structure function g1 in deep inelastic muon-proton scattering. Phys. Lett. B 206, 340–346 (1988).
Ashman, J. et al. An investigation of the spin structure of the proton in deep inelastic scattering of polarized muons on polarized protons. Nucl. Phys. B 328, 351–385 (1989).
Ellis, J. R. & Jaffe, R. L. A sum rule for deep inelastic electroproduction from polarized protons. Phys. Rev. D 9, 1444-1446 (1974).
Filippone, B. W. & Ji, X.-D. The spin structure of the nucleon. Adv. Nucl. Phys. 26, 1–88 (2001).
Bass, S. D. The spin structure of the proton. Rev. Mod. Phys. 77, 1257–1302 (2005).
Aidala, C. A., Bass, S. D., Hasch, D. & Mallot, G. K. The spin structure of the nucleon. Rev. Mod. Phys. 85, 655–691 (2013).
Leader, E. & Lorce, C. The angular momentum controversy: what’s it all about and does it matter? Phys. Rep. 541, 163–248 (2014).
Ji, X. Proton tomography through deeply virtual compton scattering. Natl Sci. Rev. 4, 213–223 (2017).
Deur, A., Brodsky, S. J. & De Taramond, G. F. The spin structure of the nucleon. Rep. Prog. Phys. 82, 076201 (2019).
Accardi, A. et al. Electron-Ion Collider: the next QCD frontier. Eur. Phys. J. A 52, 268 (2016).
Boer, D. et al. Gluons and the quark sea at high energies: distributions, polarization, tomography. Preprint at http://arXiv.org/abs/1108.1713 (2011).
Tung, W. K. Group Theory In Physics, World Scientific Publishing Company (1985).
Ji, X.-D., Tang, J. & Hoodbhoy, P. The spin structure of the nucleon in the asymptotic limit. Phys. Rev. Lett. 76, 740–743 (1996).
Ji, X. Comment on ‘spin and orbital angular momentum in Gauge theories: nucleon spin structure and multipole radiation revisited’. Phys. Rev. Lett. 104, 039101 (2010).
Bunce, G., Saito, N., Soffer, J. & Vogelsang, W. Prospects for spin physics at RHIC. Ann. Rev. Nucl. Part. Sci. 50, 525–575 (2000).
Dudek, J. et al. Physics opportunities with the 12 GeV upgrade at Jefferson Lab. Eur. Phys. J. A 48, 187 (2012).
Ji, X.-D. Gauge-invariant decomposition of nucleon spin. Phys. Rev. Lett. 78, 610–613 (1997).
Jaffe, R. L. & Manohar, A. The g1 problem: fact and fantasy on the spin of the proton. Nucl. Phys. B 337, 509–546 (1990).
Noether, E. Invariant variation problems. Gott. Nachr. 1918, 235–257 (1918).
Ji, X., Xu, Y. & Zhao, Y. Gluon spin, canonical momentum, and Gauge symmetry. J. High Energy Phys. 2012, 82 (2012).
Belinfante, F. J. On the current and the density of the electric charge, the energy, the linear momentum and the angular momentum of arbitrary fields. Physica 7, 449–474 (1940).
Ji, X.-D. Lorentz symmetry and the internal structure of the nucleon. Phys. Rev. D 58, 056003 (1998).
Moch, S., Vermaseren, J. & Vogt, A. The three-loop splitting functions in QCD: the helicity-dependent case. Nucl. Phys. B 889, 351–400 (2014).
Behring, A. et al. The polarized three-loop anomalous dimensions from on-shell massive operator matrix elements. Nucl. Phys. B 948, 114753 (2019).
de Florian, D. & Vogelsang, W. Spin budget of the proton at NNLO and beyond. Phys. Rev. D 99, 054001 (2019).
Thomas, A. W. Interplay of spin and orbital angular momentum in the proton. Phys. Rev. Lett. 101, 102003 (2008).
Hatta, Y. Notes on the orbital angular momentum of quarks in the nucleon. Phys. Lett. B 708, 186–190 (2012).
Lorce, C. & Pasquini, B. Quark Wigner distributions and orbital angular momentum. Phys. Rev. D 84, 014015 (2011).
Lorce, C., Pasquini, B., Xiong, X. & Yuan, F. The quark orbital angular momentum from Wigner distributions and light-cone wave functions. Phys. Rev. D 85, 114006 (2012).
Ji, X., Xiong, X. & Yuan, F. Proton spin structure from measurable parton distributions. Phys. Rev. Lett. 109, 152005 (2012).
Ji, X.-D. Viewing the proton through ‘color’ filters. Phys. Rev. Lett. 91, 062001 (2003).
Belitsky, A. V., Ji, X.-D. & Yuan, F. Quark imaging in the proton via quantum phase space distributions. Phys. Rev. D 69, 074014 (2004).
Wakamatsu, M. More on the relation between the two physically inequivalent decompositions of the nucleon spin and momentum. Phys. Rev. D 85, 114039 (2012).
Burkardt, M. Parton orbital angular momentum and final state interactions. Phys. Rev. D 88, 014014 (2013).
Chen, X.-S., Lu, X.-F., Sun, W.-M., Wang, F. & Goldman, T. Spin and orbital angular momentum in gauge theories: nucleon spin structure and multipole radiation revisited. Phys. Rev. Lett. 100, 232002 (2008).
Ji, X., Zhang, J.-H. & Zhao, Y. Physics of the gluon-helicity contribution to proton spin. Phys. Rev. Lett. 111, 112002 (2013).
Hatta, Y., Ji, X. & Zhao, Y. Gluon helicity ΔG from a universality class of operators on a lattice. Phys. Rev. D 89, 085030 (2014).
Hoodbhoy, P., Ji, X.-D. & Lu, W. Implications of color gauge symmetry for nucleon spin structure. Phys. Rev. D 59, 074010 (1999).
Hoodbhoy, P. & Ji, X.-D. Does the gluon spin contribute in a gauge invariant way to nucleon spin? Phys. Rev. D 60, 114042 (1999).
Hatta, Y. Gluon polarization in the nucleon demystified. Phys. Rev. D 84, 041701 (2011).
Manohar, A. V. Polarized parton distribution functions. Phys. Rev. Lett. 66, 289–292 (1991).
von Weizsacker, C. F. Radiation emitted in collisions of very fast electrons. Z. Phys. 88, 612–625 (1934).
Williams, E. J. Correlation of certain collision problems with radiation theory. Kong. Dan. Vid. Sel. Mat. Fys. Med. 13N4, 1–50 (1935).
Allen, L., Beijersbergen, M. W., Spreeuw, R. J. C. & Woerdman, J. P. Orbital angular momentum of light and the transformation of Laguerre–Gaussian laser modes. Phys. Rev. A 45, 8185–8189 (1992).
Bliokh, K. Y. & Nori, F. Transverse and longitudinal angular momenta of light. Phys. Rep. 592, 1–38 (2015).
Altarelli, G. & Parisi, G. Asymptotic freedom in parton language. Nucl. Phys. B 126, 298–318 (1977).
Hagler, P. & Schafer, A. Evolution equations for higher moments of angular momentum distributions. Phys. Lett. B 430, 179–185 (1998).
Harindranath, A. & Kundu, R. On orbital angular momentum in deep inelastic scattering. Phys. Rev. D 59, 116013 (1999).
Bashinsky, S. & Jaffe, R. L. Quark and gluon orbital angular momentum and spin in hard processes. Nucl. Phys. B 536, 303–317 (1998).
Hoodbhoy, P., Ji, X.-D. & Lu, W. Quark orbital-angular-momentum distribution in the nucleon. Phys. Rev. D 59, 014013 (1999).
Hatta, Y. & Yao, X. QCD evolution of the orbital angular momentum of quarks and gluons: genuine twist-three part. Phys. Lett. B 798, 134941 (2019).
Ji, X. & Yuan, F. Transverse spin sum rule of the proton, Phys. Lett. B 810, 135786 (2020).
Bakker, B. L. G., Leader, E. & Trueman, T. L. A critique of the angular momentum sum rules and a new angular momentum sum rule. Phys. Rev. D 70, 114001 (2004).
Leader, E. New relation between transverse angular momentum and generalized parton distributions. Phys. Rev. D 85, 051501 (2012).
Burkardt, M. Impact parameter space interpretation for generalized parton distributions. Int. J. Mod. Phys. A 18, 173–208 (2003).
Burkardt, M. Transverse deformation of parton distributions and transversity decomposition of angular momentum. Phys. Rev. D 72, 094020 (2005).
Ji, X., Xiong, X. & Yuan, F. Transverse polarization of the nucleon in parton picture. Phys. Lett. B 717, 214–218 (2012).
Brodsky, S. J., de Teramond, G. F., Dosch, H. G. & Erlich, J. Light-front holographic QCD and emerging confinement. Phys. Rep. 584, 1–105 (2015).
Wilson, K. G. Confinement of quarks. Phys. Rev. D 10, 2445–2459 (1974).
Rothe, H. J. Lattice gauge theories: an introduction. World Sci. Lect. Notes Phys. 43, 1–381 (1992).
Maris, P. & Roberts, C. D. Dyson–Schwinger equations: a tool for hadron physics. Int. J. Mod. Phys. E 12, 297–365 (2003).
Schafer, T. & Shuryak, E. V. Instantons in QCD. Rev. Mod. Phys. 70, 323–426 (1998).
Alexandrou, C. et al. Complete flavor decomposition of the spin and momentum fraction of the proton using lattice QCD simulations at physical pion mass. Phys. Rev. D 101, 094513 (2020).
Ji, X. Parton physics on a Euclidean lattice. Phys. Rev. Lett. 110, 262002 (2013).
Ji, X. Parton physics from large-momentum effective field theory. Sci. China Phys. Mech. Astron. 57, 1407–1412 (2014).
Ji, X., Liu, Y.-S., Liu, Y., Zhang, J.-H. & Zhao, Y. Large-momentum effective theory. Preprint at http://arXiv.org/abs/2004.03543 (2020).
Dong, S., Lagae, J.-F. & Liu, K. Flavor singlet gA from lattice QCD. Phys. Rev. Lett. 75, 2096–2099 (1995).
Lin, H.-W. et al. Parton distributions and lattice QCD calculations: a community white paper. Prog. Part. Nucl. Phys. 100, 107–160 (2018).
Liang, J., Yang, Y.-B., Draper, T., Gong, M. & Liu, K.-F. Quark spins and anomalous ward identity. Phys. Rev. D 98, 074505 (2018).
Alexandrou, C. et al. Nucleon spin and momentum decomposition using lattice QCD simulations. Phys. Rev. Lett. 119, 142002 (2017).
Deka, M. et al. Lattice study of quark and glue momenta and angular momenta in the nucleon. Phys. Rev. D 91, 014505 (2015).
Gong, M. et al. Strange and charm quark spins from the anomalous Ward identity. Phys. Rev. D 95, 114509 (2017).
Mathur, N., Dong, S. J., Liu, K. F., Mankiewicz, L. & Mukhopadhyay, N. C. Quark orbital angular momentum from lattice QCD. Phys. Rev. D 62, 114504 (2000).
Hagler, P. et al. Moments of nucleon generalized parton distributions in lattice QCD. Phys. Rev. D 68, 034505 (2003).
Gockeler, M. et al. Generalized parton distributions from lattice QCD. Phys. Rev. Lett. 92, 042002 (2004).
Brommel, D. et al. Moments of generalized parton distributions and quark angular momentum of the nucleon. Proc. Sci. LATTICE2007, 158 (2007).
Bratt, J. D. et al. Nucleon structure from mixed action calculations using 2 + 1 flavors of asqtad sea and domain wall valence fermions. Phys. Rev. D 82, 094502 (2010).
Syritsyn, S. N. et al. Quark contributions to nucleon momentum and spin from domain wall fermion calculations. Proc. Sci. LATTICE2011, 178 (2011).
Alexandrou, C. et al. Moments of nucleon generalized parton distributions from lattice QCD. Phys. Rev. D 83, 114513 (2011).
Alexandrou, C. et al. Nucleon form factors and moments of generalized parton distributions using Nf = 2 + 1 + 1 twisted mass fermions. Phys. Rev. D 88, 014509 (2013).
Yang, Y.-B. A lattice story of proton spin. Proc. Sci. LATTICE2018, 017 (2019).
Chen, X.-S., Sun, W.-M., Wang, F. & Goldman, T. Proper identification of the gluon spin. Phys. Lett. B 700, 21–24 (2011).
Weinberg, S. Dynamics at infinite momentum. Phys. Rev. 150, 1313–1318 (1966).
Yang, Y.-B. et al. Glue spin and helicity in the proton from lattice QCD. Phys. Rev. Lett. 118, 102001 (2017).
Zhao, Y., Liu, K.-F. & Yang, Y. Orbital angular momentum and generalized transverse momentum distribution. Phys. Rev. D 93, 054006 (2016).
Ji, X., Zhang, J.-H. & Zhao, Y. Justifying the naive partonic sum rule for proton spin. Phys. Lett. B 743, 180–183 (2015).
Gadiyak, V., Ji, X.-d. & Jung, C.-w. A lattice study of the magnetic moment and the spin structure of the nucleon. Phys. Rev. D 65, 094510 (2002).
Blum, T. et al. Lattice calculation of hadronic light-by-light contribution to the muon anomalous magnetic moment. Phys. Rev. D 93, 014503 (2016).
Engelhardt, M. Quark orbital dynamics in the proton from lattice QCD — from Ji to Jaffe–Manohar orbital angular momentum. Phys. Rev. D 95, 094505 (2017).
Engelhardt, M. et al. From Ji to Jaffe–Manohar orbital angular momentum in lattice QCD using a direct derivative method. Preprint at http://arXiv.org/abs/2008.03660 (2020).
Aslan, F. & Burkardt, M. Singularities in twist-3 quark distributions. Phys. Rev. D 101, 016010 (2020).
Ji, X. Fundamental properties of the proton in light-front zero modes, Nuclear Physics B 960, 115181 (2020).
Ji, X.-D. Deeply virtual Compton scattering. Phys. Rev. D 55, 7114–7125 (1997).
Airapetian, A. et al. Quark helicity distributions in the nucleon for up, down, and strange quarks from semi-inclusive deep-inelastic scattering. Phys. Rev. D 71, 012003 (2005).
Alekseev, M. et al. Flavour separation of helicity distributions from deep inelastic muon-deuteron scattering. Phys. Lett. B 680, 217–224 (2009).
de Florian, D., Sassot, R., Stratmann, M. & Vogelsang, W. Extraction of spin-dependent parton densities and their uncertainties. Phys. Rev. D 80, 034030 (2009).
de Florian, D., Sassot, R., Stratmann, M. & Vogelsang, W. Evidence for polarization of gluons in the proton. Phys. Rev. Lett. 113, 012001 (2014).
Nocera, E. R., Ball, R. D., Forte, S., Ridolfi, G. & Rojo, J. A first unbiased global determination of polarized PDFs and their uncertainties. Nucl. Phys. B 887, 276–308 (2014).
Ethier, J. J., Sato, N. & Melnitchouk, W. First simultaneous extraction of spin-dependent parton distributions and fragmentation functions from a global QCD analysis. Phys. Rev. Lett. 119, 132001 (2017).
Adam, J. et al. Measurement of the longitudinal spin asymmetries for weak boson production in proton-proton collisions at \(\sqrt{s}\) = 510 GeV. Phys. Rev. D 99, 051102 (2019).
Adamczyk, L. et al. Precision measurement of the longitudinal double-spin asymmetry for inclusive jet production in polarized proton collisions at \(\sqrt{s}\) = 200 GeV. Phys. Rev. Lett. 115, 092002 (2015).
Aschenauer, E.-C. et al. The RHIC SPIN program: achievements and future opportunities. Preprint at http://arXiv.org/abs/1501.01220 (2015).
Adam, J. et al. Longitudinal double-spin asymmetry for inclusive jet and dijet production in pp collisions at \(\sqrt{s}\) = 510 GeV. Phys. Rev. D 100, 052005 (2019).
Collins, J. C., Frankfurt, L. & Strikman, M. Factorization for hard exclusive electroproduction of mesons in QCD. Phys. Rev. D 56, 2982–3006 (1997).
Mankiewicz, L., Piller, G., Stein, E., Vanttinen, M. & Weigl, T. NLO corrections to deeply virtual Compton scattering. Phys. Lett. B 425, 186–192 (1998).
Ji, X.-D. Off forward parton distributions. J. Phys. G 24, 1181–1205 (1998).
Diehl, M. Generalized Parton Distributions. Phys. Rep. 388, 41–277 (2003).
Ji, X. Generalized parton distributions. Ann. Rev. Nucl. Part. Sci. 54, 413–450 (2004).
Belitsky, A. & Radyushkin, A. Unraveling hadron structure with generalized parton distributions. Phys. Rep. 418, 1–387 (2005).
Airapetian, A. et al. Measurement of azimuthal asymmetries with respect to both beam charge and transverse target polarization in exclusive electroproduction of real photons. J. High Energy Phys. 2008, 066 (2008).
Mazouz, M. et al. Deeply virtual Compton scattering off the neutron. Phys. Rev. Lett. 99, 242501 (2007).
Akhunzyanov, R. et al. Transverse extension of partons in the proton probed in the sea-quark range by measuring the DVCS cross section. Phys. Lett. B 793, 188–194 (2019).
Burkert, V., Elouadrhiri, L. & Girod, F. The pressure distribution inside the proton. Nature 557, 396–399 (2018).
Ji, X., Yuan, F. & Zhao, Y. Hunting the gluon orbital angular momentum at the electron-ion collider. Phys. Rev. Lett. 118, 192004 (2017).
Hatta, Y., Nakagawa, Y., Yuan, F., Zhao, Y. & Xiao, B. Gluon orbital angular momentum at small-x. Phys. Rev. D 95, 114032 (2017).
Blumlein, J. & Vogt, A. The singlet contribution to the structure function g1(x,Q2) at small x. Phys. Lett. B 386, 350–358 (1996).
Kovchegov, Y. V., Pitonyak, D. & Sievert, M. D. Helicity evolution at small-x. J. High Energy Phys. 2016, 72 (2016).
Kovchegov, Y. V., Pitonyak, D. & Sievert, M. D. Small-x asymptotics of the quark helicity distribution. Phys. Rev. Lett. 118, 052001 (2017).
Kovchegov, Y. V. & Sievert, M. D. Small-x helicity evolution: an operator treatment. Phys. Rev. D 99, 054032 (2019).
Boussarie, R., Hatta, Y. & Yuan, F. Proton spin structure at small-x. Phys. Lett. B 797, 134817 (2019).
Tarasov, A. & Venugopalan, R. The role of the chiral anomaly in polarized deeply inelastic scattering I: finding the triangle graph inside the box diagram in Bjorken and Regge asymptotics. Preprint at http://arXiv.org/abs/2008.08104 (2020).
Ellinghaus, F., Nowak, W.-D., Vinnikov, A. & Ye, Z. Can the total angular momentum of u-quarks in the nucleon be accessed at HERMES? Eur. Phys. J. C 46, 729–739 (2006).
Goeke, K., Polyakov, M. V. & Vanderhaeghen, M. Hard exclusive reactions and the structure of hadrons. Prog. Part. Nucl. Phys. 47, 401–515 (2001).
Acknowledgements
This Review Article is dedicated to late V. Hughes whose drive in polarized DIS experiments lead to the surge and unabated interest in the proton spin structure, and perhaps to the EIC project in the United States. The authors thank C. Aidala, C. Alexandrou, M. Burkardt, Y. Hatta, D. Hertzog, R. Jaffe and K. F. Liu for useful communications relating to this article. This material is supported by the US Department of Energy, Office of Science, Office of Nuclear Physics, under contract numbers DE-AC02-05CH1123, DE-SC0012704 and DE-SC0020682, and within the framework of the TMD Topical Collaboration.
Author information
Authors and Affiliations
Contributions
The authors contributed equally to all aspects of the article.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information
Nature Reviews Physics thanks Volker Burkert and the other, anonymous, reviewers for their contribution to the peer review of this work.
Publisher’s note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Glossary
- Pauli–Lubanski spin
-
A spin four-vector operator generalized for relativistic particles.
- Partonic picture
-
The distribution of physical observables in partons with different momentum fractions x.
- Belinfante improvement procedure
-
A process leading to a symmetric and gauge-invariant energy-momentum tensor in gauge theories.
- Boost invariant
-
A property that does not change under a boost Lorentz transformation.
- Dimensional regularization
-
A process making momentum integrals finite at ultraviolet by changing the space-time dimensions.
- (Modified) minimal subtraction
-
A process subtracting off-ultraviolet divergences in dimensional regularization, often leading to a resolution scale dependence μ.
- On-shell
-
Physical particles with the correct energy-momentum relation are called on-shell or on-mass-shell; otherwise, they are called off-shell or off-mass-shell. Off-shell particles are virtual and can exist in interaction processes.
- Light-front
-
The path or separation along a direction of a light cone.
- Flavour-singlet
-
A quantity defined by summing over all quark flavours.
- Disconnected diagrams
-
Contributions in lattice quantum chromodynamics calculations, in which the quark operators do not connect with the external hadron states.
- Quenched approximation
-
A term referring to lattice quantum chromodynamics calculations in which the fermion determinant is neglected to save computational costs.
- Exclusive hard scattering
-
A hard scattering process in which specific final states with a fixed type of particle are produced.
- Photon exclusive production
-
A hard scattering process in which only a photon is produced.
- Bethe–Heitler amplitude
-
An elastic scattering amplitude in which only an extra photon is produced.
- Gravitational form factor
-
The energy-momentum form factor, derived from the fact that energy momentum is the charge for gravitational interaction.
Rights and permissions
About this article
Cite this article
Ji, X., Yuan, F. & Zhao, Y. What we know and what we don’t know about the proton spin after 30 years. Nat Rev Phys 3, 27–38 (2021). https://doi.org/10.1038/s42254-020-00248-4
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s42254-020-00248-4
This article is cited by
-
Orbital angular momentum at small x revisited
Journal of High Energy Physics (2024)
-
Orbital angular momentum small-x evolution: exact results in the large-Nc limit
Journal of High Energy Physics (2024)
-
Small x physics beyond eikonal approximation: an effective Hamiltonian approach
Journal of High Energy Physics (2023)
-
Status on lattice calculations of the proton spin decomposition
AAPPS Bulletin (2022)
-
Perturbative calculations of gravitational form factors at large momentum transfer
Journal of High Energy Physics (2022)