Introduction

Close to quantum critical points (QCPs) where a second order thermal phase transition is suppressed to absolute zero systems are highly susceptible to small perturbations. The high susceptibility gives rise to various instabilities in materials exhibiting unconventional superconductivity. Although the vanishing temperature at the QCP renders direct experiments impossible, the related critical fluctuations1,2 extend over a wide range of temperatures and manifest themselves under experimentally feasible conditions, yielding direct consequences for the macroscopic properties of materials.

One of the consequences is the deviation from the Landau-Fermi liquid picture observed in many materials such as the cuprates, the Fe-based compounds or the heavy fermion systems3. The linear-in T variation of the resistivity has been considered a landmark experimental manifestation4,5,6,7,8 thereof9, although the interrelation of the resistivity with quantum criticality remains a subject of discussion10. On the other hand, spectroscopic fingerprints strongly indicate a connection between the Ω/T scaling of a marginal Fermi liquid9 and quantum criticality, as observed in a recent study of the optical conductivity11 of the heavy fermion superconductor YbRh2Si212. Theoretical works suggest that quantum fluctuations may have a significant impact in the case for antiferromagnetic13 as well as charge density wave ordering14 and, more specifically, in the enhancement15 or formation16,17 of superconductivity in the iron-based superconductors.

Characteristic for systems with a QCP is the presence of a quantum critical region at temperatures above a crossover temperature T*(x) ~ ∣x − xc∣νz (where ν and z are the correlation length and dynamical critical exponents, respectively, the tuning parameter x, realized in our context by chemical substitution of the metal site, and critical doping xc). Since this broad region of the phase diagram manifests the behavior of the single quantum critical point at x = xc, T = 0, Frerot et al.18 dubbed this region a “magnifying lens” for quantum criticality.

Due to the tunability by doping and the purity of the crystals, we have opted to use as a testbed the compound Ba(Fe1−xCox)2As2, one of the most studied Fe-based superconductors, to investigate this lens. Ba(Fe1−xCox)2As2 exhibits a rich phase diagram19 with overlapping regions of magnetism, nematicity, and superconductivity, and shows a strong enhancement of nematic fluctuations having \({d}_{{x}^{2}-{y}^{2}}\) symmetry (here using the 1-Fe unit cell) close to the nematic transition20,21,22. A plethora of experimental studies20,21,23,24,25,26 indicate the existence of a QCP underneath the superconducting dome in this material, and the Raman response within the superconducting phase bears an imprint of nematic quantum criticality27. Most recently, Worasaran et al.28 provided striking evidence for nematic quantum criticality by observing power-law behavior of the nematic/structural transition temperature as a function of uniaxial strain. However, the influence of the QCP on the high-temperature characteristics, for instance signatures of Ω/T scaling, remain elusive.

In this Article, we provide a comprehensive investigation of the Raman response of Ba(Fe1−xCox)2As2 for doping concentrations on both sides of optimal doping xc ~ 0.061. Specifically, we fill the gap close to xc where Ω/T scaling is expected to be most pronounced and provide a solid experimental basis for an earlier proposal29. We use electronic inelastic light (Raman) scattering which probes fluctuations of various degrees of freedom30,31 and has led to promising results particularly in Fe-based superconductors20,22,32.

Results and discussion

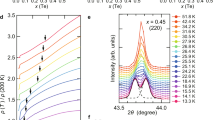
Earlier studies, investigating the low-frequency Raman response of Ba(Fe1−xCox)2As220,22, showed that the contribution of nematic fluctuations presents itself in the B1g (\({d}_{{x}^{2}-{y}^{2}}\)) symmetry of the 1-Fe unit cell whereas the temperature dependence of the A1g response is essentially independent of doping and follows the in-plane resistivity22. This suggests, that the essential critical dynamics of the system is captured by the B1g response. We therefore limit our analysis to the B1g response, with characteristic spectra shown in Fig. 1 for selected Ba(Fe1−xCox)2As2 samples with x < xc, x ~ xc, and x ≫ xc (see Figs. S1 and S2 in Supplementary Note 1 for the complete data set). All spectra have in common that the temperature dependence is limited to low frequencies, while no significant temperature dependence can be detected at higher frequencies.

Fig. 1: Temperature and doping dependence of the Raman response.
figure 1

Depicted is the Raman response function \(R{\chi }_{{{{{{{{\rm{B1g}}}}}}}}}^{^{\prime\prime} }({{\Omega }},T)\) in B1g symmetry (1-Fe unit cell) at selected doping concentrations x and temperatures T where Ω = ωi − ωs stands for the difference of the incident and scattered photon energy ωi and ωs, respectively. Tc refers to the superconducting transition temperature. a For the underdoped compound with x = 0.052, the low-frequency pile-up attributable to classical critical fluctuations exhibits a maximum above the nematic transition and decreases toward Tc. b Close to optimal doping with x = 0.059, the low-frequency response increases monotonically on cooling toward Tc. c For a sample with x ≫ xc, The temperature dependence is weak at all frequencies.

Full size image

Raman response

The underdoped compound [Fig. 1a] exhibits a nematic transition at approximately Tnem ~ 50 K. Similar to results reported elsewhere for x < xc20,22,32 the strong increase of the spectral weight between 333 and 53 K in the range below 500 cm−1 can be attributed to the presence of (classical) critical fluctuations. The decrease below Tnem, reminiscent of a gap, is characteristic for such transitions in the Fe-based superconductors, and consistent with earlier results22. Closer to optimal doping at x = 0.059 [Fig. 1b] the response increases monotonically upon cooling toward the superconducting transition temperature Tc. Remarkably, the response at this lowest temperature is nearly constant over the entire frequency range. Far away from xc, the temperature dependence is weak even at small energy transfer [Fig. 1c].

Transformation onto the imaginary time axis

To examine the possibility of quantum critical scaling, we have computed \(\beta \tilde{{{\Lambda }}}(\beta /2)\) from each of our measured spectra, with the results shown in Fig. 2. For the underdoped sample [Fig. 2a] the characteristic cusp singularity of the nematic transition is visible29. Notably, a similar increase observed using a sample with x = 0.057 doping in Fig. 2b toward Tc suggests a persisting nematic phase close to the superconducting transition. Yet, from our measurements it cannot be clearly decided whether or not nematicity sets in above Tc. Closer to optimal doping in Fig. 2c, \(\beta \tilde{{{\Lambda }}}(\beta /2)\) develops a broad maximum above Tc, though this cannot be attributed to a nematic phase transition. This maximum occurs roughly at the same temperature as for 5.2% doping and is thus too high in temperature for this doping concentration. Rather, we believe that the increase in the critical fluctuation response starts to freeze out at low temperatures and is then proceedingly compensated by the denominator in Eq. (2). Above xc at x = 0.073 [Fig. 2d], the quantity \(\beta \tilde{{{\Lambda }}}(\beta /2)\) remains constant down to the lowest measured temperature on the order of Tc. Tuning further away from xc [Fig. 2e and f], \(\beta \tilde{{{\Lambda }}}(\beta /2)\) decreases toward low temperatures, where \(\beta \tilde{{{\Lambda }}}(\beta /2)\) is generically required to vanish (see Supplementary Note 2 for a proof of this).

Fig. 2: Transformation onto the imaginary time axis.
figure 2

Shown is \(\beta \tilde{{{\Lambda }}}(\beta /2)\), the imaginary time-ordered correlation function \(\tilde{{{\Lambda }}}(\tau )\) derived from the Raman spectra of Ba(Fe1−xCox)2As2 and evaluated at τ = β/2 multiplied by the inverse temperature β = 1/kBT, versus temperature T. The dashed lines correspond to \(\beta \tilde{{{\Lambda }}}(\beta /2,T)={{{{{{{\rm{const}}}}}}}}\), and the blue regions indicate the deviation from this constant behavior, which we use to define the crossover temperatures \({T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\) and \({T}_{{{{{{{{\rm{OD}}}}}}}}}^{* }\) for x < xc and x > xc, respectively. a At x = 0.052 doping a nematic transition occurs at Tnem ~ 50 K at which \(\beta \tilde{{{\Lambda }}}(\beta /2,T)\) develops a cusp and then decreases toward Tc. b At x = 0.057, \(\beta \tilde{{{\Lambda }}}(\beta /2,T)\) increases toward lower temperatures and develops a maximum above Tc. c Closer to the quantum critical point (QCP) \(\beta \tilde{{{\Lambda }}}(\beta /2,T)\) develops a hump between \({T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\) and Tc. d On the overdoped side at x = 0.073 the Ω/T scaling persists down to Tc. e Further away, the crossover temperature increases again. f The high crossover temperature at x = 14.6 coincides with a small superconducting transition temperature.

Full size image

Doping dependence of T*

The dashed horizontal line is a guide to the eye indicating the value of \(\beta \tilde{{{\Lambda }}}\) in the regime of Ω/T scaling. Outside this quantum critical regime, where the response does not scale as Ω/T, the quantity \(\beta \tilde{{{\Lambda }}}(\beta /2)\) from this constant. The shaded regions show the crossover temperatures \({T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\) and \({T}_{{{{{{{{\rm{OD}}}}}}}}}^{* }\) for x < xc and x > xc, respectively, from Ω/T scaling to regions where other dynamics govern the low-frequency response.

\({T}_{{{{{{{{\rm{UD,OD}}}}}}}}}^{* }(x)\) exhibits a systematic doping dependence, with a global minimum at x = 0.073. To further illustrate this point, \({T}_{{{{{{{{\rm{UD,OD}}}}}}}}}^{* }(x)\) is plotted along with the relevant phase transitions of Ba(Fe1−xCox)2As2 in Fig. 3. The spin-density-wave (SDW) phase is indicated in gray, nematicity in red, and superconductivity in blue. The open red rectangles indicate the crossover temperatures derived from data presented in Lederer et al.29. The dashed lines represent guides to the eye.

Fig. 3: Phase diagram of Ba(Fe1−xCox)2As2.
figure 3

The spin-density-wave (SDW) phase is depicted in gray, nematicity in red, and superconductivity (SC) in blue as defined by the superconducting transition temperature Tc. The rectangular and circular data points indicate the crossover temperatures \({T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\) and \({T}_{{{{{{{{\rm{OD}}}}}}}}}^{* }\) from the region of Ω/T scaling for x < xc and x > xc, respectively. The dashed lines are guides to the eye. Open red squares and circles represent data points from Lederer et al.29. The gray, red, and blue phase separation lines are based on Chu et al.19. The error bars indicate the temperature window of T* depicted in Fig. 2.

Full size image

Underdoped region

For x < xc Ba(Fe1−xCox)2As2 has an SDW ground state below TSDW, and fluctuations in the temperature range \({T}_{{{{{{{{\rm{SDW}}}}}}}}} \, < \, T \, < \, {T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\). Above \({T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\) the Ω/T scaling characteristic for a marginal Fermi liquid is recovered. Moving closer to xc, the magnetic and nematic phases become unstable as signified by the decreasing transition temperatures. In the picture of quantum criticality, this results from a continously increasing superposition of entangled states which manifests itself as a divergence of the coherence length of the quantum fluctuations directly at the QCP. Simultaneously, the region of marginal Fermi liquid behavior with Ω/T scaling begins to persist over wider ranges of temperature with \({T}_{{{{{{{{\rm{UD}}}}}}}}}^{* }\) falling more rapidly after superconductivity sets in.

Overdoped region

On the overdoped side for x > xc, the crossover line re-emerges from the superconducting dome and continues to rise as a function of doping, with a significantly smaller slope than for x < xc. In the absence of a competing magnetic ground state, the quantum fluctuations may support superconductivity over a wider region of doping concentrations.

The endpoints of the dashed lines coincide with the region of optimal doping xc consistent with a scenario in which quantum fluctuations increasingly destroy the long-range order of the ground state for x < xc at temperatures T > 0. Thus, Tc is high where the quantum fluctuations dominate the normal state, and low where “classical” dynamics take over. Therefore the quantum fluctuations appear to have a twofold effect: on the classically ordered side below xc, the competing magnetic ground state is destabilized. On the overdoped side, the quantum fluctuations protect superconductivity at least partially from increasing disorder. This scenario is consistent with quantum criticality being a driving force behind the superconducting state in Ba(Fe1−xCox)2As2.

In the experimental work presented here, we have determined the crossover temperature T* separating the high-temperature quantum critical behavior from the (effectively) classical behavior at low temperatures. To this end, we have introduced a metric to identify the quantum critical regime in terms of the imaginary time response function, \(\tilde{{{\Lambda }}}(\tau )\), which can be inferred directly and unambiguously from experimental inelastic light scattering (Raman) data (see the section “Imaginary time response” in Methods and Lederer et al.29). By focusing on the longest possible imaginary time, τ = β/2 with the inverse temperature β = 1/kBT, we amplify the effect of the singular (power-law) correlations that reflect the critical behavior and maximally avoid contamination by non-universal analytic (short-range in imaginary time) non-critical portions of the imaginary part of the response function χ″. Specifically, if in the critical regime χ″ is a scaling function of Ω/T (i.e., of the marginal Fermi liquid form), this implies that \(\tilde{{{\Lambda }}}(\beta /2)\) is linear in T. In this way, as shown in Fig. 2, we can identify the quantum critical regime for each value of x as the range of (high) T over which \(\tilde{{{\Lambda }}}(\beta /2)\) is T independent, and a crossover scale T*(x) that bounds this regime from below. Applying this analysis to the present work leads to the phase diagram shown in Fig. 3, where for x < xc, the crossover is into a renormalized classical regime in which \(\beta \tilde{{{\Lambda }}}(\beta /2)\) is an increasing function of decreasing T, while for x > xc the crossover is into a quantum disordered regime in which \(\beta \tilde{{{\Lambda }}}(\beta /2)\) is a decreasing function.

Scaling

As a final remark, we wish to comment on the scaling behavior deduced from our data: From equation (S12) in Supplementary Note 2.4, \(\beta \tilde{{{\Lambda }}}(\beta /2)\) should vary as Ty where y = γ/νz. This implies y ~ 0 to be in line with a conventional scaling analysis presented in Supplementary Note 2, and thus one would have to appeal to an extreme value of one or more of these exponents. In this case, the most natural choice would be z = ∞, corresponding to the phenomenology of local quantum criticality33,34 in which only temporal correlations become long ranged at the quantum critical point. Such a scenario is of course exotic. Whether a local quantum critical point that violates scaling is indeed present in Ba(Fe1−xCox)2As2 is beyond the scope of this work and calls for further investigations of quantum criticality in this and related systems.

Conclusions

We tracked the B1g Raman response of Ba(Fe1−xCox)2As2 as a function of doping x. x is tuned from x < xc through a quantum critical point far into the overdoped regime of the phase diagram. From the data, we calculated the temperature dependence of the imaginary time-ordered correlation function \(\tilde{{{\Lambda }}}(\tau )\) at τ = β/2 and extracted crossover temperatures T*(x) above which Ω/T scaling prevails. These temperatures define the boundaries of the quantum critical fan corroborating the existence of a QCP around optimal doping. Thereby we show that the determination of the imaginary time dynamics from spectroscopic data is a straightforward way to reveal the effects of quantum criticality in the normal state of Ba(Fe1−xCox)2As2. The most important distinction is drawn between this analysis and any type of Kramers-Kronig analysis where an upper limit needs to be set. Here, the integral is naturally cut off at kBT by an exponential factor. The analysis is not limited to inelastic light scattering, but can be applied to a wide range of experimental probes as already outlined in Lederer et al.29. We hope that this transformation may enable the comparison of results from different spectroscopic probes directly and on the same footing, allowing a more unified analysis of quantum criticality.

Methods

Raman scattering

We performed inelastic light scattering experiments with the samples (for characterization, see Supplementary Note 3) attached to the cold finger of a 4He flow cryostat. Polarized photons having a wavelength of 577 nm (Coherent GENESIS MX-SLM577-500) hit the sample at an angle of incidence of 68∘. The polarized scattered photons were collected along the surface normal of the sample and focused on the entrance slit of a double monochromator. The photons transmitted at the selected energy were recorded with a CCD detector. The number of photons per second is proportional to the Van-Hove function S(q ≈ 0, Ω) = 1/π[1 + n(Ω, T)]χ″(Ω, T) where n(Ω, T) is the Bose factor and χ″ is the imaginary part of Raman response function which is displayed in Fig. 1. The factor R is the constant of proportionality which absorbs all experimental factors.

Imaginary time response

Following a method proposed in Lederer et al.29, we have used our measurements of the dissipative part of the response function, χ″, to characterize the dynamics of the corresponding imaginary time correlation function \(\tilde{{{\Lambda }}}(\tau )\) using the following exact identity:

$$\tilde{{{\Lambda }}}(\tau )=\int \frac{{{{{{{{\rm{d}}}}}}}}{{\Omega }}}{2\pi }{\chi }^{^{\prime\prime} }({{\Omega }},T)\frac{\exp [{{\Omega }}(\tau -\beta /2)]}{\sinh [\beta {{\Omega }}/2]},$$
(1)

with the imaginary time τ ∈ (0, β) and the inverse temperature β = 1/T where kB = 1 along the lines of Lederer et al.29. Since bosonic correlations have period β in imaginary time, the longest time response corresponds to τ ≈ β/2. In the following, it will be convenient to work with the specific quantity

$$\beta \tilde{{{\Lambda }}}(\beta /2)=\int \frac{{{{{{{{\rm{d}}}}}}}}{{\Omega }}}{2\pi T}\frac{{\chi }^{^{\prime\prime} }({{\Omega }},T)}{\sinh [\beta {{\Omega }}/2]},$$
(2)

which has the same universal properties as the static susceptibility \({\chi }^{\prime}({{\Omega }}=0,T)\) in the sense that the two quantities have identical scaling with temperature on cooling to both classical and quantum critical points29.

A key property of \(\beta \tilde{{{\Lambda }}}(\beta /2)\) is that it has a power-law dependence on temperature if χ″ obeys Ω/T scaling, and in particular is independent of temperature if χ″(Ω, T) is of marginal Fermi liquid form9, i.e., if it is a function of only Ω/T and not of either variable separately:

$${{{{{{{\rm{Im}}}}}}}}\bar{P}({{{{{{{\bf{q}}}}}}}}\to 0,{{\Omega }}) \sim {\chi }^{^{\prime\prime} }({{\Omega }}) \sim \frac{{{\Omega }}}{\max ({{\Omega }},T)}.$$
(3)

One significant virtue of \(\beta \tilde{{{\Lambda }}}(\beta /2)\) in comparison to \(\chi ^{\prime} ({{\Omega }}=0)\) is that it can be unambiguously computed from the measured Raman spectra. Since the measured χ″ (in this and other studies) fails to fall off at high frequency, the Kramers-Kronig integral relating χ″ to \(\chi ^{\prime}\) does not converge, and therefore requires a manual cutoff procedure. By contrast, the denominator in Eq. (2) grows exponentially for frequencies Ω ≫ T, rendering the integral convergent so long as the range of frequencies probed extends to several times the temperature.