Introduction

The application-driven discovery of functional materials is a high-risk, high-reward undertaking. Few materials reach commercial production for their intended application, but when they do, the payoff can be tremendous. For example, the discovery and subsequent application of GaN in blue light-emitting diodes (LEDs) and laser diodes has touched every aspect of the human experience from providing a low-cost affordable alternative to indoor lighting to its use in high-density recording media and full-color LCD display backlights. But materials discovery often suffers from major drawbacks. In the example of blue LEDs, GaN lacked lattice-matched substrates and failed to show p-type conductivity upon early doping attempts1. These challenges deterred the vast majority of LED researchers, who instead opted to study II–VI semiconductors which—despite obvious degradation problems—were attractive because they could easily be grown with high structural quality, achieve ambipolar doping, and be made into working devices—albeit short-lived ones2.

The ongoing search for ultra-wide bandgap (UWBG, Eg > 4 eV) semiconductors closely echoes the early stage of blue LED research, and it is motivated by the need for a semiconductor that can deliver efficient and compact switching for power conversion. The binary oxide β-Ga2O3 is winning the field’s attention due in part to—much like II–VI semiconductors—the commercial availability of large-area single-crystal lattice-matched substrates. This, and the material’s chemical simplicity, make β-Ga2O3 an attractive material system for large-area device fabrication while also eliminating the obstacles of mismatch-induced dislocations and cation nonstoichiometry. While β-Ga2O3 provides a robust platform for working devices, there remain several materials that may, if carefully investigated, present excellent prospects as UWBG semiconductors.

This work explores the mobility-limiting mechanisms in one such under-investigated UWBG semiconductor, SrSnO3 (SSO), by combining experiments and computation. SSO is a perovskite oxide with a bandgap of 4.1–4.6 eV3,4,5,6. Much like the well-studied perovskite BaSnO3, SSO’s conduction band is heavily derived from Sn 5 s orbitals, which results in a relatively low electron effective mass of m* = 0.4 me7,8. This property has been exploited in La-doped thin films grown by pulsed laser deposition (PLD) to produce films with room-temperature electron mobilities as high as 55.8 cm2 V−1 s−1 6,9,10,11,12,13. Transistors have also been produced using SSO as a channel material14,15,16,17 and improvements in fabrication have been made by optimizing ohmic metal contacts to SSO18. These promising results in a relatively new material system beg the question: what is the fundamental mobility limit of SSO? Ideally, this question should be answered with a joint effort in experiment and computation. However, experimental studies showing robust controllable doping of SSO are lacking, preventing this question from being directly addressed. Of the SSO films grown by PLD, the best dopant activation reported was 70% of the La dopants which was only possible after post-growth annealing6. In previous work, we showed a 1:1 correspondence between La dopants and charge carriers using secondary ionization mass spectrometry (SIMS) on 48 nm of MBE-grown SSO. However, thinner 12 nm films were shown to be compensated19. In a later work, we showed that the surface was responsible for this compensation, which can be negated by growing thicker films20. In this work, we systematically explore doping in SSO films exceeding 200 nm. By showing excellent doping control over nearly two orders of magnitude in carrier concentration, and using state-of-the-art calculations to investigate the mobility of SSO from first-principles, we establish SSO as a strong candidate UWBG semiconductor. SSO thin films achieve higher carrier concentrations, higher conductivity, and higher mobility at high carrier concentrations than the leading UWBG semiconductor β-Ga2O3. First-principles calculations of electron–phonon (e–ph) interactions, charge transport, and polaron effects provide a quantitative analysis of electrical transport in this semiconductor, calculating the room-temperature phonon-limited mobility at 76–99 cm2 V−1 s−1.

Results and discussion

Successful n-type doping with Nd

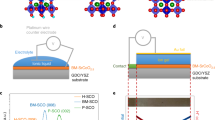
A series of samples with the structure shown in Fig. 1a were grown using hybrid molecular beam epitaxy (MBE) with identical conditions except varying the Nd effusion cell temperature TNd. Structural characterization using high-resolution X-ray diffraction (HR-XRD) confirmed phase-pure, epitaxial SSO films as shown in Supplementary Figure 1. SIMS was also performed on these samples using a Nd-implanted standard as calibration. However, as explained in detail in Supplementary Note 1, the many abundant isotopes of Nd make the SIMS results unreliable. Therefore, we rely on the relationship between the carrier concentration from Hall measurements and the Nd flux at the crucible orifice to infer the dopant activation. The Nd flux at the crucible orifice can be calculated from the kinetic theory of gases:

$${{{{{{{\mathrm{Nd}}}}}}}}\,{{{{{{{\mathrm{flux}}}}}}}}\,{{{{{{{\mathrm{at}}}}}}}}\,{{{{{{{\mathrm{orifice}}}}}}}} = \frac{P}{{\sqrt {2\pi mk_{{{{{\mathrm{B}}}}}}T_{{{{{{\mathrm{Nd}}}}}}}} }}$$

Where P is the equilibrium vapor pressure of Nd calculated with parameters from Alcock et al.21, m is the mass of a Nd atom, and kB is the Boltzmann constant. Fig 1b shows room-temperature carrier concentrations nRT as a function of the Nd flux at the crucible orifice. For a series with 100% dopant activation, nRT vs. Nd flux at the orifice should follow a straight line with an intercept of zero. We find that all samples with TNd ≤ 950 °C follow this trend with higher TNd leading to lower carrier concentrations. This behavior suggests 100% dopant activation with a doping limit of nmax = 2.0 × 1020 cm−3 similar to the results found for La:SSO19. In principle, a similar behavior could also be produced by a series where each sample has exactly the same dopant activation <100%. In practice, however, samples with <100% activation have activation percentages with a strong dependence on dopant concentration6,22,23, and therefore, should not yield a linear dependence. In fact, there are well-established theoretical explanations for this behavior24. For this reason, although not directly, the observed behavior is strong evidence of 100% activation in our samples. We note that Wei et. al. have reported slightly higher concentrations of 3.6 × 1020 cm−3 in La:SSO, which might be explained by their lower substrate temperature of 790 °C6. We also note that similarly high electron mobilities can be achieved in SSO grown by traditional ozone MBE (private communication with Prof. Darrell G. Schlom, 2021). The lower limit nmin = 3.7 × 1018 cm−3 is the lowest carrier concentration achieved to-date in conductive SSO, and it approaches Mott’s critical carrier concentration of 1.4 × 1018 cm−3, assuming an electron effective mass m* = 0.4 me7,8 and a static dielectric constant ε = 17.825. We show in Fig. 1c the room-temperature electron mobility (µRT) of these samples vs. nRT. Also shown for comparison are results for LaxBa1−xSnO3 (La:BSO) grown using the same radical-based hybrid MBE method26. The mobility of BSO is strongly dependent on carrier concentration and this dependence is attributed to the presence of charged dislocations as the dominant electron scattering mechanism26,27. Conversely, the SSO mobility is nearly independent of carrier concentration, with only a slight positive slope with increasing carrier density, suggesting an altogether different dominant scattering mechanism in SSO.

Fig. 1: Room-temperature electronic transport properties of NdxSr1−xSnO3 films.
figure 1

a Schematic of the sample structure. b Plot showing the room-temperature carrier concentration (nRT) from van der Pauw measurements as a function of the equilibrium Nd flux at the crucible orifice. c Room-temperature mobility (µRT) of NdxSr1−xSnO3 (SSO) films (as circles) compared to LaxBa1−xSnO3 thin films (as triangles) grown using the same radical-based hybrid molecular beam epitaxy method26. In all plots, the SSO marker color indicates the Nd effusion cell temperature.

Full size image

Temperature-dependent transport in SSO

To explore the mobility-limiting mechanisms in SSO, we measure temperature-dependent transport, as shown in Fig. 2. The data from the highest-doped sample—the one above the doping limit—was excluded for clarity. The resistivity vs temperature data in Fig. 2a and the temperature-independent carrier concentrations in Fig. 2b confirm that all the samples behave as degenerately doped semiconductors. Although the two lightest-doped samples show a resistivity minimum near 150 K, we show in Supplementary Fig. 2 that these two samples’ resistivities have a temperature dependence consistent with Kondo scattering28,29 suggesting that Nd3+ serves not only as a shallow donor, but also as a magnetic impurity. This result is not surprising given the unpaired f-electrons in Nd3+ (electron configuration [Xe]4f35d06s0) and the paramagnetism observed from Nd3+ impurities in structurally similar BSO30,31. The small downturn in carrier concentration below 25 K seen for the two lightest-doped samples is an artifact due to Aronov–Altshuler electron–electron interaction (EEI)32, so although the EEI is present in these two samples, it only manifests as an increase in the Hall coefficient below 25 K where the resistance is already saturated.

Fig. 2: Temperature-dependent transport data of NdxSr1−xSnO3 films.
figure 2

a Resistivity ρ, b carrier density n, and c mobility µ as a function of temperature. The color indicates Nd effusion cell temperature.

Full size image

The temperature-dependent mobility (µ) for these samples is shown in Fig. 2c. At low temperatures, there is a divergent behavior where the mobility of the lightest-doped samples increases with increasing temperature, while most samples show a monotonic decrease with temperature. At high temperatures, all samples have very similar mobilities that monotonically decrease with temperature. This mobility decrease is indicative of phonon scattering, and the similarities among samples with vastly different doping concentrations suggest that phonon scattering is the predominant mobility-limiting mechanism in SSO at room temperature. To understand the mobility-limiting mechanisms in SSO, we employ first-principles calculations (see “Methods” section) to study e–ph scattering processes and calculate the phonon-limited mobility as a function of temperature.

Electron–phonon interaction in SSO

Figure 3a shows the phonon dispersion curves in SSO along several high-symmetry lines in the Brillouin zone (BZ). Unlike perovskite oxides with simple cubic structure, such as SrTiO3 (STO), the phonon dispersions in SSO are quite complicated due to the structural distortion to orthorhombic symmetry, which leads to a large unit cell with 20 atoms. Each phonon branch in the simple cubic structure transforms into four branches in the orthorhombic structure due to BZ folding, resulting in 3 acoustic and 57 optical branches. We denote the four phonon branches above 80 meV as LO-1 and the four branches between 50 and 60 meV as LO-2 phonons; they correspond, respectively, to the highest and second-highest LO branches in the undistorted cubic perovskite structure33. The color scale on the phonon dispersions shown in Fig. 3a characterizes the e–ph coupling strength (in log scale) between different phonon modes and the electronic state at the conduction band minimum (CBM). Similar to cubic STO, the LO-1 and LO-2 phonons exhibit the strongest coupling with electrons due to the long-range Frohlich e–ph interaction34,35,36,37. Figure 3b, c shows the displacement patterns of the LO-1 and LO-2 phonons, respectively, in the long-wavelength limit. Both branches can be described as distortions of the SnO6 octahedra, with LO-1 phonons corresponding to stretching and LO-2 phonons to bending of the Sn-O bonds.

Fig. 3: Calculations of electron–phonon interactions in SrSnO3.
figure 3

a Phonon dispersions overlaid with a log-scale color map of the electron–phonon (e–ph) coupling strength |gv(k = G, q)|. b, c The atomic displacement patterns of the first longitudinal optical phonon branch of the parent cubic unit cell (LO-1) (a) and second longitudinal optical phonon branch of the parent cubic unit cell (LO-2) (b), the phonon modes that exhibit the strongest coupling with electrons. d Total and mode-resolved e–ph scattering rates (Γ) in SrSnO3 (SSO), computed at 300 K and n = 3.8 × 1018 cm−3. The black dashed line is the transport distribution function (TDF) which quantifies the contribution to transport from electronic states at a given energy. e The scattering rates and TDF for SSO doped into the deeply degenerate regime with n = 2.0 × 1020 cm−3. f Electron spectral function for the conduction band minimum (CBM) in SSO computed using the ab initio cumulant approach at temperatures of 200 and 300 K, and a carrier concentration of n = 3.8 × 1018 cm−3. For comparison, the electron spectral function in STO at 200 K is shown in g. The zero of the electron energy (E) axis in d–g is the energy of the CBM.

Full size image

Figure 3d shows the calculated e–ph scattering rates (Γ) at room temperature with a carrier concentration of 3.7 × 1018 cm−3. The scattering rates are separated into the contributions from LO-1, LO-2, and all other phonon modes. Figure 3d also shows the transport distribution function (TDF) which quantifies the contribution to the conductivity of electrons with a given energy—here the energy range of interest is primarily within 100 meV of the CBM. In this energy range, LO-2 and other phonons modes (except LO-1) give the strongest contributions to electron scattering. This trend is in noticeable contrast to the well-known perovskite semiconductor STO and other transition metal oxides, where only LO-1 and LO-2 are the main contributors to electron scattering due to their dominant Frohlich interaction33. This result points to the possibility of engineering higher mobility in SSO by fine-tuning the octahedral rotations and distortions, which are ultimately responsible for the BZ folding resulting in a large number of optical modes with 10–40 meV energy that limit the mobility.

The e–ph scattering rates are also shown for the degenerate doping regime with a carrier concentration of 2.0 × 1020 cm−3 in Fig. 3e. Although the scattering rates and TDF show a qualitatively different dependence on energy—troughing and peaking at the Fermi energy, respectively—the conclusion drawn from these data are unchanged from those at lower carrier concentration, namely that both LO-2 and other phonon modes (except LO-1) make significant contributions to the e–ph scattering.

In many oxides with ionic bonds, the e–ph coupling is strong enough to induce polaron effects38, whose treatment goes beyond the limit of weak e–ph interaction and band transport presented above. The electron spectral function, which describes the density of states of an electron in a given electronic state, can quantify such higher-order e–ph processes and polaron effects. Figure 3f shows the electron spectral function, as a function of electron energy E, for a state near the CBM in SSO, calculated using our cumulant approach37. In semiconductors with weak e–ph coupling, the spectral function exhibits only a single quasiparticle peak, whose width is proportional to the e–ph scattering rate. In ionic semiconductors and insulators with noticeable polaron effects, however, the spectral function may exhibit multiple peaks, including the original quasiparticle peak and one or more phonon satellites separated from the quasiparticle peak by the energy of the phonons with the strongest e–ph coupling37. The presence of these satellites is a signature of strong e–ph coupling, and is typically due to the coupling of electrons with LO phonons via the Frohlich interaction. The integral of the spectral function outside the quasiparticle peak (the so-called satellite spectral weight) is a measure of the strength of polaron effects and of the deviation of the transport mechanism from the conventional band transport regime37. The 200 K spectral function in SSO, shown in Fig. 3f, exhibits a sharp quasiparticle peak and weak sidebands due to the LO-1 and LO-2 modes; the main peak broadens at 300 K, but the overall weight of the polaron sidebands is still less than ~20%. For comparison, the spectral function in STO, shown in Fig. 3g, has a polaron sideband spectral weight of order 50% over a wide temperature range37. The weak polaron effect in SSO, which is partially responsible for its relatively high room-temperature mobility, is due to both the extended s-orbital character of the conduction band and the relatively weak Frohlich interaction between electrons and LO phonons in SSO due to the weaker ionic character of its bonds compared to STO (as quantified by smaller Born charges). By contrast, in STO the bonds are more strongly ionic and the conduction bands have d-orbital character, thus exhibiting both a smaller band velocity and a stronger overall Frohlich e–ph interaction and polaron effects.

First-principles mobility calculations

To clarify the microscopic origin of the room-temperature mobility, we carry out first-principles calculations of the temperature-dependent mobility of SSO, separately for each of the carrier concentrations investigated experimentally. The phonon-limited mobilities, which account for scattering from all phonon modes, are plotted as a function of temperature in Fig. 4a. The calculated room-temperature phonon-limited mobilities are 76–99 cm2 V−1 s−1 depending on carrier concentration. This dependence on carrier concentration is due to the change of scattering phase space for e–ph processes as a function of carrier concentration.

Fig. 4: Comparing experimental and calculated electron mobilities in NdxSr1−xSnO3 films.
figure 4

a Computed phonon-limited electron mobility as a function of doping, obtained by solving the Boltzmann transport equation with ab initio electron–phonon (e–ph) scattering. b Computed defect-limited carrier mobility using the effective mass model. c The computed carrier mobility, including scattering from phonons and ionized impurities, compared to the experimental data shown as symbols. d Comparison of the computed carrier mobility—including the effects from phonons, ionized impurities, and Kondo scattering—with the experimental data shown as symbols.

Full size image

The effect of the Nd dopants is included on top of the first-principles calculations using an effective mass model for ionized impurity scattering, as shown in Fig. 4b. Lower carrier concentrations show a stronger temperature dependence, because the Fermi level is near the band edge where the relaxation times due to ionized impurity scattering depend strongly on carrier energy \(\left( {\tau \propto E^{3/2}} \right)\). For the lower carrier concentrations, as temperature increases, the occupation probability above the band edge increases, leading to a strongly temperature-dependent carrier mobility. The mobilities from Fig. 4a–c are combined using Matthiessen’s rule, and the resulting mobility is compared to the experimental values in Fig. 4c. The computed mobilities match the experimental results exceptionally well at higher temperatures (>250 K), where the mobility is phonon limited and nearly independent of carrier concentration, but deviate from the experiment at low temperatures, especially for samples with low carrier concentrations. This discrepancy can be explained by Kondo scattering from Nd acting as a magnetic impurity.

To account for Kondo scattering, the residual from Fig. 4c is fit to an empirical expression for Kondo scattering obtained by Costi et al.39 and later fit to an empirical expression by Goldhaber-Gordon et al.40,41:

$$\mu _{{{{{\mathrm{K}}}}}}\left( T \right) = \mu _{{{{{{\mathrm{K}}}}}}0}\left( {1 + \left( {2^{1/S} - 1} \right)\left( {\frac{T}{{T_{{{{{\mathrm{K}}}}}}}}} \right)^2} \right)^S$$
(1)

We vary the prefactor \(\mu _{{{{{{\mathrm{K}}}}}}0}\) and the Kondo temperature TK, and fix the spin parameter S to the theoretical value S = 0.225 in Goldhaber-Gordon et al.40. The final mobility results—including phonon scattering, ionized impurity scattering, and Kondo scattering—are compared to experiment in Fig. 4d. The theoretical results closely match experiment for every sample. One feature that is not captured by the model is the small upturn in µ below 25 K which, as explained earlier, is an artifact of EEI which was not accounted for in our calculations.

Comparison to other UWBG semiconductors

To benchmark SSO as an UWBG semiconductor, we show in Fig. 5 room-temperature mobilities at different carrier concentrations in our SSO films along with their conductivities as a contour plot. For comparison, we include the highest conductivity values to date from thin films of well-known UWBG semiconductors, including β-Ga2O3 and AlxGa1−xN (AlGaN) for x = 0.3, 0.7, and 122,23,42,43,44,45,46. It is noteworthy that our doped SSO films show high conductivity of 2157 S cm−1 while maintaining a reasonably high electron mobility of 67 cm2 V−1 s−1 combined with a high carrier density, 2 × 1020 cm−3. We note that other UWBG semiconductors, such as AlGaN, can also achieve high mobilities (426 cm2 V−1 s−1 at a carrier density of 7.3 × 1014 cm−3) or high carrier concentrations (3.4 × 1019 cm−3 with electron mobility of 24 cm−2 V−1 s−1), but these properties cannot be achieved simultaneously43,47. Similarly, β-Ga2O3 films can achieve high room-temperature mobilities, 184 cm−2 V−1 s−1 at 3.2 × 1015 cm−3 48, or higher carrier concentrations of 1.7 × 1020 cm−3 but with lower mobility of 25 cm−2 V−1 s−149. In this context, our early attempt yielding record high mobilities with high carrier concentrations in perovskite SSO is especially promising. These results are particularly encouraging given that our SSO films were grown on lattice-mismatched substrates and have a high level of structural disorder as shown by rocking curves in Supplementary Fig. 1 and the full width at half-maximum values in Supplementary Table 1.

Fig. 5: Comparison to other ultra-wide bandgap (UWBG) semiconductors.
figure 5

A comparison of the room-temperature Hall mobilities of NdxSr1−xSnO3 (SSO) compared to the best-reported mobilities of other ultra-wide bandgap semiconductor thin films showing SSO’s superior mobility and conductivity above 1019 cm−3. The highest conductivity achieved in SSO from has been included from Wei et al.6. Data for homoepitaxial gallium oxide grown by metal-organic vapor phase epitaxy (MOVPE) are from Baldini et al.27. A gray dashed line has been added as a guide to the eye. Aluminum gallium nitride grown by MOVPE and aluminum nitride data grown by molecular beam epitaxy (MBE) are from refs. 28,29,30,31,32,33.

Full size image

However, the success of an UWBG semiconductor requires more than just high conductivity. In particular, high mobilities should be maintained well into the non-degenerate doping regime, ideally below 1016 cm−3. The lowest concentration we could achieve in this work was 3.7 × 1018 cm−3. It is unclear what prevented us from achieving conductivity at lower concentrations, but one likely culprit is the high structural disorder seen in rocking curves. Future work should focus on improving structural quality by producing lattice-matched substrates to SSO.

Another desirable quality for UWBG semiconductors is p-type doping. Many of the leading UWBG semiconductors are not amenable to p-type doping due to the prohibitively high activation energies or the formation of self-trapped holes50,51,52. At this early stage, it is unclear whether or not SSO supports p-type doping. But success in p-type doping of the related perovskite stannate BaSnO3 with K53 and N54 is encouraging for the prospects of p-type SSO. Future work should attempt similar doping strategies in SSO.

In summary, we have established perovskite SSO as an emerging candidate UWBG semiconductor through a joint effort of experiment and theory. The combination of controlled doping and detailed transport measurements with first-principles calculations of e–ph scattering and charge transport allowed us to understand and predict the transport behavior and mobility-limiting mechanisms in SSO.

Methods

Film growth and characterization

All samples were grown with a radical-based hybrid MBE approach. The reader is referred to previous publications for a detailed description of the method55,56, but a brief description is provided here. Single-crystal 5 mm × 5 mm GdScO3 (110) (CrysTec GmbH, Germany) were chosen as substrates for these films. This substrate choice was motivated by being the commercially available perovskite oxide substrate with the best lattice match (εm = −1.6%) with SSO films. It is also insulating and has the same Pbnm space group as SSO. For more information about substrate choice, the reader is directed to our previous works on the strain engineering of SSO8 and strain relaxation of SSO films20. The substrates were heated to 950 °C (thermocouple temperature) in the hybrid MBE system (Scienta Omicron, Germany). Growth was preceded by 20 minutes of oxygen cleaning via 250 W RF oxygen plasma that achieves a background oxygen pressure of 5 × 10−6 Torr (Mantis, UK). Strontium and neodymium were provided via thermal sublimation from an effusion cell. Strontium was supplied at 444 °C to achieve a beam equivalent pressure (BEP) of 2.4 × 10−8 Torr, whereas the neodymium effusion cell temperature was varied to control the dopant concentration. Tin was supplied by a radical-initiating chemical precursor hexamethylditin (HMDT) which offers both high volatility and high oxygen reactivity to help achieve cation and oxygen stoichiometry, respectively. HMDT was supplied at a BEP of 2 × 10−6 Torr. During growth, oxygen was supplied by the same oxygen plasma parameters that were used for oxygen cleaning. These conditions achieved a growth rate of 55 nm h−1. A 10 nm undoped buffer layer was used, motivated by its success in improving the mobility of the related material BaSnO326 although its efficacy has not yet been tested in SSO. High-resolution X-ray diffraction (HR-XRD) data were collected with a PANalytical X’Pert Pro thin film diffractometer with a Cu parabolic mirror and germanium 4-bounce monochromator. All transport data were collected in the van der Pauw geometry in the temperature- and magnetic field-controlled environment provided by a DynaCool physical property measurements system, (Quantum Design, USA). The magnetic field was swept between ±9 T.

Dynamic SIMS was performed on the Cameca IMS 7f-Auto at UC Santa Barbara. Ion implantation was performed on the undoped sample at Innovion Corporation (California, USA) to serve as a calibration sample. An O2+ primary beam was rastered across a 150 μm × 150 μm square to etch a trench into the film and produce secondary ions from which a 62 μm-diameter circle in the center of the trench was analyzed. All analyzed samples were coated in Au/Pt and an electron flood gun was used to minimize charging. Double focusing magnetic sector optics was used to filter secondary ions which used an electrostatic energy filter with a window width set to 150 eV followed by an electromagnet mass filter. The mass corresponding to 116Sn was chosen as the matrix signal.

Calculations of electron–phonon interactions and electron mobility

We carried out ab initio calculations on orthorhombic SSO with experimental lattice constants (a = 5.7093 Å, b = 5.7053 Å, c = 8.0676 Å). The ground-state electronic structure was computed using density functional theory (DFT) with the Quantum ESPRESSO code57. We employed the PBEsol-GGA exchange-correlation functional and norm-conserving pseudopotentials from PseudoDojo58. The kinetic energy cutoff for the plane-wave basis was set to 90 Ry. We use density functional perturbation theory (DFPT) to compute the lattice dynamical properties and obtain the e–ph matrix elements on electronic 8 × 8 × 6 k-point and phonon 4 × 4 × 3 q-point coarse grids using the Perturbo code36. The e–ph matrix elements were interpolated on finer Brillouin zone grids of up to 100 × 100 × 80 and employed to compute the e–ph scattering rates. The phonon-limited electron mobility was computed by iteratively solving the linearized Boltzmann transport equation with ab initio e–ph collisions36. The electron spectral functions were computed using a finite-temperature cumulant approach we recently proposed37.

The ionized impurity scattering mobility µimp was calculated using an effective mass model that uses an energy-dependent transport relaxation time \(\tau _{{{{{{{\mathrm{I}}}}}}}}\) due to ionized impurity scattering59

$$\mu _{{{{{{\mathrm{imp}}}}}}} = \frac{e}{{m^ \ast }}\frac{\langle{\tau _{{{{{\mathrm{I}}}}}}E}\rangle}{\langle E\rangle}$$
(2)

where e is the electron charge, \(m^ \ast\) is the electron effective mass, and E is the electron energy. The thermal average 〈A〉, where A is a physical quantity of interest, is defined as

$$\langle A\rangle = \int\nolimits_0^\infty {{{{{{\mathrm{d}}}}}}E\;A(E)\;g(E)\;f(E,E_{{{{{\mathrm{f}}}}}})}$$
(3)

The density of states g(E) was calculated from the effective mass assuming perfect band parabolicity:

$$g\left( E \right) = \frac{{2^{1/2}m^{ \ast 3/2}}}{{\pi ^2\hbar ^3}}E^{1/2}$$
(4)

where \(\hbar\) is the reduced Planck constant. The Fermi energy Ef used in the Fermi–Dirac distribution f (E, Ef) is calculated with an iterative method to match the carrier concentration n measured in the thin films:

$$n = \mathop {\int}\nolimits_0^\infty {{{{{{\mathrm{d}}}}}}E\;g} \left( E \right)\;f\left( {E,E_{{{{{\mathrm{f}}}}}}} \right)$$
(5)

Here we assume that all the dopants are fully ionized, that is, the carrier concentration n is equal to the doping concentration NI. The transport relaxation time due to ionized impurity scattering, \(\tau _{{{{{{{\mathrm{I}}}}}}}}\), is computed using:

$$\tau _{{{{{\mathrm{I}}}}}} = \frac{{16\pi \left( {2m^ \ast } \right)^{1/2}\varepsilon _0^2\varepsilon _s^2}}{{e^4N_{{{{{\mathrm{I}}}}}}}}E^{3/2}\left\{ {\ln \left( {1 + \frac{{8m^ \ast E}}{{{\hbar}^2q_{{{{{{\mathrm{scr}}}}}}}^2}}} \right) - \left( {1 + \frac{{{\hbar}^2q_{{{{{{\mathrm{scr}}}}}}}^2}}{{8m^ \ast E}}} \right)^{ - 1}} \right\}^{ - 1}$$
(6)

where εs is the static dielectric constant and NI is the concentration of ionized impurities assumed to be equal to the carrier concentrations in these films. The screening vector qscr is defined as

$$q_{{{{{{{{\mathrm{scr}}}}}}}}}^2 = \frac{{2^{1/2}m^{ \ast 3/2}e^2}}{{\pi ^2\hbar ^3\varepsilon _0\varepsilon _{{{{{{{\mathrm{s}}}}}}}}}}\mathop {\int}\nolimits_0^\infty {{{{{{\mathrm{d}}}}}}E\,E^{1/2}} \left( { - \frac{{\partial f}}{{\partial E}}} \right)$$
(7)

The effective masses computed with DFT using the PBEsol functional are m* = 0.38 me along the a-direction and m* = 0.36 me along the c-direction, in very good agreement with the experimental value of m* = 0.4 me7,8. Further refining the computed effective masses using hybrid functional (HSE) calculations on orthorhombic SrSnO3 (20 atoms per unit cell) would be demanding. Yet, we have verified that PBEsol and HSE calculations produce nearly identical effective masses and band dispersions in cubic SrSnO3 (5 atoms in the unit cell), where HSE calculations are feasible. In cubic SrSnO3, we found that the effective mass and the dispersion of the lowest conduction band is nearly identical in HSE and PBEsol: the effective mass is 0.234 me in PBEsol and 0.250 me in HSE. This is strong evidence that our PBEsol calculations are not affected by any errors related to the effective mass in PBEsol.