Introduction

Since the concept of laser wakefield acceleration (LWFA) being first proposed1, electron acceleration driven by femtosecond relativistic laser pulse has made remarkable progress2,3,4. Electron energy has reached 7.8 GeV with small energy spread5. Furthermore, electron beams with short pulse duration of a few femtoseconds6, small angular divergence of less than 1.5 mrad7 or beam charge up to 10 nC8 have been obtained. As the antimatter counterpart of electrons, positrons are novel sources for material science9, laboratory astrophysics10, and most of all, essential for future electron–positron colliders—an ultimate goal of laser-plasma acceleration11. Unlike electrons which naturally exist, positrons are to be generated in laboratories, usually via the Bethe–Heitler (BH) process12 and the Breit–Wheeler process13. The former relies on the collision between energetic electrons with the high-Z atomic fields. Copious positron production has been demonstrated based on either ultraintense laser directly irradiating metallic foils14,15,16 or sending the LWFA electron beams onto high-Z targets17,18,19,20.

However, acceleration of pre-generated positrons is not as straightforward as it is for electrons. A key issue is that the plasma wakefield valid for electron acceleration defocuses positively charged particles. Several methods have been proposed from simulations to mitigate the problem via wakefield driven by electrons21, hollow electron beam22, two electron beams23, vortex laser pulses24, and in plasma channel25. These methods generally require external injection of well-prepared positrons, a prominent challenge even for the more mature electron acceleration. In experiments, positrons are able to gain energy (tens of MeVs to several GeVs) from positron driven wakefiled26,27,28, which relies on tens of GeV dense positron beams only accessible in several large conventional accelerator facilities. Recently, laser-driven positron acceleration has been observed, where positrons created via the BH process in the high-Z foil gain ~MeV energies from the sheath field at the target rear surface15,29.

Here, we propose an all-optical approach by coupling the production, injection, and long-distance acceleration to generate positrons beyond GeV. We find that, when the dense electron bunch from LWFA emits from copper target into vacuum, the induced strong coherent transition radiation (CTR) is capable of trapping and accelerating positrons for a long distance. The CTR field provides an acceleration gradient up to tens of GV m−1, which is between that of the conventional accelerators (~100 MV m−1)30,31 and the plasma wakefield accelerators (~100 GV m−1). Our combined simulations (EPOCH-Geant4-EPOCH) demonstrate that a quasi-monoenergetic position beam (energy spread ~24% at peak energy ~500 MeV) with cut-off energy of 1.5 GeV. The beam is highly collimated (2.5 mrad angular divergence) and short pulsed (duration ~10 fs). About 9.4 pC beam charge is obtained by a 1.37 × 1020 W cm−2, 223 J driving laser pulse. The acceleration mechanism in our scenario addresses the key challenges in laser-positron acceleration and provides a practical approach to develop all-optical GeV-level positron sources.

Results and discussion

Overview of the scheme

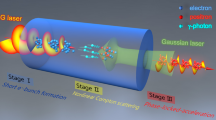
A sketch of energetic positron generation setup is shown in Fig. 1a. It is composed of three stages as follows: stage I is the generation of high-charge, high-energy electrons in LWFA; stage II is the positron generation in the copper target via the routine BH process; and stage III is the acceleration process for positrons. We simulate the electron acceleration, CTR, and positron acceleration via the particle-in-cell (PIC) code EPOCH32. The BH process is analyzed using the Monte-Carlo code Geant433. Connection between each process is made by conserving the beam features.

Fig. 1: Proposed setup and simulation results of particles’ energy spectra.
figure 1

a Sketch of the proposed setup. A laser pulse is focused over the gas jet, which accelerates electrons (blue sphere) to ~500 MeV with beam charge up to ~nC. The electrons with velocity of v further interact with the copper target. Positrons (red sphere) are generated via the Bethe–Heitler (BH) process and accelerated by longitudinal coherent transition radiation (CTR) field. The guiding magnetic field is 30 T. b Electron energy spectra after stage I, stage II/stage III (initial), and 13.3 ps (4 mm), 133.4 ps (40 mm), and 1334.3 ps (400 mm) in stage III. c The energy spectra of positrons after stage II/stage III (initial) and 13.3 ps (4 mm), 133.4 ps (40 mm), and 1334.3 ps (400 mm) in stage III.

Full size image

Simulation results of positrons generation and acceleration

To maximize the pair production events and the transition radiation intensity, in stage I we focus on generating high-charge electron beam at moderate kinetic energies. This is verified by two-dimensional (2D) PIC simulation code EPOCH. A circular-polarized laser pulse (wavelength λ = 0.8 μm, duration τ = 45 fs) with super-Gaussian spatial distribution and sin2 temporal profile interacts with gas plasma with a density downramp. Detailed parameters are shown in Methods. This setup guarantees robust and efficient injection of electrons in a single pass. After the laser pulse transverses the density transition region, several nC of electrons are loaded. Further acceleration generates a beam of a continuous energy spectrum with cutoff energy of 2 GeV, as shown by black solid line in Fig. 1b. The total charge of electrons (>5 MeV within a divergence angle of 26.2 mrad) reaches about 3 nC, estimated by multiplying the 2D results by the transverse size of the electron beam. The full width at half-maximum (FWHM) transverse size of the electron beam is 15.5 μm and the longitudinal length is 4.5 μm (FWHM). The proposed approach also works for linear-polarized laser pulses, as long as a spatially well-defined high-charge electron beam is created.

The high-charge electron beam from stage I impinges onto a 1 mm-thick copper target set 0.5 mm away from the gas target, triggering the generation of high-energy Gamma photons via bremsstrahlung34 and further production of electron–positron pairs from the BH process. In this case, the plasma collective effect is insignificant when the electron beam propagates through the target35 and this process can be accomplished by the Monte-Carlo simulation code Geant433 (see “Methods”), which also provides the energy and angular spectrum of both electrons and positrons after they exit the copper target. For the sampled 107 electrons, we obtain 5.03 × 104 positrons (generation efficiency ~ 0.5%). They show a Maxwellian energy spectrum of featured temperature Tp = 24.1 MeV, as shown by the black solid line in Fig. 1c. The positron beam has a divergence angle of 49.4 mrad (FWHM). We choose the 1 mm copper target thickness to make sure that substantial positrons are generated without significant energy loss for the electrons. The electron beam still contains 93.4% of its initial energy after the copper target. The beam is diverged due to the scattering. Its size is elongated to 44.8 μm (FWHM) in transverse and 5.3 μm (FWHM) longitudinally. The positron beam is in similar size as compared to the electron beam (42.5 µm × 5.3 µm), containing 12.1 pC beam charge according to the number ratio. Both electron and positron beams, leaving the copper target, co-propagate forward and overlap in space. Necessarily, the annihilation of positrons and electrons is negligible during the co-propagating process, as the lifetime of a positron is expressed as \(\tau = 1{\mathrm{/}}\left( {\pi r_{{\mathrm{e0}}}^2cn_{{\mathrm{e}}^ - }} \right)\), where re0 is electron classical radius and \(n_{{\mathrm{e}}^ - }\) is the electron density where positrons located. In our case, τ ~ 0.135 ms, positrons are able to propagate several kilometers without apparent annihilation.

In stage III, the electron beam exiting into vacuum from the rear surface of copper target drives intense CTR to accelerate positrons. The acceleration process is also verified by 2D PIC simulation (see “Methods”). Our PIC simulation confirms that when the electron beam emits into vacuum from the rear side of copper target, it induces strong CTR. The field co-propagates with the compound beam in vacuum and accelerates the forward-going positrons efficiently. The positron energy spectrum at different times in stage III are shown in Fig. 1c. A mono-energetic peak appears at the early beginning. After 400 mm propagation distance (1334.3 ps), the peak is accelerated to about 500 MeV. Energy spread is well reserved at about 24%. The cutoff energy reaches 1.5 GeV. This is accompanied by the gradual energy depletion for electrons, as shown in Fig. 1b.

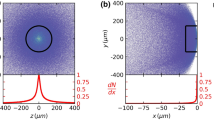
Figure 2a plots the density evolution of positron beam in this acceleration process and indicates that the positron beam keeps its high density as ~2 × 1015 cm−3 without significant increasing of the transverse size because of the focusing field. The beam is somehow compressed longitudinally. At 400 mm, the beam length of positrons decreases from 5.3 to 2.9 μm (FWHM), correspondingly to pulse duration of 9.7 fs. Some of the positrons, especially the ones located at the beam tail, undergo defocusing effect. The beam loses its charge by 2.7 pC in stage III. However, about 77% positrons are well preserved at the end of simulation. The focusing CTR field, as we will discuss later, results in good beam collimation. The angular divergence of the positron beam is 2.5 mrad (FWHM) and the normalized root-mean-squared beam emittance is 85.7 mm mrad. The phase-space distribution px − x in Fig. 2b shows continuous acceleration for the confined positrons through the 400 mm propagation distance. Accordingly, the distributions of the longitudinal electric field Ex at x = 4, 40, and 400 mm are given in Fig. 2c. The field shows a positive peak in the region occupied by electrons/positrons, followed by a decaying tail. One observes over 10 GV m−1 acceleration field at x = 4 mm. It then declines gradually during propagation. Nonetheless, the field remains above GV m−1 for the most part of the acceleration process.

Fig. 2: Simulation results of positrons’ acceleration process.
figure 2

a Positron beam density, b phase-space density of positrons, c longitudinal electric field Ex, and d transverse field at 4, 40, and 400 mm, respectively. The blue solid line in a represents transverse field at x = 4.01, 40.01, and 400.01 mm (denoted by the blue dashed line). The red solid line in b represents the on-axis longitudinal electric field. The longitudinal and transverse electric fields at 40 mm and 400 mm have been multiplied by four times and ten times, respectively. Simulation results at the beginning are shown in Supplementary Fig. 1 and Supplementary Note 1.

Full size image

One notices that the field Ex, shown by red solid line in Fig. 2b, decreases with x when x > x0 (x0 is the place where Ex peaks). For positrons mostly located at x > x0, the less energetic particles fall behind but experience higher field and gain more acceleration than those with higher initial energies. Therefore, the energy chirp is eliminated, leading to a narrow energy spread width as the acceleration goes on. The effect also induces beam compression to form a compact bunch of high density and short duration. In our simulations, we have not reached the stage of phase reverse. The focusing field for positrons is estimated by Ey − cBz, where Ey is the transverse electric field and Bz is the magnetic field in z direction, respectively. The 2D map and the lineout across the beam front are depicted in Fig. 2d and blue solid line in Fig. 2a, respectively. It has a peak value of 5 GV m−1 at 4 mm and is radially inward in the region occupied by the positrons, a typical structure that provides efficient focusing for positrons. It is worth mentioning that the radiation is coherent36, because the longitudinal length of electron beam (5.3 µm) is smaller than the radiation wavelength, which is ~9.6 µm obtained by transforming the field to the frequency regime.

Theoretical calculation

We start by studying the fields of an individual point charge in the case of the beam exiting a perfectly conducting plane at constant velocity. According to our simulation, a point charge e exits an infinite perfectly conductor plane x0 = 20 µm at constant velocity v. We shall also use spherical coordinates (R, θ, φ), with \(R = (x^2 + y^2 + z^2)^{1/2}\), the polar angle measured from the +x axis, and φ the azimuth angle, as well as coordinates (r, φ, x), with \(r = (y^2 + z^2)^{1/2} = R\sin \theta\), and x = R cosθ. The fields components are obtained37

$$E_x = \frac{e}{{4\pi \varepsilon _0\gamma ^2}}\left[ {\frac{{x - x{}_0 - vt}}{{S_ - ^3}} - \frac{{x - x_0 + vt}}{{S_ + ^3}}} \right]u(ct - R) \qquad \,\, \\ - \frac{{2\beta e\sin ^2\theta }}{{4\pi \varepsilon _0R(1 - \beta ^2\cos ^2\theta )}}\delta (ct - R){\mathrm{,}}$$
(1)
$$E_r = \frac{e}{{4\pi \varepsilon _0\gamma ^2}}\left[ {\frac{r}{{S_ - ^3}} - \frac{r}{{S_ + ^3}}} \right]u(ct - R) + \frac{{2\beta e\sin \theta \cos \theta }}{{4\pi \varepsilon _0R(1 - \beta ^2\cos ^2\theta )}}\delta (ct - R),$$
(2)

where \(\scriptstyle{S_ - \equiv \sqrt {(vt - (x - x_0))^2 + r^2{\mathrm{/}}\gamma ^2}}\), \(\scriptstyle{S_ + \equiv \sqrt {(vt + (x - x_0))^2 + r^2{\mathrm{/}}\gamma ^2}}\). The first terms of Eqs. (1) and (2), proportional to u(ct-R), are the Lorentz-transformed Coulomb fields of the moving charge. They are confined to the spherical volume R < vt, and drop quickly to zero at R = ct. The second terms are the radiation fields, confined to the infinitely thin shell at R = ct. The radiation fields decay as 1/R, whereas the Coulomb field decays as 1/R2. Then, the fields for a beam are constructed by summing those of elementary charges.

To verify the acceleration field caused by the CTR, which is three dimensional (3D) in Eqs. (1) and (2), we have done another 3D PIC simulation using the same beam parameters as in 2D simulation in stage III. Due to the limited computation recourses, we restrict the acceleration distance to be 2 mm. Simulations use a moving window with sizes of 40 µm × 200 µm × 200 µm divided into 200 × 200 × 200 cells with 1 macro particle per cell for electrons and positrons. The simulation result of longitudinal electric field Ex at 0.16 mm, plotted in Fig. 3a, shows that the maximum acceleration field reaches above 50 GV m−1, The peak value and the field distribution matches the longitudinal field of the CTR shown in Fig. 3c, which is calculated by the second term of Eq. (1). We notice that the CTR field is two orders of magnitudes higher than that of Coulomb fields [shown in Fig. 3b, calculated by the first term of Eq. (1)]. As an estimation for the latter, we calculated the potential generated by electrons via Poisson equation \(\nabla ^2\phi ^{\prime} = - n_{\mathrm{e}}^\prime {\mathrm{/}}\varepsilon _0\), where \(\nabla ^2 = \partial ^2{\mathrm{/}}\partial x^{\prime 2} + \partial ^2{\mathrm{/}}\partial y^{\prime 2}\) is the Laplace operator, ϕ′ and \(n_{\mathrm{e}}^\prime\) are potential and electron density in co-moving frame, respectively. Then the electric field in co-moving frame is obtained by E′ = −∇ϕ′. The laboratory results of the longitudinal Coulomb electric field \(E_x = E_x^\prime\), whose magnitude is also two orders less than the simulation. Thus, such a strong acceleration field in the simulation stems from the CTR, rather than its own Coulomb field. For transverse electric field Ey = Er cosφ, CTR still accounts for the vast majority.

Fig. 3: Comparison of simulation results with theory.
figure 3

a Simulation results of longitudinal electric field at x ~ 0.16 mm. b Longitudinal Coulomb field calculated by first term of Eq. (1). c Longitudinal coherent transition radiation (CTR) field calculated by second term of Eq. (1). d Simulation results of transverse electric field at x ~ 0.16 mm. e, f The first and second terms of Eq. (2), representing the transverse Coulomb field and transverse CTR field, respectively. g Theoretical (blue solid circle) and simulation (red square) results of the maximum on-axis value of the longitudinal electric field at different times.

Full size image

We plot maximum on-axis value of the longitudinal electric field at different times. The CTR field plus Coulomb field (blue solid circle) from Eq. (1) agree well with the simulation results (red square), as shown in Fig. 3g. We notice that the plasma PIC simulation results agree well with the theory for conductor material. A possible reason is that abundant free electrons exist in both media. In our calculation, we neglect the contribution of positrons to the field, as the initial positron density is lower than the electron density by two orders of magnitudes.

Discussion

Further, we explore the acceleration dependence on the drive beam energy by 3D simulations, where the beam profile remains the same as the one in Fig. 3. To compare with the CTR theory, the central energy of the electron beam is tuned from sub-MeV to GeV with mono-energetic spectrum and zero angle divergence. Figure 4a shows the peak value of the acceleration field scaling with the electron energy, obtained at 46.9 μm where the electron beam completely exits the copper target. One sees an uprising in the region of 1–10 MeV and then a saturation to about 100 GV m−1 level. The latter comes from the relativistic effect. When γ ≫ 1, the radiation field for an individual charge is ~Ex ∝ 2βe/R according to the second term of Eq. (1), i.e., the dependence on the electron energy vanishes. The theoretical calculation from Eq. (1) all matches well with the simulation results in the considered energy region. In Fig. 4b, we show the scaling of the acceleration field as a function of the electron beam charge, while the electron energy is fixed to 500 MeV. We see that the field linearly increases with the charge Q, indicating that the transition radiation power is proportional to the square of the number in the electron beam (W ∝ Q2), which again clearly confirms that the radiation here is coherent. In terms of the field strength, we find that it is more efficient to increase the beam charge rather than the beam energy, which is why we employed a large focal spot size for the laser pulse to maximize the beam loading in LWFA.

Fig. 4: Scalings of longitudinal electric field versus the electron beam energy and charge.
figure 4

a Simulation (red) and theoretical results (blue) of the longitudinal electric field Ex as a function of the central energy of the electron beam. b Scaling of the longitudinal electric field Ex versus the electron beam charge.

Full size image

As for the role of the confining magnetic field, we did 2D simulation for stage III when the external magnetic field is 1/100, 1/10, and 1/2 of 30 T. Other parameters are the same with the main 2D simulation. When the external magnetic field reaches beyond 10 T, positrons are well confined as a compact bunch, shown in Supplementary Fig. 2. Essentially, the confining magnetic field strongly depends on the divergence angle of positrons beam, which originally comes from the LWFA electron beam in stage I. The requirements for the external magnetic field will be more tolerant if the electron bunch is more collimated in stage I. In experiments, it is necessary to deplete laser pulse energy (e.g., increase the jet-foil distance or put a laser blocker between stage I and stage II) to avoid the laser–solid interaction38,39,40,41.

In conclusion, we proposed a scheme for GeV-level positron acceleration. The positrons created from the routine BH process in a copper target gain high-energies from the longitudinal electric field induced by the CTR owing to co-propagating electrons exiting into vacuum. Both simulations and theories show that the field approaches tens of GV m−1 and sustains for a long distance. Further, increasing the beam charge (rather than the beam energy) is more efficient in enhancing the field strength. Our series of simulations (EPOCH-Geant4-EPOCH) confirm the generation of a quasi-mono-energetic positron beam with central energy at about 500 MeV with an energy spread of 24.3% and a small angular divergence of 2.5 mrad (FWHM). For further acceleration, one could utilize two or more copper targets to provide multistage CTR acceleration. A detailed study will be included in our future work. This all-optical acceleration scheme is available in 100 TW ~ 100 PW laser facilities and offers a promising way to provide high-energy positron sources, which has a wide range of applications in material science, laboratory astrophysics, and potentially, as an injector for future high-energy accelerators.

Methods

Stage I: PIC simulation for electron beam acceleration

This is verified in 2D simulations in a moving window of Lx × Ly = 180λ × 360λ (2000 × 750 cells). Each cell is filled with ten macro particles. A circular-polarized laser pulse (wavelength λ = 0.8 μm, duration τ = 45 fs) with super-Gaussian with exponent 4 spatial distribution and sin2 temporal profile propagates along the x direction from the left side. The super-Gaussian profiled high power laser beams, which is a better candidate to laser particle acceleration42, are being developed in several laboratories43,44,45,46 and 10 PW-level lasers are basically super-Gaussian profile because of the full use of the amplification medium. The dimensionless laser field amplitude is \(a_0 = eA{\mathrm{/}}m_{\mathrm{e}}c^2 = 8{\mathrm{/}}\sqrt 2\) corresponding to an intensity of 1.37 × 1020 W cm−2, where A is the vector potential, e and me are the electron charge and mass respectively. A relatively large focal spot diameter of r0 = 60 λ is employed to increase the loaded beam charge47. This corresponds to peak laser power of 4.95 PW, accessible in facilities such as ELI48, SULF46, Apollon49, Vulcan50, etc. We use the shock-front injection51,52 in the gaseous plasma target: the density increases sinusoidally from 0 to 3 × 1018 cm−3 within 290λ, then decreases sinusoidally to 2 × 1018 cm−3 after a short transition length of 10λ and maintains afterwards till 13.1 mm.

Stage II: Monte-Carlo code for positron generation

Limited by the computational resource, we sample the electrons generated from stage I at 5.34 × 10−4 ratio (~107 simulation particles) by reserving the energy and angular spectrum and input them in the Geant4 simulations. The copper target with thickness of 1 mm is set 0.5 mm away from the gas target. The detection device is at the rear side of the copper target.

Stage III: PIC simulation for positron acceleration

The acceleration process is verified by 2D PIC simulation using a moving window of 40 µm × 400 µm divided into 400 × 1200 cells with 5 macro particles per cell for both electrons and positrons. The hybrid beam taken from stage II is initialized in the copper target. The plasma density is nCu = 8.49 × 1022 cm−3 and covers the region 0 < x < 20 µm. The initial electron beam containing 3 nC beam charge is approximated by a Gaussian density profile \(n_{\mathrm{e}} = n_{{\mathrm{e0}}}\exp ( { - x^2{\mathrm{/}}\delta _{{\mathrm{e}}x}^2 - y^2{\mathrm{/}}\delta _{{\mathrm{e}}y}^2} )\), where ne0 = 1.46 × 1018 cm−3, δex = 3.18 μm (δFWHM = 5.3 μm), and δey = 26.9 μm (δFWHM = 44.8 μm), respectively, according to the numbers in stage II. The 12.1 pC positron beam is profiled with δpx = 3.18 μm (δFWHM = 5.3 μm) and δpy = 25.5 μm (δFWHM = 42.5 μm), but a lower density of np0 = 6.5 × 1015 cm−3. Electron energy distribution is taken from stage II. Here we have considered the transportation effect in the copper foil for electron spectrum initially shown by the black solid line in Fig. 1b. Positrons have an initial Maxwellian energy distribution at temperature Tp = 24.1 MeV. We apply an external longitudinal magnetic field of Bx = 30 T to guide the electron/positron beam propagating in vacuum for long distances. Such fields are now available using superconducting material53.