Introduction

The bottleneck of most all-optical switching systems is the fan-out capability1, that is, one would ideally like to be able to switch several photons with only a single one2,3,4. Even the more modest requirement of switching a photon with exactly one other photon already imposes a major challenge for most photonic switching schemes. One frequently suggested measure against poor switching efficiency is local field enhancement inside a resonant optical cavity5,6. However, the resulting increase of the effective nonlinearity comes with a caveat, namely channel separation. Both interacting fields have to be launched collinearly, leaving only the wavelength as a parameter for channel separation7,8. Moreover, the resonant nonlinearity enhancement is inevitably accompanied by a decrease in switching speed and an increase of intracavity dispersion, which eventually induces a substantial group delay difference between the two interacting light pulses. Slow light has been suggested as another means for enhancing the interaction time of two pulses in a χ(3) nonlinearity9, but this idea suffers from some of the same problems as it also requires the introduction of rather large dispersion. A further criterion to allow optical technologies to compete with electronic devices is the switching time. Nowadays efficient all electronic devices with record breaking ultrafast switching times of few picoseconds10 have been demonstrated. This is on par with demonstrated all-optical concepts, enabling switching times of 1 ps11. Recent experiments suggest that all-optical switching times of 260 fs12 or even below 30 fs13 may be possible.

Further prospects arise from the rapid development of integrated photonic silicon nitride waveguides, which represent a powerful alternative to nonlinear fibers, and allow on-chip generation of supercontinuum (SC) spectra14,15,16,17. The underlying physics in these devices is mostly identical, yet their intrinsically higher nonlinearities promise energy scaling by orders of magnitude compared to photonic crystal fibers18,19. Specifically, it has recently been shown that microring combs enable data rates of hundreds of terabits per seconds20, and it was suggested that cavity solitons from these devices may revolutionize high-speed optical communications21. Despite all the progress in the field, one key component is still missing, namely a device that would enable time-division multiplexing at data rates beyond 100 Gbit/s, and this gap could obviously be filled by an all-optical transistor with near-unity fan out.

We recently proposed a concept22,23 enabling high mutual interaction for all-optical manipulation. Key idea to this concept is an effective enhancement of a χ(3) nonlinearity inside a dielectric material without any need for a resonant enhancement. Dispersion properties of almost all dielectric materials enable copropagation of light pulses at two exactly matched wavelengths in opposite dispersion regimes. Dispersive waves (DW) in the normal dispersion temporally stretch upon propagation. In contrast, in the anomalous dispersion regime, short pulses form solitons, which propagate without temporally dispersing over long distances. As solitons maintain a high peak power upon propagation, they increase the refractive index in their immediate surroundings via the weak χ(3) Kerr nonlinearity of the dielectric material24,25. This effect can lead to deceleration or acceleration of the copropagating DW in the leading or trailing part of the soliton22,26 and to trapping of the dispersive radiation19,27.

The process underlying our switching concept has been strongly inspired by findings in other fields of physics. Fluid dynamics reported a wave reflection process28, which has analogously been rediscovered in optics29. Earlier, this switching process was discussed as an optical analogy to the event horizon30, and has been used to investigate diametric acceleration allowing to mimic interaction with negative masses31,32, quantum bouncing in cold atomic clouds33, or optical rogue wave emergence34,35,36. The latter example can be understood as quantum noise induced optical switching, demonstrating the potential for minute fluctuations controlling large optical energies. Moreover, the analogy drawn to the kinetics of an event horizon30 demonstrates the strength of the interaction process and its potential for applications in optical technologies. However, early experimental realisations have been less efficient than anticipated37, and the overall concept is just beginning to develop38.

A key point for overcoming the previous shortcomings is the choice of incommensurate frequencies in the nonlinear interaction scheme in order to establish long lasting mutual interaction between two pulses. Consequently, given group-velocity matching of the DW to the soliton, both pulses remain temporally tightly locked, which acts similar to a resonant enhancement of the χ(3) interaction, yet avoids the dispersion problems of the microcavity approach. The emerging temporal lock can be exploited for all-optical switching applications22,39,40. In particular, it has already been shown that an about seven times smaller DW already suffices to spectrally shift the soliton by its full width at half maximum, i.e., the scheme fulfills the fan-out criterion. However, efficient exploitation of this switching scheme requires pulse parameters which are not immediately accessible with available laser technology.

Here, we experimentally demonstrate that a far more efficient switch can be built when a high order rather than a fundamental soliton is used. When injected near the zero-dispersion wavelength (ZDW) of the fiber, one typically expects to see a rapid decay of the high-order soliton into fundamental ones, i.e., the well-known process of soliton fission41,42,43,44,45. Subsequently, the fundamental solitons experience a red shift from the Raman effect46,47, which, in combination with four-wave mixing processes, eventually leads to the formation of a SC. Launching a DW into the soliton fission cascade now enables controlled and deterministic modification of the outcome of this highly nonlinear process. In our scheme, soliton and DW play the role of emitter and base of an optical transistor, respectively, and a third wavelength channel acts as the collector. Other than in a conventional bipolar transistor, however, our optical switching scheme does not dissipate energy and is therefore completely conservative.

Results

Experimental realization of an optical supercontinuum switch

For a demonstration of our switching concept, we conducted a series of experiments, in which we launched a soliton and a DW with controllable timing into a fiber. The experimental setup is shown and described in Fig. 1a–d (see “Methods” section for details). Conditions are chosen such that both pulses propagate for a certain propagation distance within the fiber at nearly identical group velocity. Due to the Raman self-frequency shift, therefore the initial conditions slightly deviate from exact group-velocity matching. Moreover, we have chosen wavelengths such that we can additionally benefit from phase matching of the four-wave mixing process, choosing a wavelength of 680 and 810 nm for the DW and the soliton, respectively. Figure 2 visualizes the switching concept with simulations of a simplified interaction process with only one fundamental soliton rather than a SC spectrum. Simulations have been performed for the generalized nonlinear Schrödinger equation (GNLSE) for the complex field envelope18 as well as for the forward unidirectional propagation equation for the analytic signal48,49 (see “Methods” section for more detail). Both approaches lead to the same results, showing qualitatively near-perfect agreement with the experiments. Parameters for the fundamental soliton have been taken from the experimentally observed ejected solitons, exhibiting pulse widths in the range of 20 fs. To unveil all different mechanisms involved, we have chosen a relatively high intensity for the DW (IDW/Isol  = 3/4), which still enables to switch a stronger pulse by a weaker one with respect to the fan-out criterion, identical to the ratio used in the experiments. Figure 2a, the panel denoting t0 = 800 fs, shows the case where nearly no interaction between the soliton and the DW is initiated. Due to the Raman induced soliton-self-frequency shift, the DW is initially not group-velocity matched to the soliton. With decreasing the delay, see Fig. 2a t0 = 200 fs panel, group-velocity matched interaction can eventually be realized after propagation for about 0.3 m. In turn, this synchronization leads to a stronger deceleration of the soliton accompanied by a “reflection” of the DW. In the frequency domain, this reflection manifests itself as blue and red shift for the DW and soliton, respectively. The opposite behavior is observed at initial negative delay at t0 = −50 fs, as depicted in Fig. 2b. In this exemplary case, one can therefore easily overcome Raman deceleration. The switching dynamics in dependence of time delay are shown in Fig. 2c, with output spectra emerging at z = 1 m. This behavior agrees well with earlier predictions22,23.

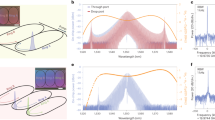
Fig. 1: Schematic of the experimental setup for all-optical switching.
figure 1

a Optical parametric chirped pulse amplifier (OPCPA) delivers 7 fs pulses with a spectrum spanning from 650 to 1150 nm. Dedicated pulse shaper delivering two pulses, i.e., a dispersive wave (DW) and a higher-order soliton (S), with tunable frequencies and adjustable relative delay. Both pulses are coupled into a photonic crystal fiber (PCF) using a microscope objective (MO). Output of PCF is collimated using a second microscope objective and coupled into a spectrometer. b Spectrum of the OPCPA (black line). Gray shaded areas depict wavelengths of soliton and DW that are used as input for interaction process. c Inverse group-velocity profile of PCF \({\beta }_{1}^{-1}\) (black line) and resulting group-velocity dispersion β2 (blue line), respectively.

Full size image
Fig. 2: Variation of the interaction between one fundamental soliton and a dispersive wave (DW) for different initial time delays.
figure 2

The initial soliton and DW parameters are kept fixed (tsol = 20 fs, λsol = 860 nm, tDW = 70 fs, λDW = 640 nm) with an intensity ratio of 4/3. a Temporal and spectral dynamics for collision processes at the trailing edge of the soliton (initial time delays are superimposed). b Corresponding dynamics for collision processes at the leading edge of the soliton (initial time delays are superimposed). A parameter sweep over the initial time delay is shown as Supplementary Movie 1. c Output spectra at z = 1 m in dependence of the initial time delay. d Induced frequency shift of the soliton in dependence of the delay time demonstrating the efficient manipulation of the soliton in both directions. e Extracted energy from the DW involved in the scattering process revealing the different areas of fan-out capability.

Full size image

To explore the fan-out potential of our switching concept, we plotted the frequency shift of the soliton (Fig. 2d), which is representative for the control together with the amount of energy (photon number ratio) of DW/soliton (Fig. 2e). This analysis indicates that significantly lower energies of the DW may be sufficient for controlling the soliton, yet it is also evident that this comes at a price. To this end, we performed a series of simulations, slowly decreasing the amount of energy of the DW. Soliton control remains down to a ratio 1/5. Further improvement of this record fan-out appears possible with solitons of longer pulse duration. Figure 3a, b depicts the output spectral range, demonstrating resulting frequency shifts for the affected pulses in dependence of their initial time delay in accordance to Fig. 2c. Numerical simulations for comparison are shown in Fig. 3c, d (see below for further details). At large delays, there is no interaction of soliton and DW, and one can observe the undistorted decay of the higher-order soliton. Due to the soliton-self-frequency shift, the first split-off fundamental soliton has experienced a red shift to 1100 nm. A secondary soliton is located at 900 nm.

Fig. 3: Supercontinuum switching with a dispersive wave (DW).
figure 3

a Experimental output spectral range of the DW and b of the solitons in dependence on the initial delay between soliton and DW. Two panels were used because measurements were made with two different spectrometers, therefore intensity scales are not immediately comparable. c, d Corresponding numerically received output spectra averaged over an ensemble over 100 disorder instances for each time delay. In both cases the switching mechanism appears significantly.

Full size image

In our experiments, we used a fiber with two ZDWs. Under these conditions, the second ZDW (ZDW2) of the used fiber at 1250 nm imposes a barrier for further red shifting of the solitons. This effect makes our scenario relatively insensitive to energy fluctuations of the soliton, as the first split-off fundamental soliton will automatically accumulate in a wavelength region below ZDW2. In the language of Miller’s criteria1, therefore, the choice of a fiber with two dispersion zeros removes the necessity of critical biasing for the 1100 nm output channel. A further advantage results from the fact that the interaction of a DW with a SC has a strong impact on the suppression of rogue solitons50.

The center wavelengths of the output solitons can now be manipulated by reducing the initial delay. In particular, launching the DW about 0–500 fs ahead of the soliton (S), the soliton fission process is inhibited, and the spectral content at 1100 nm appears significantly reduced (Fig. 3b). At the same time, the launched DW is completely blue shifted from 680 to 540 nm, and superimposes the much weaker dispersive radiation generated in the fission process (see Fig. 3a). From a switching logic point of view, the output at 540 nm can be considered as S & DW while the 1100 nm output appears when S & \(\overline{{\rm{{D}}W}}\). The most interesting observation is that input light at 680 nm does not experience any frequency shift when \(\overline{{\rm{S}}}\) & DW holds, i.e., this channel is cascadable in the sense of Miller’s criteria1. At the same time, the 680 nm channel also shows the most impressive switching contrast among all three logical AND functionalities observed. The experimentally observed logic operations are summarized in Table 1 and translated into an equivalent complementary metal oxide semiconductor (CMOS) circuitry, consisting of three field-effect transistors, shown in Fig. 4. Our optical transistor enables three parallel operations, does not rely on resonant conditions, and operations can be executed for different combinations of DW and soliton frequencies.

Table 1 Optical multigate functionality.
Full size table
Fig. 4: Schematic representation of the switching characteristics of the optical transistor, using the symbols of complementary metal oxide semiconductor (CMOS) technology for source (S), drain (D), and gate (G).
figure 4

These electronic devices come in two variants, which are known as enhancement or depletion type, indicated by either a dashed or solid line between S and drain D, respectively. The situation shown presumes temporal overlap between soliton and input dispersive wave (DW in). In this situation, soliton and DW give rise to a pulse at output port (DW 2), with no output at ports (DW out and soliton out). For other situations, please refer to Table 1.

Full size image

The findings in Fig. 3 are in excellent agreement with the previously suggested concept for an optical transistor22 and the example shown in Fig. 2, with the noted exception that a much higher soliton order was employed now. Even though the pulse energy ratio of the DW to the soliton is with 3/4 higher than in ref. 22 in our current experimental demonstration, the resulting switching efficiency exceeds the previous predictions, in particular as input channels and output channels of the gate functionalities are now separated by at least 100 nm. In view of the fan-out criterion, we have also to keep in mind, that the DW is used to switch several solitons in parallel and not only one.

Numerical simulation of the all-optical switch

To corroborate our experimental findings and understand the dynamics in more detail, we additionally conducted a number of numerical simulations. The output spectra were computed with identical conditions matched to the experiment as best as possible. In particular, as the soliton fission scenario is infamous for its high susceptibility to energy fluctuations on the quantum noise level, we simulated the propagation of pulse ensembles with suitably chosen pulse energy spread. In particular, all major features of the gate functionalities are perfectly reproduced, yet there appear changes in the exact frequency shifts, which we explain by our imperfect knowledge of the exact dispersion properties of the fiber under test. In order to verify this assumption, we performed additional simulations with dispersion profiles differing slightly from the manufacturer specifications. Moreover, we also detect a possible influence of a chirp51 on the input pulses. Our numerical simulations further indicate that we can adapt the number of output gates by changing input peak intensities.

Figure 5 depicts the temporal and spectral evolution of the interaction for the three exemplary cases, i.e., positive (Fig. 5a), zero (Fig. 5b), and negative (Fig. 5c) initial time delay and the corresponding spectra (Fig. 5d–f), respectively (see also Supplementary Movie 1). Figure 5g–i shows the corresponding cross-correlation frequency-resolved optical gating (XFROG) traces at the output (see “Methods” section for more detail).

Fig. 5: Propagation dynamics of the interaction.
figure 5

a–c Temporal and d–f spectral evolution (see also Supplementary Movie 2) of the collision process with initial negative, zero, and positive delay, respectively. g–i Corresponding cross-correlation frequency-resolved optical gating (XFROG) traces at the output of the fiber (see also Supplementary Movie 3). Negative and positive initial delays lead to acceleration or deceleration of the ejected solitons, whereas the direct superposition involves strong four-wave mixing process, generating new frequencies in combination with the ability to supress the soliton fission process itself. Clear differences of the scenarios can also be seen in the XFROG traces.

Full size image

In these simulations, one can see that several fundamental solitons are ejected in the process of soliton fission44, which are subsequently subject to the interaction with the DW. Depending on time delay, the solitons are controlled by the DW. It even appears possible to simultaneously control multiple solitons by a single DW, which allows for some adaption of the number of channels in the switching scheme. Specifically, the ejected solitons are accelerated or decelerated due to collisions with a DW at the leading or trailing edges, respectively22. This process is accompanied by corresponding shifts in the soliton frequency. Moreover, our simulations indicate the important role of a further four-wave mixing process in the scheme, as mentioned above. Figure 5b, e shows the temporal and spectral dynamics for direct overlapping initial pulses. In the temporal domain it is visible that the soliton fission process itself is affected, namely the ejection of fundamental solitons is inhibited. In the frequency domain, the inhibition enables four-wave mixing mediated generation of two new frequency components ω1 = 2ωDW − ωS and ω2 = 2ωS − ωDW. This mechanism can be understood directly from the interaction of one fundamental soliton with a DW at the initial unperturbed frequencies as given in the experiment. In the more complex SC generation process this interaction scenario has even a stronger effect as one can switch on and off the whole process, as demonstrated by the XFROG trace in Fig. 5h (and Supplementary Movie 3). Given that this overall observed behavior may suggest a probabilistic character of the interaction rather than a deterministic action expected of a logic gate, we carefully investigated a large number of simulations, which indicated that collision suppression never fails, see numerically averaged simulations in Fig. 5c, d. As we already described theoretically in ref. 50, this effect arises due to suppression of solitonic collisions in the presence of an additional DW. We further emphasize that this problem mostly affects the AND functionality of the soliton channel.

Discussion

We experimentally demonstrated a new route toward optical switching that circumvents most of the complications present in resonant enhancement. Our concept exploits the “event horizon” condition, exploiting higher-order solitons for the implementation of three different all-optical AND gate functionalities with switching times in the femtosecond regime. The logical gate complies with the fan-out criterion1, which is difficult to implement otherwise, i.e., we can switch a larger soliton energy by virtue of a DW with less energy. Moreover, critical biasing can be suitably avoided by choosing a fiber with two ZDWs, and the system is immediately cascadable in one of the three output channels. We further expect that a suitable choice of ZDW2 may also enable cascadability for the soliton output channel. Increasing ZDW2 to 1600 nm, one could use an erbium fiber amplifier to restore the soliton energy prior to frequency-doubling it back to 800 nm input wavelength, which may then also enable cascadability of the second input channel. Suitable dispersion engineering provided, it appears conceivable to also exploit other active fibers for mapping the soliton output port back to the DW input. Of course, this will require specifically designed fibers or waveguides. In particular, silicon-based platforms are highly promising, as they promise to lower pulse energies by at least two orders of magnitude compared to our experimental demonstration in this paper. The latter feat enables, e.g., implementation of THz time-division multiplexing schemes with sustainable energy consumption in the few-watt range and may be further improved to sub-watt power levels. In conclusion, while optical logic circuits are certainly still lagging ages behind electronic ones, we nevertheless believe that our experimental demonstration complies with Miller’s rules as best as demonstrated to date. Admittedly, at the same time, we may have barely reached the level that Bardeen and Brattain already demonstrated in the late 1940s: there is still a long way to go from the first experimental demonstration of a Miller-compliant logic gate functionality to a fully functional and useful optical logic circuit.

Methods

Experiments

In our experiments, we used a setup consisting of a noncollinear optical parametric chirped pulse amplification (OPCPA) system delivering energetic broadband pulses and a pulse shaper. The OPCPA delivers sub-7 fs pulses with  >1.5 μJ energy operating at 200 kHz repetition rate, with a spectrum spanning from 650 to 1150 nm (see spectrum in Fig. 1). The shaper comprises a pair of knife-edges and two folding mirrors in the Fourier plane of a folded double-pass 4f zero-dispersion line. This setup enables independent selection of two frequency components. The relative delay between the two pulses can be varied by placing one of the folding mirrors on a translation stage with a nominal resolution of 0.2 fs. In addition, one can adjust the energy ratio of the pulses by introducing an attenuator. The gray shaded areas in Fig. 1b, c show the exemplary selected output spectral regions for the experiments using the pulse shaper. The two collinear nJ pulses are then launched by a microscope objective (numerical aperture 0.40) into a 0.47 m long photonic crystal fiber (NKT Photonics, NL-PM-750) with two ZDWs at ~750 and 1250 nm, which we refer to as ZDW1 and ZDW2, respectively. Finally, the output of the fiber is analyzed by an optical spectrum analyzer (Ando AQ-6315A).

In the experiments, we launched a DW and a soliton at 680 and 800 nm, respectively. Equal separation from ZDW1 ensures nearly identical group velocities (≈2.02 × 108 m/s), with a slightly slower propagation of the DW. The input energy of the 800 nm pulse was adjusted to 1 nJ, which yields a soliton order of N = 25. Pulse energy of the DW is slightly lower (750 pJ). Pulse duration of the soliton and the DW are 200 fs and 1 ps, respectively.

Numerical simulations

Pulse propagation in the photonic crystal fiber is modeled in terms of the slowly varying envelope approximation. Therein, z-propagation of an initial complex envelope A(z = 0, t) is accomplished by numerical integration of the GNLSE:

$${\partial }_{{\rm{z}}}A(z,t)=i\left(\hat{\beta }-{\beta }_{0}-{\beta }_{1}{\partial }_{t}\right)A(z,t) +i\gamma \left(1+\frac{i}{{\omega }_{0}}{\partial }_{t}\right)A(z,t)\mathop{\int}\nolimits_{-\infty }^{\infty }h(\zeta )| A(z,t-\zeta ){| }^{2}\,{\rm{d}}\zeta ,$$

including the effects of dispersion, self-phase modulation, self-steepening and the Raman effect. The above GNLSE resolves the pulse dynamics in a retarded frame of reference moving with group velocity \({v}_{0}={\beta }_{1}^{-1}\). Linear dispersion obeys \(({\hat{\beta }}-{\beta }_{0}-{\beta }_{1}{\partial }_{t}){e}^{-i\omega t}=\tilde{\beta }(\omega ){e}^{-i\omega t}\), in the Fourier-domain specified by the polynomial approximation \(\tilde{\beta }(\omega )\equiv \mathop{\sum }\nolimits_{m = 2}^{11}\frac{{\beta }_{m}}{m!}{(\omega +{\omega }_{S}-{\omega }_{e})}^{m}\) with expansion coefficients β2 = −0.38 ps2 km−1, β3 = 4.97 × 10−3 ps3 km−1, β4 = 2.68 × 10−4 ps4 km−1, β5 =−1.25 × 10−6 ps5 km−1, β6 = 7.7 × 10−9 ps6 km−1, β7 = −5.83 × 10−11 ps7 km−1, β8 = 3.0 × 10−13 ps8 km−1, β9 = −3.74 × 10−16 ps9 km−1, β10 = −7.45 × 10−18 ps10 km−1, and, β11 = 6.96 × 10−20 ps11 km−1, determined for ωe = 1.85 rad fs−1. Here, ω specifies a frequency detuning with respect to the reference angular frequency ωS = 2.325 rad fs−1 (λS = 2πc0/ωS = 810 nm). The assumed nonlinear coefficient is γ = 3.8 W−1 km−1. The group-velocity-dispersion profile \({\beta }_{2}(\omega )={\partial }_{\omega }^{2}\beta (\omega )\) exhibits two roots ωZDW1 = 1.505 rad fs−1 (λZDW1 = 1252 nm) and ωZDW2 = 2.535 rad fs−1 (λZDW2 = 743 nm) with anomalous dispersion for ωZDW1 < ω < ωZDW2.

The total response function in the nonlinear part of the GNLSE includes the instantaneous Kerr and delayed Raman response in the form h(t) = (1 − fR) δ(t) + fR hR(t), with fR = 0.18 and a Raman-response function:

$${h}_{{\rm{R}}}(t)=\frac{{\tau }_{1}^{2}+{\tau }_{2}^{2}}{{\tau }_{1}{\tau }_{2}^{2}}{e}^{-t/{\tau }_{2}}\sin (t/{\tau }_{1})\ \theta (t),$$
(1)

with unit step function θ(t) and parameter values τ1 = 12.2 fs, τ2 = 32 fs. We verified that our findings are reproduced by considering other commonly used Raman-response functions.

For our numerical simulations we consider initial conditions of the form:

$$A(z=0,t)= {A}_{{\rm{S}}}\ {\rm{sech}}\left(\frac{t}{{t}_{{\rm{S}}}}\right)+{A}_{{\rm{DW}}}\ {\rm{sech}}\left(\frac{t+{t}_{0}}{{t}_{{\rm{DW}}}}\right) {e}^{-i({\omega }_{{\rm{S}}}-{\omega }_{{\rm{DW}}})t}.$$

Therein, AS, ωS, and tS are the amplitude, center frequency and duration of the initial soliton pulse. The initial amplitude is defined by the relation \(\gamma {A}_{{\rm{S}}}^{2}={N}_{{\rm{S}}}^{2}| {\beta }_{2}(\omega =0)| /{t}_{{\rm{S}}}^{2}\), entering the soliton order NS. Above, ADW, ωDW, and tDW specify the amplitude, center frequency and duration of the DW, injected with a temporal offset t0 relative to the soliton pulse. The numerical simulations reported in Fig. 2c, d are obtained using the GNLSE with additional quantum noise perturbing the initial condition, i.e., A(z = 0, t) → A(z = 0, t) + ΔA(t). The complex-valued random noise amplitudes ΔA(t) are obtained by spectral synthesis and satisfy the time-domain autocorrelation function 〈ΔA*(t)ΔA(t + τ)〉 = ℏωSδ(t)/2, where ℏ denotes the reduced Planck constant. We verified that the general features of the switching behavior reported in Fig. 1c, d are supported for a wide range of soliton orders NS = 10–30 and amplitude ratios ADW/AS < 1. The particular numerical results reported in Figs. 1 and 2 are obtained for NS = 17 and ADW/AS ≤ 1. The time delay in Fig. 2c, d is shown at increments of 25 fs. For the numerical solution of the GNLSE we use a standard split-step pseudospectral scheme.

The XFROG spectrograms in Fig. 3 are calculated as:

$${I}_{{\rm{XFROG}}}(z,\tau ,\omega )=\frac{1}{2\pi }{\left|\mathop{\int}\nolimits_{-\infty }^{\infty }A(z,t)h(t-\tau ){e}^{-i\omega t}{\rm{d}}t\right|}^{2},$$
(2)

where the field is localized in time using a Gaussian window function \(h(x)=\exp (-{x}^{2}/2{\sigma }^{2})\), centered at delay time t = τ and having root-mean-square width σ = 50 fs.