Abstract
Interpreting volcanic unrest is a highly challenging and non-unique problem at calderas, since large hydrothermal systems may either hide or amplify the dynamics of buried magma(s). Here we use the exceptional ground displacement and geochemical datasets from the actively degassing Campi Flegrei caldera (Southern Italy) to show that ambiguities disappear when the thermal evolution of the deep hydrothermal system is accurately tracked. By using temperatures from the CO2-CH4 exchange of 13C and thermodynamic analysis of gas ascending in the crust, we demonstrate that after the last 1982–84 crisis the deep hydrothermal system evolved through supercritical conditions under the continuous isenthalpic inflow of hot CO2-rich gases released from the deep (~8 km) magma reservoir of regional size. This resulted in the drying of the base of the hot hydrothermal system, no more buffered along the liquid-vapour equilibrium, and excludes any shallow arrival of new magma, whose abundant steam degassing due to decompression would have restored liquid-vapour equilibrium. The consequent CO2-infiltration and progressive heating of the surrounding deforming rock volume cause the build-up of pore pressure in aquifers, and generate the striking temporal symmetry that characterizes the ongoing uplift and the post-1984 subsidence, both originated by the same but reversed deformation mechanism.
Similar content being viewed by others
Introduction
Caldera unrest is a complex phenomenon consisting in a departure from the background or baseline behaviour of geophysical and geochemical indicators, such as changes in ground level, seismicity, gravity and degassing (total discharge, CO2 emissions and changes in gas chemistry) due to magma and/or hydrothermal system dynamics1,2,3. However, large uncertainties in the knowledge of the links between hydrothermal and magmatic processes affect the forecasting of the unrest evolution. Such uncertainties in determining the source and likely outcome of the unrest are even larger for calderas than for other volcanoes, essentially because of the stronger influence of hydrothermal systems at calderas2,3,4. Ground uplift, a typical marker of caldera unrest, may reflect magma movement and pressurization as well as pore pressure increase due to the changes in fluid phase and hydraulic flow patterns5,6,7,8,9,10,11. Variations in gas discharge are commonly perceived to represent a delayed response to magmatic changes, being mainly controlled by the hydrothermal system and rock permeability8,9,10,11,12. Furthermore, interpretations of ground deformation and geochemical data are too model-dependent and very often contrasting, such as in the case of determining the nature, size and depth of the overpressure source causing deformation13,14.
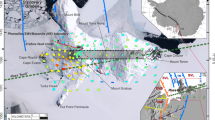
Ambiguities and difficulties in understanding the role of magmatic and hydrothermal triggering processes are well illustrated by the non eruptive unrest occurring at Campi Flegrei caldera (CFc) for decades (Fig. 1). CFc is a large (~12 km in diameter), restless caldera surrounding the Neapolitan area (Southern Italy), exposed to the highest volcanic risk in the world given the intense urbanisation15,16,17. Its evolution in historical times is marked by the Monte Nuovo eruption18, the last one, occurred in 1538 after a repose period of circa 2500 years, and by the continuous alternation of ground uplift and subsidence phases15,16,19,20. A vigorous hydrothermal system, fed by magmatic gases, drives the diffuse degassing of huge amounts of CO2, fumarole activity and mud pools14,15,21,22,23,24.
CFc setting and long-term ground displacement. (a) Location and structural sketch of Campi Flegrei caldera. Also reported are locations of (2) present Solfatara main fumaroles, Bocca Grande (BG), Bocca Nuova (BN), plus Forum Vulcani, which collapsed during the 1982–84 unrest, and (2) the Pisciarelli fumarole (b). Chronogram of the maximum vertical ground displacement15,19. Sharp uplifts characterized unrest episodes occurred in 1950–52, 1969–72 and 1982–84. A 20 year-long subsidence took place at the end of the 1982–84 crisis. A new unrest phase started around year 2005, characterized by a lower uplift rate than during the previous uplift episodes.
Here, we discriminate uplift episodes driven by magma intrusion at shallow depth from those driven by deep fluid influx in the aquifers, taking advantage of the exceptional, more than 35-years long, CFc geochemical record of CO2-rich gases discharged from fumaroles located within the Solfatara crater (Forum Vulcani, Bocca Grande, and Bocca Nuova vents) and the Pisciarelli area15,21,22,23,24 (Fig. 1a). Our approach is based on the reconstruction of the thermal evolution and phase changes of the deep hydrothermal system, independently recorded by carbon isotopic fractionation, and on thermodynamic analysis of CO2-rich ascending hydrothermal fluids. Combined with a model-independent description of ground-deformation patterns, and contrary to other recent model outcomes25, this analysis unravels the non-magmatic nature of the ongoing CFc unrest, due to supercritical excursion of the deep hydrothermal system. Our model-independent approach can also be applied widely to determine the source of unrest and its temporal evolution at other calderas.
Results
Non-eruptive unrest at the Campi Flegrei caldera
The presently ongoing CFc uplift episode occurs after ~20 years of subsidence that followed the 1984 peak ground uplift, when a maximum of ~4.5 m cumulative vertical displacement occurred over three major unrest episodes since 1950 (Fig. 1b). For this reason it is raising serious concern, such that since 2012 Civil Protection issues a volcanic alert of level 2 (‘Attention’) on a scale of four. Although the maximum uplift attained in 1984 was recognized to led the system very close to the purely fragile behaviour, the subsequent subsidence (Fig. 1b) clearly represents an anomalous behaviour with respect to other volcanoes, such as Rabaul caldera26, drawing back the system away from the critical rupture point26. It is then of paramount importance to establish the actual nature of the ongoing uplift, as compared to the 1982–1984 episod, in order to assess its hazard implications.
During the 1982–84 episode, H2O/CO2 increased rapidly up to ~7 in 1983 and then abruptly decreased to ~4 at the apex of ground deformation in 1984; it then attained a new maximum of ~7 in year 2000, to then decrease smoothly down to ~3 in the present unrest (Fig. 2a). This contrasting geochemical behavior requires triggering mechanisms that are different for the 1982–84 and the ongoing unrest episodes, and highlights that if unrest were magmatic in 1982–84, it must be hydrothermal now15,27. It was then proposed that decreasing H2O/CO2 ratio in post-1984 fumarole gases reflects the progressive arrival of hot gas, with H2O/CO2 between 1 and 1.5, released from the deep (8 km) magma and percolating through the shallow and permeable 1982–84 magma, which around 2000 exhausted its gas and solidified15,23. In an alternative model, gas signatures would be compatible with a magma progressively decompressing since 1984 and releasing gases with increasing H2O/CO2 ratio24,25,27. The subsequent condensation of large amounts of steam within the hydrothermal system would be invoked, in this hypothesis, to explain why a concomitant decrease of the H2O/CO2 ratio is observed in Solfatara and Pisciarelli fumarole fluids (Fig. 2a). Such a process would progressively heat up the hydrothermal reservoir rocks and increase pore pressure since 1984, thus triggering an accelerating deformation that could culminate in rock failure and eruption on attainment of a critical decompression threshold of the degassing magma26. Despite the different geochemical signatures and interpretations, we observe here for the first time that a striking temporal symmetry characterizes the evolution of ground displacement after the 1984 apex: two mirror exponential functions create a symmetric pattern of ground displacement whose minimum (0.86 m) is located in mid-2003 and is mainly due to a permanent deformation (0.77 m) residual from the 1982–84 unrest (Fig. 2b). This permanent deformation is very likely due to magma emplaced in 1982–84, still molten or eventually solidified23,28, but it may also represent a residual deformation of aquifers under drained conditions29. Subsidence recovered ~1 m of the maximum 1982–84 uplift (1.8 m) and led the system towards the 2003 bottom value. In the period 2000–2005 subsidence stopped, and a new uplift period started. Both subsidence and ongoing uplift have a symmetric baseline that can be described by means of a simple exponential law, having the same argument, changed of sign, and opportunely shifted in time to account for relative contributions of deflation and inflation in reproducing the main trend of vertical displacement (Fig. 2b). The fact that the involved rock volume experiences deflation and then inflation following mirror mechanisms is a powerful constraint to detect the nature of unrest, and is a further observation excluding any arrival of new magma after 1984. It also suggests that the deforming rock volume preserves the same mechanical properties both in the subsidence and in the ongoing uplift phases (Fig. 2b).
Major geochemical and ground deformation features since 1982. (a) Chronogram of H2O/CO2 (molar ratio) at Solfatara (Forum Vulcani, Bocca Grande and Bocca Nuova vents) and Pisciarelli fumaroles (Supplementary Data and refs15,22,23,24,25). (b) Baseline displacement (red-solid line) involving the post-1984 subsidence and the ongoing unrest (the zero is set at the beginning of the 1982-84 uplift). Error bars on measured displacements are ±4 mm for leveling (until year 2000; ref.19) and ±3 mm for continuous GPS (after year 2000; from INGV-OV surveillance bulletins: http://www.ov.ingv.it/ov/en/campi-flegrei/275.html). Three deformation regions are distinguished (R1, R2 and R3). (c) Chronogram of CO2-CH4 isotopic temperatures at the hydrothermal bottom (see Methods and Table 1). Heating trends (grey arrows) show the drying of the hydrothermal system.
Thermal features and evolution of the hydrothermal system
The Solfatara-Pisciarelli deep hydrothermal system was earlier interpreted as a liquid + vapor system located close to the critical point of water, at Pfluid ≈ 25 MPa, or PH2O ≈ 20 MPa, and ~360 °C (ref.22). It is fed by hot magmatic gases (~900 °C) that vaporize and mix with the pure hydrothermal (meteoric) component22. This interaction produces the final CH4-bearing fluid plume which formation temperature is recorded by the CO2 and CH4 isotopic exchange21,23,30,31 (Fig. 2c and Table 1; see Methods). However, by considering such isotopic temperatures we observe a progressive heating of the deepest part of the hydrothermal system, above the critical point of water. The only data available before 2002 (365 °C, measured in Forum Vulcani fluids in 1983) allow tying the entire thermal evolution to an initial condition corresponding to the 1982–84 unrest21, when CH4 clearly formed in a boiling aquifer very close to the critical point of water. Because fluids discharged at Solfatara and Pisciarelli point to the same deep physico-chemically homogenous hydrothermal system21,22, this initial condition is common to all fumarole systems in these areas.
The subsequent evolution up to 476 °C (Table 1) is due to the progressive drying of the boiling low-saline liquid water at the bottom of the hydrothermal system after 1984 and since around year 2003 at Pisciarelli. The observed deep heating is a strong argument against the presence of a shallow magma injecting increasing amounts of steam that subsequently condense (see Supplementary Information). In fact, steam condensation should keep the deep portion of the hydrothermal system thermally buffered along the liquid-vapor coexistence originally close to the critical point of water22. On the contrary, the dried hydrothermal low-saline liquid has been replaced by a high-T vapor or even supercritical gas (see Supplementary Information). This easily explains the decreasing H2O/CO2 ratio in fumarole gases and the relative increase of the gas magmatic fraction (>0.4) (refs22,23), as well as the high gas fluxes at surface14,24.
Pressure-temperature-enthalpy (Pfluid-T-H) paths (Fig. 3; see Methods) encountered in the subsoil of the fumarole system and of the area of maximum deformation from 1984 to present, shows that the hydrothermal system bottom is presently too hot to supply a gas condensing on its rise toward surface along the fumarole channel, which at first approximation is isenthalpic (Fig. 3a,b), corresponding to an irreversible adiabatic decompression (IAD) (see Methods). In Fig. 3a we plot P-H properties of pure water and a (molar) 80:20 H2O-CO2 real mixture (critical point at 52.5 MPa and 326 °C; Hvapor = 36.7 kJ/mol; ref.32), which is a good average of the last 10-year discharges (Fig. 2a). If we take as reference pure water (in green in Fig. 3a), upon IAD, condensation cannot occur for H > 50.5 kJ/mol, thus the present-day deep hydrothermal gas (~450 °C) would condense only if its initial (water) pressure rises from ~20 MPa (ref.22) to ~30 MPa. If we consider, the 80:20 H2O-CO2 real mixture (in blue in Fig. 3a), upon IAD, condensation cannot occur for H > 48.3 kJ/mol, such that the present-day deep hydrothermal gas at 450 °C would not condense during isenthalpic expansion (unless starting IAD from pressure unreasonably high for the CFc system, i.e. >50 MPa; Fig. 3a).
P-H-T properties of the hydrothermal system. (a) Pfluid-H diagrams for pure water (thin green lines: isotherms; thick green line: vapour-liquid equilibrium) and for a H2O-CO2 80:20 (mol) mixture (thin blues lines: isotherms; thick blue line: vapor-liquid equilibrium-dew line). Green and blue labels refer to pure water and H2O-CO2 mixture, respectively. Numbers labeling isotherms refer to °C. See Methods for source of data. (b) Vapor-liquid equilibria and phase fields for pure water (green line and green labels) and for the 80:20 H2O-CO2 mixture (blue line and blue labels), together with the critical line for intermediate fluid compositions (turquoise line). L: Liquid; L + V: coexisting liquid and vapor; SC: supercritical gas. Also shown (with respect to pure water only, for the sake of graphical clarity) are: 1) the P-T path of the 1982-84 fluid plume (solid red line), 2) the locus of condensation-free ascent fluid paths between 2001 and 2013 (enveloped by the dotted closed red line), and 3) the P-T paths outside the hot fumarolic area (solid and dotted black straight lines). See Methods for sources of data.
With reference to pure water, the ABCDEFG quadrangle (Fig. 3a) then surrounds the domain of bottom hydrothermal conditions for enthalpies corresponding to temperatures increasing from 360 °C to 450 °C, as required by the CO2-CH4 13C exchange (Fig. 2c and Table 1) and pressures of interest (PH2O from ~20 to 30 MPa). Assuming at first approximation the validity of the Raoult’s law, the pressure excursion at the base of the hydrothermal system would become ~25 MPa to ~40 MPa for the 80:20 H2O-CO2 fluid, thus defining, for the same T-interval, the B’C’D’E’F’G’ quadrangle (Fig. 3a). It is worth noting that in both cases, the pressure increase (10 MPa for pure H2O and 15 Mpa H2O-CO2 mixture) does not exceed the threshold imposed by CFc rock tensile strength13.
The pure-H2O ABCDEFG quadrangle (Fig. 3a) embraces liquid, vapour and supercritical phases, and shows that the deep hydrothermal system has evolved as a supercritical fluid around the critical point of pure water. With reference to the 80:20 H2O-CO2 mixture, we see that the deep hydrothermal gas (B’C’D’E’F’G’ quadrangle; Fig. 3a) would be directly placed in the superheated vapour region. Therefore, accounting for the H2O-CO2 mixture, rather than pure water, expands the range of conditions for which liquid condensation cannot be encountered. This allows us stating that gas emissions associated with the ongoing unrest, and more generally with the post-1984 subsidence (Fig. 2a), result from a single-gas expansion, from the base of the hydrothermal system upward. On the other hand, the 1982-84 gas has evolved by condensing either water, or saturated H2O-CO2 vapour, as shown by independent P-T estimates of the last equilibrium recorded by 1982–84 fumarolic gases (Supplementary Figure 1), which are compatible with either the point of maximum enthalpy of the saturated steam (236 °C, 3.1 MPa) or the saturated H2O-CO2 vapor (~250 °C and ~5 MPa).
These features can be appreciated in Fig. 3b, which reports the P-T curves for pure water and for the 80:20 H2O-CO2 mixture, the critical line for intermediate H2O-CO2 mixtures, as well as the P-T region (with reference to steam) within which Solfatara fluids evolve from PH2O ≈ 20 MPa and temperatures between 400 °C to 450 °C (CD line in Fig. 3b) up to surface fumaroles. Differently from the 1982–84 fluid plume, which condensed up to the point of maximum enthalpy of the saturated steam (solid red line), fumarole fluids discharged between 2001 and 2013 (enclosed within the dotted red line, Fig. 3b) do not condense and can reach, by IAD (isenthalpically), pressures up to PH2O ≈ 0.3 MPa and temperatures between 210 °C and 300 °C. They then reach fumarole outlets at ~160 °C (BG) and ~145 °C (BN, as well as Forum Volcani, collapsed in 1984) (gray box along the X-axis in Fig. 3b), vent temperatures being fixed by the gas flow energy conservation throughout the terminal section of fumarolic conduits33.
In agreement with geothermal gradients measured at CFc drilling sites34, Fig. 3b also shows the evolution of the P-T paths originating from initial bottom temperatures of 360 °C (solid black line representing the 1982–84 path) and 450 °C (dashed black line, representing attainment of steady state conditions). These are external to the fumarole field but internal to the area of maximum deformation and intersect the sequence of aquifers that saturate the rock medium accommodating the whole deformation on top of the deep hydrothermal system. The Solfatara and Pisciarelli fumarole fields are located along structural discontinuities (fault planes) that probe the physico-chemical evolution of the hydrothermal reservoir, spilling directly its deepest portion. Solfatara and Pisciarelli fumaroles are located along the same axis at 1.8 and 2.3 km, respectively, north-east from Rione Terra (Pozzuoli downtown), where maximum vertical displacement is observed. Fumaroles are a window on the deep hydrothermal system, but horizontally offset from the area of the maximum deformation that is modulated by the Pfluid-T evolution of aquifers on top of the drying hydrothermal system. Considering a uniform bottom heating up from 360 °C to 450 °C, the P-T path crossing aquifers should progressively vary until reaching a new steady state (dashed black line, Fig. 3b), still enclosed within the liquid region. Despite the temperature of the hydrothermal bottom zone increased of ~90 °C in around 30 years (Figs 2c and 3b), thermal diffusivity analysis (κ = k/ρCp; with k = 2.8 W/m·K and Cp = 1000 J/kg·K, ρ = 2000 kg/m3) (refs35,36) shows that the new steady state geothermal gradient through aquifers has not been reached yet, and that heat conduction alone may have propagated the thermal anomaly to a distance \(\lambda \approx 2\sqrt{\kappa \tau }\) of 73 m above the ~2.5 km-deep base of the hydrothermal system (τ = 30 years). On the other hand, the efficient advective transport of heat in fumaroles produced, since 2007, a T-increase of 15 °C in the very shallow (<0.5 km-deep) single gas equilibrium zone15 (Supplementary Figure 1). Therefore, outside the hot fumarole area, in the water-saturated porous medium hosting the main aquifers of the deformed area, where heat transport is neither purely advective nor conductive, we should expect at present an average thermal increase of few degrees (<5 °C). This small increase is determined by the permeability-driven slow infiltration of hot CO2 and its dissolution in groundwaters and is consistent with the comparison of results at different time-steps37,38 from numerical simulations of the CFc thermal field, which is dominated by the advective flux of CO2-rich hot fluids.
It is worth noting that based on 2D simulations of the only conductive and convective heat transfer resulting from the sudden sill emplacement at 4 km depth, we can, in fact, rule out any appreciable effect related to the long-lasting conductive thermal release of the 1982–84 sill intrusion on the surrounding rocks and the base of the hydrothermal system (see Methods and Fig. S2). In addition, simulations returns rapid cooling below solidus, in the order of ~10 years, confirming previous findings15,23,28 and further marking the impossibility that a shallow magma emplaced in 1982–84 may supply fluids until nowadays and even further (see Supplementary information). Therefore, the thin (<0.8 m; Fig. 2b) 1982–84 intrusion cannot have determined the progressive T-increase over time, as inferred from geochemical data. The very rapid cooling and the lack of a a long-lasting thermal effect associated with the thin-sill intrusion requires that a different heating source, associated with the rise of CO2-rich deep fluids, has 1) dried over time the base of the hydrothermal system and 2) determined, in the shallow aquifers, thermoelastic effects that are reponsible of the ongoing ground displacement (Fig. 2b).
Discussions and Conclusions
The 1982–84 unrest was determined by the shallow intrusion of a sill-like magmatic body, that suddenly injected hot steam-rich fluids into the hydrothermal reservoir23. Steam condensation determined the shallow hydrothermal heating (up to 240 °C; Supplementary Figure 1 and ref.15) and contributed to the rapid 1982–84 uplift via undrained expansion29,39 (R1 in Fig. 2b). The intrusion thickness shoud approximate quite well the permanent deformation of ~0.8 m (Fig. 2b), but we cannot exclude that a residual thermoelastic deformation of aquifers29 contributes to the latter.
The 20-year long subsidence of ~1 m after the 1984 peak (R2 in Fig. 2b) can only be explained via relaxation of the hydrothermal system and aquifers, which soon restored drained conditions29,39 by rapidly releasing the excess hot fluids and progressively dissipating pore pressures. Solfatara fumaroles record this process by the decrease of shallow hydrothermal temperature and the rapid drop of pressures, already started in 1984 (Supplementary Figure 1).
Around year 2000, upon solidification and fracturing of the shallow sill emplaced in 1982, continuous percolation of the deep (8 km) magmatic gases replaced the previously pulsating behaviour, as shown by the H2O/CO2 ratio (Fig. 2a). This process was accompanied by the increase in seismicity observed since year 2000, also showing 3-4 km deep hypocenters (see Methods and ref.40) that can be related to the ongoing fracturing within the solidified magma sill. The observed drying of the deep hydrothermal component was then caused by the heat supplied by the CO2-rich gas, on its ascent from the deep degassing magma (200 MPa and 1000 °C) (refs23,41,42,43) to the bottom of the hydrothermal system at 25 MPa (see Methods and Supplementary Figure 2). Around year 2003, the deep hydrothermal drying became effective at Pisciarelli too, and likely involved most of the aquifers of the deformed area. Then a new uplift phase became evident, reversing the previous scenario (R3 in Fig. 2b). The ongoing uplift should be then ultimately caused by infiltration of deep CO2-rich hot gases into the hydrothermal system and aquifers with subsequent temperature increase and pressure relaxation in response to the upward propagation of the deep thermal anomaly (Fig. 2c). Consistent with this, fumarole gases display an increasing equilibrium temperature (since 2007) and a constant equilibrium pressure, close to the atmospheric one since 2005 (Supplementary Figure 1 and ref.15), attained along nearly-isenthalpic ascent paths, sketched by the violet arrow in Fig. 3a and enveloped by the red dotted area in Fig. 3b.
In a porous medium expansion of the water + gas filled rock volume occurs in response to changes of temperatures within the reservoir and involves a volume in the range 15–35 km3 (considering the present uplift of 40 cm; Fig. 2b) (see Methods). We do not model here the time dependence of ground deformation (Fig. 2b), which for hydrothermal unrest is well-established even for fixed, constant source parameters8,9,10,11. However, our analysis points out that the ongoing unrest is restoring, partially or totally, the same conditions that in 1984 led the system very close to the critical fragile behavior, which is the critical point when an eruption becomes very likely25,41,42. Therefore, despite the ongoing ground deformation not being linked so far to magma migration to shallow depths, the unrest could switch to a phase of magma ascent43,44,45: our findings in fact suggest that a chemical window is opening over the deep magmatic system, and that direct connections with it may be activated, depending on the accumulated stress and the attainment of the fragile threshold for rock fracture26. We speculate here that the onset of such connections may explain why the CFc magma reservoir could be activated at any time44, leading to explosive behaviour on a short timescale of hours or days46,47.
Given the present poor knowledge of subsoil mechanical, hydraulic and thermal features in the Solfatara-Pisciarelli area, our study also highlights the need for detailed subsurface information that could only be obtained via deep scientific drilling16,17 and extensive high-resolution 3D geophysical surveys48,49. These would allow direct monitoring of thermal anomalies due to deep hot sources and complement geochemical information from fumaroles. Fumaroles are in fact useful “hot control points” that locally spill quasi-isenthalpically the deep hydrothermal system, but whose variations cannot be entirely transposed into the lower-T and water-saturated deforming rock volume.
Methods
Thermodynamic analysis of hydrothermal and magmatic fluids
Our main goal is the reconstruction of the temporal evolution of the thermodynamic state of the deep hydrothermal system, particularly its temperature and phase constitution. Its thermal history is determined independently of the geochemical composition of fumarole discharges and based on the C-isotopic exchange between CO2 and CH4 species. The ensuing thermodynamic analysis shows that deep hydrothermal fluids experience after 1984 a supercritical excursion (with respect to pure water) or evolution to superheated vapour (with respect to a real H2O-CO2 80:20 molar mixture), such that the liquid phase disappeared (see also Supplementary information). The locus of P-T-H paths connecting the deep dried hydrothermal system and fumarole vent conditions, through the shallow hydrothermal system, shows that the ascending fluid plume cannot experience steam condensation and follows nearly isenthalpic rising paths. This demonstrates that the increasing CO2-fraction observed in fumaroles is determined by the increased percolation and inflow of CO2-rich magmatic fluids released by the 8 km deep magma reservoir. Isenthalpic rise of such CO2-rich deep magmatic fluids represents the effective heat source responsible of the observed drying of the deep hydrothermal system. The shallow P-T equilibrium conditions of the hydrothermal fluid have been estimated by geothermobarometric functions within the CO2-H2O-CO-CH4-H2 system and show that single gas-phase equilibrium conditions take place after 1984 (Supplementary Figure 1). It was already demonstrated15 that CH4 participates to the full chemical equilibrium at temperatures lower than those recorded by the isotopic exchange between CO2 and CH4 (Fig. 2c). For CH4, rates of chemical and isotopic exchanges are in fact different, such that isotopic equilibration is ~400 times slower than the chemical one50 and cannot be recorded by fumarolic gases.
Thermodynamic properties of pure water and ideal H2O-CO2 mixtures (Fig. 3a,b and Supplementary Figure 2) have been computed by using the web-calculator provided by the National Institute of Standards and Technologies, available at: http://webbook.nist.gov/chemistry/fluid © 2016 by the U.S. Secretary of Commerce on behalf of the United States of America.). Vapor-liquid P-H-T conditions for the real H2O-CO2 80:20 (molar) mixture in Fig. 3a,b are from ref.32.
Computation of isotopic temperatures of the deep hydrothermal system
Because isotopic equilibration is ~400 times slower than the chemical one50,51, 13C exchange between CO2-CH4 quenches the thermal condition of CH4 formation, hence the thermal condition of the generation of deep hydrothermal system upon interaction with magmatic gases22. Based on available measurements of 13C exchange between CO2 and CH4 (Table 1), isotopic temperatures have been computed by using the following thermometer52 between 473.15 K and 873.15 K (or 400 °C and 600 °C):
Standard deviation is 0.14‰ on 103lnα, where α is defined as:
Computation of equilibrium temperatures and pressures of the upper hydrothermal system
In order to compute the temperature of the equilibration zone of gas species within the hydrothermal system (upper hydrothermal reservoir) (Supplementary Figure 1) we adopted the water-gas shift equilibrium15,53,54:
whose equilibrium constant (1 bar, T of interest standard state) provides a pressure- and redox-independent estimate of equilibrium temperature:
Terms in square brackets denote thermodynamic activities, which can be replaced by concentrations. Note that equilibrium temperatures, recorded by gas species of the single vapor phase, hold valid also for the liquid phase eventually coexisting with vapor15.
From the following reaction15,54
The hydrothermal pressure, P (Supplementary Figure 1), is obtained by reworking on the expression for the equilibrium constant of reaction 5:
Partial pressures (i.e., PH2O and PCO2) can be then computed on the basis of Raoult’s law.
Plotting T vs PH2O (Supplementary Figure 1) allows a straight evaluation of the rare attainment of liquid-vapour coexistence, with 1983 Forum Vulcani points falling close to the point of maximum enthalpy of either the pure saturated steam (236 °C, 3.1 MPa) or the saturated 80:20 H2O-CO2 vapor (~250 °C and ~5 MPa).
Isenthalpic paths of deep gas ascent
Isenthalpic deep gas ascent, or irreversible adiabatic decompression (IAD), obeys the following thermodynamic relation55:
in which μ JT is the Joule-Thomson coefficient), V the partial molar volume, α the therrmal expansivity, and C p the constant pressure heat capacity. Supplementary Figure 2 shows IAD paths for pure H2O, pure CO2 and a H2O/CO2 = 1 mixture rising from the deep magmatic source into the deep hydrothermal system (~25 MPa). These paths are compared to that followed by a steam-rich (H2O/CO2 = 2.5) magmatic gas released from the shallow decompressing magma hypothesized in ref.24 (1000 °C and 120 MPa at the end of the 1984–2004 subsidence phase). At the entrance of the hydrothermal system, all paths end at a temperature compatible with that of magmatic gases (~900 °C; ref.22) inferred from independent energetic considerations. In particular, because of the Joule-Thomson effect, the pure CO2 gas records a temperature increase due to gas heating on ascent (1055 °C at 25 MPa), whereas the H2O-CO2 mixture keeps a high temperature (961 °C at the hydrothermal interface, or 25 MPa), that is 100 °C higher than that of pure H2O (863 °C) at the hydrothermal interface. The hypothetical steam-rich gas released at 120 MPa, under isenthalpic conditions, would enter the hydrothermal system at 935 °C. Therefore, the H2O-CO2 1:1 mixture percolating since year 2000, brings 43.5 J/g ( = ρC p ΔT, with ΔT temperature difference at 25 MPa between the two gas mixtures) more than the shallow steam-rich magmatic gas released by a shallow magma at 120 MPa. Such a difference makes the deep degassing of a CO2-rich supercritical gas energetically more advantageous than the release of steam-rich gas from a shallower magma, which in any case cannot support the degassing process for long-time and soon reaches exhaustion. It is clear that only the persistent continuous influx of the CO2-rich deep gas can sustain the observed heating and drying of the hydrothermal bottom, and that in the case of pure CO2 infiltration, heating effects would be magnified by the CO2 Joule-Thomson effect.
Modeling of conductive/convective heat transfer after sill emplacement
In order to evaluate the thermal effect of a thin-sill intrusion on the thermal profile of the magma surrounding rocks and of the hydrothermal system, we perfomed simulations of the conductive/convective heat transfer following emplacement by using the Heat3D code56. This solves heat flow by finite difference solution of energy and momentum conservation equations (i.e., Navier-Stokes). These equations describe heat transfer by conduction and convection with nonlinearities arising from variation of thermal conductivity in a non-isotropic, heterogeneous, material with heat sources/sinks (e.g., latency). The Boussinesq approximation is employed in the Navier-Stokes equations and momentum conservation by Darcy’s equation is employed because of its empirical success. HEAT3D employs an explicit finite differencing scheme, such that the original differential equation solved is exactly reproduced by the finite difference equation when time and spatial steps are infinitesimal. Truncation errors that might evolve when using very short time steps are minimized by utilizing double precision. Continuous thermal gradients are assigned along the boundaries where symmetry planes are not specified, and initial conditions use a designated regional thermal gradient. The heat advection caused by rock displacement necessary to make space for the magma was considered in our simulations. Used rock/magma properties are given in Supplementary Table 1.
We modelled a 800 × 200 element planar regular mesh (grid size = 25 m), corresponding to 20 km in length and 5 km in depth. For computational limits, it was not possible to decrease the grid size to values comparable with the expected thickness of the intruding sill,that is in the order of one meter (Fig. 2b). Convection was assumed to occur in a hydrothermal system extending over a distance of 4 km from the center of the calculation domain, thus 8 km in total. Two possible depths of the hydrothermal system were considered: 2.5 km (ref.22) and 4 km (i.e., along the base of the B’C’D’E’F’G’ quadrangle in Fig. 3a). From previous drillings made by AGIP34 we know anyway that the aquifer bottom, i.e. the depth at which the supercritical temperature for water, lies in the whole area in the depth range 2.5-3.0 km. The higher depth of 4 km then maximize the speed of propagation of the heat coming from the sill, because of the convective effects in the aquifer, which is here in contact with the sill.
As thermal boundary condition, we set a thermal gradient of 0.15 °C/m. After 4400 years (i.e., the time elapsed since the last eruption also originating the Solfatara-Pisciarelli system44), this condition returns a temperaure-depth profile compatible with geochemistry22, as well as borehole thermal gradients34.
Then, in our model, a sill, having a thickness corresponding to the grid size (i.e., 25 m), suddenly emplaces at 4 km depth. Supplementary Figure 2 shows that, over a time span of 100 years after emplacement, it produces negligible thermal perturbations in the surrounding hydrothermal system. Even extending the base of the hydrothermal system down to 4 km, i.e. right on top of the sill, does not change appreciably the initial temperature profile. In fact, only the very bottom layer records a sudden temperature increase upon sill-emplacement, from 520 °C to ~570 °C after 20 years, but then remains stable.
Finally, it is worth noting that in both simulations, magma solidus conditions are achieved very quickly (~10–20 year). All above results, all the more so, apply also to a sill thinner than the one modelled here.
Deformation modelling
The expansion of the water + gas filled rock volume (V r ) in response to changes of temperatures and gas content (n) within the reservoir is given by:
with V0,r the initial volume prior to expansion. Considering that the second term in equation (8) is relevant only within the limited portion of the fumarole area, and that injected gas (i.e., CO2) can be entirely considered dissolved in acquifers without appreciable pore volume gain (i.e., along black P-T paths in Fig. 3b), we can focus our attention on the first term in integral. This would produce a volumetric deformation (ε = ΔV r /V0,ρ) proportional to α rock = ϕα w + (1 − ϕ)α s with α w the coefficient of (volumetric) thermal expansion of water, α s the coefficient of (volumetric) thermal expansion of the solid matrix and ϕ the porosity. By using α w = 5·10−4 °C−1, α s = 0.5·10−4 °C−1 and ϕ = 0.2 (refs29 and 35), and for ΔT between 1 °C and 5 °C, ε ranges from 0.00014 to 0.0007. In order to translate such volumetric deformation of the hosting rocks (i.e. at the source) into surface deformation, we use here the established relationships between volume expansion at the source volume change (ΔV) and surface uplift volume (UV), which depend on the source model assumed56. For a sill-like source, the volume expansion at the source is equal to the uplift volume at surface, whereas for a point-source model57 ΔV = UV/2(1 − ν), where ν is the Poisson coefficient of the host rock. Since we are modelling a source involving a rather large volume of the heated porous medium, we can approximate the source model to a point-source model, because it is well known58 that the point source approximation works well also for non negligible r/d ratios (where r is the radius of a sphere undergoing volume or pressure changes, and d is the depth of its centre). Assuming a spherical source with center at 3.0 km of depth (d), a radius of 2 km (i.e. V = 33.5·109 m3), we can compute the volume increase, using both the values of ε computed for the limiting temperature changes of 1 °C and 5 °C, as ΔV = ε·V. We obtain a value ΔV = 47.3·105 m3 for ΔT = 1 °C, and ΔV = 236.6·105 m3 for ΔT = 5 °C. The maximum vertical surface displacement (i.e., just above the source) resulting from the volume change ΔV is hmax = ΔV(1 − ν)/(πd2) (ref.59). Using a value of ν = 0.25, the resulting maximum displacement ranges between 0.13 m (for ΔT = 1 °C) and 0.65 m (for ΔT = 5 °C), which compares well with an observed value of 0.40 m. In principle, the source could be centred at lower depth: as an example, a source centred at 2 km, with a radius of 1.5 km (i.e., V = 14.1·109 m3), would produce almost exactly the same values. The initial rock volume so far interested by deformation would then be on the order of ~15 to ~35 km3.
Data availability
Solfatara fumaroles data are fully available from refs15,22,23,24,25,53. Supplementary data file reports the full listing of composition of the Pisciarelli fumarole, sampled since 1999. For this fumarole, data until September 2009 can also be found in ref.53. In all cases, concentrations are expressed in μm/mol and analytical methods are reported in ref.22. Seismological data (magnitudo and hypocenters depth can be found on the Plinio seismic data repository (http://www.ov.ingv.it/ov/en/banche-dati.html) or from INGV-OV bulletins (http://www.ov.ingv.it/ov/en/campi-flegrei/275.html). All data, computations and time series are available on request to the corresponding author.
References
Newhall, C. G. & Dzurisin, D. Historical unrest at large calderas of the world. U.S. Geol. Surv. Bull 1855, 1108 pp. (1988).
Acocella, V., Di Lorenzo, R., Newhall, C. & Scandone, R. An overview of recent (1988 to 2014) caldera unrest: knowledge and perspectives. Reviews of Geophysics 53, https://doi.org/10.1002/2015RG000492 (2015).
Lowenstern, J. B. & Hurwitz, S. Monitoring a supervolcano in repose: Heat and volatile flux at the Yellowstone Caldera. Elements 4, 35–40 (2008).
Fournier, R. O. Geochemistry and dynamics of the Yellowstone National Park hydrothermal system. Annual Review of Earth and Planetary Sciences 17, 13–53 (1989).
Wicks, C. W., Thatcher, W., Dzurisin, D. & Svarc, J. Uplift, thermal unrest and magma intrusion at Yellowstone caldera. Nature 440(7080), 72–75 (2006).
Gregg, P. M., De Silva, S. L. & Grosfils, E. B. Thermomechanics of shallow magma chamber pressurization: Implications for the assessment of ground deformation data at active volcanoes. Earth and Planetary Science Letters 384, 100–108 (2013).
Battaglia, M., Troise, C., Obrizzo, F., Pingue, F. & De Natale, G. Evidence for fluid migration as the source of deformation at Campi Flegrei caldera (Italy). Geophys. Res. Lett. 33, L01307 (2006).
Hurwitz, S., Christiansen, L. B. & Hsieh, P. A. Hydrothermal fluid flow and deformation in large calderas: Inferences from numerical simulations. Journal of Geophysical Research: Solid Earth 112(B2), B02206 (2007).
Hutnak, M., Hurwitz, S., Ingebritsen, S. E. & Hsieh, P. A. Numerical models of caldera deformation: Effects of multiphase and multicomponent hydrothermal fluid flow. Journal of Geophysical Research: Solid Earth 114(B4), B04411 (2009).
Fournier, N. & Chardot, L. Understanding volcano hydrothermal unrest from geodetic observations: Insights from numerical modeling and application to White Island volcano, New Zealand. Journal of Geophysical Research 117, B11208, https://doi.org/10.1029/2012JB009469 (2012).
Todesco, M., Rinaldi, A. P. & Bonafede, M. Modeling of unrest signals in heterogeneous hydrothermal systems. J. Geophys. Res. 115, B09213, https://doi.org/10.1029/2010JB007474 (2013).
Tedesco, D., Pece, R. & Sabroux, J. C. No evidence of a new magmatic gas contribution to the Solfatara volcanic gases, during the Bradyseismic crisis at Campi Flegrei (Italy). Geophysical Research Letters 15, 1441–1444 (1988).
Gottsmann, J., Rymer, H. & Berrino, G. Unrest at the Campi Flegrei caldera (Italy): A critical evaluation of source parameters from geodetic data inversion. Journal of Volcanology and Geothermal Research 150, 132–145 (2006).
Aiuppa, A. et al. First observations of the fumarolic gas output from a restless caldera: Implications for the current period of unrest (2005–2013) at Campi Flegrei. Geochem. Geophys. Geosyst. 14, 4153–4169 (2013).
Moretti, R., De Natale, G. & Troise, C. A geochemical and geophysical reappraisal to the significance of the recent unrest at Campi Flegrei caldera (Southern Italy). Geophysics Geochemistry and Geosystems 18, 1244–1269 (2017).
De Natale, G., Troise, C., Kilburn, C. J., Somma, R. & Moretti, R. Understanding volcanic hazard at the most populated caldera in the world: Campi Flegrei, Southern Italy. Geophysics, Geochemistry and Geosystems 18, https://doi.org/10.1002/2017GC006972 (2017).
De Natale, G. et al. The Campi Flegrei Deep Drilling Project (CFDDP): New insight on caldera structure, evolution and hazard implications for the Naples area (Southern Italy). Geophysics, Geochemistry and Geosystems 18, 4836–4847 (2016).
Di Vito, M. A. et al. Magma transfer at Campi Flegrei caldera (Italy) before the 1538 AD eruption. Scientific Reports 6, 32245 (2016).
Del Gaudio, C., Aquino, I., Ricciardi, G. P., Ricco, C. & Scandone, R. Unrest episodes at Campi Flegrei: A reconstruction of vertical ground movements during 1905–2009. J. Volcanol. Geotherm. Res. 195, 48–56 (2010).
Morhange, C., Marriner, N., Laborel, J., Todesco, M. & Oberlin, C. Rapid sea-level movements and noneruptive crustal deformations in the Phlegrean Fields caldera, Italy. Geology 34, 93–96 (2006).
Bolognesi, L., Noto, P. & Nuti, S. Studio chimico ed isotopico della solfatara di Pozzuoli: ipotesi sull’origine e sulle temperature profonde dei fluidi. Rend. Soc. It. Mineral. Petrol. 41, 281–295 (1986).
Caliro, S. et al. The origin of the fumaroles of La Solfatara (Campi Flegrei, South Italy). Geochimica et Cosmochimica Acta 71, 3040–3055 (2007).
Moretti, R., Orsi, G., Civetta, L., Arienzo, I. & Papale, P. Multiple magma degassing sources at an explosive volcano. Earth and Planetary Science Letters 367, 95–104 (2013).
Chiodini, G. et al. Evidence of thermal-driven processes triggering the 2005–2014 unrest at Campi Flegrei caldera. Earth Planet. Sci. Lett. 414, 58–67 (2015).
Chiodini, G. et al. Magmas near the critical degassing pressure drive volcanic unrest towards a critical state. Nature Communications 7, 13712 (2016).
Kilburn, C. R., De Natale, G. & Carlino, S. Progressive approach to eruption at Campi Flegrei caldera in southern Italy. Nature Communications 8, 15312 (2017).
Caliro, S., Chiodini, G. & Paonita, A. Geochemical evidences of magma dynamics at Campi Flegrei (Italy). Geochimica et Cosmochimica Acta 132, 1–15 (2014).
Woo, J. Y. & Kilburn, C. R. Intrusion and deformation at Campi Flegrei, southern Italy: sills, dikes, and regional extension. Journal of Geophysical Research: Solid Earth 115 ( B12 ) (2010).
Aversa, S. & Evangelista, A. Thermal expansion of Neapolitan yellow tuff. Rock Mechanics and Rock Engineering 26, 281–306 (1993).
Fiebig, J., Tassi, F., D’Alessandro, W., Vaselli, O. & Woodland, A. B. Carbon-bearing gas geothermometers for volcanic-hydrothermal systems. Chemical Geology 351, 66–75 (2013).
Fiebig, J. et al. Isotopic patterns of hydrothermal hydrocarbons emitted from Mediterranean volcanoes. Chemical Geology 396, 152–163 (2015).
Gallagher, J. S., Crovetto, R. & Levelt-Sengers, J. M. H. The thermodynamic behavior of the CO2‐H2O system from 400 to 1000 K, up to 100 MPa and 30% mole fraction of CO2. Journal of Physical and Chemical Reference Data 22, 431–513 (1993).
Bird, R. B., Stewart, W. E. & Lightfoot, E. N. Fenomeni di trasporto, C.E.A. Casa Editrice Ambrosiana, Milano, 804 pp. (1979).
Di Renzo, V. et al. The thermal regime of the Campi Flegrei magmatic system reconstructed through 3D numerical simulations. Journal of Volcanology and Geothermal Research 328, 210–221 (2016).
Troiano, A., Di Giuseppe, M. G., Petrillo, Z., Troise, C. & De Natale, G. Ground deformation at calderas driven by fluid injection: modelling unrest episodes at Campi Flegrei (Italy). Geophys. J. Int 187, 833–847 (2011).
Capuano, P. et al. The active portion of the Campi Flegrei caldera structure imaged by 3‐D inversion of gravity data. Geochemistry Geophysics Geosystems 14, 4681–4697 (2013).
Todesco, M., Chiodini, G. & Macedonio, G. Monitoring and modelling hydrothermal £uid emission at La Solfatara (Phlegrean Fields, Italy). An interdisciplinary approach to the study of diffuse degassing. Journal of Volcanology and Geothermal Research 125, 57–79 (2003).
Todesco, M., Rutqvist, J., Chiodini, G., Pruess, K. & Oldenburg, C. M. Modeling of recent volcanic episodes at Phlegrean Fields (Italy): geochemical variations and ground deformation. Geothermics 33, 531–547 (2004).
Gaeta, F. S. et al. Genesis and evolution of unrest episodes at Campi Flegrei caldera: the role of thermal-fluid-dynamical processes in the geothermal system. J. Geophys. Res. 108(B9), 20921–20933 (1998).
D’Auria, L. et al. Repeated fluid‐transfer episodes as a mechanism for the recent dynamics of Campi Flegrei caldera (1989–2010). Journal of Geophysical Research: Solid Earth 116(B4), B04313 (2011).
Bakker, R., Violay, E. S., Benson, P. M. & Vinciguerra, S. V. Ductile flow in sub-volcanic carbonate basement as the main control for edifice stability: new experimental insights. Earth Planet Sci. Lett. 430, 553–541, https://doi.org/10.1016/j.epsl.2015.08.017 (2015).
Bakker, R., Fazio, M., Benson, P. M., Hess, K.-U. & Dingwell, D. B. The propagation and seismicity of dyke injection, new experimental evidence. Geophys. Res. Lett. 43, 1876–1883, https://doi.org/10.1002/2015GL066852 (2016).
Cannatelli, C. Understanding magma evolution at Campi Flegrei (Campania, Italy) volcanic complex using melt inclusions and phase equilibria. Mineralogy and Petrology 104, 29–42 (2012).
Arienzo, I., Moretti, R., Civetta, L., Orsi, G. & Papale, P. The feeding system of Agnano–Monte Spina eruption (Campi Flegrei, Italy): dragging the past into present activity and future scenarios. Chemical Geology 270, 135–147 (2010).
Moretti, R., Arienzo, I., Civetta, L., Orsi, G. & D’Antonio, M. The deep plumbing system of the Ischia island: a physico-chemical window on the fluid-saturated and CO2-sustained Neapolitan volcanism (Southern Italy). Journal of Petrology 54, 951–984 (2013).
Pappalardo, L. & Mastrolorenzo, G. Rapid differentiation in a sill-like magma reservoir: a case study from the Campi Flegrei caldera. Scientific Reports 2, 712 (2012).
Perugini, D., Poli, G., Petrelli, M., De Campos, C. P. & Dingwell, D. B. Time-scales of recent Phlegrean Fields eruptions inferred from the application of a ‘diffusive fractionation’ model of trace elements. Bulletin of Volcanology 72, 431–447 (2010).
Gresse et al. Three-Dimensional Electrical Resistivity Tomography of the Solfatara Crater (Italy): Implication for the Multiphase Flow Structure of the Shallow Hydrothermal System. Journal of Geophysical Research: Solid Earth 122, https://doi.org/10.1002/2017JB014389.
De Landro et al. 3D ultra-high resolution seismic imaging of shallow Solfatara crater in Campi Flegrei (Italy): New insights on deep hydrothermal fluid circulation processes. Scientific Reports 7, 3412, https://doi.org/10.1038/s41598-017-03604-0 (2017).
Giggenbach, W. F. Relative importance of thermodynamic and kinetic pro-cesses in governing the chemical and isotopic composition of carbon gases in high-heat flow sedimentary basins. Geochim. Cosmochim. Acta 61, 3763–3785 (1997).
Fiebig, J. et al. Chemical and isotopic equilibrium between CO2 and CH4 in fumarolic gas discharges: Generation of CH4 in arc magmatic-hydrothermal systems. Geochim. Cosmochim. Acta 68, 2321–2334 (2004).
Horita, J. Carbon isotope exchange in the system CO2-CH4 at elevated temperatures. Geochimica et Cosmochimica Acta 65, 1907–1919 (2001).
Chiodini, G., Avino, R., Caliro, S. & Minopoli, C. Temperature and pressure gas geoindicators at the Solfatara fumaroles (Campi Flegrei). Annals of Geophysics 54, 151–160 (2011).
Chiodini, G. & Marini, L. Hydrothermal gas equilibria: the H2O-H2-CO2-CO-CH4 system. Geochimica et Cosmochimica Acta 62, 2673–2687 (1998).
Ganguly, J. Thermodynamics in Earth and planetary sciences. Springer-Verlag Berlin Heidelberg, 501 pp. (2008).
Wohletz, K. Heat3D: Magmatic Heat Flow Calculation. Los Alamos National Laboratory, LA-CC-99-27, Los Alamos, New Mexico. (http://geodynamics.lanl.gov/Wohletz/Heat.htm) (1999).
Delaney, P. T. & McTigue, D. F. Volume of magma accumulation or withdrawal estimated from surface uplift or subsidence, with application to the 1960 collapse of Kilauea Volcano. Bulletin of Volcanology 56, 417–424 (1994).
McTigue, D. F. Elastic stress and deformation near a finite spherical magma body: resolution of the point source paradox. Journal of Geophysical Research: Solid Earth 92(B12), 12931–12940 (1987).
Mogi, K. Relations between the eruptions of various volcanoes and the deformations of the ground surfaces around them, Bull. Earthquake Res. Inst. Univ. Tokyo 36, 99–134 (1958).
Acknowledgements
This work was supported by the MIUR Project PON-MONICA. We thank Yuri Taran, three anonymous reviewers and Philip Benson for their constructive comments that greatly improved the manuscript.
Author information
Authors and Affiliations
Contributions
R.M. and F.S. defined the core hypothesis about hydrothermal drying; R.M. performed thermodynamic calculations and the geochemical modelling; R.M., C.T. and G. DeN. exploited the implications about unrest; in particular, G. DeN. and C.T. carried out the modelling of the thermally-derived deformation. All the authors wrote the manuscript. R.M. and F.S. prepared figures.
Corresponding author
Ethics declarations
Competing Interests
The authors declare no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Moretti, R., Troise, C., Sarno, F. et al. Caldera unrest driven by CO2-induced drying of the deep hydrothermal system. Sci Rep 8, 8309 (2018). https://doi.org/10.1038/s41598-018-26610-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41598-018-26610-2
This article is cited by
-
Feedback responses between endogenous and exogenous processes at Campi Flegrei caldera dynamics, Italy
Bulletin of Volcanology (2024)
-
Potential for rupture before eruption at Campi Flegrei caldera, Southern Italy
Communications Earth & Environment (2023)
-
Data analysis of the unsteadily accelerating GPS and seismic records at Campi Flegrei caldera from 2000 to 2020
Scientific Reports (2022)
-
Phreatic and Hydrothermal Eruptions: From Overlooked to Looking Over
Bulletin of Volcanology (2022)
-
Anatomy of the Campi Flegrei caldera using Enhanced Seismic Tomography Models
Scientific Reports (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.