Introduction

Silicene, a silicon-based graphene-like 2-D material1,2, stimulated great interests among the researchers due to its outstanding mechanical and electronic properties3,4,5,6. Silicene is a monolayer of silicon atoms arranged in hexagonal honeycomb lattice where the atoms are not purely in the sp2 hybridised state but share a π bond among them7. Therefore, silicene has an analogous structure to graphene. However, recent studies suggested that the honeycomb lattice structure of silicene is slightly buckled due to sp3 hybridisation, unlike sp2 hybridisation in Graphene8.

Silicene is a zero band gap semiconductor like graphene9,10 and has a tremendous potential in future electronic industry11,12,13. While recent research works have explored the mechanical properties of single crystal silicene14,15,16, the properties of polycrystalline silicene remain poorly understood. Since most of the practical applications involve polycrystalline structure, it makes the comprehensive study of polycrystalline silicene an evident necessity. We note that polycrystalline silicene has not yet been synthesised in laboratory. However, a recent investigation predicted the possibility of polycrystalline structure in the synthesis of silicene17. That spurred a significant interest to investigate the mechanical properties of nc-silicene.

The presence of grains significantly influences mechanical properties of any materials18,19. The grain boundaries (GBs) reduce the strength of a polycrystalline material compared to their single crystal structure20. In polycrystalline ductile materials, the strength as a function of grain size is expressed by the following Hall-Petch relation21,22,

$${\sigma }_{y}={\sigma }_{0}+\frac{{K}_{f}}{\sqrt{d}}.$$
(1)

Here, σ y is the yield strength of the material; σ 0 and K f are the material constants and d is the average grain size. This equation explains an increase in material strength with a decreasing grain size. Interestingly, for ultra-fine grained materials, commonly known as nanocrystalline materials (polycrystal with a grain size less than 100 nm), this effect is inversed, thus contradicts the common notion of “Smaller is stronger”22. This phenomenon is called inverse Hall-Petch effect. Conrad et al.23 reported that the grain size softening or inverse Hall-Petch effect is observed in the nanocrystalline material of grain size less than a critical value. The authors reported a range of 10–50 nm as the critical value for ductile materials. These two phenomena are also observed in 3D ductile materials for their glissile nature of dislocations. Recent studies20,24 have predicted similar phenomena for single atom thick 2D materials like graphene. The underlying physics of these two cases are distinct, and hence for 2D materials, this is called pseudo Hall-Petch behaviour.

The fracture behaviour of single crystal materials with pre-existing crack has been analysed extensively. Due to the stress concentration at the crack tip, the failure always initiates from the crack25,26. Multiple grains in a material add uncertainty to the nature of crack propagation. Often times, nanocrystalline materials with pre-existing cracks may fail far away from the crack, typically at the grain boundary (GB) junction27,28,29. This resistance to crack propagation or failure from the initial crack tip is coined as flaw tolerance of the material. Zhang et al.29 showed that nanocrystalline graphene ribbons can be flaw tolerant when the ribbon width is smaller than a critical value of 17.16 nm. Length scale, besides the presence of grain and crack, also affects properties of any material30,31. Furthermore, the properties, such as fracture toughness of nano-materials considerably differ from their bulk manifestation. Yin et al.32 reported that Griffith criterion, based on continuum theory, is not valid for crack sizes less than 10 nm in graphene. In this regard, molecular dynamics (MD) simulations can be used for a comparative study to quantify the amount of deviation in fracture toughness from that of Griffith’s criterion.

Therefore, the gap in the understanding of the effect of grain size on fracture strength, fracture toughness, and crack propagation of nanocrystalline silicene (nc-silicene) necessitates a comprehensive study. In this article, we investigate the effect of grain size and crack length on the mechanical properties of nc-silicene under uniaxial tensile loading using MD simulations. Furthermore, we compare the fracture toughness of nanocrystalline materials with Griffith’s theory to illustrate its limitation in describing nano-materials’ fracture properties. Finally, our findings show that the failure of nc-silicene can be flaw intolerant (crack sensitive) or flaw tolerant (crack insensitive) depending on the crack and grain size as well as the interaction between the cracks and grains.

Simulation Results and Analysis

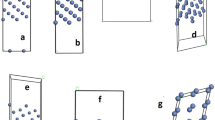
To study the mechanical properties of nc-silicene, a 30 nm × 30 nm nc-silicene sheet with randomly oriented grains is constructed by Voronoi tessellation method33 (see Fig. 1). The grain centres and crystallographic orientations are seeded randomly for each grain structures. Different initial seeds of the grain structures resulted in different sizes of the grains in the material (Details of grain size calculation are in section 1 of Supplementary Information). The size effects of the nc-silicene grains on mechanical properties are studied using average grain sizes of 2.5 nm, 5 nm, 10 nm, 15 nm, 17.32 nm, and 21.2 nm under uniaxial tensile loading at a constant temperature of 300 K. Statistical analysis is performed on 10 different samples for each cases to quantify the uncertainties stemming from the randomness of the grain orientations (see Supplementary Fig. S1 online). The uncertainties are incorporated with error bars in the results presented in this article.

Figure 1
figure 1

Atomic configuration of nanocrystalline silicene with an average grain size of 5 nm. (a) Initial geometry of nanocrystalline silicene sheet of 30 nm × 30 nm size with randomly oriented grains. The grain boundaries are generated by Voronoi tessellation method. Each grain structure is assigned a different colour. (b) After relaxation, the structure shows an out-of-plane deformation due to various defects along the grain boundaries.

Full size image

In order to validate our approach, Young’s moduli of single crystal and nc-silicene are calculated using Stillinger-Weber (SW) potential34. Our calculated Young’s modulus of a single crystal silicene is 85.3 GPa, which is in excellent agreement with the reported literature value of 82.2 GPa35. We also carried out biaxial tensile loading simulations at 300 K on nc-silicene sheet of 48 nm × 48 nm size with an average grain size of 8 nm to validate our method. The Young’s modulus calculated from this simulation is 143.7 GPa which agrees well with the reported value of 136.3 GPa36 by Liu et al. Additional validation of SW potential regarding grain boundary, vacancy formation, edge energy, etc. are provided in Supplementary Information (Tables S1, S2, S3 and Figs S2, S3). Stability of grain boundary is an important aspect in studying properties of nanocrystalline materials. We performed energy minimisation simulations in order to calculate GB Energy and to evaluate the relative stability of various grain sizes considered in this study (see Supplementary Fig. S3 online ). The results suggest an increasing trend in the stability of nc-silicene structures with the increase in the grain sizes and predict 17.32 nm as the most stable grain size. However, the stability decreases with the further increase in the grain size.

Stress vs strain behaviour of single crystal and nc-silicene obtained from tensile test simulations are presented in Fig. 2. The figure reveals that the behaviour of the stress vs strain curve resembles that of 2D polycrystalline graphene sheet obtained from MD simulations24. For nc-silicene, the stress initially increases non-linearly up to a certain strain and thereafter increases linearly with additional strain until the failure. This non-linearity stems from the entropic elastic behaviour of nc-silicene sheet. Initially, there are out-of-plane deformations (wrinkles) in the nc-silicene sheet due to grain boundaries. These out-of-plane deformations are known to improve the stability of the 2D structures37,38. Once the applied stress flattens out the sheet, the bonds of silicene get stretched linearly with the applied stress. We observe brittle failures for both single and nc-silicene. However, there is no significant non-linearity in the elastic behaviour of single crystal silicene.

Figure 2
figure 2

Stress-strain relation of pristine silicene and nc-silicene of different grain sizes under uniaxial tension at 300 K temperature at a loading rate of 109 s−1. The lower gradient of the curves at the early stage is due to the presence of out-of-plane deformations in the initial structure after relaxation.

Full size image

The fracture stress vs grain size data shown in Fig. 3(a) indicate that the fracture stress of nc-silicene has a well-defined relationship to the grain size at a particular temperature. Here, the fracture stress corresponds to the peak stress of the stress-strain curve (Fig. 2), as nc-silicene is found to exhibit a brittle failure. The brittle breaking of nc-silicene resembles the ductile fracture of polycrystalline materials following the Hall-Petch and inverse Hall-Petch relations. However, the original Hall-Petch behaviour in ductile materials initiates from the motion of dislocations and grain boundary sliding, while these phenomena are absent in the case of nc-silicene. The reason can be attributed to the brittle failure of the nc-silicene. In light of these contrasting facts, this behaviour in nc-silicene can be termed as pseudo Hall-Petch and inverse pseudo Hall-Petch behaviour. Fig. 3(a) shows that enlargement of the grain size at nano-scale improves the strength up to a critical grain size and further enlargement causes a reduction in the strength of nc-silicene sheet. The critical grain size is observed as 17.32 nm. Grain boundary energy analysis also supports the conclusion of 17.32 nm as the critical grain size (see Supplementary Fig. S3 online). However, this value depends largely on the orientation of grains and the direction of applied stress with respect to the other grains. The observed pseudo Hall-Petch behaviour can be explained by the stress field created by pentagon-heptagon defects and other dislocations created along the grain boundaries. These dislocations develop a repulsive stress field along the grains or a stress barrier. The applied stress must overcome that barrier in order to initiate a fracture. As the grain size is reduced, the number of dislocations increases (see Fig. 3(d)) which strengthens the sheet. However, this argument cannot alone explain the inverse pseudo Hall-Petch region. To elucidate inverse pseudo Hall-Petch behaviour, we are required to examine the weakest-link model for brittle fracture of a material. The weakest-link model suggests that the failure strength of a brittle material follows a power-law relation with the number of weak links in the material39. The inherent assumption of the weakest-link hypothesis is that a brittle material is comprised of smaller non-interacting sub-structures and the original material fails immediately after the failure of the sub-structure or weakest link. According to this model, the defects along the grain boundaries, especially in the triple junctions, act as a source of crack or void growth or weak links. Fig. 3(c) suggests that as the grain size of the nanocrystalline silicene sheet is decreased, the density of triple junctions increases. By fitting the points of Fig. 3(c), we observe a power-law relation between the densities of triple junctions and grain sizes. As the grain size is reduced below a critical value, that is, into the inverse pseudo Hall-Petch regime, high density of triple junction causes the material to become weaker, thus acting as the weakest-links. In this context, one might argue that triple junctions are also present in the coarse grained structures where physical outcomes are opposite. However, for larger grain sizes, the impact of pentagon and heptagon defects supersedes the effect of triple junctions. We also obtained the values of the constants σ 0 and K f of equation (1) by calculating the slope of the straight line in pseudo inverse Hall-Petch region of Fig. 3(a) and its intercept on the axis of fracture stress respectively. In this way, we have derived the following mathematical equation similar to the Hall-Petch relation as in equation (1) to quantify the fracture stress as a function of grain size:

$${\sigma }_{f}=15.91\,-\,\frac{6.476}{\sqrt{d}},$$
(2)

where, d is in nm and σ f is in GPa. Variation of Young’s modulus with grain size (see Fig. 3(b)) exhibits that Young’s modulus has a positive relationship with the grain size. As the grain size increases, out-of-plane deformations or wrinkles in the sheet are reduced making the sheet stiffer like the brittle materials. Smaller grain size (large number of grains) actually makes the sheet more compliant to the applied load by creating wrinkles in the sheet.

Figure 3
figure 3

Variation of (a) fracture stress, (b) Young’s modulus, (c) density of triple junctions, and (d) number of defects along the grain boundary of nc-silicene with average grain sizes (expressed as d). In Fig. 3(a), fracture stress follows inverse pseudo Hall-Petch relation up to the grain size of 17.32 nm, then shifts into pseudo Hall-Petch region. The “Fitted Curve” in Fig. 3(a) is obtained by fitting the “Data” corresponding to fracture stress.

Full size image

In this study, the pre-cracked sheets of nc-silicene for different crack lengths are also simulated under uniaxial tensile loading in the direction perpendicular to the direction of crack length. The cracks in the nanocrystalline sheets are formed at the centre by removing multiple lines of atoms to maintain the shape of Griffith’s cracks. The variation of fracture stresses and strains with the increasing crack lengths are shown in Fig. 4. These graphs are plotted with an error bar obtained from 10 different samples for each case. This approach allows us to quantify the fluctuations in the fracture stress and strain values due to the randomness of grain boundaries.

Figure 4
figure 4

Variation of (a) fracture stress and (b) fracture strain for different crack lengths of 2a = 1–6 nm under uniaxial tension at 300 K for various grain sizes. Both fracture stress and strain show a decreasing trend with increasing crack lengths.

Full size image

It is evident from Fig. 4 that both fracture stress and strain decrease with the increase of crack length irrespective of the average grain size. The local stress concentration at the crack tip is more prominent for larger crack lengths. Thus, the atoms near the crack tip undergo an irreversible deformation which promotes faster bond breaking at the crack tip. Due to brittle nature of nc-silicene, once a bond is broken, the failure becomes imminent. It is also observed that the fracture stress of pre-cracked sheets reduces with the decrease in grain sizes. Despite the existence of some discrepancies owing to the random GBs, their orientations, and the nature of defects (Heptagon-pentagon defects) in the GBs, the patterns of stress and strain with the variation of crack length are quite consistent in each case.

We note that anomalous decrements in the fracture stress and strains for a few cases with larger pre-cracks are observed. This can be attributed to the position of cracks with respect to the GBs and the interaction between them. When the stress concentration region produced by the grain boundaries intersects the crack in a direction almost perpendicular to the direction of applied loading, the failure initiates faster. Therefore, the actual fracture stress and strain, in some cases, become lower than the expected value.

Our simulation results for pre-cracked nc-silicene sheet clearly indicate a local stress concentration at the crack tip. According to the classical theory of stress concentration, the failure is always expected to be initiated from the crack tip. However, the nc-silicene behaves quite differently. Often times fracture occurs at the crack or at the triple junction of the grain boundaries contradicting the prediction of the classical theory of fracture mechanics. Our findings show that the crack sensitivity and insensitivity depends on the relative size, shape of the crack and grains as well as their interactions. Thus we observe two types of fracture mechanisms in the present study— crack sensitive and crack insensitive fracture.

To verify the suggested mechanisms of fracture of nc-silicene, the normalised tensile strength is calculated, which is defined as29

$$\bar{\sigma }=\frac{{\sigma }_{m}(a)}{{\sigma }_{t}(a)}.$$
(3)

Here, σ m (a) is the strength of nc-silicene without any crack and σ t (a) is the limiting strength of the cracked sheet of nc-silicene, where

$${\sigma }_{t}(a)=S(1\,-\,\varphi ).$$
(4)

Here, S is the strength of the pre-cracked sheet and Φ = W/2a; W is the width of the strip and 2a is the length of the centre crack.

In Fig. 5, the results of normalised strength are shown as a function of different crack lengths for various average grain sizes. Values of the normalised strength closer to 1 indicates the fracture mechanism is crack insensitive. Figure 5 depicts that the failure is more likely to be crack insensitive for smaller crack lengths (2 nm and 1 nm). Furthermore, in our simulations, we observed that for 1 nm and 2 nm crack lengths, failure is crack insensitive for all grain sizes with the exception of sample with 10 nm grain size and 2 nm crack length. Therefore, it is difficult to suggest a critical crack size for crack insensitivity due to the random orientations of grain boundaries (GBs). This randomness in orientation also governs the interaction between GBs and cracks which dictate the failure mechanism. Overall, the trend of the graph in Fig. 5 shows a decrement with the increase of crack length suggesting a more crack sensitive fracture as the normalised strength decreases. Note that, there may be exceptions in the trend for some grain sizes due to the random orientations of the GBs and the interactions of GBs and cracks.

Figure 5
figure 5

The normalised strength of nanocrystalline silicene at different crack lengths for an average grain size of d = 2.5 nm, 5 nm, 10 nm, and 15 nm. Here, σ m denotes the strength of nc-silicene without a crack. σ t is the limiting strength of the cracked sheet of nc-silicene. If the normalised strength is closer to 1, failure for the sample is crack insensitive.

Full size image

To elucidate the mechanism of crack insensitive failure, stress distribution of nc-silicene with average grain size of 15 nm with a crack length of 1 nm is shown in Fig. 6. The figure also presents the process of crack insensitive deformation of nc-silicene. To maintain the crack length, the crack, in this case, is made almost circular, hence blunter than any elliptical crack tip geometry. In Fig. 6(b), the stress concentration region is visible near the crack tip as well as at grain boundaries. However, the stress concentration region near the crack tip remains within the single crystal structure and is also weaker due to the roundness of the crack tip. This single crystal structure is known to be stronger and to break the Si-Si bond, the maximum stress required is reported to be more than 25 GPa40. As a result, despite the presence of a stress concentration region at the crack tip, the maximum atomic stress at the crack tip is much lower than the fracture strength required to break a bond near the circular crack. The circular crack tip also makes the crack propagation energetically less favourable than any elliptical and sharp crack tips. This explains why the failure is not initiated from the crack. On the other hand, the GBs are much weaker than the pristine crystalline structure, and triple junctions are also energetically favourable for rupture with localised atomic stress values exceeding 25 GPa. Therefore, failure is likely to occur from triple junction instead of the crack tip contradicting the classical theory of crack propagation. This physical reasoning justifies the results shown in Fig. 6.

Figure 6
figure 6

Atomistic configurations of crack insensitive fracture. (a) The initial atomic configuration of nc-silicene of 15 nm average grain size with a crack length of 1 nm. (b) Distribution of atomic stress right before the fracture. Here, the blue circle indicates a triple junction which shows higher stress concentration and is weaker than the crack. The black circle indicates that the stress concentration region at the crack tip is inside a pristine crystal (“zoomed-in” views of both the indicated regions are given). (c) Failure from the triple junction. (Another demonstration of crack insensitive fracture in case of nc-silicene of 2.5 nm grain size with 1 nm crack length is shown in the Supplementary Video SV1).

Full size image

From atomistic simulations, it is found that the crack insensitive type of failure is dependent on three conditions related to the relative size and shape of cracks and also on the interaction between the GBs and crack. All of these conditions must have to be fulfilled for the occurrence of crack insensitive fracture. The conditions for crack insensitive failure are: (i) the crack size must be smaller than the average grain size, (ii) the crack must be nearly round shaped, and (iii) the stress concentration region produced by the crack must not interact with the stress concentration region of grain boundaries.

The atomic stress distribution related to the crack sensitive deformation process of nc-silicene with an average grain size of 2.5 nm and a crack length of 4 nm is shown in Fig. 7. The figure indicates a sharper crack tip (sharper than the circular crack geometry), significant stress concentration near the crack tip, and the stress concentration region does not remain localised within a single grain. Therefore, the failure is initiated at the crack tip. Since the crack length is more than the average grain size, the stress concentration region is not localised within the same pristine crystalline structure. This results in a crack sensitive failure. Figure 8 shows another case of crack sensitive failure of nc-silicene sheet with an average grain size and a crack length of 5 nm. Here, the stress concentration region from a grain boundary intersects the stress concentration region of the sharp crack tip, and the intersection occurs in a direction almost perpendicular to the loading direction as shown in Fig. 8 (b). According to previous studies41,42,43, the crack propagates along the crack edges and in the direction perpendicular to the applied load because it is energetically favourable. Since the stress concentration region of the GB interacts with that of the crack almost along the crack edges, it favours the crack propagation in that direction. Moreover, the interaction between stress concentration region of the GB and crack tip increases the intensity of local stress at the crack tip initiating a faster bond breaking than the usual crack sensitive fracture. Further bond breaking near the crack tip renders the tip sharper which speeds up the crack propagation. Therefore, for these cases, failure of pre-cracked nc-silicene occurs at a stress lower than the usual crack sensitive fracture. This phenomenon is also evident in the anomaly in the decrement pattern of strains and stresses with the increase of crack length as shown in the Fig. 4. This irregularity can be attributed to the tendency of GBs to favour the nucleation and propagation of crack tip.

Figure 7
figure 7

Atomistic configurations of crack sensitive fracture. (a) The initial atomic configuration of nanocrystalline silicene of 2.5 nm grain size with 4 nm length of the crack. (b) Distribution of atomic stress before the fracture showing strong stress concentration at crack tip indicated by black circle (“zoomed-in” view is given). (c) Failure from the crack tip.

Full size image
Figure 8
figure 8

Crack sensitive fracture due to overlapping of stress concentration between crack tips and GBs. (a) The initial atomic configuration of nc-silicene of 5 nm grain size with 5 nm crack length. (b) Distribution of atomic stress before the fracture. Here, black circle indicates overlapping of stress concentration region of crack tip and GB (“zoomed-in” view is given). (c) Failure from a crack tip from the side of overlap.

Full size image

The aforementioned analysis implies that crack sensitive failure in nc-silicene occurs under three conditions. If a system satisfies any of these conditions, it becomes crack sensitive. These three conditions are: (i) the crack length is higher than the average grain size, or (ii) the crack is not round shaped, or (iii) the crack is smaller than average grain size, but the stress concentration region produced by GBs interact with the stress concentration region of the crack, especially in the direction of crack edges as shown in Fig. 8.

In our analysis, the samples of nc-silicene with crack length more than 2 nm are found to fulfil any one of the above mentioned conditions. Thus, they experience the crack sensitive fracture phenomenon. To further explore the crack sensitive and insensitive modes of fracture, we simulated the cases demonstrated in Figs 6–8 at lower strain rates of 7.5 × 108 s−1, 5 × 108 s−1 and 108 s−1 to check whether their fracture behaviours remain the same or not (the results are included in Supplementary Figs S5 and S6). The results indicate that the crack sensitive and insensitive fracture are independent of the strain rate.

We have studied the fracture toughness of nc-silicene from both MD simulations and Griffith’s theory. The fracture toughness measures the ability of a pre-cracked material to resist its failure. The theoretical formulation of fracture toughness is made by the classical Griffith’s equation for a central crack44. According to this equation, the critical stress for fracture of a strip with finite central crack is given by-

$${\sigma }_{f}=\frac{1}{f({\rm{\Phi }})}\sqrt{\frac{E{\rm{\Gamma }}}{\pi a}}.$$
(5)

Here, E is the Young’s modulus, Γ is the surface energy for 3D material; edge energy for 2D material; crack formation energy for polycrystalline materials, and a is half of the crack length, and the function f(Ω) is a geometrical factor given by-

$$f({\rm{\Phi }})=[1\,-\,0.025{{\rm{\Phi }}}^{2}+0.06{{\rm{\Phi }}}^{4}]\times {\rm{Sec}}{(\frac{\pi {\rm{\Phi }}}{2})}^{1/2},$$
(6)

where Φ = 2a/W; W is the width of the strip with a central crack of length 2a. The edge energy in equation (5) is the difference of the energy released by the nc-silicene samples with and without cracks. Edge energy is a constant for a nanocrystalline material of the same average grain size, GB and crack orientation irrespective of the crack size45. The calculated edge energies obtained in our analysis for different crack lengths are in close proximity. Therefore, an average value of Γ for all crack lengths is considered. By calculating the edge energy, the fracture toughness is determined using the following equation derived from equation (5)-

$${\sigma }_{f}\sqrt{a}=\frac{1}{f({\rm{\Omega }})}\sqrt{\frac{E{\rm{\Gamma }}}{\pi }}.$$
(7)

Furthermore, the fracture toughness of nc-silicene obtained from MD simulations is calculated by σ f √a, where σ f is the fracture stress of the pre-cracked sheet. The fracture toughness values calculated using Griffith’s equations and MD simulations are presented in Fig. 9 for various crack lengths and average grain sizes. The fracture toughness predicted by MD simulations are plotted with error bars to represent the uncertainties associated with the randomness of GB distributions. The figures show that the MD simulation results deviate significantly from the fracture toughness obtained from Griffith’s equations.

Figure 9
figure 9

Prediction of fracture toughness by Griffith’s criterion and molecular dynamics at different crack lengths for grain size of (a) 2.5 nm, (b) 5 nm, (c) 10 nm, and (d) 15 nm. Fracture toughness obtained by Griffith’s theory is constant for all the crack lengths because the edge energy Γ is averaged for all crack lengths and assumed as constant. So, theoretical fracture toughness is an average prediction.

Full size image

This deviation particularly stems from the limitations of Griffith's theory —which is based on continuum assumptions—in describing nano-crystalline materials. Moreover, the assumption of a perfectly elliptical crack in this theory cannot be maintained in the nc-silicene sheets due to its hexagonal lattice structure. It is also worth mentioning that the Griffith’s theory is governed by the local strength of the atoms at the crack tip, while the MD simulation results focus on global energy balance according to virial stress theorem46. The limitation of the Griffith theory to describe nano-materials has been reported in previous studies. Yin et al. reported the failure of the Griffith’s theory for graphene nano-sheets with a crack size smaller than 10 nm32. In this study, the validity of this theory is also limited for nc-silicene with nano-sized crack. Likewise, Griffith’s theory can be proved to be invalid for most of the 2D nanocrystalline materials due to the lack of plasticity in the fracture of these materials. The absence of plasticity yields no significant change in lattice structure until the failure. This phenomenon leads to intrinsic notch effect which is one of the reasons for the breakdown of Griffith’s theory47. The critical crack size for the limitation of Griffith’s theory in describing nc-silicene will be explored in a future study.

The pseudo inverse Hall-Petch effect depicts that with the increase in average grain size, the fracture stress is increased as we observed in nc-silicene (shown in Fig. 3). It is interesting to note that the fracture toughness as a function of grain size shows the similar trend. Both theoretical and MD simulation results show an increase in fracture toughness with the enlargement of average grain sizes. This observation explains that the cracks in a nanocrystalline material with a larger grain size resist failure more than that of the nanocrystal with a smaller grain size.

Furthermore, in a crack insensitive fracture, the failure does not initiate from a crack tip. Rather, it fails from a triple junction and behaves more like nc-silicene sheet without a crack. According to the definition of fracture toughness, it is the ability of a material to resist crack propagation. Since the crack insensitive fracture demonstrates a fracture mechanism similar to nc-silicene sheet without crack, the ultimate stress of crack insensitive fracture cannot depict fracture toughness. Thus, these are not incorporated in Fig. 9.

It is also important to note that the results of fracture toughness reflect greatly on the anomalous decrement of fracture stress observed in Fig. 4. Due to the position of the crack tip with respect to GB, there is an interaction of stress concentration regions between them. This leads to an increase in the local stress which causes the bond breaking faster than the usual. Therefore, the fracture stress is lowered than the usual value, and as a result, there is also a decrease in the fracture toughness as obtained from our simulations. This phenomenon is evident in the cases of 2.5 nm grain size with 5 nm crack length, 5 nm grain size with 5 nm crack length, and 10 nm grain size with 4 nm crack length, 15 nm grain size with 5 nm crack length.

Discussions

In this study, we have found that the mechanical properties of nc-silicene are contingent on average grain and crack sizes. We also elucidate the failure mechanisms of nc-silicene and demonstrate the deviation of classical theories at nano-scale. Our simulation results reveal that nanocrystalline silicene fails at lower values of ultimate stress than the single crystal silicene due to the presence of grain edges. We observe brittle failures in both single and nc-silicene. The fracture stress of nc-silicene increases with the increased grain size contradicting classical Hall-Petch effect up to a critical grain size. This phenomenon is termed as inverse pseudo Hall-Petch effect. We have  found the critical grain size as 17.32 nm, above which the fracture stress follows the pseudo Hall-Petch effect. Typically, fracture stress and strain show decreasing trends with the increase of crack length. However, in some cases, due to the interaction between stress concentration region of crack and GBs, the failure may occur at a lower stress than the usual values. One of our key findings is that nc-silicene exhibits two types of fracture behaviour, namely, crack insensitive and crack sensitive fracture. In crack insensitive fracture, fracture proceeds from the triple junction of GBs even in the presence of a crack. Crack insensitive fracture occurs when the crack is almost round shaped, the crack length is less than the average grain size and the stress concentration region of GB does not interact with the stress concentration region of the crack. On the other hand, the crack sensitive fracture occurs at the crack tip as predicted by the classical theory. We observe that the fracture is crack sensitive when the crack shape is not round, or its length is more than the average grain size. Furthermore, nc-silicene may also experience crack sensitive failure even if the crack shape is not round or its length is not smaller than the average grain size. This may occur due to the interaction of GB stress concentration region with that of crack, especially in the direction along the crack edges. Finally, we observe that the fracture toughness of nc-silicene calculated from MD simulation deviates from fracture toughness obtained from the Griffith’s theory. This deviation limits the applicability of Griffith’s theory at nano-scale. Griffith’s theory also over-predicts the fracture toughness than that of MD simulations. This prediction is contrary to the case of single crystal silicene because of the higher energy release from the defects at the crack edges in nc-silicene than in single crystal silicene. We believe that this study paves a way to comprehensive and deeper understanding of the mechanical properties and failure mechanism of nc-silicene which is regarded as one of the future materials for manufacturing of nano-electronic devices.

Methods

MD simulation method has been established as an effective way to explore deformation mechanism and fracture of polycrystalline materials at atomic scale48,49,50. In this study, we used an optimised Stillinger-Weber (SW) potential to describe the atomic interactions of silicon atoms. Previously, this potential has been used for describing thermal34 and mechanical properties of single crystal silicene40.The potential is validated against the structural properties calculated by Density Functional Theory (DFT)51. For the details of the validation calculations, see section 3 of the Supplementary Information. The SW potential consists of two terms; a two-body term describing the bond stretching interactions and a three-body term describing the bond breaking interactions. These interactions are expressed as follows

$${\rm{\Phi }}=\sum _{i < j}{V}_{2}+\sum _{i < j < k}{V}_{3},$$
(8)
$${V}_{2}=\varepsilon A(B{{\sigma }_{a}}^{p}{r}_{ij}^{-p}\,-\,{{\sigma }_{a}}^{q}{r}_{ij}^{-q}){e}^{[{\sigma }_{a}{({r}_{ij}-{a}_{1}{\sigma }_{a})}^{-1}]},$$
(9)
$${V}_{3}=\varepsilon \lambda {e}^{[\gamma {\sigma }_{a}{({r}_{ij}-{a}_{1}{\sigma }_{a})}^{-1}+\gamma {\sigma }_{a}{({r}_{jk}-{a}_{1}{\sigma }_{a})}^{-1}]}{(cos{\theta }_{ijk}-cos{\theta }_{0})}^{2},$$
(10)

where, V 2 and V 3 are two-body and three-body terms respectively; r ij is the distance between atoms i and j; σ a is the finite distance at which the inter particle potential is zero; θ ijk is the angle between bond ij and jk; θ 0 is the equilibrium angle between two bonds; other parameters like a 1, A and B are the coefficients required to fit when developing the potential. The values of these parameters are shown in Table 1.

Table 1 Optimized SW potential parameters for silicene. Here, the unit of ε is in electron volts and σ a is in Å. The other parameters are dimensionless and their definitions are given in the paper34.
Full size table

We used LAMMPS software package52 for all the simulations. Periodic boundary conditions are employed for in-plane directions, and the out-of-plane direction is considered as a free surface. The geometries were relaxed by using conjugant gradient minimisation scheme. Then the system was equilibrated using NVE simulations, followed by NPT relaxation at 300 K temperature and atmospheric pressure. The equation of atomic motion was integrated with time step 1 fs. Next, uniaxial stress was applied at a constant strain rate of 109 s−1. We note that the strain rate applied in our simulations is considerably higher than the practical cases. The higher strain facilitates us to explore the material failure mechanisms using a moderate computational resource. We observed an out- of-plane deformation of nc-silicene sheet after the full relaxation as shown in Fig. 1. Under deformation, the atomic stresses are calculated using the following equation46

$${\sigma }_{ij}^{\alpha }=\frac{1}{{{\rm{\Omega }}}^{\alpha }}(\frac{1}{2}{m}^{\alpha }{v}_{i}^{\alpha }{v}_{j}^{\alpha }+\sum _{\beta =1,n}{r}_{\alpha \beta \,}^{j}{f}_{\alpha \beta }^{i}),$$
(11)

where i and j denote indices in the Cartesian coordinate system; α and β are the atomic indices; mα and vα denote the mass and velocity of atom α; rαβ is the distance between atoms α and β; fαβ is the force between atoms α and β; Ωα is the atomic volume of atom α. Zhou53 definitively proved that inclusion of the kinetic term (first term in the right side of equation 11) erroneously calculates the stress and hence in this study kinetic contribution of the atoms to stress is neglected. Then the reported stress during deformation is obtained by averaging the atomic stresses of the system.